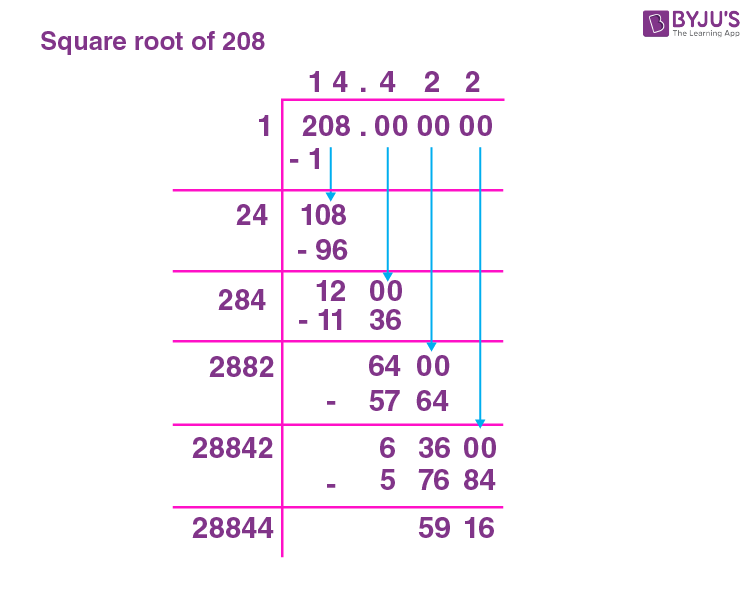
Topic x square root 2: Understanding the concept of x square root 2 is essential for simplifying mathematical expressions and solving various algebraic problems. This article will guide you through the steps and methods to simplify and utilize x square root 2 effectively in your calculations.
Table of Content
- Understanding \( x \sqrt{2} \)
- Introduction to x Square Root 2
- Understanding the Concept of Square Roots
- Properties of the Square Root of 2
- Multiplication and x Square Root 2
- Examples and Applications of x Square Root 2
- Graphical Representation of x Square Root 2
- Common Uses in Geometry and Algebra
- Practical Problems Involving x Square Root 2
- Simplifying Expressions with x Square Root 2
- Conclusion and Further Reading
- YOUTUBE: Căn bậc hai của X bình phương x^2 - Hướng dẫn chi tiết về cách tính căn bậc hai của một số bình phương và ứng dụng trong toán học và khoa học máy tính.
Understanding \( x \sqrt{2} \)
The expression \( x \sqrt{2} \) represents the product of a variable \( x \) and the square root of 2. This is a common form in various mathematical contexts, especially in algebra and geometry. The square root of 2, denoted as \( \sqrt{2} \), is an irrational number approximately equal to 1.414.
Properties of \( \sqrt{2} \)
- It is an irrational number, which means it cannot be expressed as a fraction of two integers.
- Its decimal representation is non-terminating and non-repeating: 1.41421356237...
- It is the positive root of the equation \( x^2 = 2 \).
Multiplication with a Variable \( x \)
When multiplying \( \sqrt{2} \) by a variable \( x \), the properties of the square root function and the variable both apply. The product \( x \sqrt{2} \) scales the value of \( x \) by the factor of \( \sqrt{2} \).
Examples of \( x \sqrt{2} \) in Use
- If \( x = 1 \), then \( x \sqrt{2} = \sqrt{2} \approx 1.414 \).
- If \( x = 2 \), then \( x \sqrt{2} = 2 \sqrt{2} \approx 2 \times 1.414 = 2.828 \).
- If \( x = \frac{1}{\sqrt{2}} \), then \( x \sqrt{2} = \frac{\sqrt{2}}{\sqrt{2}} = 1 \).
Graphical Representation
Graphing the function \( y = x \sqrt{2} \) yields a straight line through the origin with a slope of \( \sqrt{2} \). This is because \( \sqrt{2} \) is a constant multiplier.
Applications in Geometry
In geometry, the expression \( x \sqrt{2} \) frequently appears in calculations involving right triangles and other figures where the diagonal or hypotenuse is involved. For example, in a 45-45-90 triangle, the hypotenuse is \( \sqrt{2} \) times the length of each leg.
Conclusion
Understanding \( x \sqrt{2} \) is essential for various fields of mathematics and science. It is a simple yet powerful expression that shows up in many different contexts, providing a clear example of how irrational numbers and variables interact in mathematical expressions.

READ MORE:
Introduction to x Square Root 2
The concept of "x square root 2" combines algebraic operations with fundamental properties of square roots. Understanding this concept is essential for solving various mathematical problems involving radicals. The square root symbol (√), also known as the radical, represents a value that, when multiplied by itself, yields the original number. For example, √2 is an irrational number approximately equal to 1.414.
In algebra, square roots play a crucial role in simplifying expressions, solving equations, and understanding the properties of numbers. Below, we delve into the details of working with the square root of 2, including its mathematical representation, approximation methods, and practical applications.
- Mathematical Representation: The square root of 2 can be represented as √2. This value is irrational, meaning it cannot be expressed as a simple fraction and has a non-repeating, non-terminating decimal expansion.
- Approximation Methods: One common method to approximate √2 is the iterative method, which uses the formula yn+1 = (x/yn + yn)/2. Starting with an initial guess, this formula converges to the square root of 2 through successive iterations.
- Iteration 1: y1 = (2 + 1)/2 = 1.5
- Iteration 2: y2 = (4/3 + 3/2)/2 ≈ 1.4167
- Iteration 3: y3 = (24/17 + 17/12)/2 ≈ 1.4142
The approximation becomes increasingly accurate with more iterations, converging to the true value of √2.
Square roots also have various practical applications, such as calculating distances, understanding geometric shapes, and solving quadratic equations. For example, the diagonal of a square with side length 1 unit is √2 units, demonstrating how the square root of 2 arises naturally in geometry.
Understanding the Concept of Square Roots
The concept of square roots is fundamental in mathematics, representing a number that, when multiplied by itself, gives the original number. For instance, the square root of 9 is 3, because \(3 \times 3 = 9\). This section explores the principles behind square roots, their properties, and their applications.
- Definition: A square root of a number \(x\) is a number \(r\) such that \(r^2 = x\).
- Positive and Negative Roots: Every positive number \(x\) has two square roots: one positive (the principal square root) and one negative. For example, the square roots of 25 are 5 and -5.
- Principal Square Root: The principal square root is the non-negative root, often denoted by the radical symbol \(\sqrt{}\). For example, \(\sqrt{25} = 5\).
Properties of Square Roots
Square roots have unique properties that make them useful in various mathematical calculations:
- Multiplication: The square root of a product is the product of the square roots. For example, \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\) if \(a \geq 0\) and \(b \geq 0\).
- Division: The square root of a quotient is the quotient of the square roots. For example, \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\) if \(b \neq 0\).
- Exponents: The square root is equivalent to raising a number to the power of one-half. For example, \(\sqrt{a} = a^{1/2}\).
Examples and Applications
Square roots are widely used in geometry, algebra, and various real-world applications:
- Geometry: In geometry, the length of the diagonal of a square can be found using square roots. For a square with side length \(s\), the diagonal length is \(s\sqrt{2}\).
- Algebra: Solving quadratic equations often involves square roots. For example, solving \(x^2 = 16\) gives \(x = \pm 4\).
- Real-world Applications: Square roots are used in calculating areas, volumes, and in various engineering and scientific computations.
Understanding square roots is essential for advancing in mathematics, providing a foundation for more complex topics and practical applications.
Properties of the Square Root of 2
The square root of 2, often represented as \( \sqrt{2} \) or \( 2^{1/2} \), is an important irrational number in mathematics, which means it cannot be expressed as a simple fraction. Its approximate value is 1.41421356, and it has several interesting properties and applications.
- Irrational Number: The square root of 2 is an irrational number, which means it has an infinite number of non-repeating decimal places.
- Geometric Significance: The square root of 2 is the length of the diagonal of a square with side length 1. This relationship is derived from the Pythagorean theorem.
- Historical Context: The discovery of the irrationality of \( \sqrt{2} \) is attributed to the ancient Greeks, particularly the Pythagoreans.
- Continued Fraction: The square root of 2 can be represented as a continued fraction: \( \sqrt{2} = 1 + \frac{1}{2 + \frac{1}{2 + \frac{1}{2 + \ldots}}} \).
- Decimal Expansion: The value of \( \sqrt{2} \) to 50 decimal places is 1.41421356237309504880168872420969807856967187537694...
Understanding the properties of \( \sqrt{2} \) helps in various fields such as geometry, algebra, and number theory. It is also crucial in practical applications like engineering and computer science.
Multiplication and x Square Root 2
The square root of 2, denoted as \( \sqrt{2} \), is an important mathematical constant that often appears in various equations and applications. Understanding how to multiply this constant with other numbers is essential for solving many mathematical problems. Below, we will explore the properties and steps involved in multiplication with \( x \sqrt{2} \).
- Basic Multiplication: When multiplying \( \sqrt{2} \) by a number \( x \), the result is \( x \sqrt{2} \). For example, if \( x = 3 \), then \( 3 \times \sqrt{2} = 3 \sqrt{2} \).
- Square of \( \sqrt{2} \): The square of \( \sqrt{2} \) is 2. This property is useful in simplifying expressions. For instance, \( (\sqrt{2})^2 = 2 \).
- Multiplying Roots: When multiplying \( \sqrt{2} \) with another square root, say \( \sqrt{y} \), the result is \( \sqrt{2y} \). For example, \( \sqrt{2} \times \sqrt{3} = \sqrt{6} \).
Here is a step-by-step example to illustrate these concepts:
- Example: Multiply \( 4 \sqrt{2} \) by \( 3 \sqrt{2} \).
- Step 1: Multiply the coefficients: \( 4 \times 3 = 12 \).
- Step 2: Multiply the square roots: \( \sqrt{2} \times \sqrt{2} = 2 \).
- Step 3: Combine the results: \( 12 \times 2 = 24 \).
Therefore, \( 4 \sqrt{2} \times 3 \sqrt{2} = 24 \).
These steps demonstrate how multiplication involving \( \sqrt{2} \) can be handled with basic arithmetic operations and the properties of square roots.

Examples and Applications of x Square Root 2
The square root of 2, represented as \( \sqrt{2} \), has numerous applications in various fields of mathematics and science. Below are some detailed examples and applications:
- Geometry: The square root of 2 is crucial in geometry, especially when dealing with right-angled triangles. For example, the diagonal of a square with side length \( x \) is \( x \sqrt{2} \).
- Algebra: In algebra, \( \sqrt{2} \) is often used in solving equations involving quadratic roots and irrational numbers. For instance, solving equations like \( x^2 - 2 = 0 \) leads to \( x = \pm \sqrt{2} \).
- Trigonometry: Trigonometric functions can also involve \( \sqrt{2} \). For example, the sine and cosine of 45 degrees are both \( \frac{1}{\sqrt{2}} \) or \( \frac{\sqrt{2}}{2} \).
Let's explore a few practical examples:
| Example | Explanation |
|---|---|
| Geometry | Find the length of the diagonal of a square with side length 5 units: \( 5 \sqrt{2} = 7.071 \) units. |
| Algebra | Solve \( x^2 = 2 \): \( x = \pm \sqrt{2} = \pm 1.414 \). |
| Trigonometry | Calculate \( \sin(45^\circ) \) and \( \cos(45^\circ) \): Both are \( \frac{\sqrt{2}}{2} = 0.707 \). |
In addition to these examples, the square root of 2 plays a significant role in various advanced mathematical concepts and real-world applications. It is an irrational number, which means it cannot be expressed as a simple fraction and its decimal representation goes on infinitely without repeating. This property makes \( \sqrt{2} \) unique and essential in both theoretical and practical contexts.
Graphical Representation of x Square Root 2
The graphical representation of \(x \sqrt{2}\) helps to visualize the relationship between the variable \(x\) and the constant \(\sqrt{2}\). Understanding this graph involves plotting the function \(y = x \sqrt{2}\), which represents a straight line passing through the origin with a slope of \(\sqrt{2}\).
Here are the key steps to plot the function and understand its characteristics:
- Identify the equation of the function: \(y = x \sqrt{2}\).
- Plot the graph by calculating several points. For instance:
- When \(x = 0\), \(y = 0\).
- When \(x = 1\), \(y = \sqrt{2}\).
- When \(x = 2\), \(y = 2\sqrt{2}\).
- When \(x = -1\), \(y = -\sqrt{2}\).
- Draw a straight line through these points, which should extend in both positive and negative directions.
- Analyze the slope, which is constant and equal to \(\sqrt{2}\). This indicates that for every unit increase in \(x\), \(y\) increases by \(\sqrt{2}\).
The graph of \(y = x \sqrt{2}\) is a straight line, showcasing a linear relationship where the slope is the square root of 2. This graphical representation is useful in various mathematical and real-world applications, where proportional relationships involving square roots are studied.
Additionally, understanding the graphical representation allows for better comprehension of the behavior of the function under different transformations and can be used in conjunction with other mathematical tools to solve more complex problems.
Common Uses in Geometry and Algebra
The square root of 2, often denoted as \( \sqrt{2} \), plays a significant role in both geometry and algebra. It appears in various mathematical contexts due to its unique properties. Here, we explore its common uses and applications:
-
Geometry:
-
Pythagorean Theorem: The square root of 2 is crucial in the Pythagorean theorem, which states that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. For a right triangle with legs of length 1, the hypotenuse is \( \sqrt{2} \).
-
Diagonal of a Square: The length of the diagonal of a square with side length \( x \) is \( x \sqrt{2} \). This property is widely used in various geometric constructions and proofs.
-
-
Algebra:
-
Solving Quadratic Equations: The square root of 2 frequently appears in the solutions of quadratic equations, especially when solving by the quadratic formula or completing the square method. For example, the equation \( x^2 = 2 \) has solutions \( x = \pm \sqrt{2} \).
-
Irrational Numbers: The square root of 2 is one of the most well-known irrational numbers. It cannot be expressed as a simple fraction, making it a key example in the study of irrationality in number theory.
-
-
Trigonometry:
-
Special Angles: The square root of 2 is also involved in trigonometric functions of special angles. For instance, \( \sin(45^\circ) \) and \( \cos(45^\circ) \) both equal \( \frac{\sqrt{2}}{2} \).
-
Practical Problems Involving x Square Root 2
In this section, we will explore practical problems that involve the expression \( x \sqrt{2} \). These problems often appear in geometry, algebra, and real-world applications where the properties of square roots and the irrational number \(\sqrt{2}\) play a significant role.
Example 1: Solving Equations
Consider the equation:
\[ x \sqrt{2} = 10 \]
To solve for \( x \), divide both sides by \(\sqrt{2}\):
\[ x = \frac{10}{\sqrt{2}} \]
Rationalizing the denominator:
\[ x = \frac{10 \sqrt{2}}{2} = 5 \sqrt{2} \]
Thus, the solution is \( x = 5 \sqrt{2} \).
Example 2: Geometry - Diagonal of a Square
If a square has a side length of \( x \), the length of the diagonal \( d \) can be calculated using the Pythagorean theorem:
\[ d = x \sqrt{2} \]
For a square with side length \( 7 \) units:
\[ d = 7 \sqrt{2} \]
The diagonal is \( 7 \sqrt{2} \) units long.
Example 3: Simplifying Expressions
Simplify the expression \( 3x \sqrt{2} + 2x \sqrt{2} \):
Combine like terms:
\[ 3x \sqrt{2} + 2x \sqrt{2} = (3 + 2)x \sqrt{2} = 5x \sqrt{2} \]
The simplified expression is \( 5x \sqrt{2} \).
Example 4: Solving a Quadratic Equation
Solve the quadratic equation:
\[ (x \sqrt{2})^2 - 8 = 0 \]
First, rewrite the equation:
\[ 2x^2 - 8 = 0 \]
Add 8 to both sides:
\[ 2x^2 = 8 \]
Divide by 2:
\[ x^2 = 4 \]
Take the square root of both sides:
\[ x = \pm 2 \]
The solutions are \( x = 2 \) and \( x = -2 \).
Example 5: Real-World Application - Construction
In construction, if a rectangular beam needs to be cut such that the diagonal is \( x \sqrt{2} \) meters and the length of the beam is 4 meters, what is the width of the beam?
Using the Pythagorean theorem for the right triangle formed by the length, width, and diagonal:
\[ (4)^2 + w^2 = (x \sqrt{2})^2 \]
\[ 16 + w^2 = 2x^2 \]
If \( x = 3 \), then the equation becomes:
\[ 16 + w^2 = 2(3)^2 \]
\[ 16 + w^2 = 18 \]
Subtract 16 from both sides:
\[ w^2 = 2 \]
Take the square root:
\[ w = \sqrt{2} \]
The width of the beam is \(\sqrt{2}\) meters.
These examples illustrate the diverse applications of \( x \sqrt{2} \) in mathematical problems and real-world scenarios. Understanding how to manipulate and solve equations involving \( x \sqrt{2} \) is essential for various fields, including geometry, algebra, and engineering.
Simplifying Expressions with x Square Root 2
Simplifying expressions that involve the multiplication of x with the square root of 2, \( x\sqrt{2} \), can be straightforward if you follow some basic algebraic rules. Here are a few practical steps and examples to help you understand the process:
Basic Rules
- Product Rule: \( \sqrt{a} \cdot \sqrt{b} = \sqrt{a \cdot b} \)
- Quotient Rule: \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \)
- Combining Like Terms: Combine terms with the same radical part.
Example Problems
Let's look at some examples to illustrate these rules:
- Simplifying a Single Term
Expression: \( x\sqrt{2} \cdot \sqrt{8} \)
Steps:
- Apply the product rule: \( x\sqrt{2} \cdot \sqrt{8} = x\sqrt{2 \cdot 8} = x\sqrt{16} \)
- Simplify the square root: \( x\sqrt{16} = x \cdot 4 = 4x \)
Result: \( 4x \)
- Combining Like Terms
Expression: \( 3x\sqrt{2} + 2x\sqrt{2} \)
Steps:
- Identify like terms: Both terms have the same radical part, \( \sqrt{2} \).
- Combine coefficients: \( (3x + 2x)\sqrt{2} = 5x\sqrt{2} \)
Result: \( 5x\sqrt{2} \)
- Simplifying Complex Expressions
Expression: \( \sqrt{50x^2y^3z} \)
Steps:
- Factor inside the radical to find perfect squares: \( \sqrt{25 \cdot 2 \cdot x^2 \cdot y^2 \cdot y \cdot z} \)
- Separate into product of square roots: \( \sqrt{25} \cdot \sqrt{2} \cdot \sqrt{x^2} \cdot \sqrt{y^2} \cdot \sqrt{y} \cdot \sqrt{z} \)
- Simplify each term: \( 5 \cdot \sqrt{2} \cdot x \cdot y \cdot \sqrt{y} \cdot \sqrt{z} \)
- Combine like terms: \( 5xy \cdot \sqrt{2yz} \)
Result: \( 5xy\sqrt{2yz} \)
Practice Problems
- Simplify \( \sqrt{32x^4y^6} \)
- Combine \( 4x\sqrt{5} + 3x\sqrt{5} \)
- Simplify \( \frac{\sqrt{18x^2}}{\sqrt{2}} \)
Remember to always look for perfect squares within the radicand (the number inside the square root) and to combine like terms where possible. With practice, simplifying these expressions will become second nature.
Conclusion and Further Reading
The concept of x multiplied by the square root of 2 is fundamental in various branches of mathematics, including geometry and algebra. Understanding how to work with this irrational number enhances your ability to solve complex mathematical problems and apply these solutions in practical scenarios.
Throughout this guide, we have explored the definition, properties, and applications of x square root 2. We have seen its relevance in simplifying expressions, solving equations, and even in graphical representations. This thorough exploration highlights the significance of this mathematical concept in both theoretical and practical contexts.
As you continue your mathematical journey, consider the following resources for further reading and deeper understanding:
- - A comprehensive resource for learning algebraic concepts, including operations with square roots.
- - An easy-to-understand explanation of square roots and their properties.
- - Detailed lessons on working with radicals, including multiplication with square roots.
- - Online courses that cover a range of algebraic topics, suitable for all levels of learners.
- - Educational videos explaining the concept of square roots and their applications.
By leveraging these resources, you can deepen your understanding and master the applications of x square root 2 in various mathematical fields.
Căn bậc hai của X bình phương x^2 - Hướng dẫn chi tiết về cách tính căn bậc hai của một số bình phương và ứng dụng trong toán học và khoa học máy tính.
Căn bậc hai của X bình phương x^2
READ MORE:
Câu hỏi cơ bản: Căn bậc hai của X-bình phương - Giải đáp đầy đủ về cách tính căn bậc hai của một số bình phương và các sai lầm phổ biến trong suy nghĩ của học sinh.
Câu hỏi cơ bản: Căn bậc hai của X-bình phương