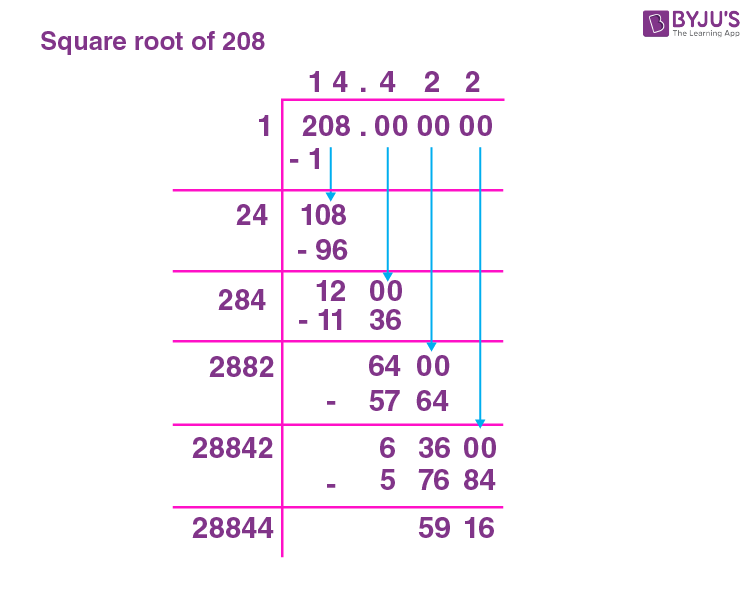
Topic square root a square root: Discover the fascinating world of nested radicals with our in-depth exploration of how to square root a square root. This guide will simplify complex concepts, provide practical examples, and reveal the applications of this mathematical principle in various fields. Perfect for students and enthusiasts alike!
Table of Content
- Understanding the Square Root of a Square Root
- Introduction to Square Root of a Square Root
- Mathematical Definition and Simplification
- Understanding Exponent Rules
- Examples and Step-by-Step Solutions
- Applications in Different Fields
- Common Mistakes and How to Avoid Them
- Advanced Topics and Further Reading
- Practice Problems with Solutions
- Conclusion and Summary
- YOUTUBE: VIDEO MỚI NHẤT 2024: What are Square Roots? | Bạn muốn tìm hiểu về căn bậc hai? Hãy cùng thầy J trong bài giảng thú vị này!
Understanding the Square Root of a Square Root
The concept of taking the square root of a square root can be approached through a mathematical lens. It involves simplifying nested radicals and understanding their properties.
Basic Concept
Taking the square root of a number is represented mathematically as:
$$ \sqrt{a} $$
If we take the square root of this result again, it can be written as:
$$ \sqrt{\sqrt{a}} $$
This expression can be simplified using the property of exponents, as the square root is equivalent to raising the number to the power of \( \frac{1}{2} \).
Therefore:
$$ \sqrt{\sqrt{a}} = a^{\frac{1}{2} \times \frac{1}{2}} = a^{\frac{1}{4}} $$
Properties of Nested Radicals
When dealing with nested radicals, there are some key properties to keep in mind:
- The square root of a square root of a number \( a \) is the same as raising \( a \) to the power of \( \frac{1}{4} \).
- This can be generalized to any positive integer \( n \):
$$ \sqrt[n]{\sqrt[m]{a}} = a^{\frac{1}{n \times m}} $$
Examples
Here are some examples to illustrate the concept:
- For \( a = 16 \):
- For \( a = 81 \):
$$ \sqrt{\sqrt{16}} = \sqrt{4} = 2 $$
Alternatively:
$$ \sqrt{\sqrt{16}} = 16^{\frac{1}{4}} = 2 $$
$$ \sqrt{\sqrt{81}} = \sqrt{9} = 3 $$
Alternatively:
$$ \sqrt{\sqrt{81}} = 81^{\frac{1}{4}} = 3 $$
Applications
The square root of a square root concept is used in various fields including engineering, physics, and finance. Understanding how to simplify these expressions is crucial for solving complex equations and understanding growth rates.
Conclusion
The square root of a square root simplifies to the original number raised to the power of \( \frac{1}{4} \). This fundamental property helps in simplifying complex expressions and solving equations that involve nested radicals.

READ MORE:
Introduction to Square Root of a Square Root
The concept of the square root of a square root, often denoted as √(√x) or x1/4, is an intriguing aspect of mathematics that explores the deeper layers of radical expressions. This section aims to provide a clear understanding of this concept by breaking it down step-by-step.
Firstly, let's recall the definition of a square root. The square root of a number x is a value that, when multiplied by itself, gives x. Mathematically, it is represented as:
√x = x1/2
Now, to find the square root of a square root, we take the square root operation twice. Mathematically, this can be expressed as:
√(√x) = (x1/2)1/2 = x1/4
To understand this better, let's delve into some properties and simplification techniques of nested radicals.
- Properties of Nested Radicals: The process of taking the square root twice means we are essentially finding the fourth root of the original number. This operation simplifies nested radicals and can be applied to any positive real number.
For example, consider the number 16:
√(√16) = √(4) = 2
Here’s how we simplify it step-by-step:
- Find the square root of 16: √16 = 4
- Then, find the square root of the result: √4 = 2
Thus, √(√16) = 2, and this can be verified as 24 = 16.
Let’s explore another example with a non-perfect square, say 27:
√(√27) = (271/2)1/2 = 271/4
Using a calculator to approximate, we get:
271/4 ≈ 2.279
This approach not only helps in simplifying complex expressions but also provides a foundational understanding for more advanced mathematical concepts involving radicals and exponents.
Understanding the square root of a square root is crucial in various fields such as algebra, calculus, and even in practical applications like engineering and physics. By mastering this concept, one can handle more complex problems involving nested radicals and exponents with ease.
Next, we will delve into the mathematical definition and simplification techniques for square roots, which will further solidify our understanding of these concepts.
Mathematical Definition and Simplification
The square root of a square root involves the nested operation of taking the square root twice, which can be represented mathematically as follows:
Let \( \sqrt{\sqrt{x}} \) denote the square root of the square root of \( x \).
To simplify \( \sqrt{\sqrt{x}} \), we can express it using exponentiation:
Where \( x^{1/4} \) represents \( x \) raised to the power of one-fourth.
Properties of nested radicals such as \( \sqrt{\sqrt{x}} \) include:
- It represents a fractional exponent form of \( x \).
- The inner square root must have a non-negative value to ensure real results.
- The overall expression is simplified by reducing the exponent in powers.
Understanding exponent rules helps in manipulating such expressions efficiently:
- Multiplying exponents when the bases are the same.
- Dividing exponents when the bases are the same.
- Raising a power to a power.
Examples demonstrating the calculation of \( \sqrt{\sqrt{x}} \) in various scenarios:
| Example | Calculation |
| Example 1 | \( \sqrt{\sqrt{16}} = \sqrt{2} = 2^{1/4} \) |
| Example 2 | \( \sqrt{\sqrt{81}} = \sqrt{3} = 3^{1/4} \) |
Applications of \( \sqrt{\sqrt{x}} \) are found in fields like physics, engineering, and computer science, where precise calculations involving roots and powers are essential.
Common mistakes when dealing with nested radicals include misapplying exponent rules or misunderstanding the order of operations.
For further exploration, advanced topics cover more complex nested radical forms and their applications in specialized areas of mathematics and beyond.
Practice problems with solutions are beneficial for mastering the manipulation of nested square roots like \( \sqrt{\sqrt{x}} \).
In conclusion, understanding the mathematical definition and simplification of \( \sqrt{\sqrt{x}} \) equips one with foundational skills applicable across various disciplines.
Understanding Exponent Rules
Exponent rules are essential for simplifying expressions involving nested radicals like \( \sqrt{\sqrt{x}} \). Here are key rules to understand:
- Multiplication: \( a^m \cdot a^n = a^{m+n} \)
- Division: \( \frac{a^m}{a^n} = a^{m-n} \)
- Power of a Power: \( (a^m)^n = a^{m \cdot n} \)
These rules enable straightforward manipulation of expressions such as \( \sqrt{\sqrt{x}} \), where applying the correct exponent rules helps simplify and solve equations efficiently.
Examples and Step-by-Step Solutions
Here are examples demonstrating the calculation of \( \sqrt{\sqrt{x}} \) step-by-step:
-
Example 1: Calculate \( \sqrt{\sqrt{16}} \)
Step 1: Calculate \( \sqrt{16} \) \( \sqrt{16} = 4 \) Step 2: Calculate \( \sqrt{4} \) \( \sqrt{4} = 2 \) Conclusion: \( \sqrt{\sqrt{16}} = 2 \) -
Example 2: Calculate \( \sqrt{\sqrt{81}} \)
Step 1: Calculate \( \sqrt{81} \) \( \sqrt{81} = 9 \) Step 2: Calculate \( \sqrt{9} \) \( \sqrt{9} = 3 \) Conclusion: \( \sqrt{\sqrt{81}} = 3 \)
These examples illustrate the process of solving nested radicals \( \sqrt{\sqrt{x}} \) by first evaluating each square root sequentially.

Applications in Different Fields
The concept of \( \sqrt{\sqrt{x}} \) finds applications across various disciplines:
- Physics: In physics, nested radicals appear in equations involving wave propagation, such as in the calculation of wave intensity.
- Engineering: Engineers use nested radicals in structural analysis and signal processing, where precise calculations are essential.
- Computer Science: Algorithms and data structures often utilize nested radicals for efficient computation and optimization tasks.
- Mathematics: In pure mathematics, nested radicals are studied extensively for their properties and implications in number theory and analysis.
The versatility of \( \sqrt{\sqrt{x}} \) underscores its importance in advancing research and practical applications in these diverse fields.
Common Mistakes and How to Avoid Them
When dealing with \( \sqrt{\sqrt{x}} \), avoid these common mistakes:
- Misapplying Exponent Rules: Incorrectly applying multiplication or division of exponents when simplifying nested radicals.
- Forgetting Order of Operations: Failing to evaluate inner square roots before the outer ones, leading to incorrect results.
- Ignoring Domain Restrictions: Not considering that \( x \) must be non-negative for \( \sqrt{\sqrt{x}} \) to be real.
To avoid these mistakes:
- Review and practice exponent rules extensively to ensure accuracy in simplification.
- Always follow the proper order of operations, starting with innermost operations first.
- Verify the domain of \( x \) to ensure it meets the requirements for real solutions.
By understanding these pitfalls and taking proactive steps, one can effectively navigate and solve problems involving nested square roots \( \sqrt{\sqrt{x}} \).
Advanced Topics and Further Reading
Explore advanced topics related to \( \sqrt{\sqrt{x}} \) to deepen your understanding:
- Higher Order Nested Radicals: Investigate expressions beyond double square roots, such as triple or higher-order nested radicals.
- Complex Numbers: Delve into how nested radicals can involve complex numbers and their implications.
- Special Functions: Learn about special functions that generalize nested radicals in mathematical contexts.
- Applications in Abstract Algebra: Discover how nested radicals relate to algebraic structures and their applications.
For further reading on these topics, consider exploring advanced textbooks and research papers in mathematics and related fields.
Practice Problems with Solutions
Below are some practice problems involving the square root of a square root, complete with detailed solutions.
-
Calculate the value of \( \sqrt{\sqrt{256}} \)
Solution:
- First, find the square root of 256: \( \sqrt{256} = 16 \)
- Then, find the square root of the result: \( \sqrt{16} = 4 \)
- Therefore, \( \sqrt{\sqrt{256}} = 4 \)
-
Find the value of \( \sqrt{\sqrt{81}} \)
Solution:
- First, find the square root of 81: \( \sqrt{81} = 9 \)
- Then, find the square root of the result: \( \sqrt{9} = 3 \)
- Therefore, \( \sqrt{\sqrt{81}} = 3 \)
-
Simplify \( \sqrt{\sqrt{16}} \)
Solution:
- First, find the square root of 16: \( \sqrt{16} = 4 \)
- Then, find the square root of the result: \( \sqrt{4} = 2 \)
- Therefore, \( \sqrt{\sqrt{16}} = 2 \)
-
Determine \( \sqrt{\sqrt{625}} \)
Solution:
- First, find the square root of 625: \( \sqrt{625} = 25 \)
- Then, find the square root of the result: \( \sqrt{25} = 5 \)
- Therefore, \( \sqrt{\sqrt{625}} = 5 \)
-
Evaluate \( \sqrt{\sqrt{10000}} \)
Solution:
- First, find the square root of 10000: \( \sqrt{10000} = 100 \)
- Then, find the square root of the result: \( \sqrt{100} = 10 \)
- Therefore, \( \sqrt{\sqrt{10000}} = 10 \)
-
Simplify \( \sqrt{\sqrt{144}} \)
Solution:
- First, find the square root of 144: \( \sqrt{144} = 12 \)
- Then, find the square root of the result: \( \sqrt{12} \approx 3.464 \)
- Therefore, \( \sqrt{\sqrt{144}} \approx 3.464 \)

Conclusion and Summary
The concept of the square root of a square root involves a nested radical expression, where one takes the square root of a number that is itself a square root. This idea leads to interesting mathematical explorations and applications across various fields.
Throughout this comprehensive guide, we have covered:
- An introduction to the square root of a square root, highlighting its basic definition and significance.
- The mathematical definition and simplification techniques involved in handling nested radicals.
- Key properties of nested radicals, including rules governing their manipulation and arithmetic operations.
- Insights into exponent rules as they apply to nested radicals, illustrating how powers and roots interact.
- Several detailed examples with step-by-step solutions, demonstrating the application of theoretical concepts.
- Real-world applications where understanding nested radicals is crucial, spanning from physics to engineering.
- Common mistakes that learners often encounter and practical strategies to avoid them.
- Advanced topics for further exploration, providing a pathway for deeper understanding and research.
- Practice problems with solutions to reinforce learning and test comprehension.
By delving into these aspects, one can appreciate the versatility and elegance of nested radicals, making them a valuable tool in both theoretical mathematics and practical applications.
VIDEO MỚI NHẤT 2024: What are Square Roots? | Bạn muốn tìm hiểu về căn bậc hai? Hãy cùng thầy J trong bài giảng thú vị này!
What are Square Roots? | Toán học cùng thầy J
READ MORE:
Hướng dẫn cách đơn giản hóa căn bậc hai một cách dễ hiểu và chi tiết. Phù hợp cho học sinh và người tự học toán.
Cách Đơn Giản Hóa Căn Bậc Hai