Topic x b+-square root b2-4ac 2a: Unlock the secrets of solving quadratic equations with the quadratic formula: x b+-square root b2-4ac 2a. This comprehensive guide will take you through each step, making the complex simple. Master the quadratic formula and confidently solve any quadratic equation with ease and precision.
Table of Content
- Quadratic Formula
- Introduction to Quadratic Equations
- The Quadratic Formula Explained
- Derivation of the Quadratic Formula
- Steps to Solve Quadratic Equations Using the Quadratic Formula
- Understanding the Discriminant
- Examples of Solving Quadratic Equations
- Special Cases in Quadratic Equations
- Applications of Quadratic Equations
- Common Mistakes to Avoid
- Practice Problems and Solutions
- Conclusion and Summary
- Additional Resources
- YOUTUBE:
Quadratic Formula
The quadratic formula is used to find the roots of a quadratic equation of the form:
The roots can be found using the quadratic formula:
Steps to Solve Using the Quadratic Formula
- Identify the coefficients , , and in the quadratic equation.
- Substitute these values into the quadratic formula.
- Calculate the value under the square root (the discriminant): .
- Evaluate the square root of the discriminant.
- Perform the arithmetic to find the two potential values for .
Example
Given the quadratic equation , solve for :
Here, , , and .
Substituting into the quadratic formula:
Simplifying inside the square root:
Since , we have:
So the solutions are:
Thus, the solutions to the quadratic equation are and .

READ MORE:
Introduction to Quadratic Equations
Quadratic equations are polynomial equations of degree two, typically in the form of \( ax^2 + bx + c = 0 \), where \( a \), \( b \), and \( c \) are constants and \( a \neq 0 \). These equations are fundamental in algebra and appear frequently in various areas of mathematics and applied sciences.
The solutions to quadratic equations, also known as roots, can be found using several methods, one of the most popular being the quadratic formula:
\[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \]
This formula provides the solutions to any quadratic equation by substituting the values of \( a \), \( b \), and \( c \) into the equation. The term inside the square root, \( b^2 - 4ac \), is known as the discriminant and it determines the nature of the roots:
- If the discriminant is positive, the equation has two distinct real roots.
- If the discriminant is zero, the equation has exactly one real root (a repeated root).
- If the discriminant is negative, the equation has two complex roots.
Let's consider a simple example to illustrate the use of the quadratic formula. Suppose we have the quadratic equation:
\[ 2x^2 - 4x - 6 = 0 \]
Here, \( a = 2 \), \( b = -4 \), and \( c = -6 \). Plugging these values into the quadratic formula, we get:
\[ x = \frac{-(-4) \pm \sqrt{(-4)^2 - 4 \cdot 2 \cdot (-6)}}{2 \cdot 2} \]
\[ x = \frac{4 \pm \sqrt{16 + 48}}{4} \]
\[ x = \frac{4 \pm \sqrt{64}}{4} \]
\[ x = \frac{4 \pm 8}{4} \]
This results in two solutions:
\[ x = \frac{4 + 8}{4} = 3 \]
\[ x = \frac{4 - 8}{4} = -1 \]
Thus, the solutions to the equation \( 2x^2 - 4x - 6 = 0 \) are \( x = 3 \) and \( x = -1 \).
Understanding and solving quadratic equations is crucial for progressing in algebra and serves as a foundation for more advanced mathematical concepts and problem-solving techniques.
The Quadratic Formula Explained
The quadratic formula is a fundamental tool for solving quadratic equations of the form \( ax^2 + bx + c = 0 \), where \( a \), \( b \), and \( c \) are constants and \( a \neq 0 \).
It is expressed as:
This formula provides the solutions, or roots, of the quadratic equation. Here’s how it works:
- Identify the coefficients \( a \), \( b \), and \( c \) from the quadratic equation \( ax^2 + bx + c = 0 \).
- Substitute these values into the quadratic formula.
- Calculate the discriminant \( \Delta = b^2 - 4ac \).
- Determine the nature of the roots based on the discriminant:
- If \( \Delta > 0 \), there are two distinct real roots.
- If \( \Delta = 0 \), there is one real root (a repeated root).
- If \( \Delta < 0 \), there are two complex roots (conjugate pairs).
- Apply the quadratic formula to find the solutions:
- For \( \Delta > 0 \): \( x = \frac{{-b \pm \sqrt{\Delta}}}{{2a}} \).
- For \( \Delta = 0 \): \( x = \frac{{-b}}{{2a}} \).
- For \( \Delta < 0 \): \( x = \frac{{-b \pm i \sqrt{|\Delta|}}}{{2a}} \), where \( i \) is the imaginary unit.
- Verify the solutions by substituting them back into the original equation to ensure correctness.
The quadratic formula is versatile and applicable across various fields, including physics, engineering, and economics, where quadratic equations model real-world phenomena.
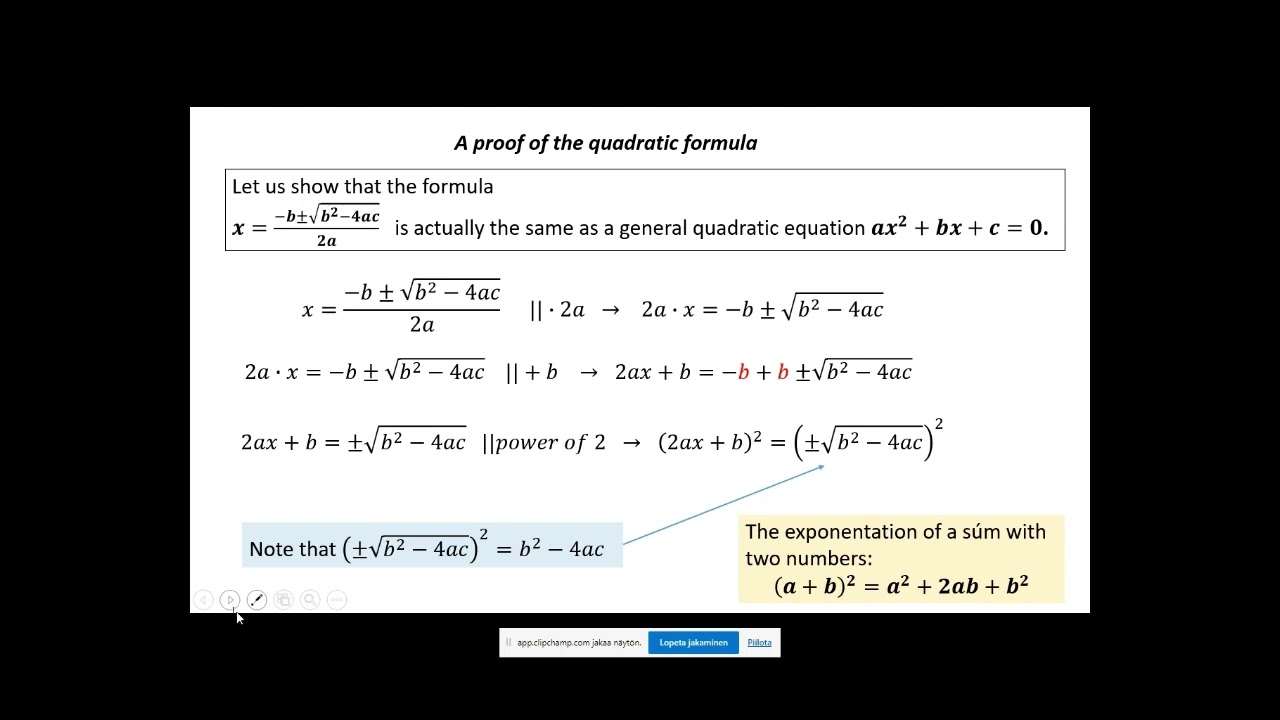
Derivation of the Quadratic Formula
The quadratic formula is a powerful tool for solving quadratic equations of the form ax2 + bx + c = 0. The derivation of this formula involves completing the square. Below are the detailed steps to derive the quadratic formula:
- Start with the standard form of a quadratic equation:
ax2 + bx + c = 0
- Divide every term by a to normalize the equation:
x2 + (b/a)x + c/a = 0
- Subtract c/a from both sides to isolate the quadratic and linear terms:
x2 + (b/a)x = -c/a
- To complete the square, add and subtract (b/2a)2 on the left side:
x2 + (b/a)x + (b/2a)2 - (b/2a)2 = -c/a
x2 + (b/a)x + (b/2a)2 = -c/a + (b/2a)2
- Rewrite the left side as a perfect square:
(x + b/2a)2 = -c/a + b2/4a2
- Combine the terms on the right side over a common denominator:
(x + b/2a)2 = (b2 - 4ac) / 4a2
- Take the square root of both sides:
x + b/2a = ±√(b2 - 4ac) / 2a
- Solve for x by isolating it on one side:
x = -b/2a ± √(b2 - 4ac) / 2a
- Combine the terms over a common denominator to get the quadratic formula:
x = (-b ± √(b2 - 4ac)) / 2a
This is the quadratic formula, which provides the solutions to any quadratic equation of the form ax2 + bx + c = 0.
Steps to Solve Quadratic Equations Using the Quadratic Formula
The quadratic formula is used to find the solutions of a quadratic equation in the form \(ax^2 + bx + c = 0\). The formula is:
\[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \]
Here are the detailed steps to solve a quadratic equation using the quadratic formula:
- Identify the coefficients: In the quadratic equation \(ax^2 + bx + c = 0\), identify the coefficients \(a\), \(b\), and \(c\).
- Calculate the discriminant: Compute the discriminant using the formula \(b^2 - 4ac\). This will determine the nature of the roots.
- If the discriminant is positive (\(b^2 - 4ac > 0\)), there are two real and distinct roots.
- If the discriminant is zero (\(b^2 - 4ac = 0\)), there is one real and repeated root.
- If the discriminant is negative (\(b^2 - 4ac < 0\)), there are two complex roots.
- Apply the quadratic formula: Substitute the values of \(a\), \(b\), and the discriminant into the quadratic formula.
- For two real roots:
\[ x_1 = \frac{-b + \sqrt{b^2 - 4ac}}{2a} \]
\[ x_2 = \frac{-b - \sqrt{b^2 - 4ac}}{2a} \] - For one real root:
\[ x = \frac{-b}{2a} \] - For two complex roots:
\[ x_1 = \frac{-b + \sqrt{b^2 - 4ac} \cdot i}{2a} \]
\[ x_2 = \frac{-b - \sqrt{b^2 - 4ac} \cdot i}{2a} \]
- For two real roots:
- Simplify the results: Simplify the results to get the final solutions for \(x\).
Let's consider an example:
Example: Solve \(2x^2 - 4x - 6 = 0\)
- Identify the coefficients: \(a = 2\), \(b = -4\), \(c = -6\)
- Calculate the discriminant: \(b^2 - 4ac = (-4)^2 - 4(2)(-6) = 16 + 48 = 64\)
- Apply the quadratic formula:
\[ x = \frac{-(-4) \pm \sqrt{64}}{2(2)} \]
\[ x = \frac{4 \pm 8}{4} \]
\]
\]- For \(x_1\):
\[ x_1 = \frac{4 + 8}{4} = \frac{12}{4} = 3 \] - For \(x_2\):
\[ x_2 = \frac{4 - 8}{4} = \frac{-4}{4} = -1 \]
- For \(x_1\):
Therefore, the solutions are \(x = 3\) and \(x = -1\).
Understanding the Discriminant
The discriminant is a crucial component in the quadratic formula, which helps determine the nature and number of roots for a given quadratic equation of the form \(ax^2 + bx + c = 0\). The quadratic formula is given by:
\[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \]
The discriminant is the expression under the square root in the quadratic formula, represented as:
\[ \Delta = b^2 - 4ac \]
The value of the discriminant \(\Delta\) indicates the nature of the roots of the quadratic equation:
- If \(\Delta > 0\), the quadratic equation has two distinct real roots.
- If \(\Delta = 0\), the quadratic equation has exactly one real root (also known as a repeated or double root).
- If \(\Delta < 0\), the quadratic equation has no real roots but two complex conjugate roots.
Understanding the discriminant is essential because it not only tells us the number of roots but also provides insight into the behavior of the graph of the quadratic function \(f(x) = ax^2 + bx + c\):
- A positive discriminant (\(\Delta > 0\)) means the parabola intersects the x-axis at two points.
- A zero discriminant (\(\Delta = 0\)) means the parabola touches the x-axis at exactly one point (vertex).
- A negative discriminant (\(\Delta < 0\)) means the parabola does not intersect the x-axis, implying the roots are complex.
Here are examples to illustrate different cases:
- Positive Discriminant: For \(x^2 - 3x + 2 = 0\), the discriminant \(\Delta = 1\). The equation has two real roots, \(x = 1\) and \(x = 2\).
- Zero Discriminant: For \(x^2 - 2x + 1 = 0\), the discriminant \(\Delta = 0\). The equation has one real root, \(x = 1\).
- Negative Discriminant: For \(x^2 + x + 1 = 0\), the discriminant \(\Delta = -3\). The equation has two complex roots, \(x = -\frac{1}{2} + \frac{\sqrt{3}}{2}i\) and \(x = -\frac{1}{2} - \frac{\sqrt{3}}{2}i\).
By examining the discriminant, one can quickly assess the number and type of solutions without solving the entire quadratic equation. This insight is valuable for both theoretical and practical applications, such as in physics, engineering, and economics, where understanding the nature of the roots can inform decisions and interpretations of models.
Examples of Solving Quadratic Equations
Let's walk through some examples of solving quadratic equations using the quadratic formula:
- Solve \( 2x^2 - 5x + 3 = 0 \)
- Solve \( x^2 + 4x + 4 = 0 \)
- Solve \( 3x^2 + 2x - 7 = 0 \)
| Given equation: | \( 2x^2 - 5x + 3 = 0 \) |
| Identify coefficients: | \( a = 2, b = -5, c = 3 \) |
| Calculate discriminant: | \( \Delta = b^2 - 4ac = (-5)^2 - 4 \cdot 2 \cdot 3 = 25 - 24 = 1 \) |
| Apply quadratic formula: | \( x = \frac{{-b \pm \sqrt{\Delta}}}{{2a}} = \frac{{5 \pm \sqrt{1}}}{{4}} \) |
| Solutions: | \( x = \frac{{5 + 1}}{{4}} = 1 \) or \( x = \frac{{5 - 1}}{{4}} = \frac{{4}}{{4}} = 1 \) |
| Given equation: | \( x^2 + 4x + 4 = 0 \) |
| Identify coefficients: | \( a = 1, b = 4, c = 4 \) |
| Calculate discriminant: | \( \Delta = b^2 - 4ac = 4^2 - 4 \cdot 1 \cdot 4 = 16 - 16 = 0 \) |
| Apply quadratic formula: | \( x = \frac{{-b \pm \sqrt{\Delta}}}{{2a}} = \frac{{-4 \pm \sqrt{0}}}{{2 \cdot 1}} \) |
| Solution: | \( x = \frac{{-4}}{{2}} = -2 \) |
| Given equation: | \( 3x^2 + 2x - 7 = 0 \) |
| Identify coefficients: | \( a = 3, b = 2, c = -7 \) |
| Calculate discriminant: | \( \Delta = b^2 - 4ac = 2^2 - 4 \cdot 3 \cdot (-7) = 4 + 84 = 88 \) |
| Apply quadratic formula: | \( x = \frac{{-b \pm \sqrt{\Delta}}}{{2a}} = \frac{{-2 \pm \sqrt{88}}}{{6}} \) |
| Solutions: | \( x = \frac{{-2 + \sqrt{88}}}{{6}} \) or \( x = \frac{{-2 - \sqrt{88}}}{{6}} \) |
Special Cases in Quadratic Equations
Quadratic equations can present unique scenarios that require special consideration. Below, we explore these special cases, providing detailed steps and examples for each:
Case 1: Perfect Square Trinomials
A quadratic equation is a perfect square trinomial if it can be factored into a binomial squared. The general form is:
Example:
Case 2: Zero Discriminant
If the discriminant of a quadratic equation is zero, the equation has exactly one real root (a repeated root). The discriminant (\(\Delta\)) is calculated as:
When \(\Delta = 0\):
Example:
Case 3: Negative Discriminant
If the discriminant is negative, the quadratic equation has no real roots, but two complex roots. The roots are calculated using:
Example:
Here, \(\Delta = 4 - 20 = -16\), so the roots are:
Case 4: Zero Leading Coefficient
If the leading coefficient \(a = 0\), the equation is no longer quadratic but linear. Solve as follows:
Example:
Applications of Quadratic Equations
Quadratic equations have a wide range of applications in various fields. Here are some of the most common applications:
- Physics and Engineering: Quadratic equations are often used to model physical phenomena such as the motion of objects under the influence of gravity, where the path of a projectile follows a parabolic trajectory.
- Finance: In finance, quadratic equations can be used to calculate the maximum profit or minimum cost. For example, they are used in modeling revenue and cost functions where the profit function is quadratic.
- Biology: Quadratic equations are used to model population growth where the rate of reproduction and mortality might follow a quadratic pattern.
- Chemistry: In chemistry, quadratic equations can be used to determine the concentrations of reactants and products at equilibrium in chemical reactions.
- Astronomy: Quadratic equations help in calculating the orbits of celestial bodies and the shape of their paths, which are often elliptical and can be described by quadratic equations.
Here are a few specific examples illustrating these applications:
1. Projectile Motion in Physics
The height \(h\) of an object in projectile motion can be described by the equation:
\[ h(t) = -\frac{1}{2}gt^2 + v_0t + h_0 \]
where \( g \) is the acceleration due to gravity, \( v_0 \) is the initial velocity, and \( h_0 \) is the initial height. Solving for \( t \) when \( h(t) = 0 \) gives the time when the object hits the ground.
2. Profit Maximization in Business
The profit \( P \) can be modeled by a quadratic equation:
\[ P(x) = ax^2 + bx + c \]
where \( x \) is the number of units produced and sold, and \( a \), \( b \), and \( c \) are constants. The vertex of the parabola given by this equation provides the maximum profit point.
3. Population Dynamics in Biology
The population \( P \) at time \( t \) can sometimes be modeled as:
\[ P(t) = at^2 + bt + c \]
This quadratic equation helps in understanding how the population changes over time under certain conditions.
4. Chemical Equilibrium
The concentrations of substances in a chemical reaction at equilibrium can be determined using the quadratic equation derived from the equilibrium constant expression.
For a reaction \( aA + bB \rightleftharpoons cC + dD \), the equilibrium concentrations can be found by solving the equation:
\[ K_{eq} = \frac{[C]^c [D]^d}{[A]^a [B]^b} \]
5. Orbital Mechanics in Astronomy
The path of a planet or satellite can be described by a quadratic equation of the form:
\[ r(\theta) = \frac{a(1-e^2)}{1+e\cos\theta} \]
where \( r \) is the radius, \( \theta \) is the angle, \( a \) is the semi-major axis, and \( e \) is the eccentricity of the orbit.
These examples demonstrate the versatility and importance of quadratic equations in modeling and solving real-world problems across different disciplines.

Common Mistakes to Avoid
When solving quadratic equations using the quadratic formula, several common mistakes can hinder accurate solutions. Here are the most frequent errors and tips to avoid them:
- Misidentifying Coefficients:
Ensure that you correctly identify the coefficients \(a\), \(b\), and \(c\) from the quadratic equation \(ax^2 + bx + c = 0\). Mixing up these values can lead to incorrect solutions.
- Incorrect Discriminant Calculation:
The discriminant, \(b^2 - 4ac\), determines the nature of the roots. Errors in this calculation can lead to wrong conclusions about the type and number of roots. Always double-check your calculations.
- Sign Errors:
Pay close attention to the signs in the quadratic formula \(x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\). A common mistake is to mix up the plus and minus signs, which can drastically alter the result.
- Overlooking Complex Roots:
When the discriminant is negative, the quadratic equation has complex roots. Ensure that you recognize this scenario and solve for the complex roots appropriately.
- Incorrectly Simplifying the Square Root:
Ensure that you simplify the square root of the discriminant correctly. Missteps here can lead to incorrect roots.
- Arithmetic Errors:
Basic arithmetic mistakes, such as errors in addition, subtraction, multiplication, or division, can lead to incorrect solutions. Carefully verify each step of your calculations.
- Application Misunderstanding:
Remember that the quadratic formula is just one method to solve quadratic equations. Sometimes, factoring or completing the square might be more efficient depending on the problem.
By being mindful of these common mistakes, you can enhance your accuracy and confidence in solving quadratic equations using the quadratic formula.
Practice Problems and Solutions
Below are several practice problems to help you master solving quadratic equations using the quadratic formula. Each problem is followed by a detailed solution.
Problem 1
Solve the quadratic equation \( x^2 - 3x - 10 = 0 \) using the quadratic formula.
Solution:
- Identify the coefficients: \( a = 1 \), \( b = -3 \), \( c = -10 \).
- Substitute these values into the quadratic formula: \[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \] \[ x = \frac{-(-3) \pm \sqrt{(-3)^2 - 4 \cdot 1 \cdot (-10)}}{2 \cdot 1} \] \[ x = \frac{3 \pm \sqrt{9 + 40}}{2} \] \[ x = \frac{3 \pm \sqrt{49}}{2} \] \[ x = \frac{3 \pm 7}{2} \]
- Simplify to find the roots: \[ x = \frac{3 + 7}{2} = 5 \quad \text{and} \quad x = \frac{3 - 7}{2} = -2 \]
The solutions are \( x = 5 \) and \( x = -2 \).
Problem 2
Solve the quadratic equation \( 2x^2 + 4x - 6 = 0 \) using the quadratic formula.
Solution:
- Identify the coefficients: \( a = 2 \), \( b = 4 \), \( c = -6 \).
- Substitute these values into the quadratic formula: \[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \] \[ x = \frac{-4 \pm \sqrt{4^2 - 4 \cdot 2 \cdot (-6)}}{2 \cdot 2} \] \[ x = \frac{-4 \pm \sqrt{16 + 48}}{4} \] \[ x = \frac{-4 \pm \sqrt{64}}{4} \] \[ x = \frac{-4 \pm 8}{4} \]
- Simplify to find the roots: \[ x = \frac{-4 + 8}{4} = 1 \quad \text{and} \quad x = \frac{-4 - 8}{4} = -3 \]
The solutions are \( x = 1 \) and \( x = -3 \).
Problem 3
Solve the quadratic equation \( x^2 + 4x + 5 = 0 \) using the quadratic formula.
Solution:
- Identify the coefficients: \( a = 1 \), \( b = 4 \), \( c = 5 \).
- Substitute these values into the quadratic formula: \[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \] \[ x = \frac{-4 \pm \sqrt{4^2 - 4 \cdot 1 \cdot 5}}{2 \cdot 1} \] \[ x = \frac{-4 \pm \sqrt{16 - 20}}{2} \] \[ x = \frac{-4 \pm \sqrt{-4}}{2} \] \[ x = \frac{-4 \pm 2i}{2} \]
- Simplify to find the roots: \[ x = \frac{-4 + 2i}{2} = -2 + i \quad \text{and} \quad x = \frac{-4 - 2i}{2} = -2 - i \]
The solutions are \( x = -2 + i \) and \( x = -2 - i \).
Problem 4
Solve the quadratic equation \( 3x^2 - 2x - 8 = 0 \) using the quadratic formula.
Solution:
- Identify the coefficients: \( a = 3 \), \( b = -2 \), \( c = -8 \).
- Substitute these values into the quadratic formula: \[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \] \[ x = \frac{2 \pm \sqrt{(-2)^2 - 4 \cdot 3 \cdot (-8)}}{2 \cdot 3} \] \[ x = \frac{2 \pm \sqrt{4 + 96}}{6} \] \[ x = \frac{2 \pm \sqrt{100}}{6} \] \[ x = \frac{2 \pm 10}{6} \]
- Simplify to find the roots: \[ x = \frac{2 + 10}{6} = 2 \quad \text{and} \quad x = \frac{2 - 10}{6} = -\frac{4}{3} \]
The solutions are \( x = 2 \) and \( x = -\frac{4}{3} \).
Problem 5
Solve the quadratic equation \( x^2 + 6x + 9 = 0 \) using the quadratic formula.
Solution:
- Identify the coefficients: \( a = 1 \), \( b = 6 \), \( c = 9 \).
- Substitute these values into the quadratic formula: \[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \] \[ x = \frac{-6 \pm \sqrt{6^2 - 4 \cdot 1 \cdot 9}}{2 \cdot 1} \] \[ x = \frac{-6 \pm \sqrt{36 - 36}}{2} \] \[ x = \frac{-6 \pm 0}{2} \]
- Simplify to find the root: \[ x = \frac{-6}{2} = -3 \]
The solution is \( x = -3 \). Since the discriminant is zero, there is only one root.
Conclusion and Summary
The quadratic formula, \( x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \), is a powerful tool for solving quadratic equations. It offers a straightforward method to find the roots of any quadratic equation of the form \( ax^2 + bx + c = 0 \).
-
Derivation and Application:
The quadratic formula is derived from the process of completing the square. It provides a universal solution to all quadratic equations, regardless of their complexity. By substituting the coefficients \( a \), \( b \), and \( c \) into the formula, we can calculate the solutions efficiently.
-
Understanding the Discriminant:
The discriminant, \( \Delta = b^2 - 4ac \), plays a crucial role in determining the nature of the roots:
- If \( \Delta > 0 \), there are two distinct real roots.
- If \( \Delta = 0 \), there is exactly one real root (repeated).
- If \( \Delta < 0 \), the equation has two complex roots.
-
Common Mistakes:When using the quadratic formula, it is important to avoid common mistakes such as incorrect arithmetic operations, neglecting the \( \pm \) symbol, and misinterpreting the discriminant.
-
Practical Applications:
Quadratic equations are not just theoretical constructs but have practical applications in various fields such as physics, engineering, economics, and biology. They can be used to model phenomena ranging from projectile motion to optimization problems.
In summary, mastering the quadratic formula and understanding the role of the discriminant are essential for solving quadratic equations effectively. With practice, these tools can become invaluable in both academic and real-world problem-solving scenarios.
Additional Resources
For those looking to deepen their understanding of quadratic equations and the quadratic formula, here are some valuable resources:
-
Brilliant Math & Science Wiki:
This resource provides detailed explanations and proofs of the quadratic formula, including various methods such as completing the square and substitution. It is excellent for students who want to understand the derivation of the formula.
Visit the website:
-
Khan Academy:
Khan Academy offers comprehensive tutorials on quadratic equations, the quadratic formula, and the discriminant. Their interactive exercises and videos are perfect for learners who prefer visual and hands-on learning.
Explore more at:
-
Math.net:
Math.net provides concise explanations and examples of solving quadratic equations using the quadratic formula. They also offer tips on remembering the formula and understanding its geometric interpretation.
Learn more at:
-
Wolfram Alpha:
Wolfram Alpha is an excellent tool for solving quadratic equations. You can input any quadratic equation, and it will not only solve it but also show the steps involved in the solution.
Try it out:
These resources provide a wealth of information and practice opportunities to master the quadratic formula and its applications.

Nếu α, β, γ, δ là các nghiệm của phương trình x^4 - Kx^3 + Kx^2 + Lx + M = 0, nơi K, L & M là số thực
READ MORE:
TOÁN 9 - PHƯƠNG TRÌNH BẬC HAI