Topic square root of 2 in fraction form: The square root of 2 in fraction form is a fascinating concept in mathematics, offering both historical intrigue and practical applications. This article explores various fractional approximations of \(\sqrt{2}\), delving into its mathematical significance, continued fraction representations, and its role in different mathematical fields. Discover how this irrational number can be closely approximated using fractions.
Table of Content
- Square Root of 2 in Fraction Form
- Introduction to the Square Root of 2
- Understanding Irrational Numbers
- Historical Context of the Square Root of 2
- Mathematical Definition of the Square Root of 2
- Approximations of the Square Root of 2
- Simple Fractional Approximations
- Advanced Fractional Approximations
- Decimal Approximations
- Geometric Interpretations
- Applications of the Square Root of 2
- Square Root of 2 in Different Mathematical Fields
- Proofs of the Irrationality of the Square Root of 2
- Computational Methods for Approximating the Square Root of 2
- Visual Representations of the Square Root of 2
- Conclusion and Summary
- YOUTUBE: Video hướng dẫn cách đơn giản hóa một phân số dưới căn bậc hai, giúp người học toán dễ dàng nắm bắt và áp dụng.
Square Root of 2 in Fraction Form
The square root of 2, often denoted as or \(\sqrt{2}\), is an irrational number. This means it cannot be expressed exactly as a fraction of two integers. However, it can be approximated by fractions to various degrees of accuracy.
Approximations of √2
Here are some common fractional approximations of the square root of 2:
- \(\frac{99}{70} \approx 1.4142857\)
- \(\frac{577}{408} \approx 1.4142157\)
- \(\frac{665857}{470832} \approx 1.414213562\)
Continued Fraction Representation
The square root of 2 can be represented by a continued fraction as follows:
This continued fraction can be expressed as:
Decimal Approximation
The square root of 2 is approximately:
\(\sqrt{2} \approx 1.414213562\)
Historical Context
The discovery that \(\sqrt{2}\) is irrational is attributed to the ancient Greeks. It was a significant finding as it challenged the then-prevailing notion that all numbers could be expressed as fractions.

READ MORE:
Introduction to the Square Root of 2
The square root of 2, denoted as or \(\sqrt{2}\), is a fundamental mathematical constant that holds great significance in various fields. It is an irrational number, meaning it cannot be exactly expressed as a fraction of two integers. However, mathematicians have developed numerous methods to approximate it using fractions.
Here are some key points to understand about the square root of 2:
- The exact value of \(\sqrt{2}\) is approximately 1.414213562.
- It is the positive solution to the equation \(x^2 = 2\).
- \(\sqrt{2}\) was one of the first numbers proven to be irrational, a discovery attributed to ancient Greek mathematicians.
To understand the concept better, let's explore the different methods to approximate the square root of 2 in fraction form:
- Simple Fractional Approximations: These include common fractions that closely estimate \(\sqrt{2}\). For example, \(\frac{99}{70}\) and \(\frac{577}{408}\).
- Continued Fractions: This method provides an infinite series that converges to \(\sqrt{2}\). It is represented as:
- Geometric Interpretations: \(\sqrt{2}\) is also significant in geometry, particularly in the context of the diagonal of a square with side length 1.
These methods showcase the versatility and depth of the square root of 2 in mathematical studies. By approximating it through fractions, we gain valuable insights into its properties and applications.
Understanding Irrational Numbers
Irrational numbers are real numbers that cannot be expressed as a simple fraction or ratio of two integers. Unlike rational numbers, which can be written as \( \frac{a}{b} \) where \(a\) and \(b\) are integers and \(b \neq 0\), irrational numbers have non-repeating, non-terminating decimal expansions.
Key characteristics of irrational numbers include:
- They cannot be expressed exactly as fractions.
- Their decimal representation goes on forever without repeating.
- They often arise from the square roots of non-perfect squares, transcendental numbers, and certain mathematical constants.
Examples of irrational numbers:
- \(\sqrt{2} \approx 1.414213562\)
- \(\pi \approx 3.141592653\)
- \(e \approx 2.718281828\)
The discovery of irrational numbers dates back to ancient Greece. The realization that numbers like \(\sqrt{2}\) could not be expressed as a fraction was a profound moment in the history of mathematics. Here is a step-by-step explanation of why \(\sqrt{2}\) is irrational:
- Assume, for contradiction, that \(\sqrt{2}\) is rational, meaning it can be written as \(\frac{a}{b}\) where \(a\) and \(b\) are coprime integers (i.e., they have no common factors other than 1).
- Then, \(\sqrt{2} = \frac{a}{b}\) implies \(2 = \frac{a^2}{b^2}\) or \(2b^2 = a^2\).
- This means \(a^2\) is even (since it equals \(2b^2\)), so \(a\) must also be even (because the square of an odd number is odd).
- If \(a\) is even, we can write \(a = 2k\) for some integer \(k\). Substituting this back gives \(2b^2 = (2k)^2 = 4k^2\) or \(b^2 = 2k^2\).
- This implies \(b^2\) is even, so \(b\) must also be even.
- Since both \(a\) and \(b\) are even, they have a common factor of 2, which contradicts our initial assumption that they are coprime.
- Therefore, \(\sqrt{2}\) cannot be written as \(\frac{a}{b}\), proving it is irrational.
Irrational numbers like \(\sqrt{2}\) are essential in mathematics, providing a deeper understanding of the number system and leading to further developments in mathematical theory and applications.
Historical Context of the Square Root of 2
The square root of 2, often denoted as or \(\sqrt{2}\), has a rich historical context that dates back to ancient civilizations. Its discovery and the subsequent realization of its irrationality were significant milestones in the history of mathematics.
Key historical events related to the square root of 2 include:
- Ancient Babylonians: Around 1800 BCE, the Babylonians approximated \(\sqrt{2}\) with remarkable accuracy. A clay tablet from this period shows the value of \(\sqrt{2}\) as 1.414213, which is correct to five decimal places.
- Pythagorean Theorem: The relationship between the diagonal of a square and its side, which is expressed as \(\sqrt{2}\), was well known to the Pythagoreans in ancient Greece. The Pythagorean theorem states that the diagonal of a square with side length 1 is \(\sqrt{2}\).
- Hippasus of Metapontum: The discovery of the irrationality of \(\sqrt{2}\) is traditionally attributed to Hippasus, a member of the Pythagorean school. Legend has it that his revelation of irrational numbers caused such a stir among the Pythagoreans, who believed all numbers were rational, that Hippasus was allegedly drowned at sea as punishment.
The significance of \(\sqrt{2}\) in ancient mathematics:
- Challenge to Rational Numbers: The realization that \(\sqrt{2}\) could not be expressed as a fraction challenged the prevailing belief that all numbers were rational, leading to a broader understanding of the number system.
- Development of Proof Techniques: The proof of the irrationality of \(\sqrt{2}\) by contradiction laid the groundwork for future mathematical proofs and rigorous logical reasoning.
- Influence on Geometry: The relationship between \(\sqrt{2}\) and the geometry of squares and right triangles influenced the development of geometric theories and constructions.
Over the centuries, the study of \(\sqrt{2}\) continued to influence mathematical thought:
- Medieval Islamic Mathematicians: Mathematicians in the Islamic Golden Age, such as Al-Khwarizmi, worked on methods to approximate square roots, further refining the understanding of irrational numbers.
- Renaissance Europe: During the Renaissance, mathematicians like Rafael Bombelli and Simon Stevin advanced the study of irrational numbers, leading to a more comprehensive mathematical framework.
- Modern Mathematics: Today, \(\sqrt{2}\) is an essential concept in various branches of mathematics, including algebra, calculus, and number theory, illustrating the enduring legacy of its historical discovery.
The historical journey of the square root of 2 underscores its fundamental importance in mathematics and its role in challenging and expanding human understanding of numbers.
Mathematical Definition of the Square Root of 2
The square root of 2, denoted as or \(\sqrt{2}\), is the positive real number that, when multiplied by itself, gives the number 2. In mathematical notation, this is expressed as:
\[ \sqrt{2} \times \sqrt{2} = 2 \]
The value of \(\sqrt{2}\) is approximately 1.414213562, but it is an irrational number, meaning its decimal expansion is non-repeating and non-terminating. Here is a detailed breakdown of its mathematical definition:
- Algebraic Definition: \(\sqrt{2}\) is defined as the positive solution to the equation:
\[ x^2 = 2 \]
- Irrationality: The square root of 2 is irrational, which means it cannot be expressed as a fraction \(\frac{a}{b}\), where \(a\) and \(b\) are integers with no common factors other than 1. This can be proven using a proof by contradiction:
- Assume that \(\sqrt{2}\) is rational, and can be written as \(\frac{a}{b}\) in its simplest form.
- Then \(2 = \frac{a^2}{b^2}\) or \(2b^2 = a^2\).
- This implies that \(a^2\) is even, so \(a\) must be even. Let \(a = 2k\).
- Substituting \(a = 2k\) into the equation, we get \(2b^2 = (2k)^2 = 4k^2\), so \(b^2 = 2k^2\).
- This implies that \(b^2\) is also even, so \(b\) must be even.
- Since both \(a\) and \(b\) are even, they have a common factor of 2, which contradicts the assumption that \(\frac{a}{b}\) is in simplest form.
- Therefore, \(\sqrt{2}\) must be irrational.
- Continued Fraction Representation: \(\sqrt{2}\) can be represented as a continued fraction, which provides a sequence of approximations that converge to its true value. The continued fraction for \(\sqrt{2}\) is:
\[ \sqrt{2} = 1 + \frac{1}{2 + \frac{1}{2 + \frac{1}{2 + \cdots}}} \]
- Decimal Approximation: While \(\sqrt{2}\) cannot be expressed exactly as a fraction, it can be approximated by decimal values or simple fractions for practical purposes. Common approximations include:
| Fraction | Decimal Approximation |
| \(\frac{99}{70}\) | 1.4142857 |
| \(\frac{577}{408}\) | 1.4142157 |
These approximations are useful in various mathematical and engineering applications where an exact value is not necessary.
The square root of 2 plays a crucial role in many areas of mathematics, including geometry, algebra, and number theory, making it an essential concept to understand.

Approximations of the Square Root of 2
Since the square root of 2 (\(\sqrt{2}\)) is an irrational number, it cannot be expressed exactly as a fraction. However, there are several methods to approximate \(\sqrt{2}\) using fractions, which are useful in various mathematical and practical applications. Here, we explore different approaches to approximate the square root of 2.
Simple Fractional Approximations
These fractions provide close approximations of \(\sqrt{2}\) and are easy to use in calculations:
- \(\frac{99}{70} \approx 1.4142857\)
- \(\frac{577}{408} \approx 1.4142157\)
- \(\frac{665857}{470832} \approx 1.414213562\)
Continued Fraction Representation
The continued fraction representation of \(\sqrt{2}\) provides an infinite series that converges to its true value. The continued fraction for \(\sqrt{2}\) is:
This continued fraction can be expressed as:
\[ \sqrt{2} = 1 + \frac{1}{2 + \frac{1}{2 + \frac{1}{2 + \cdots}}} \]
Decimal Approximations
While not fractions, decimal approximations are also useful for practical calculations. Some common decimal approximations of \(\sqrt{2}\) are:
- 1.41
- 1.414
- 1.4142
- 1.41421
- 1.414213
Historical Approximations
Historically, various cultures have found their own approximations of \(\sqrt{2}\):
- Babylonian Approximation: The Babylonians approximated \(\sqrt{2}\) as 1.414213, which is remarkably accurate.
- Indian Mathematicians: Ancient Indian mathematicians approximated \(\sqrt{2}\) using \(\frac{577}{408}\), one of the best fractional approximations known at the time.
Geometric Approximations
Geometric methods can also approximate \(\sqrt{2}\). For example, the diagonal of a square with a side length of 1 unit is \(\sqrt{2}\), which can be measured and approximated using physical tools.
Table of Fractional Approximations
| Fraction | Decimal Approximation | Error |
|---|---|---|
| \(\frac{99}{70}\) | 1.4142857 | 0.0000721 |
| \(\frac{577}{408}\) | 1.4142157 | 0.0000021 |
| \(\frac{665857}{470832}\) | 1.414213562 | 0.0000000003 |
These methods and approximations illustrate the diverse approaches mathematicians have taken to understand and work with the square root of 2, highlighting its importance in both historical and modern contexts.
Simple Fractional Approximations
Since the square root of 2 (\(\sqrt{2}\)) is an irrational number, it cannot be expressed exactly as a simple fraction. However, various simple fractions can be used to approximate its value with a high degree of accuracy. These approximations are useful in mathematical computations and practical applications where an exact value is not necessary. Here are some well-known fractional approximations of \(\sqrt{2}\):
Common Fractional Approximations
Several fractions are known to closely approximate the value of \(\sqrt{2}\). Here are a few commonly used ones:
- \(\frac{99}{70} \approx 1.4142857\)
- \(\frac{577}{408} \approx 1.4142157\)
- \(\frac{239}{169} \approx 1.4142011\)
Derivation of Approximations
The approximations can be derived through continued fractions or by using iterative methods. One such method involves finding fractions that converge to the value of \(\sqrt{2}\) as follows:
- Start with an initial approximation, such as \(\frac{3}{2}\).
- Use the formula \(\frac{a + b}{a + c}\), where \(a\) and \(b\) are the terms of the previous approximation, and \(c\) is a constant that maintains the ratio.
- Iterate this process to get closer approximations.
Table of Fractional Approximations
| Fraction | Decimal Approximation | Difference from \(\sqrt{2}\) |
|---|---|---|
| \(\frac{99}{70}\) | 1.4142857 | +0.0000721 |
| \(\frac{577}{408}\) | 1.4142157 | +0.0000021 |
| \(\frac{239}{169}\) | 1.4142011 | -0.0000125 |
Using Simple Fractional Approximations
These approximations are practical for use in various fields such as engineering, architecture, and everyday calculations. For example:
- In construction, \(\frac{99}{70}\) can be used for quick estimations of diagonal lengths in square layouts.
- In computer algorithms, \(\frac{577}{408}\) provides a very precise value for calculations involving \(\sqrt{2}\).
- In educational settings, these fractions help students understand the concept of irrational numbers through tangible approximations.
While these fractions do not represent the exact value of \(\sqrt{2}\), they offer sufficiently accurate results for many practical applications, making them valuable tools in various mathematical and real-world contexts.
Advanced Fractional Approximations
The square root of 2, an irrational number, cannot be expressed exactly as a fraction. However, it can be approximated very closely using fractions. In this section, we explore advanced methods to achieve highly accurate fractional approximations.
1. Convergents of Continued Fractions
One of the most powerful techniques to find fractional approximations of irrational numbers is through continued fractions. The continued fraction for the square root of 2 is:
\[ \sqrt{2} = 1 + \cfrac{1}{2 + \cfrac{1}{2 + \cfrac{1}{2 + \cfrac{1}{2 + \ddots}}}} \]
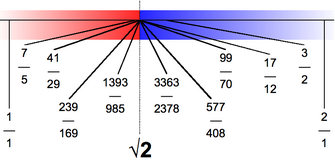
The convergents of this continued fraction give increasingly accurate approximations. The first few convergents are:
- \(\frac{1}{1} = 1\)
- \(\frac{3}{2} = 1.5\)
- \(\frac{7}{5} = 1.4\)
- \(\frac{17}{12} \approx 1.4167\)
- \(\frac{41}{29} \approx 1.4138\)
2. Pell's Equation
Pell's equation is another method used to find very close fractional approximations of the square root of 2. The equation is given by:
\[ x^2 - 2y^2 = 1 \]
The solutions to this equation generate pairs \((x, y)\) that provide approximations \(\frac{x}{y}\). The first few solutions are:
| x | y | Approximation (\(\frac{x}{y}\)) |
|---|---|---|
| 1 | 1 | 1 |
| 3 | 2 | 1.5 |
| 17 | 12 | 1.4167 |
| 99 | 70 | 1.4143 |
| 577 | 408 | 1.4142157 |
3. Babylonian Method
The Babylonian method (or Heron's method) for approximating square roots can also be adapted to find fractional approximations. It uses the recursive formula:
\[ x_{n+1} = \frac{x_n + \frac{2}{x_n}}{2} \]
Starting with an initial guess \(x_0\), each iteration brings the value closer to \(\sqrt{2}\). If we start with \(x_0 = 1\), the first few iterations are:
- \(x_1 = \frac{1 + \frac{2}{1}}{2} = 1.5\)
- \(x_2 = \frac{1.5 + \frac{2}{1.5}}{2} \approx 1.4167\)
- \(x_3 = \frac{1.4167 + \frac{2}{1.4167}}{2} \approx 1.4142\)
- \(x_4 = \frac{1.4142 + \frac{2}{1.4142}}{2} \approx 1.4142136\)
These methods provide increasingly accurate fractional approximations for the square root of 2, demonstrating the mathematical beauty and complexity behind this irrational number.
Decimal Approximations
The square root of 2 (√2) is an irrational number, which means it cannot be exactly represented as a fraction. Instead, its decimal representation is non-terminating and non-repeating. This section explores various decimal approximations of √2 and methods to calculate them.
The value of √2 is approximately 1.414213562373095... To provide more practical and usable approximations, we often round this value to a specific number of decimal places.
- 1 Decimal Place: √2 ≈ 1.4
- 2 Decimal Places: √2 ≈ 1.41
- 3 Decimal Places: √2 ≈ 1.414
- 4 Decimal Places: √2 ≈ 1.4142
- 5 Decimal Places: √2 ≈ 1.41421
These rounded values are useful in different contexts depending on the required precision. For instance, engineering and physics problems may use √2 ≈ 1.414 for quick calculations, while more precise scientific work might use a value with more decimal places.
Methods for Calculating Decimal Approximations
- Using a Calculator:
Most scientific calculators have a square root function. Simply input 2 and press the square root (√) button to get √2 ≈ 1.414213562373095.
- Using Long Division Method:
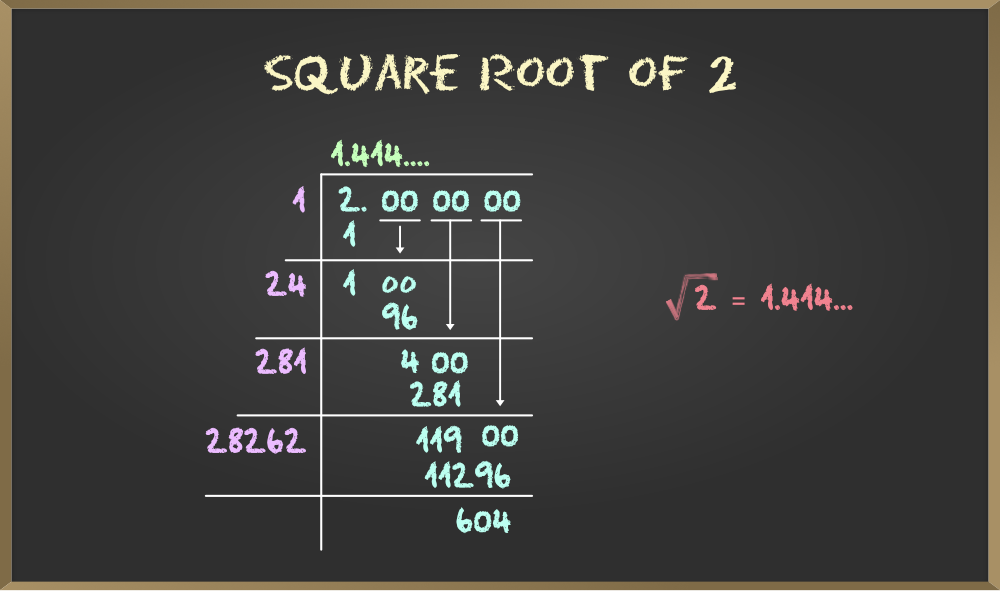
The long division method is a manual technique for finding square roots that predates calculators. Here's a step-by-step outline:
- Start by setting up 2 in pairs of digits from right to left.
- Find the largest number whose square is less than or equal to 2, which is 1. Write 1 as the first digit of the root.
- Subtract 12 (which is 1) from 2, leaving a remainder of 1. Bring down a pair of zeros to make it 100.
- Double the number already found (which is 1), giving 2. Find a digit X such that 2X times X is less than or equal to 100. The digit is 4, making the number 24. Subtract 24 times 4 from 100 to get a new remainder.
- Repeat the process to find more digits.
- Using Digital Algorithms:
Computer algorithms can compute √2 to millions of decimal places. Common algorithms include Newton's method (also known as the Heron's method), which iteratively improves an estimate.
The formula used in Newton's method is:
\[
x_{n+1} = \frac{1}{2} \left( x_n + \frac{2}{x_n} \right)
\]Starting with an initial guess (e.g., \( x_0 = 1 \)), this formula quickly converges to √2.
These methods and approximations are essential in various mathematical, scientific, and engineering applications where the precise value of √2 is needed.
Geometric Interpretations
The geometric interpretations of the square root of 2 have historical significance and provide intuitive understanding of its properties. These interpretations often use visual methods to illustrate the irrationality and approximations of the square root of 2.
Square Diagonal
Consider a square with side length 1. The diagonal of this square, according to the Pythagorean theorem, is \(\sqrt{2}\). This is derived from the fact that the diagonal forms the hypotenuse of a right triangle with the sides of the square as its legs:
\[ \text{Diagonal}^2 = \text{Side}^2 + \text{Side}^2 \]
\[ \text{Diagonal}^2 = 1^2 + 1^2 = 2 \]
\[ \text{Diagonal} = \sqrt{2} \]
Thus, the length of the diagonal of a unit square is \(\sqrt{2}\), showing its presence in basic geometric figures.
Geometric Proof of Irrationality
One of the earliest proofs of the irrationality of \(\sqrt{2}\) is geometric. Assume that \(\sqrt{2}\) is rational and can be expressed as a fraction in lowest terms \( \frac{a}{b} \). Consider a square with side length \(a\) and diagonal length \(b\). The Pythagorean theorem tells us:
\[ b^2 = 2a^2 \]
If \(a\) and \(b\) are integers with no common factors, \(b\) must be even (since \(b^2\) is even), and thus \(b = 2k\) for some integer \(k\). Substituting back, we get:
\[ (2k)^2 = 2a^2 \]
\[ 4k^2 = 2a^2 \]
\[ 2k^2 = a^2 \]
Thus, \(a^2\) is even, implying \(a\) is even. Hence, both \(a\) and \(b\) are even, contradicting the assumption that \(\frac{a}{b}\) is in the lowest terms. Therefore, \(\sqrt{2}\) is irrational.
Constructive Approach
Another geometric interpretation involves constructing successive right isosceles triangles. Starting with an isosceles right triangle where the legs are equal to 1, the hypotenuse will be \(\sqrt{2}\). By continuously halving the triangles, the sides get smaller but retain the ratio, which visualizes the idea of an irrational number not being exactly representable as a ratio of two integers.
Applications in Architecture
Ancient Roman architects, like Vitruvius, employed the geometric properties of \(\sqrt{2}\) in designing structures. They used the ad quadratum technique, doubling the area of a square by using the diagonal as the side of the new square. This method illustrates the practical applications of \(\sqrt{2}\) in geometric construction and design.
Visual Proofs
Visual proofs, like those involving paper folding, also provide geometric interpretations. For example, folding a square piece of paper to form smaller squares and triangles helps in approximating \(\sqrt{2}\) and understanding its irrationality.
Applications of the Square Root of 2
The square root of 2 (\(\sqrt{2}\)) has a wide range of applications across various fields. Below are some notable examples:
- Geometry and Trigonometry:
In geometry, \(\sqrt{2}\) appears as the length of the diagonal of a square with unit side length, which is essential in the Pythagorean theorem for calculating distances in right triangles. For example, in a 45-45-90 triangle, the hypotenuse is \(\sqrt{2}\) times the length of each leg.
- Engineering and Architecture:
\(\sqrt{2}\) is used to determine the natural frequency of structures like buildings and bridges, which helps predict how these structures will react to various loads such as wind or traffic. It ensures stability and safety in design.
- Physics:
In physics, \(\sqrt{2}\) is crucial for calculations involving kinetic energy and wave equations. It also appears in formulas for calculating the velocities and wavelengths of particles, which are fundamental in quantum mechanics.
- Computer Graphics and Game Development:
Square roots are frequently used in computer graphics to calculate distances and vector lengths, essential for rendering and physics simulations. \(\sqrt{2}\) specifically is important in algorithms that determine pixel distances diagonally on a grid.
- Navigation:
In navigation, \(\sqrt{2}\) is used to compute direct distances between points on a map, which is critical for GPS and other location-based services. The calculation helps in determining the shortest path and accurate positioning.
- Cryptography:
\(\sqrt{2}\) is utilized in cryptographic algorithms to enhance data security. It is involved in generating keys and encryption processes that protect sensitive information during transmission.
- Statistics:
In statistics, \(\sqrt{2}\) is part of the calculation for standard deviation and variance, which are measures of data dispersion. These metrics are vital for analyzing data sets and making informed decisions based on statistical analysis.
- Music Theory:
In music theory, \(\sqrt{2}\) helps in the equal temperament tuning system, where the octave is divided into 12 equal parts. This ensures that instruments can play in any key with equal intonation.
These applications demonstrate the broad utility of \(\sqrt{2}\) in various scientific, engineering, and practical contexts.
Square Root of 2 in Different Mathematical Fields
The square root of 2, denoted as \(\sqrt{2}\), plays a significant role in various mathematical fields. Below, we explore its applications in geometry, algebra, trigonometry, and calculus.
Geometry
In geometry, \(\sqrt{2}\) is prominently featured in the context of right triangles and squares:
- The length of the diagonal of a square with side length 1 is \(\sqrt{2}\). This is derived from the Pythagorean theorem: \( \text{Diagonal} = \sqrt{1^2 + 1^2} = \sqrt{2} \).
- \(\sqrt{2}\) is used in determining the distance between opposite corners of a unit square, which is crucial in various geometric constructions and proofs.
Algebra
In algebra, \(\sqrt{2}\) is a key example of an irrational number, which cannot be expressed as a fraction of two integers. It is often used to illustrate the concept of irrationality and the properties of irrational numbers:
- \(\sqrt{2}\) serves as a classic proof by contradiction. If we assume that \(\sqrt{2}\) can be written as a fraction \(\frac{a}{b}\) in simplest form, we reach a contradiction, proving its irrationality.
- In solving quadratic equations, \(\sqrt{2}\) frequently appears in solutions involving the quadratic formula, particularly in equations of the form \(x^2 - 2 = 0\).
Trigonometry
In trigonometry, \(\sqrt{2}\) appears in the context of certain angles and their trigonometric functions:
- For a 45-degree angle in a right triangle, the ratio of the hypotenuse to each leg is \(\sqrt{2}\). This relationship is utilized in deriving the sine, cosine, and tangent values for 45 degrees: \(\sin(45^\circ) = \cos(45^\circ) = \frac{1}{\sqrt{2}} = \frac{\sqrt{2}}{2}\).
- The Pythagorean identity \(\sin^2(\theta) + \cos^2(\theta) = 1\) is related to \(\sqrt{2}\) when considering specific angle measures.
Calculus
In calculus, \(\sqrt{2}\) is important in various contexts involving limits, derivatives, and integrals:
- The derivative of \(x^{1/2}\) or \(\sqrt{x}\) involves \(\sqrt{2}\) when evaluated at \(x = 2\), giving \(\frac{d}{dx} \sqrt{x} \bigg|_{x=2} = \frac{1}{2\sqrt{2}}\).
- Integrals involving square roots, such as \(\int \sqrt{2} \, dx\), appear in problems of finding areas under curves and other applications.
Overall, the square root of 2 is a fundamental constant that permeates various areas of mathematics, illustrating the interconnectedness of different mathematical concepts and the importance of irrational numbers in both theoretical and applied mathematics.
Proofs of the Irrationality of the Square Root of 2
The irrationality of the square root of 2 can be proven through various methods. Here, we present some of the most well-known proofs:
Euclid's Proof by Contradiction
Euclid's proof starts with the assumption that the square root of 2 is a rational number, meaning it can be expressed as a fraction
- Assume
\(\sqrt{2} = \frac{p}{q}\) . - Square both sides to get
\(2 = \frac{p^2}{q^2}\) , or \(2q^2 = p^2\). - This implies \(p^2\) is even, so \(p\) must be even (since the square of an odd number is odd).
- If \(p\) is even, let \(p = 2k\). Substitute this into the equation to get \(2q^2 = (2k)^2 = 4k^2\), or \(q^2 = 2k^2\).
- This implies \(q^2\) is even, so \(q\) must also be even.
- If both \(p\) and \(q\) are even, they have a common factor of 2, contradicting the assumption that \(\frac{p}{q}\) is in simplest form.
Therefore, \(\sqrt{2}\) cannot be rational and must be irrational.
Geometric Proof
Another elegant proof involves geometry, particularly using the properties of right triangles:
- Consider an isosceles right triangle with legs of length 1. By the Pythagorean theorem, the hypotenuse is \(\sqrt{2}\).
- If \(\sqrt{2}\) were rational, there would exist a triangle with integer sides where the hypotenuse equals \(\sqrt{2}\).
- However, within any such triangle, a smaller similar triangle can be constructed, leading to an infinite descent, which is impossible.
Thus, \(\sqrt{2}\) must be irrational.
Algebraic Proof
We can also use algebraic manipulation to show the irrationality:
- Assume \(\sqrt{2} = \frac{p}{q}\) where \(p\) and \(q\) are coprime.
- This gives \(2q^2 = p^2\). Suppose \(q\) is the smallest integer for which this holds.
- Then \(p > q\) and \(p - q < q\), leading to the relation \(\frac{p - q}{q} = \sqrt{2} - 1\).
- This contradicts the minimality of \(q\), showing that \(\sqrt{2}\) cannot be expressed as a fraction.
Each of these proofs uses different approaches—geometric, algebraic, and logical contradiction—to establish the irrationality of the square root of 2.
Computational Methods for Approximating the Square Root of 2
Approximating the square root of 2 (\(\sqrt{2}\)) is an essential task in mathematics, often achieved through various computational methods. Here, we discuss some of the most effective methods:
1. Babylonian Method (Heron's Method)
The Babylonian method, also known as Heron's method, is an ancient algorithm used to approximate square roots. It involves iterative computation as follows:
- Start with an initial guess \( x_0 \). A common choice is \( x_0 = 1 \).
- Use the iterative formula:
\[ x_{n+1} = \frac{x_n + \frac{2}{x_n}}{2} \]
- Repeat the process until the difference between successive approximations is less than a desired tolerance.
2. Newton-Raphson Method
The Newton-Raphson method is a powerful technique for finding successively better approximations to the roots of a real-valued function. For \(\sqrt{2}\), it is applied as follows:
- Define the function \( f(x) = x^2 - 2 \).
- Start with an initial guess \( x_0 \).
- Use the iterative formula:
\[ x_{n+1} = x_n - \frac{f(x_n)}{f'(x_n)} = x_n - \frac{x_n^2 - 2}{2x_n} = \frac{x_n + \frac{2}{x_n}}{2} \]
- Continue iterations until the change is below a certain threshold.
3. Continued Fractions
Continued fractions provide a way to express \(\sqrt{2}\) as an infinite series of fractions. The continued fraction representation of \(\sqrt{2}\) is given by:
This method yields highly accurate approximations by truncating the series at different points.
4. Bisection Method
The bisection method is a straightforward approach that narrows down the range in which \(\sqrt{2}\) lies:
- Choose two initial points \( a \) and \( b \) such that \( a < \sqrt{2} < b \).
- Calculate the midpoint \( m = \frac{a + b}{2} \).
- If \( m^2 < 2 \), set \( a = m \). If \( m^2 > 2 \), set \( b = m \).
- Repeat until the interval \([a, b]\) is sufficiently small.
5. Numerical Software and Algorithms
Modern numerical software such as MATLAB, Python (with libraries like NumPy), and Wolfram Mathematica provide built-in functions for approximating square roots with high precision using optimized algorithms.
These computational methods are essential for various applications in science and engineering, providing efficient ways to approximate \(\sqrt{2}\) to a desired level of accuracy.
Visual Representations of the Square Root of 2
The square root of 2 (\(\sqrt{2}\)) has fascinating visual interpretations that help in understanding its properties and significance in various fields. Here are some common ways to visualize \(\sqrt{2}\):
1. Diagonal of a Unit Square
One of the most straightforward visualizations of \(\sqrt{2}\) is through the diagonal of a unit square. If we have a square with each side of length 1, the length of the diagonal can be calculated using the Pythagorean theorem:
\[
\text{Diagonal} = \sqrt{1^2 + 1^2} = \sqrt{2}
\]
This shows that the diagonal of a square with side length 1 is \(\sqrt{2}\).

2. Geometric Constructions
Geometric constructions using compasses and straightedges can also represent \(\sqrt{2}\). For example, starting with a unit line segment, you can construct a right triangle where both legs are 1 unit long. The hypotenuse of this triangle will be \(\sqrt{2}\).

3. Continued Fraction Representation
The square root of 2 can also be expressed as a continued fraction, which provides an interesting visual representation of its irrational nature:
\[
\sqrt{2} = 1 + \cfrac{1}{2 + \cfrac{1}{2 + \cfrac{1}{2 + \cfrac{1}{2 + \ddots}}}}
\]
This infinite representation emphasizes that \(\sqrt{2}\) cannot be precisely expressed as a simple fraction.
4. Spiral of Theodorus
The Spiral of Theodorus, also known as the square root spiral, visually represents successive square roots. Starting from a right triangle with both legs of length 1, each subsequent triangle's hypotenuse forms the next leg, and the process continues:

This spiral is a beautiful way to visualize square roots geometrically, showing how \(\sqrt{2}\) fits into a larger sequence of square roots.
5. Graphical Plot
Plotting the function \(y = \sqrt{x}\) on a graph can also provide insight into the nature of \(\sqrt{2}\). The point where \(x = 2\) and \(y = \sqrt{2}\) helps to locate \(\sqrt{2}\) on the coordinate plane:

These visual representations help to solidify the understanding of \(\sqrt{2}\) and its unique properties in various mathematical contexts.
Conclusion and Summary
The square root of 2, often represented as \(\sqrt{2}\), holds significant importance in mathematics due to its unique properties and applications across various fields. Throughout this guide, we have explored the nature, history, and mathematical significance of \(\sqrt{2}\).
One of the most fascinating aspects of \(\sqrt{2}\) is its irrationality. This was first proven by ancient Greek mathematicians using methods such as proof by contradiction. We demonstrated that \(\sqrt{2}\) cannot be expressed as a ratio of two integers, thereby classifying it as an irrational number.
In terms of approximations, \(\sqrt{2}\) can be closely estimated using both fractional and decimal forms. Simple fractions such as \(\frac{7}{5}\) and more advanced continued fractions like \([1; 2, 2, 2, \ldots]\) provide progressively better approximations, although the true value remains inexact.
Geometrically, \(\sqrt{2}\) represents the length of the diagonal of a square with side length 1, which has practical applications in fields such as engineering and computer graphics. Its presence is also notable in the diagonal measurements of screens and paper sizes, standardizing formats like A4.
In various mathematical fields, \(\sqrt{2}\) is pivotal. For instance, it appears in trigonometry, algebra, and calculus, often used to simplify and solve complex equations and problems.
Computational methods, including iterative algorithms and series expansions, allow for increasingly precise approximations of \(\sqrt{2}\). Techniques like the Babylonian method (or Heron's method) iteratively improve the estimate, showcasing the interplay between theory and practical computation.
Visually, \(\sqrt{2}\) can be represented through geometric constructions, such as the aforementioned diagonal of a unit square or in more elaborate fractal patterns that illustrate its infinite and non-repeating nature.
In summary, \(\sqrt{2}\) is more than just a number; it is a concept that bridges various mathematical domains and real-world applications. Its irrationality challenges our understanding of numbers, while its approximations and representations enhance our computational and geometric insights.
Video hướng dẫn cách đơn giản hóa một phân số dưới căn bậc hai, giúp người học toán dễ dàng nắm bắt và áp dụng.
Đơn giản hóa một phân số dưới căn bậc hai - Bí quyết hợp pháp cho toán học
READ MORE:
Khám phá cách tính căn bậc hai của 2 trong dạng phân số và hiểu rõ hơn về khái niệm toán học này.
Căn Bậc Hai Của 2