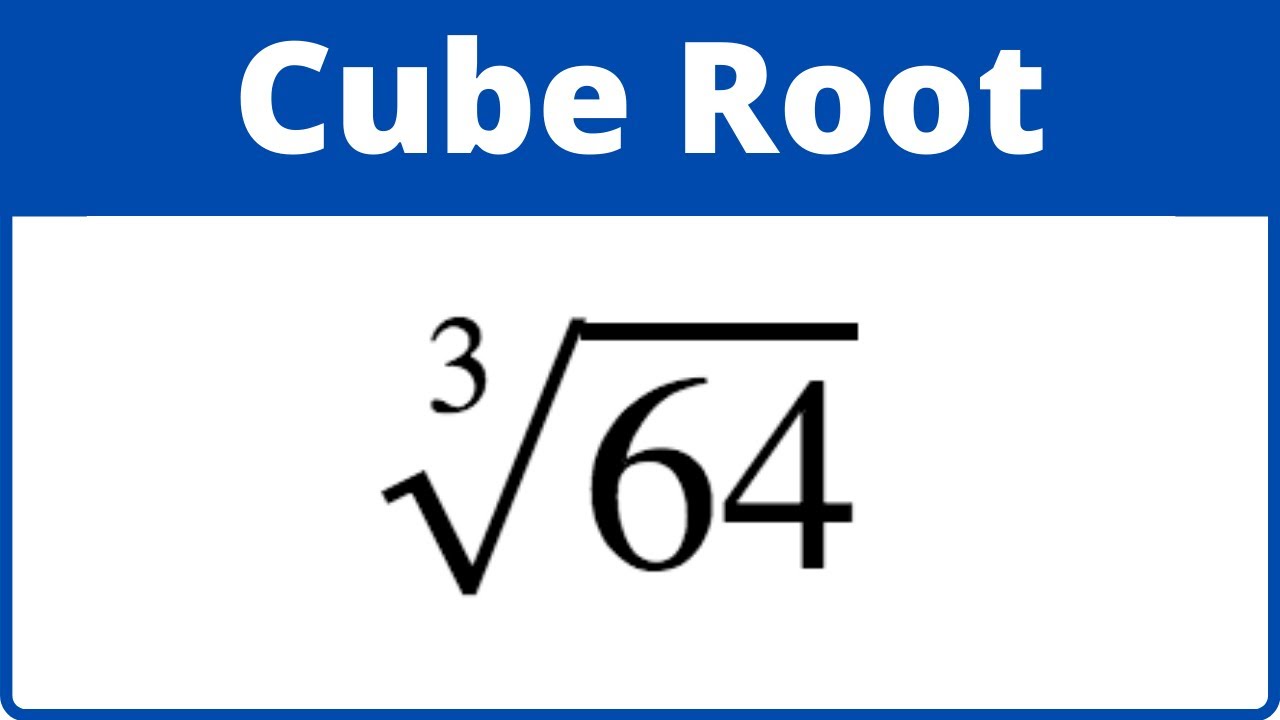
Topic square root of 180 in radical form: Discover how to simplify the square root of 180 into its radical form with easy-to-follow steps. This guide breaks down the process, using prime factorization and fundamental properties of square roots to make understanding the simplification of √180 straightforward and accessible. Perfect for students and math enthusiasts looking to deepen their knowledge.
Table of Content
- Square Root of 180 in Radical Form
- Introduction to Square Roots
- Understanding Radical Expressions
- Prime Factorization Method
- Steps to Simplify the Square Root of 180
- Properties of Square Roots
- Breaking Down 180 into Prime Factors
- Simplifying the Radical Form
- Combining and Simplifying Factors
- Final Simplified Form of √180
- Practical Applications of Simplifying Radicals
- Common Mistakes and Tips
- YOUTUBE:
Square Root of 180 in Radical Form
The square root of 180 can be expressed in its simplest radical form by breaking it down into its prime factors and simplifying:
Prime Factorization of 180
First, find the prime factors of 180:
- 180 is divisible by 2: \(180 \div 2 = 90\)
- 90 is divisible by 2: \(90 \div 2 = 45\)
- 45 is divisible by 3: \(45 \div 3 = 15\)
- 15 is divisible by 3: \(15 \div 3 = 5\)
- 5 is a prime number
So, the prime factorization of 180 is: \(180 = 2^2 \times 3^2 \times 5\)
Simplifying the Radical
To simplify the square root of 180, we take the square root of the prime factorization:
\[\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5}\]
Using the property of square roots \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\), we can separate the factors:
\[\sqrt{180} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{5}\]
Simplify the square roots of the perfect squares:
\[\sqrt{180} = 2 \times 3 \times \sqrt{5}\]
Multiply the integers together:
\[\sqrt{180} = 6\sqrt{5}\]
Conclusion
The square root of 180 in its simplest radical form is:
\[\sqrt{180} = 6\sqrt{5}\]

READ MORE:
Introduction to Square Roots
The concept of square roots is fundamental in mathematics, representing one of the two equal factors of a number. For any positive number \(x\), the square root, denoted as \(\sqrt{x}\), is the value that, when multiplied by itself, equals \(x\). For example, the square root of 9 is 3, because \(3 \times 3 = 9\).
Square roots can be either positive or negative, but the principal square root is always taken as positive. For instance, while both 4 and -4 are square roots of 16, \(\sqrt{16}\) is 4.
Square roots are particularly useful in various fields of science, engineering, and everyday calculations. They play a crucial role in solving quadratic equations, optimizing areas and volumes, and analyzing geometric shapes.
To better understand square roots, let's look at their properties and methods to simplify them, especially when dealing with non-perfect squares, like 180.
Consider the square root of 180. To find its simplest radical form, we need to use the prime factorization method. This involves:
- Breaking down 180 into its prime factors
- Grouping the prime factors into pairs
- Extracting these pairs from under the radical sign
By following these steps, we can simplify the square root of 180 to a more manageable form. This process will be detailed in the subsequent sections, providing a clear and comprehensive guide to understanding and simplifying square roots.
Understanding Radical Expressions
Radical expressions are expressions that contain a square root, cube root, or any higher-order root symbol. The square root symbol (√) is the most common radical symbol and it denotes the principal square root of a number.
A radical expression can be written in the form:
√a
where a is the radicand (the number under the radical sign). When the radicand is a positive number, the radical expression represents the positive root of that number.
There are some important properties of radicals that help in understanding and simplifying them:
- The square root of a product is equal to the product of the square roots:
√(a * b) = √a * √b - The square root of a quotient is equal to the quotient of the square roots:
√(a / b) = √a / √b - Squaring a square root returns the original number:
(√a)^2 = a
To simplify a radical expression, it is often helpful to use the prime factorization of the radicand. By expressing the radicand as a product of its prime factors, we can often identify pairs of factors that can be "taken out" of the square root. This process is illustrated in the following sections, where we simplify the square root of 180.
Prime Factorization Method
The prime factorization method involves breaking down a composite number into a product of its prime factors. This method is particularly useful in simplifying square roots, as it helps to identify pairs of factors that can be taken out of the radical.
Here are the steps to perform prime factorization:
- Identify the smallest prime number that divides the given number.
- Divide the number by this prime number to get a quotient.
- Continue dividing the quotient by prime numbers until the quotient is 1.
- The original number is now expressed as a product of these prime factors.
Let's apply this method to find the prime factorization of 180:
- 180 is divisible by 2 (the smallest prime number):
\( 180 \div 2 = 90 \) - 90 is divisible by 2:
\( 90 \div 2 = 45 \) - 45 is divisible by 3 (the next smallest prime number):
\( 45 \div 3 = 15 \) - 15 is divisible by 3:
\( 15 \div 3 = 5 \) - 5 is a prime number:
\( 5 \div 5 = 1 \)
So, the prime factorization of 180 is:
\[
180 = 2 \times 2 \times 3 \times 3 \times 5
\]
We can rewrite this as:
\[
180 = 2^2 \times 3^2 \times 5
\]
In the next section, we will use these prime factors to simplify the square root of 180.
Steps to Simplify the Square Root of 180
Simplifying the square root of a number involves expressing it in its simplest radical form. Here, we will use the prime factorization of 180 to simplify \( \sqrt{180} \).
The prime factorization of 180 is:
\[
180 = 2^2 \times 3^2 \times 5
\]
To simplify \( \sqrt{180} \), we will follow these steps:
- Write the square root expression with the prime factors inside:
\[ \sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} \] - Apply the property of square roots:
\[ \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \]
This allows us to separate the factors under the square root:
\[ \sqrt{2^2 \times 3^2 \times 5} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{5} \] - Simplify the square roots of the perfect squares:
\[ \sqrt{2^2} = 2 \quad \text{and} \quad \sqrt{3^2} = 3 \] - Combine the simplified factors:
\[ 2 \times 3 \times \sqrt{5} = 6\sqrt{5} \]
Therefore, the simplified form of \( \sqrt{180} \) is:
\[
\sqrt{180} = 6\sqrt{5}
\]
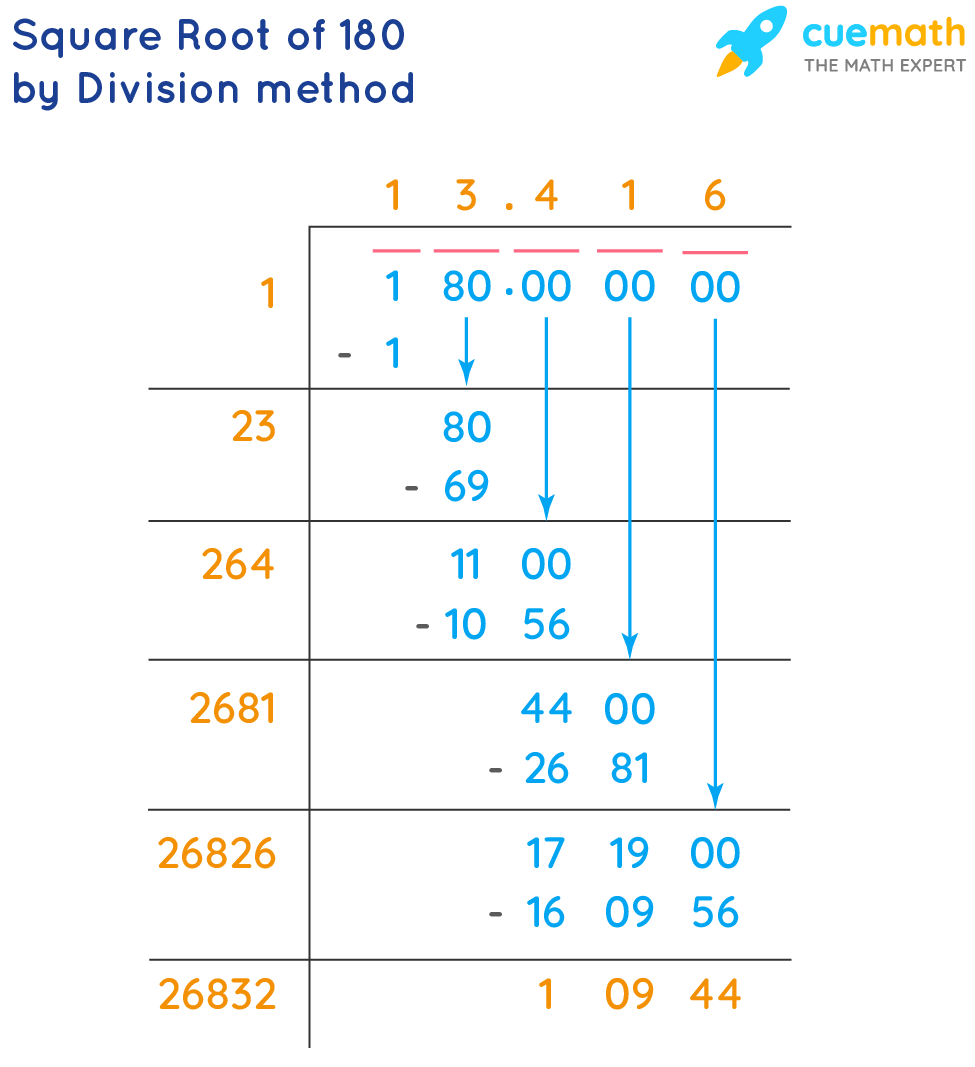
Properties of Square Roots
The square root is a mathematical operation that helps us find a number which, when multiplied by itself, gives the original number. The properties of square roots are crucial in simplifying and understanding radical expressions. Here are some key properties:
- Non-negativity: The square root of any non-negative number is also non-negative. For any non-negative number \(a\), \(\sqrt{a} \geq 0\).
- Product Property: The square root of a product is equal to the product of the square roots of the factors. For any non-negative numbers \(a\) and \(b\), \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\).
- Quotient Property: The square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator. For any non-negative numbers \(a\) and \(b\) (where \(b \neq 0\)), \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\).
- Power of Two Property: The square root of a number squared returns the original number. For any non-negative number \(a\), \(\sqrt{a^2} = a\).
- Addition and Subtraction: Unlike multiplication and division, the square root of a sum or difference is not generally equal to the sum or difference of the square roots. For any non-negative numbers \(a\) and \(b\), \(\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\) and \(\sqrt{a - b} \neq \sqrt{a} - \sqrt{b}\).
These properties are foundational for working with radicals and simplifying expressions involving square roots. By applying these rules, we can break down complex radical expressions into simpler forms.
Breaking Down 180 into Prime Factors
To simplify the square root of 180, we first need to break down 180 into its prime factors. Here are the steps to find the prime factorization of 180:
- Start with the smallest prime number: 180 is an even number, so it is divisible by 2.
\[180 \div 2 = 90\]
- Continue with 90: 90 is also an even number and divisible by 2.
\[90 \div 2 = 45\]
- Move to the next smallest prime number: 45 is not divisible by 2, so we try the next prime number, which is 3.
\[45 \div 3 = 15\]
- Continue with 15: 15 is also divisible by 3.
\[15 \div 3 = 5\]
- Finally, factor 5: 5 is a prime number.
\[5 \div 5 = 1\]
Now, we have the prime factors of 180:
\[180 = 2 \times 2 \times 3 \times 3 \times 5\]
We can group these factors to help simplify the square root:
\[180 = 2^2 \times 3^2 \times 5\]
Simplifying the Radical Form
After breaking down 180 into its prime factors, the next step is to simplify the square root of 180. Here are the steps to simplify the radical form:
- Write the prime factorization under the square root:
\[\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5}\]
- Apply the product property of square roots: The square root of a product is the product of the square roots.
\[\sqrt{2^2 \times 3^2 \times 5} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{5}\]
- Simplify the square roots of the perfect squares: The square root of a number squared is the number itself.
\[\sqrt{2^2} = 2\]
\[\sqrt{3^2} = 3\]
- Combine the simplified terms: Multiply the simplified square roots together.
\[2 \times 3 \times \sqrt{5} = 6\sqrt{5}\]
Thus, the simplified form of the square root of 180 is:
\[\sqrt{180} = 6\sqrt{5}\]
Combining and Simplifying Factors
To simplify the square root of 180, we need to follow a series of steps to break down the number into its prime factors and then simplify the radical expression. Here is the detailed process:
- First, find the prime factorization of 180.
- Express 180 as a product of prime factors:
\[ 180 = 2^2 \times 3^2 \times 5 \]
- Rewrite the square root of 180 using its prime factors:
\[ \sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} \]
- Apply the property of square roots that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\):
\[ \sqrt{180} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{5} \]
- Simplify the square roots of the perfect squares:
\[ \sqrt{2^2} = 2 \] and \[ \sqrt{3^2} = 3 \]
- Combine the simplified factors:
\[ \sqrt{180} = 2 \times 3 \times \sqrt{5} \]
- Multiply the integers together to get the final simplified form:
\[ \sqrt{180} = 6\sqrt{5} \]
Therefore, the simplified radical form of the square root of 180 is \( 6\sqrt{5} \).

Final Simplified Form of √180
To find the final simplified form of √180, we follow these steps:
Prime Factorization: First, we find the prime factorization of 180. The prime factors of 180 are:
\[ 180 = 2^2 \times 3^2 \times 5 \]
Group the Factors: Group the prime factors into pairs of identical factors:
\[ \sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} \]
Simplify the Radical: For each pair of identical factors, we take one factor out of the radical. This gives us:
\[ \sqrt{180} = \sqrt{(2 \times 2) \times (3 \times 3) \times 5} = 2 \times 3 \times \sqrt{5} \]
Final Simplified Form: Multiply the factors outside the radical:
\[ \sqrt{180} = 6\sqrt{5} \]
Therefore, the final simplified form of √180 is \( 6\sqrt{5} \).
This simplification process helps to understand the structure of the number inside the radical and expresses it in its simplest form, making calculations easier and more intuitive.
Practical Applications of Simplifying Radicals
Simplifying radicals has numerous practical applications across various fields. Here are some key areas where understanding and simplifying square roots, such as
- Geometry and Construction:
In geometry, simplifying square roots is essential for calculating distances and dimensions. For example, when determining the diagonal length of a rectangle or the height of a triangle, simplified radicals make the computations straightforward. Builders and architects use these calculations to ensure accuracy in constructing buildings, bridges, and other structures.
- Physics:
Physics often involves calculations with square roots, especially when dealing with motion, energy, and wave phenomena. For example, the formula for the period of a pendulum,
\(T = 2\pi \sqrt{\frac{L}{g}}\) , requires simplifying radicals to predict the pendulum's behavior accurately. - Engineering:
Engineers use square roots in various calculations, such as determining the natural frequency of structures or analyzing stress and strain in materials. Simplifying these radicals helps in designing stable and efficient structures and machinery.
- Finance:
In finance, square roots are used to calculate volatility, which is a measure of the risk associated with a particular investment. The formula for standard deviation,
\(\sigma = \sqrt{\frac{\sum (x_i - \mu)^2}{N}}\) , involves simplifying square roots to assess market fluctuations. - Computer Science:
Square roots are crucial in computer graphics, algorithms, and encryption. For example, distance calculations between points in 2D and 3D space use the Pythagorean theorem, which involves square roots. Simplifying these calculations enhances the performance of graphics rendering and cryptographic security.
- Statistics:
In statistics, the standard deviation is calculated using the square root of the variance. This measure is vital for understanding data dispersion and making informed decisions based on statistical analysis.
- Everyday Problem Solving:
Simplified radicals can help in various day-to-day activities, such as adjusting recipes, determining the correct amount of materials for home projects, or even calculating travel distances more accurately.
Overall, the ability to simplify radicals like
Common Mistakes and Tips
When simplifying radicals, it's essential to avoid common mistakes to ensure accuracy. Here are some common pitfalls and tips to help you simplify radicals correctly:
- Incorrect Prime Factorization: Ensure that you correctly factorize the number into its prime factors. Missing a prime factor can lead to an incorrect simplified form.
- Combining Non-Like Terms: Remember that only like radicals can be combined. For example, \( \sqrt{3} + \sqrt{2} \) cannot be simplified further because the radicands are different.
- Forgetting to Simplify Completely: After simplifying the radical, always check if it can be simplified further. For instance, \( \sqrt{50} \) simplifies to \( 5\sqrt{2} \), not just \( \sqrt{50} \).
- Sign Errors: Be careful with signs when simplifying radicals, especially when dealing with negative numbers or when applying properties like the product and quotient rules.
Step-by-Step Tips
- Find Prime Factors: Begin by breaking down the number under the radical into its prime factors. For example, \( 180 = 2^2 \cdot 3^2 \cdot 5 \).
- Pair the Factors: Group the prime factors into pairs. Each pair of identical factors can be taken out of the radical. For example, from \( \sqrt{2^2 \cdot 3^2 \cdot 5} \), take out the pairs of 2's and 3's.
- Simplify Outside the Radical: Multiply the factors outside the radical. For \( \sqrt{2^2 \cdot 3^2 \cdot 5} \), this gives \( 2 \cdot 3 \cdot \sqrt{5} = 6\sqrt{5} \).
- Check for Further Simplification: Always re-check the simplified form to see if it can be simplified further. In this case, \( 6\sqrt{5} \) is already in its simplest form.
Examples
Let's go through a couple of examples to illustrate these steps:
- Example 1: Simplify \( \sqrt{72} \)
- Prime factorization: \( 72 = 2^3 \cdot 3^2 \)
- Pair the factors: \( \sqrt{2^2 \cdot 2 \cdot 3^2} \)
- Simplify outside the radical: \( 2 \cdot 3 \cdot \sqrt{2} = 6\sqrt{2} \)
- Result: \( 6\sqrt{2} \)
- Example 2: Simplify \( \sqrt{45} \)
- Prime factorization: \( 45 = 3^2 \cdot 5 \)
- Pair the factors: \( \sqrt{3^2 \cdot 5} \)
- Simplify outside the radical: \( 3\sqrt{5} \)
- Result: \( 3\sqrt{5} \)
By following these steps and being mindful of common mistakes, you can simplify radicals accurately and efficiently.
Đơn giản hóa căn bậc hai của 180
READ MORE:
Đơn giản hóa căn bậc hai của 180