Topic simplify square root of negative 121: Discover how to simplify the square root of negative 121 with our easy-to-follow guide. Learn about imaginary numbers, the role of the imaginary unit 'i', and the steps needed to transform √-121 into a simplified form. Master this mathematical concept and boost your understanding of complex numbers today!
Table of Content
- Simplifying the Square Root of -121
- Introduction to Imaginary Numbers
- Understanding the Square Root of a Negative Number
- The Concept of the Imaginary Unit 'i'
- Steps to Simplify √-121
- Breaking Down the Square Root of -121
- Identifying the Perfect Square
- Combining Real and Imaginary Parts
- Final Simplified Form of √-121
- Practical Applications of Imaginary Numbers
- Common Mistakes and How to Avoid Them
- Further Reading and Resources
- YOUTUBE: Video này hướng dẫn cách đơn giản hóa căn bậc hai của các số âm như -121, -24 và -200, giúp bạn hiểu rõ hơn về khái niệm số ảo 'i' và các bước tính toán chi tiết.
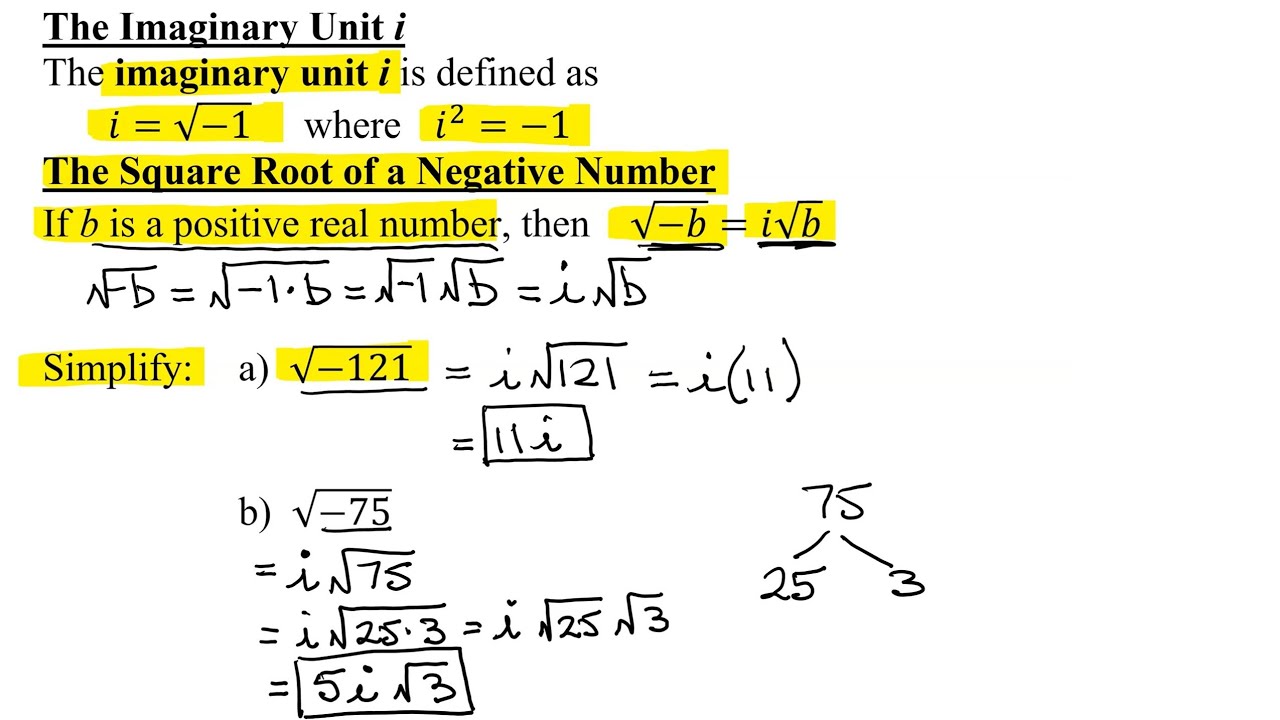
Simplifying the Square Root of -121
To simplify the square root of a negative number, we need to understand the concept of imaginary numbers. The square root of -1 is represented by the imaginary unit i. Thus, the square root of a negative number can be expressed in terms of i.
Steps to Simplify √-121
- Identify the negative sign inside the square root and separate it using the imaginary unit i.
√-121 = √(-1 * 121) - Use the property of square roots to split the product inside the square root into separate roots.
√(-1 * 121) = √-1 * √121 - Recognize that
√-1is i.√-1 = i - Simplify
√121which is a perfect square.√121 = 11 - Combine the results to get the final simplified form.
√-121 = 11i
Therefore, the simplified form of √-121 is 11i.
READ MORE:
Introduction to Imaginary Numbers
Imaginary numbers are a fundamental concept in mathematics, especially when dealing with the square roots of negative numbers. The key idea is that the square root of a negative number is not a real number, but an imaginary one.
The imaginary unit, denoted as i, is defined as the square root of -1:
\(\sqrt{-1} = i\)
Using this definition, we can express the square root of any negative number in terms of i. For example, to find the square root of -121, we follow these steps:
- Express the negative number as a product of -1 and a positive number.
\(-121 = -1 \times 121\)
- Use the property of square roots to separate the product inside the square root.
\(\sqrt{-121} = \sqrt{-1 \times 121}\)
- Apply the definition of the imaginary unit i:
\(\sqrt{-1 \times 121} = \sqrt{-1} \times \sqrt{121} = i \times 11 = 11i\)
Thus, the square root of -121 is expressed as 11i, an imaginary number. Imaginary numbers extend the real number system, allowing for the solution of equations that have no real solutions. They are essential in various fields such as engineering, physics, and complex number theory.
Understanding the Square Root of a Negative Number
The square root of a negative number introduces the concept of imaginary numbers. In the real number system, the square root of a negative number is not defined. However, by using the imaginary unit i, which is defined as \(\sqrt{-1}\), we can work with these values.
To understand the square root of a negative number, follow these steps:
- Recognize that the negative number can be expressed as the product of -1 and a positive number.
For example, \(-121\) can be written as \(-1 \times 121\).
- Apply the property of square roots to separate the negative factor from the positive factor.
\(\sqrt{-121} = \sqrt{-1 \times 121}\)
- Use the definition of the imaginary unit i, where \(\sqrt{-1} = i\).
Thus, \(\sqrt{-1 \times 121} = \sqrt{-1} \times \sqrt{121} = i \times 11\).
- Combine the results to obtain the imaginary number.
\(\sqrt{-121} = 11i\)
In this way, we see that the square root of -121 is \(11i\). This method can be applied to any negative number, allowing us to extend our understanding of square roots beyond the real numbers and into the realm of complex numbers. Imaginary numbers are crucial in various mathematical applications, including solving quadratic equations and performing complex analysis.
The Concept of the Imaginary Unit 'i'
In mathematics, particularly in the realm of complex numbers, the imaginary unit 'i' is a fundamental concept. It is defined as the square root of -1, which does not have a real number counterpart. This definition arises because the equation \( x^2 = -1 \) has no real number solution, hence the need for a new type of number to satisfy certain mathematical operations.
The imaginary unit 'i' is characterized by its unique property that \( i^2 = -1 \). This property allows for the extension of the number system to include complex numbers, which are expressed in the form \( a + bi \), where \( a \) and \( b \) are real numbers, and \( i \) represents the imaginary unit.
Complex numbers, involving the imaginary unit 'i', are essential in various fields of science and engineering, including electrical engineering, quantum mechanics, and signal processing. They provide a powerful framework for solving equations that involve roots of negative numbers and are integral to understanding phenomena that involve oscillations and waves.
Furthermore, the concept of 'i' allows for the representation of vectors in a plane, where real and imaginary components correspond to coordinates on the x-axis and y-axis, respectively. This representation, known as the Argand diagram, visually illustrates complex numbers and their relationships.
Steps to Simplify √-121
To simplify \( \sqrt{-121} \), follow these steps:
- Recognize that \( \sqrt{-121} \) involves the square root of a negative number, which is not a real number.
- Express \( \sqrt{-121} \) in terms of its components: \( \sqrt{-1 \cdot 121} \).
- Apply the property of square roots: \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \). Thus, \( \sqrt{-1 \cdot 121} = \sqrt{-1} \cdot \sqrt{121} \).
- Since \( \sqrt{-1} \) is represented by the imaginary unit \( i \), we have \( \sqrt{-1} = i \).
- Calculate \( \sqrt{121} \), which simplifies to \( 11 \) because \( 11^2 = 121 \).
- Combine the results: \( \sqrt{-121} = 11i \).
Breaking Down the Square Root of -121
To simplify the square root of -121, we need to understand the concept of imaginary numbers. Imaginary numbers are used to represent the square root of negative numbers. The imaginary unit is denoted as 'i', where:
\[ i = \sqrt{-1} \]
Using this concept, we can break down the square root of -121 as follows:
Identify the square root of the positive part of the number:
\[ \sqrt{121} = 11 \]Apply the imaginary unit 'i' to account for the negative part:
\[ \sqrt{-121} = \sqrt{121} \cdot \sqrt{-1} \]Combine the results:
\[ \sqrt{-121} = 11 \cdot i \]
Therefore, the simplified form of \( \sqrt{-121} \) is:
\[ 11i \]
Identifying the Perfect Square
To simplify the square root of -121, we first need to identify the perfect square within the number. A perfect square is a number that can be expressed as the product of an integer with itself. For example, \( 1, 4, 9, 16, \) and \( 25 \) are all perfect squares.
In the case of -121, we focus on the positive part of the number, which is 121. We need to determine if 121 is a perfect square:
Identify a number that, when multiplied by itself, equals 121:
\[ 11 \times 11 = 121 \]Since 11 multiplied by itself equals 121, we can conclude that 121 is indeed a perfect square:
\[ 121 = 11^2 \]
Now that we have identified 121 as a perfect square, we can use this information to simplify the square root of -121 by recognizing that:
\[ \sqrt{-121} = \sqrt{121 \cdot (-1)} = \sqrt{121} \cdot \sqrt{-1} \]
This allows us to express the square root of -121 in terms of the imaginary unit 'i'. Thus:
\[ \sqrt{-121} = 11 \cdot i \]
Combining Real and Imaginary Parts
To simplify the square root of -121, we need to combine the real and imaginary parts of the number. This involves using the concept of imaginary numbers and the imaginary unit 'i', where:
\[ i = \sqrt{-1} \]
Let's go through the process step by step:
First, identify the square root of the positive part of the number. In this case, the positive part is 121, and its square root is 11:
\[ \sqrt{121} = 11 \]Next, incorporate the imaginary unit 'i' to account for the negative part of the number. The square root of -1 is represented by 'i':
\[ \sqrt{-1} = i \]Combine these results to simplify the square root of -121. Multiply the square root of the positive part by the imaginary unit:
\[ \sqrt{-121} = \sqrt{121} \cdot \sqrt{-1} = 11 \cdot i \]
Therefore, the simplified form of \( \sqrt{-121} \) is:
\[ 11i \]
This process of combining the real part (11) and the imaginary part (i) gives us the final simplified form, showing how real and imaginary components interact to represent the square root of a negative number.
Final Simplified Form of √-121
To find the final simplified form of \( \sqrt{-121} \), we will use the concepts of perfect squares and imaginary numbers. Here are the steps to achieve this:
Recognize that the number 121 is a perfect square. The square root of 121 is 11:
\[ \sqrt{121} = 11 \]Understand that the square root of a negative number involves the imaginary unit 'i', which is defined as:
\[ i = \sqrt{-1} \]Combine the real part (11) with the imaginary part (i) to simplify \( \sqrt{-121} \). This is done by multiplying the square root of the positive part by the imaginary unit:
\[ \sqrt{-121} = \sqrt{121} \cdot \sqrt{-1} \]Substituting the values, we get:
\[ \sqrt{-121} = 11 \cdot i \]
Thus, the final simplified form of \( \sqrt{-121} \) is:
\[ 11i \]
This shows that when we take the square root of a negative number, we extract the square root of the positive part and multiply it by the imaginary unit 'i', resulting in a complex number.

Practical Applications of Imaginary Numbers
Imaginary numbers, and by extension complex numbers, have numerous practical applications in various fields of science and engineering. Here are some key areas where they are used:
Electrical Engineering: Imaginary numbers are crucial in the analysis of electrical circuits, particularly in the study of alternating current (AC) circuits. They help in representing the phase difference between voltage and current using complex numbers. The impedance in AC circuits is often expressed as a complex number.
Signal Processing: In signal processing, complex numbers are used to analyze and manipulate signals. The Fourier transform, which is a fundamental tool in signal processing, converts signals from the time domain to the frequency domain using complex numbers.
Control Systems: Imaginary numbers play a vital role in control theory, which deals with the behavior of dynamic systems. They are used to design and analyze systems for stability and performance through techniques such as the Laplace transform.
Quantum Mechanics: In quantum mechanics, the state of a system is described by a wave function, which often involves complex numbers. The Schrödinger equation, which governs the behavior of quantum systems, relies on complex numbers for its solutions.
Fluid Dynamics: Complex numbers are used in fluid dynamics to solve problems related to fluid flow. Potential flow theory, which is used to analyze the flow of incompressible fluids, employs complex functions to describe the flow patterns.
Vibration Analysis: In mechanical engineering, complex numbers are used to analyze vibrations in systems. The use of complex notation simplifies the calculations of oscillatory systems and helps in understanding the resonance behavior.
These applications demonstrate how imaginary numbers extend beyond theoretical mathematics and are integral to solving real-world problems. Their ability to simplify and solve complex equations makes them invaluable tools in various scientific and engineering disciplines.
Common Mistakes and How to Avoid Them
Simplifying the square root of a negative number can be tricky, and there are several common mistakes students often make. Here are some of those mistakes along with tips on how to avoid them:
-
Mistake: Ignoring the Imaginary Unit 'i'
One common mistake is to forget to include the imaginary unit i when simplifying the square root of a negative number. For example, simplifying
√-121without considering the i results in an incorrect solution.How to Avoid: Always remember that the square root of a negative number introduces the imaginary unit i. For
√-121, the correct simplification is11i. -
Mistake: Incorrect Application of Square Root Properties
Another mistake is incorrectly applying the properties of square roots to negative numbers. For instance, assuming
√(a * b) = √a * √bholds for negative a and b can lead to errors.How to Avoid: Recognize that the property
√(a * b) = √a * √bis only valid for non-negative a and b. For negative numbers, first separate the negative factor as√(-1) = iand then simplify the positive part. -
Mistake: Confusing the Principal Root
Some students might mix up the principal root with other roots, especially in the context of imaginary numbers. The principal root is always the non-negative root.
How to Avoid: Understand that the principal square root is defined as the non-negative root. For example, the principal square root of
121is11, not-11. Hence,√121 = 11and thus√-121 = 11i. -
Mistake: Misinterpreting Complex Conjugates
In more advanced problems involving complex numbers, students sometimes confuse complex conjugates with roots of negative numbers.
How to Avoid: Differentiate between the concept of a complex conjugate and the imaginary unit. The complex conjugate of a number
a + biisa - bi, whereas the square root of a negative number is always of the formbi.
By being mindful of these common mistakes and understanding the correct procedures, you can avoid errors and simplify expressions involving square roots of negative numbers accurately.
Further Reading and Resources
To deepen your understanding of simplifying the square root of negative numbers, consider exploring the following resources:
-
Khan Academy - Simplifying roots of negative numbers: This video tutorial explains how to handle square roots of negative numbers and covers the concept of the imaginary unit 'i' in detail.
-
Mathway - Algebra Examples: This step-by-step guide simplifies the square root of -121, illustrating the process clearly and concisely.
-
Mathwarehouse - Square Root Calculator: Use this calculator to simplify any square root, including negative ones, and explore additional resources on simplifying radicals.
-
Virtual Nerd - Imaginary and Complex Numbers: This tutorial helps you understand how to simplify the square root of a negative number and includes background information on factors, square roots, and prime factorization.
Video này hướng dẫn cách đơn giản hóa căn bậc hai của các số âm như -121, -24 và -200, giúp bạn hiểu rõ hơn về khái niệm số ảo 'i' và các bước tính toán chi tiết.
Đơn giản hóa Căn Bậc Hai của -121, -24 và -200 (Căn Bậc Hai của Số Âm)
READ MORE:
Video này hướng dẫn cách đơn giản hóa căn bậc hai của các số âm như -121, -75 và -96, giúp bạn hiểu rõ hơn về khái niệm số ảo 'i' và các bước tính toán chi tiết.
Đơn giản hóa Căn Bậc Hai của -121, -75 và -96 (Căn Bậc Hai của Số Âm)