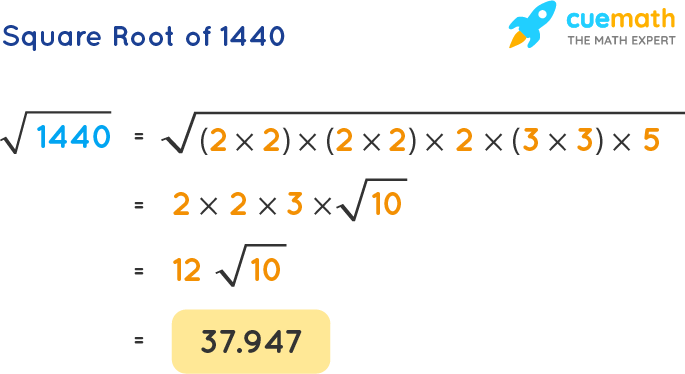Discover the Square Root of 1960: Methods and Applications

The square root of 1960 is approximately:

\(\sqrt{1960} \approx 44.27189\)

The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 9 is 3 because \(3 \times 3 = 9\). In mathematical notation, the square root of a number \(x\) is represented as \(\sqrt{x}\).

Square roots are fundamental in various mathematical fields, including algebra, geometry, and calculus. Understanding how to calculate and use square roots is essential for solving many types of problems.

Here are some key points about square roots:

• The square root of a positive number is always a positive number or zero.
• Every positive number has two square roots: one positive and one negative. For example, the square roots of 16 are 4 and -4 because \(4 \times 4 = 16\) and \(-4 \times -4 = 16\).
• The square root of zero is zero.
• Square roots of negative numbers are not real numbers. They are represented as imaginary numbers. For example, the square root of -1 is represented as \(i\), where \(i\) is the imaginary unit.

Calculating square roots can be done using various methods, including:

1. Prime Factorization: Breaking down the number into its prime factors and pairing them to find the square root.
2. Long Division Method: A manual method similar to long division, used to find square roots of larger numbers.
3. Using a Calculator: The simplest method, especially for complex or large numbers.

Understanding the concept of square roots is crucial for advancing in mathematics and solving real-world problems effectively.

The square root of a number is a value that, when multiplied by itself, yields the original number. Mathematically, if \( x \) is the square root of \( y \), then \( x \times x = y \). This is expressed using the radical symbol as \( x = \sqrt{y} \).

The concept of square roots can be detailed as follows:

• Positive and Negative Roots: Every positive number \( y \) has two square roots: a positive square root and a negative square root. This is because both \( x \) and \( -x \) satisfy the equation \( x^2 = y \). For example, the square roots of 25 are \( 5 \) and \( -5 \).
• Principal Square Root: The principal square root is the non-negative square root of a number. It is denoted by \( \sqrt{y} \). For example, the principal square root of 25 is 5.
• Imaginary Numbers: If \( y \) is negative, the square root of \( y \) is an imaginary number. Imaginary numbers are defined using the imaginary unit \( i \), where \( i = \sqrt{-1} \). For example, the square root of -4 is \( 2i \).

To better understand the square root, consider the following table of some common numbers and their square roots:

In summary, the square root function reverses the squaring function. It is a fundamental mathematical operation with applications in various fields such as engineering, physics, and finance.

Calculating the square root of a number can be approached through various methods. Here, we outline several common techniques:

1. Prime Factorization Method

This method involves breaking down the number into its prime factors and pairing them to find the square root. For example, to find the square root of 1960:

• First, find the prime factors of 1960: \(1960 = 2^2 \times 5 \times 7^2\).
• Next, pair the prime factors: \( (2 \times 7) \times (2 \times 7) = 14 \times 14 \).
• The square root of 1960 is therefore \( \sqrt{1960} = 14 \sqrt{5} \), since 5 is not a perfect square.
2. Long Division Method

This method is a manual technique similar to long division. Here are the steps:

• Group the digits of the number in pairs from right to left. For 1960, it becomes (19)(60).
• Find the largest number whose square is less than or equal to the first pair (19). This number is 4 because \( 4^2 = 16 \).
• Subtract 16 from 19 to get the remainder 3, then bring down the next pair (60) to get 360.
• Double the quotient obtained (4) to get 8. Find a digit \( x \) such that \( 8x \times x \leq 360 \). Here, \( x = 4 \) since \( 84 \times 4 = 336 \).
• Subtract 336 from 360 to get the remainder 24. Since there are no more pairs to bring down, the quotient is 44.
• The square root of 1960 is approximately 44.
3. Using a Calculator

The simplest and most efficient method is to use a calculator:

• Enter the number (1960) into the calculator.
• Press the square root (√) button.
• The calculator will display the square root of 1960, which is approximately 44.27.

Each of these methods has its own applications and advantages, allowing for flexibility in calculating square roots based on the tools and information available.

The square root of 1960 is a number which, when multiplied by itself, equals 1960. To find this value, we can use several methods, including prime factorization, the long division method, and using a calculator. Below, we explore these methods step-by-step:

1. Prime Factorization Method

This method involves expressing 1960 as a product of its prime factors:

• First, determine the prime factors of 1960: \(1960 = 2^2 \times 5 \times 7^2\).
• Pair the prime factors: \( (2 \times 7) \times (2 \times 7) = 14 \times 14 \).
• Since 5 is not a perfect square, it remains under the square root: \( \sqrt{1960} = 14 \sqrt{5} \).

Therefore, the exact square root of 1960 using prime factorization is \(14 \sqrt{5}\), approximately equal to 44.27.

2. Long Division Method

This manual method is effective for larger numbers:

• Group the digits in pairs from right to left: (19)(60).
• Find the largest number whose square is less than or equal to the first pair (19). This number is 4 because \( 4^2 = 16 \).
• Subtract 16 from 19 to get 3, then bring down the next pair (60) to get 360.
• Double the quotient obtained (4) to get 8. Find a digit \( x \) such that \( 8x \times x \leq 360 \). Here, \( x = 4 \) since \( 84 \times 4 = 336 \).
• Subtract 336 from 360 to get 24. Since there are no more pairs to bring down, the quotient is 44.
• The square root of 1960 is approximately 44.
3. Using a Calculator

The most straightforward method is to use a calculator:

• Enter 1960 into the calculator.
• Press the square root (√) button.
• The calculator will display the square root of 1960, which is approximately 44.27.

In summary, the square root of 1960 is \( \sqrt{1960} \approx 44.27 \). This value can be calculated using different methods, each offering a unique approach to understanding and solving square roots.

Calculating the square root of 1960 manually can be accomplished using the long division method. Here is a step-by-step guide to this technique:

1. Group the Digits

First, group the digits of 1960 in pairs from right to left: (19)(60).

2. Find the Largest Square

Find the largest number whose square is less than or equal to the first pair (19). This number is 4 because \(4^2 = 16\).

• Write 4 as the first digit of the quotient.
• Subtract 16 from 19 to get 3, and bring down the next pair (60) to get 360.
3. Double the Quotient

Double the quotient obtained so far (4) to get 8.

• Find a digit \(x\) such that \(8x \times x \leq 360\). Here, \(x = 4\) because \(84 \times 4 = 336\).
• Write 4 as the next digit of the quotient, making it 44.
• Subtract 336 from 360 to get a remainder of 24.
4. Repeat the Process

Since there are no more pairs of digits to bring down, the calculation ends here with the quotient 44 and a remainder of 24.

5. Approximation

For a more precise value, you can continue the process by adding decimal places and bringing down pairs of zeros. This will give you a more accurate approximation.

Using the long division method, we find that the square root of 1960 is approximately 44. To improve precision, the process can be extended, yielding a result closer to the exact value of 44.27.

Finding the square root of 1960 using a calculator is a quick and straightforward process. Here are the steps to follow:

1. Turn On the Calculator

Ensure your calculator is powered on and functioning correctly.

2. Enter the Number

Input the number 1960 using the calculator's keypad.

3. Locate the Square Root Button

Find the square root button on your calculator. This is usually represented by the symbol \( \sqrt{} \).

4. Press the Square Root Button

Press the square root button. The calculator will perform the calculation and display the result.

5. Read the Result

The calculator will show the square root of 1960, which is approximately 44.27188724235731.

This method is not only efficient but also ensures accuracy, making it the preferred choice for quickly finding square roots, especially for large or non-perfect square numbers.

Square roots play a vital role in various real-life applications, ranging from engineering to everyday problem-solving. Here are some key areas where square roots are commonly used:

1. Architecture and Engineering

Square roots are essential in calculating areas and volumes, which is crucial for designing buildings and structures. For example, determining the length of the diagonal of a square plot requires the square root function.

2. Physics

In physics, square roots are used in formulas involving gravitational force, kinetic energy, and wave equations. For instance, the formula for calculating the period of a pendulum involves the square root of its length.

3. Finance

Square roots are used in financial calculations, such as determining compound interest and analyzing investment returns. The standard deviation, a measure of investment risk, is derived using square roots.

4. Computer Graphics

In computer graphics, square roots are used to calculate distances between points, gradients, and magnitudes of vectors. These calculations are fundamental for rendering images and animations accurately.

5. Medicine

Square roots are used in various medical calculations, including determining body mass index (BMI) and drug dosages. Accurate measurements are crucial for effective treatment and patient safety.

6. Surveying and Navigation

Surveyors and navigators use square roots to calculate distances between geographical points. This is essential for creating maps, planning routes, and measuring land areas.

7. Statistics

In statistics, square roots are used to calculate standard deviation and variance, which are measures of data dispersion. These metrics help in understanding the spread and reliability of data.

Overall, square roots are a fundamental mathematical tool with wide-ranging applications that make them indispensable in both professional fields and everyday life.

Calculating square roots can sometimes lead to common errors, especially for those new to the concept. Here are some frequent mistakes to watch out for:

• Ignoring Both Positive and Negative Roots: When calculating the square root of a positive number, remember there are always two roots: a positive and a negative one. For example, both \( \sqrt{9} = 3 \) and \( \sqrt{9} = -3 \) because \(3^2 = 9\) and \((-3)^2 = 9\).
• Confusing Squares and Square Roots: Do not confuse squaring a number with finding its square root. Squaring a number means multiplying it by itself, while finding the square root is determining what number, when multiplied by itself, gives the original number.
• Incorrect Use of Calculators: Make sure to use the correct function on your calculator. Using the general exponentiation function instead of the square root function can lead to errors. For instance, \( 1960^{0.5} \) should be used to find \( \sqrt{1960} \).
• Assuming Non-Terminating Decimals are Always Large: Understanding irrational numbers is crucial. An irrational number, such as the square root of a non-perfect square (like \( \sqrt{1960} \approx 44.272 \)), is a non-terminating decimal, but this does not mean it is a large number.
• Forgetting to Simplify Radicals: When possible, simplify the square root. For instance, \( \sqrt{50} \) can be simplified to \( 5\sqrt{2} \).
• Incorrect Prime Factorization: This method is useful for perfect squares but can be misapplied. Ensure you use the long division method for non-perfect squares to avoid inaccuracies.

By keeping these common mistakes in mind, you can improve your accuracy in calculating square roots.

Understanding the square root of 1960 offers a clear example of the importance and application of mathematical principles in various contexts. The square root of 1960 is approximately 44.27, and it is an irrational number, meaning it cannot be expressed as a simple fraction. This value can be used in different mathematical problems and real-life scenarios.

Through this exploration, we learned different methods to calculate square roots, including manual calculations and the use of calculators. Manual methods, like the long division method, provide insight into the mechanics behind the calculation, while calculators offer a quick and accurate solution.

Knowing how to calculate and apply square roots is essential in many fields, such as engineering, physics, and everyday problem-solving. For instance, understanding that the square root of the area of a square gives the side length can help in practical tasks like determining the dimensions of a space.

In conclusion, mastering the concept of square roots, including their calculation and application, enhances one's mathematical toolkit, enabling more efficient and accurate problem-solving. Whether through manual methods or modern technology, the ability to determine the square root of numbers like 1960 is a valuable skill in both academic and real-world settings.