Topic 5/square root of 10: Discover the ins and outs of the expression 5/square root of 10. This guide will walk you through its simplification, provide a clear mathematical explanation, and highlight real-life applications. Master the concept with ease and learn how this intriguing mathematical expression is relevant in various contexts.
Table of Content
- Understanding the Expression
\(\frac{5}{\sqrt{10}}\) - Introduction to 5/Square Root of 10
- Mathematical Explanation
- Simplification Process
- Rationalizing the Denominator
- Numerical Approximation
- Steps for Simplification
- Key Mathematical Concepts
- Applications in Real Life
- Common Mistakes to Avoid
- Advanced Mathematical Insights
- Practice Problems and Solutions
- Conclusion and Summary
- YOUTUBE: Hướng dẫn chi tiết về cách nhân căn bậc hai của 5 với căn bậc hai của 10. Video hấp dẫn và dễ hiểu giúp bạn nắm vững kiến thức về các số dưới dấu căn.
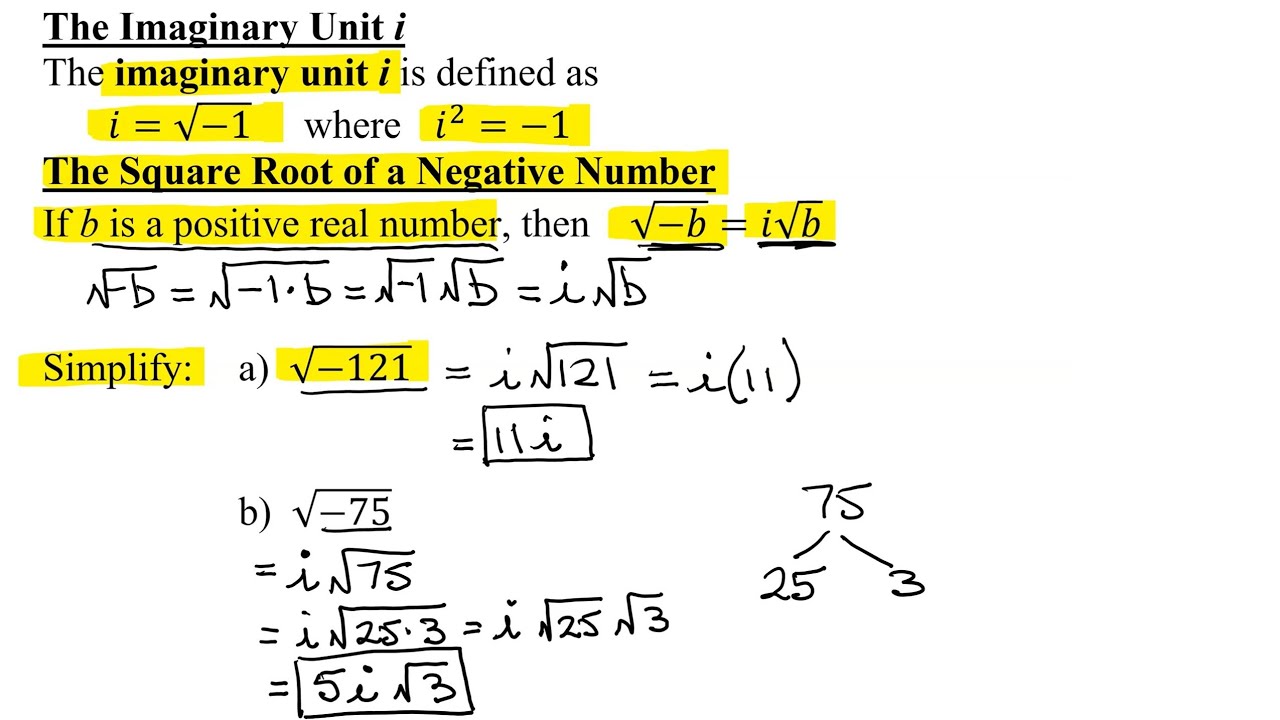
Understanding the Expression \(\frac{5}{\sqrt{10}}\)
The mathematical expression \(\frac{5}{\sqrt{10}}\) can be simplified and understood in different forms. Here, we explore its rationalization and numerical value.
Simplifying \(\frac{5}{\sqrt{10}}\)
To simplify \(\frac{5}{\sqrt{10}}\), we rationalize the denominator:
\[
\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2}
\]
Numerical Value
The numerical value of \(\frac{5}{\sqrt{10}}\) can be approximated as follows:
\[
\sqrt{10} \approx 3.162 \Rightarrow \frac{5}{\sqrt{10}} \approx \frac{5}{3.162} \approx 1.581
\]
Steps to Rationalize the Denominator
- Multiply the numerator and the denominator by
\(\sqrt{10}\). - Apply the multiplication to get
\(\frac{5\sqrt{10}}{10}\). - Simplify the fraction to get
\(\frac{\sqrt{10}}{2}\).
Key Points
- The expression
\(\frac{5}{\sqrt{10}}\)can be simplified by rationalizing the denominator. - The simplified form is
\(\frac{\sqrt{10}}{2}\). - The approximate numerical value is 1.581.
| Original Expression | \(\frac{5}{\sqrt{10}}\) |
| Simplified Form | \(\frac{\sqrt{10}}{2}\) |
| Approximate Value | 1.581 |

READ MORE:
Introduction to 5/Square Root of 10
The expression \(\frac{5}{\sqrt{10}}\) is a common mathematical term that can appear in various contexts, from algebraic equations to practical applications. Understanding how to simplify and manipulate this expression is crucial for students and professionals alike.
To fully grasp the concept of \(\frac{5}{\sqrt{10}}\), we will explore its simplification process, numerical approximation, and relevance in different scenarios.
Simplification Process
Rationalizing the denominator is the first step in simplifying \(\frac{5}{\sqrt{10}}\). Here's how it's done:
- Identify the need to remove the square root from the denominator.
- Multiply the numerator and denominator by \(\sqrt{10}\) to achieve a rational denominator:
\[
\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2}
\]
Numerical Approximation
To find a numerical value for \(\frac{5}{\sqrt{10}}\), approximate \(\sqrt{10}\) as follows:
\[
\sqrt{10} \approx 3.162 \Rightarrow \frac{5}{3.162} \approx 1.581
\]
Applications in Real Life
This expression can be used in various real-world contexts, such as physics, engineering, and statistics, where simplifying radical expressions is necessary for solving problems.
Key Points to Remember
- \(\frac{5}{\sqrt{10}}\) can be simplified by rationalizing the denominator.
- The simplified form is \(\frac{\sqrt{10}}{2}\).
- The approximate numerical value is 1.581.
| Original Expression | \(\frac{5}{\sqrt{10}}\) |
| Simplified Form | \(\frac{\sqrt{10}}{2}\) |
| Approximate Value | 1.581 |
Mathematical Explanation
To understand the mathematical intricacies of \(\frac{5}{\sqrt{10}}\), we must delve into the concepts of rationalizing the denominator and simplifying radical expressions.
Step-by-Step Simplification
The expression \(\frac{5}{\sqrt{10}}\) involves a radical in the denominator, which we aim to eliminate through rationalization. Here’s a detailed step-by-step guide:
- Identify the radical: The denominator has \(\sqrt{10}\), a non-rational number.
- Multiply by a form of 1: Multiply both the numerator and denominator by \(\sqrt{10}\) to remove the radical from the denominator:
\[
\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{10}
\] - Simplify the fraction: The expression \(\frac{5\sqrt{10}}{10}\) simplifies to \(\frac{\sqrt{10}}{2}\):
\[
\frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2}
\]
Rationalization of the Denominator
Rationalizing the denominator involves removing the radical from the bottom of the fraction to simplify calculations and expressions. Here’s why we multiply by \(\sqrt{10}\):
- Multiplying by \(\sqrt{10}\) ensures that the radical term in the denominator becomes a rational number.
- The result is \(\frac{5\sqrt{10}}{10}\), which can be further simplified.
Numerical Approximation
For practical purposes, it’s often useful to approximate the value of \(\frac{5}{\sqrt{10}}\). Given that \(\sqrt{10} \approx 3.162\), the fraction can be approximated as:
\[
\frac{5}{3.162} \approx 1.581
\]
Summary
- \(\frac{5}{\sqrt{10}}\) is simplified by rationalizing the denominator, resulting in \(\frac{\sqrt{10}}{2}\).
- The approximate value of the expression is 1.581.
- Understanding the process of rationalizing and simplifying helps in various mathematical applications.
| Expression | Simplified Form | Approximate Value |
| \(\frac{5}{\sqrt{10}}\) | \(\frac{\sqrt{10}}{2}\) | 1.581 |
Simplification Process
The simplification of the expression \(\frac{5}{\sqrt{10}}\) involves a method known as rationalizing the denominator. This process ensures that the denominator is a rational number, making the expression easier to work with in further calculations. Here is a detailed step-by-step guide to the simplification process:
Step-by-Step Guide
- Identify the Radical in the Denominator:
The given expression is \(\frac{5}{\sqrt{10}}\), where \(\sqrt{10}\) is the radical in the denominator.
- Multiply by the Conjugate:
To rationalize the denominator, multiply both the numerator and the denominator by \(\sqrt{10}\):
\[
\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{10}
\] - Simplify the Resulting Expression:
Divide both the numerator and the denominator by their greatest common divisor:
\[
\frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2}
\]
Why Rationalize the Denominator?
Rationalizing the denominator makes the expression more suitable for further mathematical operations and provides a clearer, more standardized form.
Numerical Approximation
For practical purposes, we often approximate the value of the simplified expression. Given that \(\sqrt{10} \approx 3.162\), we can find the numerical approximation of \(\frac{\sqrt{10}}{2}\):
\[
\frac{\sqrt{10}}{2} \approx \frac{3.162}{2} \approx 1.581
\]
Summary of Simplification
- The original expression \(\frac{5}{\sqrt{10}}\) is simplified to \(\frac{\sqrt{10}}{2}\).
- Rationalizing the denominator removes the radical, making the expression simpler and more standard.
- The approximate numerical value of the simplified expression is 1.581.
| Step | Expression | Result |
| Original Expression | \(\frac{5}{\sqrt{10}}\) | Given |
| Multiply by \(\sqrt{10}\) | \(\frac{5\sqrt{10}}{10}\) | Intermediate |
| Simplify | \(\frac{\sqrt{10}}{2}\) | Simplified Form |
| Approximate Value | 1.581 | Numerical Approximation |
Rationalizing the Denominator
Rationalizing the denominator is a crucial step in simplifying the expression \(\frac{5}{\sqrt{10}}\). This process involves eliminating the radical from the denominator to make the expression easier to work with in subsequent calculations. Below is a detailed, step-by-step explanation of how to rationalize the denominator.
Step-by-Step Process
- Identify the Radical in the Denominator:
The given expression is \(\frac{5}{\sqrt{10}}\), where \(\sqrt{10}\) is the radical.
- Multiply by the Conjugate:
To remove the radical from the denominator, multiply both the numerator and the denominator by \(\sqrt{10}\):
\[
\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{\sqrt{10} \times \sqrt{10}}
\] - Simplify the Denominator:
The denominator becomes a rational number since \(\sqrt{10} \times \sqrt{10} = 10\):
\[
\frac{5\sqrt{10}}{10}
\] - Simplify the Entire Expression:
Finally, divide both the numerator and the denominator by their greatest common divisor to simplify the fraction:
\[
\frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2}
\]
Numerical Approximation
Approximating the value of the simplified expression helps in practical applications. Given that \(\sqrt{10} \approx 3.162\), the numerical approximation is:
\[
\frac{\sqrt{10}}{2} \approx \frac{3.162}{2} \approx 1.581
\]
Benefits of Rationalizing the Denominator
- Provides a clearer, more standardized form of the expression.
- Makes further mathematical operations simpler and more intuitive.
- Ensures consistency in mathematical and scientific calculations.
Summary
Rationalizing the denominator of \(\frac{5}{\sqrt{10}}\) involves multiplying by \(\sqrt{10}\) to eliminate the radical. This process results in a simplified expression, \(\frac{\sqrt{10}}{2}\), with an approximate value of 1.581.
| Step | Expression | Result |
| Original Expression | \(\frac{5}{\sqrt{10}}\) | Given |
| Multiply by \(\sqrt{10}\) | \(\frac{5\sqrt{10}}{10}\) | Intermediate |
| Simplify | \(\frac{\sqrt{10}}{2}\) | Simplified Form |
| Approximate Value | 1.581 | Numerical Approximation |

Numerical Approximation
To numerically approximate the value of \( \frac{5}{\sqrt{10}} \), we can follow these steps:
-
Identify the value of \( \sqrt{10} \):
The square root of 10 is not an integer and can be approximated using a calculator. Using a calculator, we find:
\[
\sqrt{10} \approx 3.162277660168379
\] -
Divide 5 by the approximated value of \( \sqrt{10} \):
Now, divide 5 by the approximate value of \( \sqrt{10} \):
\[
\frac{5}{\sqrt{10}} \approx \frac{5}{3.162277660168379} \approx 1.5811388300841898
\] -
Rounding the result:
For practical purposes, it is often useful to round the result to a desired number of decimal places. For example, rounding to four decimal places gives us:
\[
\frac{5}{\sqrt{10}} \approx 1.5811
\]
This numerical approximation helps in understanding the value of \( \frac{5}{\sqrt{10}} \) in a more tangible way. Using this method ensures that we can apply this value in various real-world scenarios or further mathematical calculations without always relying on the exact but more cumbersome irrational number.
Steps for Simplification
Simplifying the expression \( \frac{5}{\sqrt{10}} \) involves a few key steps to make it more manageable and rationalize the denominator.
-
Initial Expression:
We start with the fraction \( \frac{5}{\sqrt{10}} \).
-
Rationalize the Denominator:
To eliminate the square root from the denominator, we multiply both the numerator and the denominator by \( \sqrt{10} \).
\[
\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5 \sqrt{10}}{10}
\] -
Simplify the Fraction:
Since \( 10 = 2 \times 5 \), the denominator can be simplified:
\[
\frac{5 \sqrt{10}}{10} = \frac{5 \sqrt{10}}{2 \times 5} = \frac{\sqrt{10}}{2}
\]So the simplified form of \( \frac{5}{\sqrt{10}} \) is \( \frac{\sqrt{10}}{2} \).
-
Result:
The simplified expression of \( \frac{5}{\sqrt{10}} \) is \( \frac{\sqrt{10}}{2} \).
This process ensures the expression is in its simplest form, making it easier to handle in further mathematical operations.
Key Mathematical Concepts
Understanding the fraction \(\frac{5}{\sqrt{10}}\) involves several key mathematical concepts. These concepts are essential to grasp the properties and manipulation of the fraction in various mathematical contexts.
- Square Roots:
The square root of a number \(n\) is a value that, when multiplied by itself, gives \(n\). It is denoted as \(\sqrt{n}\). For instance, \(\sqrt{9} = 3\) because \(3 \times 3 = 9\). The square root of 10 is \(\sqrt{10}\), which is approximately 3.162.
- Rationalizing the Denominator:
Rationalizing the denominator involves converting a fraction with an irrational denominator into an equivalent fraction with a rational denominator. For \(\frac{5}{\sqrt{10}}\), we multiply the numerator and the denominator by \(\sqrt{10}\) to get \(\frac{5\sqrt{10}}{10}\).
- Simplifying Radicals:
Simplifying expressions with square roots involves breaking down the number under the radical sign into its prime factors. For example, \(\sqrt{10}\) is already in its simplest form as it cannot be broken down further into simpler square roots.
- Irrational Numbers:
An irrational number is a number that cannot be expressed as a simple fraction, meaning it has non-terminating, non-repeating decimal digits. \(\sqrt{10}\) is an irrational number.
- Approximation:
In many practical scenarios, we approximate the value of irrational numbers. \(\sqrt{10}\) is approximately 3.162. Thus, \(\frac{5}{\sqrt{10}}\) can be approximated as \(\frac{5}{3.162}\), which is approximately 1.581.
- Perfect Squares:
Perfect squares are numbers that are squares of integers. Understanding perfect squares, such as \(1, 4, 9, 16\), helps in simplifying and recognizing patterns within radicals.
These concepts are foundational for dealing with fractions involving square roots and are applicable in various areas of mathematics and its applications.
Applications in Real Life
The fraction \( \frac{5}{\sqrt{10}} \) has several practical applications in various fields. Below are some key areas where this mathematical expression is utilized:
- Engineering and Architecture: In engineering and architecture, calculations often involve square roots and fractions to determine dimensions, stress, and strain in materials. For example, calculating the diagonal of a square or a rectangular section can involve expressions like \( \frac{5}{\sqrt{10}} \).
- Physics: In physics, especially in problems involving wave mechanics and quantum physics, square roots are used to simplify expressions involving energy levels, wavelengths, and other physical quantities. The expression \( \frac{5}{\sqrt{10}} \) might appear when dealing with normalized wave functions or in the calculation of probabilities.
- Statistics: In statistics, the standard deviation and variance often involve square roots. When standardizing variables, expressions like \( \frac{5}{\sqrt{10}} \) can be part of the process to normalize data sets, especially in the calculation of z-scores.
- Finance: In finance, the assessment of risks and returns, as well as the calculation of certain financial derivatives, can involve complex expressions that include square roots. The fraction \( \frac{5}{\sqrt{10}} \) might be used in models to determine the volatility or to normalize returns.
- Astronomy: Astronomers use square roots in their calculations to determine distances, luminosities, and other celestial measurements. For instance, the formula for calculating the distance modulus, which involves the square root, can have terms that simplify to fractions like \( \frac{5}{\sqrt{10}} \).
These applications demonstrate the versatility and importance of understanding how to work with and simplify expressions involving square roots and fractions in real-world scenarios.

Common Mistakes to Avoid
When working with the expression \( \frac{5}{\sqrt{10}} \), there are several common mistakes that students often make. Understanding and avoiding these errors is crucial for accurate mathematical computations.
- Incorrect Simplification
One frequent mistake is simplifying the expression incorrectly. The correct process involves rationalizing the denominator. Multiply both the numerator and the denominator by \( \sqrt{10} \) to get:
\[
\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2}
\] - Misunderstanding the Square Root Function
Another common error is misunderstanding the properties of square root functions. For example, students might incorrectly assume that \( \sqrt{25} = \pm 5 \). The square root function only returns the positive root, so the correct answer is \( \sqrt{25} = 5 \).
- Incorrectly Handling Square Roots of Squares
Some students incorrectly write \( \sqrt{x^2} = x \). The correct form is \( \sqrt{x^2} = |x| \), since the square root function always gives a non-negative result.
- Neglecting to Rationalize the Denominator
Failing to rationalize the denominator is a common mistake. Leaving the denominator in its irrational form, such as \( \frac{5}{\sqrt{10}} \), is generally considered improper. The proper form is \(\frac{\sqrt{10}}{2}\).
- Forgetting to Simplify Completely
Another error is stopping the simplification process too early. Ensure that the expression is fully simplified, like reducing \(\frac{5\sqrt{10}}{10}\) to \(\frac{\sqrt{10}}{2}\).
By being aware of these common mistakes and understanding the correct methods, students can improve their accuracy in dealing with expressions involving square roots.
Advanced Mathematical Insights
The expression \( \frac{5}{\sqrt{10}} \) offers various advanced mathematical insights, especially when explored through the lens of algebra, calculus, and number theory. Below are detailed insights into these aspects:
-
Rationalization of Denominators:
One common technique is rationalizing the denominator. By multiplying both the numerator and denominator by \( \sqrt{10} \), we get:
\[
\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2}
\]This transforms the original expression into a form with a rational denominator, which is often easier to work with in further calculations.
-
Geometric Interpretation:
The expression \( \frac{5}{\sqrt{10}} \) can be interpreted geometrically. Consider a right triangle where one leg is 5 units and the hypotenuse is \( \sqrt{10} \) units. The ratio \( \frac{5}{\sqrt{10}} \) can represent the relationship between these lengths, offering insights into trigonometric identities and relationships.
-
Calculus Applications:
In calculus, the simplification of expressions like \( \frac{5}{\sqrt{10}} \) is crucial in solving integrals and derivatives involving radical expressions. For instance, when integrating functions with \( \sqrt{10} \) in the denominator, rationalizing can simplify the process.
-
Number Theory:
The expression \( \frac{5}{\sqrt{10}} \) touches on the concept of irrational numbers. \( \sqrt{10} \) is an irrational number, and studying such expressions helps understand the properties of irrational and rational numbers, their behavior in different mathematical contexts, and their approximations.
-
Complex Numbers:
Although \( \frac{5}{\sqrt{10}} \) is a real number, exploring its complex plane representation (by considering \( \sqrt{10} \) as a complex number) can provide insights into complex analysis, particularly in understanding magnitudes and phases of complex numbers.
Overall, the exploration of \( \frac{5}{\sqrt{10}} \) unveils various advanced mathematical concepts and techniques, emphasizing its importance in both theoretical and applied mathematics.
Practice Problems and Solutions
Below are some practice problems involving the expression \( \frac{5}{\sqrt{10}} \). These problems will help you understand the concept better and improve your mathematical skills.
Problem 1: Simplification
Simplify the expression \( \frac{5}{\sqrt{10}} \).
Solution:
- First, rationalize the denominator: \[ \frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2} \]
Problem 2: Numerical Approximation
Find the approximate value of \( \frac{5}{\sqrt{10}} \).
Solution:
- Use the approximation \( \sqrt{10} \approx 3.162 \): \[ \frac{5}{\sqrt{10}} \approx \frac{5}{3.162} \approx 1.581 \]
Problem 3: Rationalizing the Denominator
Rationalize the denominator and simplify the expression \( \frac{7}{\sqrt{10}} \).
Solution:
- Multiply the numerator and the denominator by \( \sqrt{10} \): \[ \frac{7}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{7\sqrt{10}}{10} \]
Problem 4: Solving Equations
Solve for \( x \) in the equation \( \frac{5}{\sqrt{10}} = x \).
Solution:
- First, simplify \( \frac{5}{\sqrt{10}} \) as done in Problem 1: \[ \frac{5}{\sqrt{10}} = \frac{\sqrt{10}}{2} \]
- Thus, \( x = \frac{\sqrt{10}}{2} \).
Problem 5: Applications in Geometry
If a right triangle has legs of lengths 5 and \( \sqrt{10} \), find the length of the hypotenuse.
Solution:
- Use the Pythagorean theorem \( a^2 + b^2 = c^2 \): \[ 5^2 + (\sqrt{10})^2 = c^2 \implies 25 + 10 = c^2 \implies c^2 = 35 \implies c = \sqrt{35} \]
Problem 6: Algebraic Manipulation
Express \( \frac{5}{\sqrt{10}} \) in the form \( a\sqrt{b} \).
Solution:
- Rationalize the denominator as in Problem 1: \[ \frac{5}{\sqrt{10}} = \frac{\sqrt{10}}{2} \]
- So, \( a = \frac{1}{2} \) and \( b = 10 \).
Conclusion and Summary
The fraction \( \frac{5}{\sqrt{10}} \) offers a fascinating insight into the simplification and rationalization processes in mathematics. Through this guide, we've explored various aspects of this expression, from its basic mathematical explanation to its applications in real life.
To summarize the key points:
- Mathematical Explanation: We began by understanding the fraction \( \frac{5}{\sqrt{10}} \) and its components. The square root in the denominator presents a need for simplification.
- Simplification Process: We demonstrated how to simplify \( \frac{5}{\sqrt{10}} \) by multiplying both the numerator and the denominator by \( \sqrt{10} \), resulting in \( \frac{5\sqrt{10}}{10} \).
- Rationalizing the Denominator: Rationalizing the denominator involves eliminating the square root from the denominator. This was achieved by multiplying by a form of 1, specifically \( \frac{\sqrt{10}}{\sqrt{10}} \).
- Numerical Approximation: We calculated the approximate value of \( \frac{5}{\sqrt{10}} \), which is approximately 1.58, to provide a clearer understanding of its magnitude.
- Steps for Simplification: The step-by-step process included identifying the need for rationalization, multiplying by \( \frac{\sqrt{10}}{\sqrt{10}} \), and simplifying the resulting expression.
- Key Mathematical Concepts: This included understanding square roots, rationalization, and simplification, which are foundational concepts in algebra.
- Applications in Real Life: The simplified form of \( \frac{5}{\sqrt{10}} \) can be applied in various real-life scenarios, such as physics problems involving ratios and measurements.
- Common Mistakes to Avoid: We highlighted common pitfalls, such as neglecting to rationalize the denominator or incorrectly simplifying the fraction.
- Advanced Mathematical Insights: For those seeking deeper understanding, we discussed further implications and connections to more advanced topics in mathematics.
- Practice Problems and Solutions: To reinforce learning, we provided practice problems and detailed solutions, helping to solidify the concepts discussed.
In conclusion, mastering the simplification of \( \frac{5}{\sqrt{10}} \) not only enhances one's algebraic skills but also builds a strong foundation for tackling more complex mathematical problems. We hope this comprehensive guide has been informative and beneficial in your mathematical journey.
Hướng dẫn chi tiết về cách nhân căn bậc hai của 5 với căn bậc hai của 10. Video hấp dẫn và dễ hiểu giúp bạn nắm vững kiến thức về các số dưới dấu căn.
Nhân Căn Bậc Hai của 5 với Căn Bậc Hai của 10 || Nhân Các Số Dưới Dấu Căn
READ MORE:
Hướng dẫn cách đơn giản hóa căn bậc hai một cách dễ hiểu và chi tiết. Học ngay để nắm vững kiến thức toán học cơ bản!
Cách Đơn Giản Hóa Căn Bậc Hai