Topic square root of 10 simplified radical form: The square root of 10 in its simplified radical form is an intriguing mathematical concept. This article explores why \(\sqrt{10}\) cannot be simplified further, provides an approximation for practical use, and delves into its applications. Gain a deeper understanding of the square root of 10 and how to work with it effectively.
Table of Content
Simplifying the Square Root of 10
The square root of 10 cannot be simplified to remove the radical because 10 is not a perfect square and does not have any square factors other than 1. Therefore, the simplified radical form of the square root of 10 is simply:
\(\sqrt{10}\)
However, for educational purposes, here is a breakdown of why it cannot be simplified further:
Prime Factorization Method
To determine if a square root can be simplified, we can use prime factorization:
- Find the prime factors of 10. Since 10 = 2 × 5, the prime factors are 2 and 5.
- Check if there are any pairs of the same factor. In this case, there are no pairs because 10 is a product of two different primes.
Since there are no pairs, we cannot simplify the square root any further.
Approximating the Square Root of 10
While \(\sqrt{10}\) cannot be simplified, it can be approximated for practical purposes:
\(\sqrt{10} \approx 3.162\)
This approximation can be useful in various applications, but remember it is not the exact value.
Conclusion
In conclusion, the square root of 10 remains in its simplified radical form as \(\sqrt{10}\). Understanding the principles behind simplification can help in recognizing when a square root is already in its simplest form.

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, gives the original number. The square root symbol is denoted as \( \sqrt{} \), and for any positive number \( x \), the square root is written as \( \sqrt{x} \).
For instance, \( \sqrt{25} = 5 \) because \( 5 \times 5 = 25 \). Similarly, \( \sqrt{36} = 6 \) because \( 6 \times 6 = 36 \). This property is essential in various fields, including algebra, geometry, and real-world applications.
Square roots can be either rational or irrational numbers. A rational square root results in a whole number, while an irrational square root cannot be expressed as a simple fraction. An example of an irrational square root is \( \sqrt{10} \), which cannot be simplified to a precise fractional value.
Let's consider the square root of 10:
\[
\sqrt{10}
\]
The factors of 10 are 1, 2, 5, and 10. Since none of these factors are perfect squares (except 1), \( \sqrt{10} \) is already in its simplest radical form.
To understand the value better, we can approximate \( \sqrt{10} \) using the inequality method:
\[
3 < \sqrt{10} < 4
\]
By squaring 3 and 4, we get:
\[
9 < 10 < 16
\]
Hence, \( \sqrt{10} \) lies between 3 and 4. For a more accurate approximation, consider the decimal form, which is approximately 3.162.
In conclusion, square roots play a crucial role in mathematics by helping us solve equations and understand the properties of numbers. The square root of 10 is a prime example of an irrational number that illustrates these principles effectively.
Understanding the Square Root of 10
The square root of 10, denoted as \( \sqrt{10} \), is an irrational number, which means it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating. Let's delve into the specifics of this number and its properties.
Simplified Radical Form:
The number 10 is already in its simplest radical form because it cannot be broken down into smaller square roots. Unlike perfect squares such as 9 (which is \(3^2\)) or 16 (which is \(4^2\)), 10 does not have a whole number square root. Thus, \( \sqrt{10} \) remains as it is.
Decimal Approximation:
Using approximation methods, we can find that \( \sqrt{10} \approx 3.162 \). This approximation helps in practical calculations and provides a sense of the value of \( \sqrt{10} \).
Step-by-Step Approximation:
- Recognize that \( \sqrt{9} < \sqrt{10} < \sqrt{16} \) (i.e., \(3 < \sqrt{10} < 4\)).
- To refine this, consider the squares of numbers between 3 and 4. For instance, \(3.1^2 = 9.61\) and \(3.2^2 = 10.24\), so \( 3.1 < \sqrt{10} < 3.2 \).
- Further refining, we find that \(3.16^2 \approx 10\), thus \( \sqrt{10} \approx 3.16\).
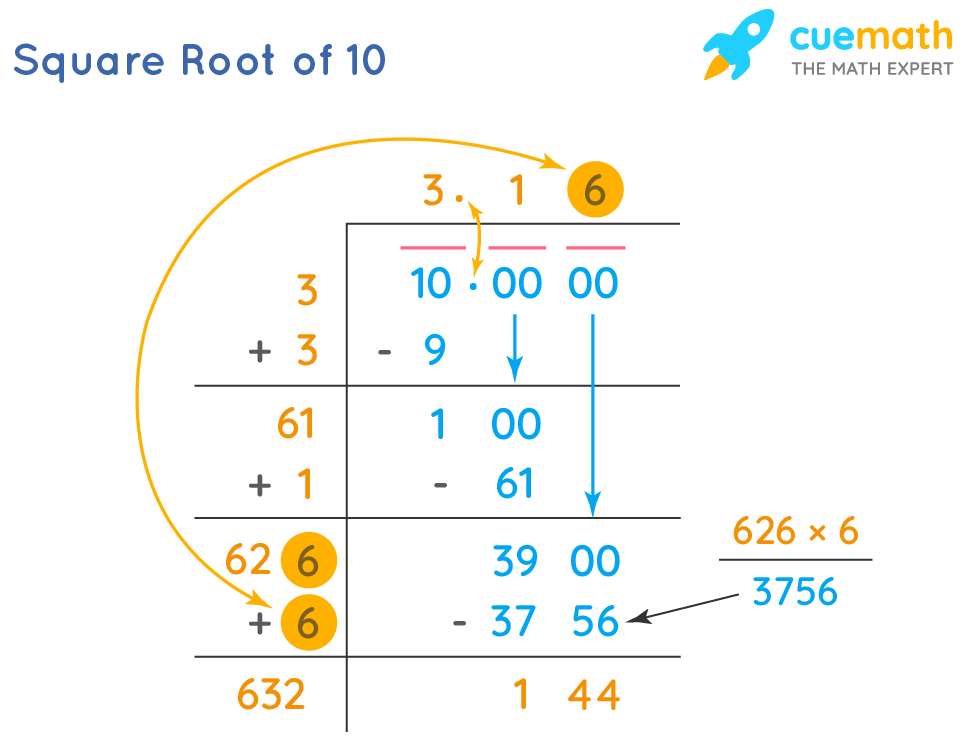
Long Division Method:
The long division method provides a more accurate value of \( \sqrt{10} \). Here's a concise overview of the steps involved:
- Start by dividing 10 by a number close to its square root (e.g., 3).
- Find the average of the result and the divisor to get a better approximation.
- Repeat the process with increased precision by adding decimal places.
- Continue until the desired level of accuracy is achieved.
Why the Square Root of 10 is Irrational:
The prime factorization of 10 is \(2 \times 5\). Since neither 2 nor 5 are perfect squares, \( \sqrt{10} \) cannot be simplified further and is classified as an irrational number.
Visualizing the Square Root of 10:
Imagine a square with an area of 10 square units. The length of each side of this square is \( \sqrt{10} \) units, which helps in understanding the geometric interpretation of this irrational number.
Understanding the square root of 10 involves recognizing its approximate value, its irrational nature, and how it fits into the broader context of square roots and radicals.
Why the Square Root of 10 Cannot Be Simplified
The square root of 10, denoted as \( \sqrt{10} \), is an interesting number to analyze. To understand why it cannot be simplified, let's delve into the concept of simplification and prime factorization.
Prime Factorization
To simplify a square root, we typically look for factors of the number that are perfect squares. Here's how we approach it for the number 10:
- List the factors of 10: 1, 2, 5, 10.
- Identify the perfect squares among these factors. In this case, the only perfect square is 1.
- Since there are no other perfect squares, we cannot simplify \( \sqrt{10} \) further.
Mathematical Explanation
The simplification of a square root involves expressing it in the form \( a\sqrt{b} \), where \( b \) has no square factors other than 1. However, for 10:
\[
10 = 2 \times 5
\]
Neither 2 nor 5 is a perfect square, thus \( \sqrt{10} \) cannot be simplified using prime factorization.
Irrational Nature of \( \sqrt{10} \)
Another reason \( \sqrt{10} \) cannot be simplified is because it is an irrational number. This means it cannot be expressed as a simple fraction and its decimal representation is non-terminating and non-repeating. The decimal approximation of \( \sqrt{10} \) is approximately 3.16228.
Conclusion
Therefore, the square root of 10 remains in its simplest radical form as \( \sqrt{10} \). Its unique nature makes it an important number in various mathematical and scientific applications, despite the fact that it cannot be simplified further.
Practical Applications of the Square Root of 10
The square root of 10, approximately 3.162, has several practical applications in various fields. Understanding these applications helps appreciate the importance of this mathematical constant in real-world scenarios.
- Geometry and Trigonometry: In geometry, the square root of 10 can be used to calculate distances and dimensions. For instance, in right triangles, it may appear in the calculation of side lengths when using the Pythagorean theorem.
- Physics: In physics, the square root of 10 can be used in formulas to determine quantities such as acceleration, velocity, and kinetic energy. It can be particularly useful when dealing with equations involving square roots and quadratic expressions.
- Engineering: Engineers use the square root of 10 in structural calculations and design. For example, determining the diagonal support in a rectangular frame or calculating stress and strain in materials often involves square root calculations.
- Computer Science: In computer graphics and game development, calculating distances between points in a 2D or 3D space frequently involves the square root of 10. The distance formula, which derives from the Pythagorean theorem, is essential for rendering realistic graphics.
- Construction: In construction, precise measurements are crucial. The square root of 10 can help in determining lengths and angles in design plans, ensuring accuracy in the layout and construction of buildings and structures.
- Statistics and Probability: In statistics, the square root of 10 might be used in standard deviation and variance calculations. It helps in understanding the spread of data points around the mean, providing insights into data distribution.
- Finance: In finance, square roots are used in risk assessment and financial modeling. Calculating volatility or the rate of return over time can involve square root functions to determine the potential risks and rewards of investments.
Overall, the square root of 10 plays a vital role in various disciplines, making it a crucial concept to understand and apply.
Visualizing the Square Root of 10
Visualizing the square root of 10 can be quite fascinating. Since the square root of 10 is an irrational number, it cannot be expressed as a simple fraction. Instead, it can be approximated and represented visually in several ways.
1. Number Line Representation
One of the simplest ways to visualize the square root of 10 is on a number line. To do this, identify the perfect squares nearest to 10, which are 9 and 16. The square roots of these numbers are 3 and 4, respectively. Therefore, we know that the square root of 10 lies between 3 and 4.
By placing a point between 3 and 4 on the number line, closer to 3.16, we can approximate the position of √10.
2. Geometric Representation
Another way to visualize √10 is geometrically. Consider a right-angled triangle with one side of 1 unit and the other side of 3 units. According to the Pythagorean theorem, the hypotenuse of this triangle would be the square root of (12 + 32) = √10. This triangle can help in visualizing √10 as the length of the hypotenuse.
3. Using a Circle
We can also use a circle to visualize the square root of 10. Draw a circle with a radius of √10 units. The circumference of this circle will be 2π√10, which can help to understand the magnitude of √10 in relation to the circle’s radius.
4. Graphical Plot
A graphical plot can be used to approximate √10. By plotting the function y = √x and finding the point where x = 10, the y-coordinate will give us the value of √10. This method provides a clear visual understanding of how √10 compares to other square roots.
5. Using the Long Division Method
The long division method is another approach to approximate √10 more accurately. By performing the long division, we find that √10 is approximately 3.162. This value can then be plotted or used in various calculations to visualize its magnitude.
By using these different methods, we can get a better visual and conceptual understanding of the square root of 10.
Comparing Square Roots: 10 and Other Numbers
Understanding and comparing square roots of different numbers helps in grasping their relative sizes and properties. Below, we compare the square root of 10 with the square roots of other numbers.
- Square Root of 10: \( \sqrt{10} = 3.162 \)
- Square Root of 4: \( \sqrt{4} = 2 \)
- Square Root of 9: \( \sqrt{9} = 3 \)
- Square Root of 16: \( \sqrt{16} = 4 \)
From the above values, we observe that \( \sqrt{10} \) lies between \( \sqrt{9} \) and \( \sqrt{16} \). This indicates that 10 is not a perfect square, and its square root is an irrational number that is slightly greater than 3 but less than 4.
Detailed Comparison with Other Square Roots
| Number | Square Root | Approximate Value |
|---|---|---|
| 1 | \( \sqrt{1} \) | 1 |
| 2 | \( \sqrt{2} \) | 1.414 |
| 3 | \( \sqrt{3} \) | 1.732 |
| 5 | \( \sqrt{5} \) | 2.236 |
| 6 | \( \sqrt{6} \) | 2.449 |
| 8 | \( \sqrt{8} \) | 2.828 |
| 10 | \( \sqrt{10} \) | 3.162 |
| 15 | \( \sqrt{15} \) | 3.873 |
Through these comparisons, we can see how the square roots of non-perfect squares like 10 fit into the broader spectrum of square roots. Unlike perfect squares such as 4, 9, and 16, which have integer square roots, 10 and other non-perfect squares result in irrational numbers.
Visual Representation
Below is a visual representation to help illustrate the comparison between these square roots:
\( \sqrt{1} < \sqrt{2} < \sqrt{3} < \sqrt{4} < \sqrt{5} < \sqrt{6} < \sqrt{7} < \sqrt{8} < \sqrt{9} < \sqrt{10} < \sqrt{11} < \sqrt{12} < \sqrt{13} < \sqrt{14} < \sqrt{15} < \sqrt{16} \)
This linear representation highlights the gradual increase in value as we move from one square root to the next, with \( \sqrt{10} \) positioned between \( \sqrt{9} \) and \( \sqrt{11} \).
Dạng Đơn Giản Nhất Của Căn Bậc Hai
READ MORE:
Học cách đơn giản hóa căn bậc hai










