Topic square root 7: The square root of 7 is an intriguing mathematical concept with applications in various fields such as geometry, algebra, and engineering. This article explores its properties, calculation methods, and significance, offering a comprehensive understanding of this unique and fascinating number. Join us on a journey to uncover the mysteries and uses of the square root of 7.
Table of Content
- Square Root of 7
- Introduction to Square Root of 7
- Mathematical Definition
- Properties of Square Root of 7
- Methods to Calculate Square Root of 7
- Decimal Approximation of Square Root of 7
- Using Square Root of 7 in Equations
- Square Root of 7 in Geometry
- Applications of Square Root of 7
- Historical Context and Significance
- Square Root of 7 in Different Number Systems
- Common Misconceptions
- Advanced Mathematical Topics Involving Square Root of 7
- Square Root of 7 in Technology and Engineering
- Conclusion
- YOUTUBE: Giải thích về căn bậc hai của số 7 và các tính chất liên quan.
Square Root of 7
The square root of 7, denoted as \( \sqrt{7} \), is an irrational number. Its approximate value is \( \sqrt{7} \approx 2.6457513110645906 \).
Mathematical Properties
- \( \sqrt{7} \) is irrational, meaning it cannot be expressed as a simple fraction.
- It is a prime number because 7 is a prime number and its square root is not a rational number.
- It has a decimal expansion that goes on forever without repeating.
Applications
The square root of 7 is used in various fields such as mathematics, engineering, and physics for calculations involving areas, distances, and other measurements.
Related Topics
- Other irrational numbers and their properties.
- Approximation methods for irrational numbers.
- Applications of irrational numbers in real-life situations.

READ MORE:
Introduction to Square Root of 7
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root is often denoted using the radical symbol √. In the case of the number 7, the square root of 7 (√7) is an irrational number, which means it cannot be expressed exactly as a simple fraction and its decimal representation is non-terminating and non-repeating.
Mathematically, the square root of 7 can be represented as:
√7 ≈ 2.6457513110645906...
This approximation shows the first few decimal places of √7. The value continues infinitely without repeating, making it an irrational number.
Understanding the square root of 7 involves recognizing its properties and significance in various mathematical contexts:
- Properties: As an irrational number, √7 cannot be precisely written as a fraction. It is also greater than 2 (since 2² = 4) and less than 3 (since 3² = 9).
- Prime Factorization: Unlike perfect squares, 7 cannot be factored into smaller integers other than 1 and 7 itself, reinforcing its classification as an irrational number.
The concept of square roots extends beyond basic arithmetic, finding applications in algebra, geometry, and calculus. For instance, in algebra, solving quadratic equations often involves finding square roots, and in geometry, the Pythagorean theorem frequently necessitates calculating the square roots of sums of squares.
Understanding and working with the square root of 7 enhances comprehension of more complex mathematical theories and problems. It is fundamental in various scientific and engineering calculations where precise measurements and relationships are crucial.
Mathematical Definition
The square root of a number \( x \) is a value that, when multiplied by itself, gives \( x \). In mathematical terms, if \( r \) is the square root of \( x \), then:
\( r^2 = x \)
The square root symbol is called the radical sign and is written as \( \sqrt{} \). For example, the square root of 7 is denoted as \( \sqrt{7} \).
Since 7 is not a perfect square, \( \sqrt{7} \) is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating.
In general, the principal square root of a positive number is positive. However, every positive number has two square roots: one positive and one negative. Thus, the square roots of 7 are:
\( \pm \sqrt{7} \)
This can be written as:
\( \sqrt{7} \) and \( -\sqrt{7} \)
Properties of Square Root of 7
The square root of 7 has several interesting mathematical properties that are important to understand. Here are some key properties:
- Irrational Number: The square root of 7 is an irrational number, meaning it cannot be expressed as a simple fraction. Its decimal representation is non-repeating and non-terminating.
- Decimal Approximation: The square root of 7 is approximately 2.6457513110645906.
- Algebraic Property: The square root of 7 satisfies the equation \( (\sqrt{7})^2 = 7 \).
- Conjugate Property: The square root of 7 has a conjugate, which is \(-\sqrt{7}\). This means that both \( \sqrt{7} \) and \(-\sqrt{7} \) are solutions to the equation \( x^2 = 7 \).
- Product Property: For any positive numbers \( a \) and \( b \), \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \). For example, \( \sqrt{7 \times 2} = \sqrt{14} \).
- Quotient Property: For any positive numbers \( a \) and \( b \), \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \). For example, \( \sqrt{\frac{7}{2}} = \frac{\sqrt{7}}{\sqrt{2}} \).
These properties make the square root of 7 a fundamental element in various mathematical contexts, from algebra to geometry.
Methods to Calculate Square Root of 7
The square root of 7 can be calculated using various methods. Here, we will explore three common techniques: the Prime Factorization Method, the Long Division Method, and Newton's Method (also known as the Newton-Raphson Method).
1. Prime Factorization Method
This method is primarily used for perfect squares and involves expressing the number as a product of its prime factors. However, since 7 is a prime number, it cannot be simplified further using this method. Therefore, we move to other methods for non-perfect squares.
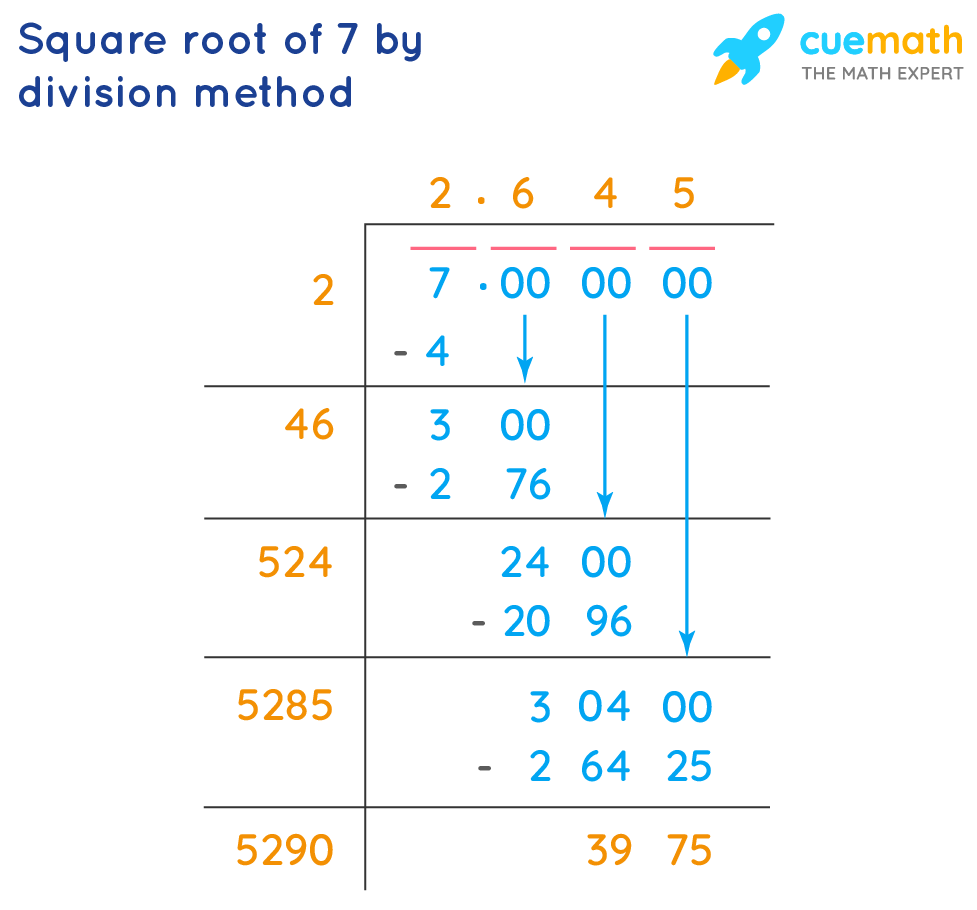
2. Long Division Method
The Long Division Method provides a systematic approach to finding the square root of any number, including non-perfect squares like 7. Follow these steps:
- Write 7 as 7.00000000 (adding pairs of zeros for precision).
- Find a number whose square is less than 7. Here, 22 = 4, and 4 is less than 7.
- Divide 7 by 2, resulting in 3.5. Place 2 as the quotient and 4 as the divisor.
- Subtract 4 from 7, resulting in 3. Bring down the next pair of zeros, making it 300.
- Double the current quotient (2), making it 4, and find a digit (x) such that 4x * x is less than or equal to 300. Here, x = 6 works since 46 * 6 = 276.
- Subtract 276 from 300, resulting in 24. Bring down the next pair of zeros, making it 2400.
- Repeat the process to achieve the desired precision. Continuing in this manner, you will get √7 ≈ 2.64575131.
3. Newton's Method (Newton-Raphson Method)
Newton's Method is an iterative technique that quickly converges to the square root of a number. Here are the steps:
- Start with an initial guess, x0. For √7, we can start with x0 = 2.5.
- Use the formula: xi+1 = (xi + 7/xi) / 2.
- Calculate the next approximation:
- x1 = (2.5 + 7/2.5) / 2 ≈ 2.65
- x2 = (2.65 + 7/2.65) / 2 ≈ 2.645
- x3 = (2.645 + 7/2.645) / 2 ≈ 2.64575131
- Continue iterating until the difference between successive approximations is negligible. The result will be √7 ≈ 2.64575131.
These methods provide different approaches to calculating the square root of 7, each useful in various contexts. The Long Division Method is straightforward for manual calculations, while Newton's Method is efficient for computer algorithms.
Decimal Approximation of Square Root of 7
The square root of 7, denoted as \( \sqrt{7} \), is an irrational number. This means it cannot be expressed as a simple fraction and its decimal representation is non-terminating and non-repeating. The approximate value of \( \sqrt{7} \) is often required in various mathematical and practical applications. Here, we will explore its decimal approximation in detail.
The value of \( \sqrt{7} \) up to 10 decimal places is approximately:
\( \sqrt{7} \approx 2.6457513111 \)
To understand this better, let's look at the process of calculating this approximation step by step:
-
Initial Guess:
We start with an initial guess for \( \sqrt{7} \). A reasonable guess might be a number between 2 and 3, since \( 2^2 = 4 \) and \( 3^2 = 9 \). Let's take 2.5 as the initial guess.
-
Refinement Using the Babylonian Method:
The Babylonian method (or Newton's method) is an iterative process that improves the approximation. The formula used is:
\[
x_{n+1} = \frac{x_n + \frac{7}{x_n}}{2}
\]
Starting with \( x_0 = 2.5 \):- \( x_1 = \frac{2.5 + \frac{7}{2.5}}{2} \approx 2.65 \)
- \( x_2 = \frac{2.65 + \frac{7}{2.65}}{2} \approx 2.645 \)
- \( x_3 = \frac{2.645 + \frac{7}{2.645}}{2} \approx 2.645751 \)
- Continuing this process yields values that converge to the actual square root of 7.
For practical purposes, we can use a calculator to find a more precise value:
| Decimal Places | Approximate Value |
|---|---|
| 1 | 2.6 |
| 2 | 2.65 |
| 3 | 2.646 |
| 4 | 2.6458 |
| 5 | 2.64575 |
| 6 | 2.645751 |
| 7 | 2.6457513 |
| 8 | 2.64575131 |
| 9 | 2.645751311 |
| 10 | 2.6457513111 |
This detailed step-by-step approximation helps in understanding the nature of the square root of 7 and its decimal expansion. For most practical applications, using \( 2.645751311 \) or even \( 2.64575 \) might be sufficiently accurate.
Using Square Root of 7 in Equations
The square root of 7, denoted as \( \sqrt{7} \), is often encountered in various mathematical equations. Here, we will explore different types of equations where \( \sqrt{7} \) is used and demonstrate how to solve them.
1. Solving Quadratic Equations
Quadratic equations can have solutions involving \( \sqrt{7} \). Consider the equation:
To solve this, take the square root of both sides:
Thus, the solutions are \( x = \sqrt{7} \) and \( x = -\sqrt{7} \).
2. Solving Equations with Radical Expressions
Equations involving square roots can be solved by isolating the radical and squaring both sides. For example:
Consider the equation:
First, isolate the square root:
Square both sides:
In this case, \( x = 9 \), but if the original equation had \( \sqrt{x-7} + 2 = 5 \), it would require additional steps:
Square both sides:
Solve for \( x \):
3. Applications in Geometry
In geometry, \( \sqrt{7} \) can be used to find the side length of a square given its area. For example, if the area \( A \) of a square is 7, the side length \( s \) is given by:
Thus, \( s = \sqrt{7} \).
4. Complex Equations Involving \( \sqrt{7} \)
Consider solving more complex equations like:
This simplifies to:
Since \( x \) involves the square root of a negative number, the solutions are complex:
5. Rationalizing Denominators
Sometimes \( \sqrt{7} \) appears in fractions. Consider rationalizing \( \frac{1}{\sqrt{7}} \):
Multiply by \( \frac{\sqrt{7}}{\sqrt{7}} \):
This simplifies to \( \frac{\sqrt{7}}{7} \).
Square Root of 7 in Geometry
The square root of 7, approximately 2.6457513110645906, appears in various geometric contexts and applications. Below are some examples of how the square root of 7 is used in geometry:
1. Diagonal of a Rectangle
In a rectangle where the sides are of length 1 and
2. Right Triangles
In a right triangle with legs of lengths 1 and
3. Geometric Constructions
The square root of 7 can be constructed using a compass and straightedge. One common method is:
- Draw a unit length segment (AB = 1).
- Construct a perpendicular bisector of AB at its midpoint M.
- Set a compass with radius AM and draw an arc that intersects the perpendicular bisector at point C such that AC = 1.
- Now, draw a segment from C perpendicular to AB until it intersects AB at point D.
- The length CD is
\sqrt{7} .
4. Area Calculations
Consider a right triangle with legs of lengths
5. Circle and Inscribed Shapes
In a circle with radius
These examples illustrate the significance of the square root of 7 in geometric problems and constructions, showcasing its diverse applications in various geometric contexts.
Applications of Square Root of 7
The square root of 7 finds various applications across different fields due to its mathematical properties. Below are some notable applications:
1. Geometry and Architecture
In geometry, the square root of 7 is used in calculations involving the Pythagorean theorem to find the length of sides in right triangles. For example, if one leg of a right triangle is 7 units, the hypotenuse can be calculated using the square root of 7. This principle is also applied in architecture to ensure accurate measurements and structural integrity.
- Pythagorean Theorem: For a right triangle with legs of lengths 1 and √7, the hypotenuse is √(1² + (√7)²) = √8.
2. Physics and Engineering
Square roots are fundamental in physics and engineering calculations, including determining natural frequencies of structures and solving equations related to wave propagation and vibrations. The square root of 7 can appear in these contexts when dealing with specific constants or measurements.
- Natural Frequency: The natural frequency (f) of a system can be calculated as f = 1/(2π)√(k/m), where k is the stiffness and m is the mass. If k or m involves the number 7, the square root of 7 will be part of the calculation.
3. Finance
In finance, the square root function is used in various models, such as calculating stock volatility. Volatility (σ) is the square root of the variance of stock returns, which helps investors assess the risk associated with a particular stock.
- Volatility Calculation: σ = √(variance). If the variance is 7, then σ = √7.
4. Computer Science and Cryptography
In computer science, square roots are used in algorithms for encryption and data security. Cryptographic systems often rely on mathematical properties of square roots for generating keys and secure communication protocols.
- Encryption Algorithms: Algorithms such as RSA use square roots in modular arithmetic to create public and private keys.
5. Navigation
Square roots are used in navigation to compute distances between points. For example, the distance between two points in a coordinate system can be calculated using the distance formula, which involves the square root of the sum of squared differences in coordinates.
- Distance Formula: For points (x1, y1) and (x2, y2), the distance is √((x2 - x1)² + (y2 - y1)²). If one of the differences is 7, the square root of 7 will be part of the calculation.
6. Statistics
In statistics, the square root of 7 can appear in formulas for standard deviation and variance, which are crucial for data analysis and interpretation. The standard deviation is the square root of the variance, providing insight into data spread.
- Standard Deviation: If the variance (σ²) is 7, then the standard deviation (σ) is √7.
These examples illustrate the broad applicability of the square root of 7 in various domains, showcasing its importance in both theoretical and practical contexts.

Historical Context and Significance
The concept of the square root has a rich historical background, deeply intertwined with the evolution of mathematics in various ancient civilizations. The square root of 7, specifically, plays a significant role in the broader understanding of irrational numbers and their properties.
In Ancient Babylon, mathematicians were already dealing with square roots around 1800 BC. They used clay tablets like the famous YBC 7289 to calculate the square roots of numbers, primarily focusing on the square root of 2, but laying the groundwork for understanding other square roots, including that of 7.
During the Greek Era, around 300 BC, the understanding of irrational numbers was significantly advanced by mathematicians like Pythagoras and Euclid. They demonstrated that the square roots of non-square integers, such as 7, could not be expressed as the ratio of two integers, thereby proving their irrationality.
In Ancient India, the Sulba Sutras, dated around 800-500 BC, provide methods for calculating square roots. Indian mathematicians like Aryabhata and Brahmagupta made significant contributions to this field, improving techniques to approximate square roots with high accuracy. Although their work primarily focused on numbers like 2 and 3, their methodologies were applicable to finding the square root of 7 as well.
By the Islamic Golden Age around 820 AD, scholars like Al-Khwarizmi were further developing algebraic methods that included solving quadratic equations, indirectly enhancing the understanding of square roots. These works were crucial in spreading advanced mathematical knowledge across Europe and Asia.
During the European Renaissance, mathematical advancements resumed with a renewed interest in algebra and geometry. Mathematicians began to more systematically approach the computation and properties of square roots, including that of 7, building on the foundations laid by earlier civilizations.
Overall, the historical journey of understanding the square root of 7 highlights the interconnectedness of mathematical progress across different cultures and eras. This journey not only reflects the development of mathematical theory but also its practical applications in fields such as engineering, architecture, and astronomy.
Square Root of 7 in Different Number Systems
The square root of 7, denoted as \(\sqrt{7}\), can be represented in various number systems. Here, we explore its representation and significance across different numeral systems.
Decimal System
In the decimal system (base 10), the square root of 7 is approximately 2.6457513110645906. This is a non-repeating, non-terminating decimal, indicating that \(\sqrt{7}\) is an irrational number.
Binary System
In the binary system (base 2), \(\sqrt{7}\) is represented as an infinite series:
\[ \sqrt{7}_{10} = 10.101011000111111101101010100010011011..._2 \]
This binary expansion continues infinitely without repeating.
Hexadecimal System
In the hexadecimal system (base 16), \(\sqrt{7}\) can be approximated as:
\[ \sqrt{7}_{10} \approx 2.A4D_6A_{16} \]
This shows the value in a compact form, useful in computing and digital systems.
Sexagesimal System
In the ancient Babylonian sexagesimal system (base 60), numbers were often represented with a mix of digits and fractions. While the exact representation of \(\sqrt{7}\) would be complex, it's noteworthy that such numeral systems laid the foundation for modern positional notations.
Other Systems
- Octal System (Base 8): In the octal system, \(\sqrt{7}\) is represented as: \[ \sqrt{7}_{10} \approx 2.512_634_170_265_3..._8 \]
- Quaternary System (Base 4): In the quaternary system, \(\sqrt{7}\) is represented as: \[ \sqrt{7}_{10} \approx 2.123_103_030_331..._4 \]
Imaginary Representation
In complex number systems, the square root of -7 can be expressed using the imaginary unit \(i\):
\[ \sqrt{-7} = \sqrt{7} \cdot i \approx 2.645 \cdot i \]
where \(i = \sqrt{-1}\). This representation is crucial in fields like electrical engineering and quantum physics.
Understanding the representation of \(\sqrt{7}\) in different numeral systems highlights its fundamental properties and the versatility of mathematical notation across various fields and applications.
Common Misconceptions
There are several common misconceptions about the square root of 7 and square roots in general. Understanding these misconceptions is crucial for a clearer grasp of mathematical concepts.
-
Misconception 1: The Square Root of a Number Always Has Two Values
Many believe that the square root of a number, such as 7, always has two values: positive and negative. This stems from the fact that when solving equations like \( x^2 = 7 \), the solutions are \( x = \pm \sqrt{7} \). However, the square root function, denoted \( \sqrt{x} \), is defined to be the non-negative value that, when squared, gives \( x \). Therefore, \( \sqrt{7} \) is only positive and equals approximately 2.64575, not \( \pm 2.64575 \).
-
Misconception 2: Square Root Can Be Solved Exactly for All Numbers
Another common misconception is that the square root of every number can be solved exactly. While this is true for perfect squares like 4 or 9, it is not true for numbers like 7. The square root of 7 is an irrational number, meaning it cannot be expressed as an exact fraction and has a non-repeating, non-terminating decimal expansion.
-
Misconception 3: Squaring and Square Rooting Are Always Inverses
While squaring and square rooting are inverse operations, this is only true within the context of non-negative numbers. For example, \( (\sqrt{7})^2 = 7 \). However, \( \sqrt{(-7)^2} \) does not equal -7, but rather 7, because the principal square root function always returns a non-negative result.
-
Misconception 4: All Roots Are Real Numbers
It is often assumed that all roots are real numbers. However, the square root of a negative number is not real but complex. For instance, \( \sqrt{-7} \) is not a real number but an imaginary number, expressed as \( \sqrt{-7} = \sqrt{7}i \), where \( i \) is the imaginary unit.
-
Misconception 5: Surds Are Always Simplifiable
Surds, or irrational roots like \( \sqrt{7} \), are often thought to be always simplifiable to a rational number. However, surds cannot be simplified exactly to a rational number; they remain in their radical form to preserve their exact value.
Addressing these misconceptions helps in developing a better understanding of the properties and applications of square roots, particularly for non-perfect squares like 7.
Advanced Mathematical Topics Involving Square Root of 7
The square root of 7, denoted as \( \sqrt{7} \), is an irrational number that appears in various advanced mathematical contexts. Here are some key areas where \( \sqrt{7} \) plays a significant role:
- Continued Fractions: The representation of \( \sqrt{7} \) as a continued fraction is periodic and can be expressed as: \[ \sqrt{7} = [2; \overline{1, 1, 1, 4}] \] This continued fraction is useful in number theory and for approximations of \( \sqrt{7} \).
- Diophantine Equations: The solutions to certain Diophantine equations involve \( \sqrt{7} \). For example, the equation \( x^2 - 7y^2 = 1 \) has integer solutions that can be found using properties of \( \sqrt{7} \).
- Algebraic Number Theory: \( \sqrt{7} \) is an example of a quadratic irrationality. It can be used to study the field \( \mathbb{Q}(\sqrt{7}) \), which consists of all numbers of the form \( a + b\sqrt{7} \) where \( a \) and \( b \) are rational numbers. This field has interesting properties and applications in algebraic number theory.
- Geometry: In geometric constructions, \( \sqrt{7} \) is significant. For example, it appears in the calculation of the diagonal of a rectangle with sides in the ratio 1:7, and in the construction of certain triangles where the side lengths are related to \( \sqrt{7} \).
- Complex Analysis: The value \( \sqrt{7} \) can be extended to the complex plane, and its properties are studied in the context of complex numbers. For instance, \( \sqrt{7}i \) where \( i \) is the imaginary unit, has applications in solving polynomial equations with complex coefficients.
- Eigenvalues and Eigenvectors: In linear algebra, matrices with entries involving \( \sqrt{7} \) can have eigenvalues and eigenvectors that are important in various applications, such as in solving systems of linear equations or in transformations.
- Hyperbolic Geometry: In hyperbolic geometry, lengths and distances can be related to expressions involving \( \sqrt{7} \). This appears in the study of hyperbolic triangles and other figures.
The square root of 7 is not just a simple numerical value; it has deep connections and applications across various branches of mathematics. Understanding these connections enhances the comprehension of both theoretical and applied mathematics.

Square Root of 7 in Technology and Engineering
The square root of 7 (\(\sqrt{7}\)) plays a significant role in various fields of technology and engineering. Below are some of the key applications and concepts where \(\sqrt{7}\) is utilized:
-
Electrical Engineering
In electrical engineering, the square root function is fundamental in calculating power, voltage, and current. For example, the root mean square (RMS) value of an AC voltage or current is derived using the square root to obtain the effective value that represents the same power as a DC circuit. The formula for RMS voltage (\(V_{rms}\)) is given by:
\[
V_{rms} = \sqrt{\frac{1}{T} \int_0^T [V(t)]^2 dt}
\]where \(T\) is the period of the waveform.
-
Mechanical Engineering
In mechanical engineering, square roots are used to determine natural frequencies of structures. For instance, the natural frequency (\(\omega_n\)) of a mass-spring-damper system is given by:
\[
\omega_n = \sqrt{\frac{k}{m}}
\]where \(k\) is the stiffness of the spring and \(m\) is the mass.
-
Computer Science
In computer algorithms, especially those related to graphics and cryptography, square roots are essential. For instance, the distance between two points in a 2D plane is calculated using the Euclidean distance formula:
\[
d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}
\] -
Signal Processing
In signal processing, the square root is used in various algorithms, including those for calculating the magnitude of a complex number, which is critical in the analysis and interpretation of signals:
\[
|z| = \sqrt{a^2 + b^2}
\]where \(z = a + bi\) is a complex number.
-
Structural Engineering
Square roots are used in structural engineering to assess the load-bearing capacity and stability of structures. For example, the critical buckling load \(P_{cr}\) for a column is determined by:
\[
P_{cr} = \frac{\pi^2 EI}{(KL)^2}
\]where \(E\) is the modulus of elasticity, \(I\) is the moment of inertia, \(K\) is the effective length factor, and \(L\) is the length of the column.
-
Navigation and GPS
In navigation, the square root is used to calculate distances between points on the Earth's surface. The haversine formula, which includes square roots, is used to determine the shortest path between two points on a sphere:
\[
a = \sin^2\left(\frac{\Delta \phi}{2}\right) + \cos(\phi_1) \cos(\phi_2) \sin^2\left(\frac{\Delta \lambda}{2}\right)
\]\[
c = 2 \cdot \atan2\left( \sqrt{a}, \sqrt{1-a} \right)
\]\[
d = R \cdot c
\]where \(\phi\) is latitude, \(\lambda\) is longitude, \(R\) is the Earth’s radius, and \(d\) is the distance.
Conclusion
The square root of 7 is a fascinating and significant number in mathematics, with a variety of applications in different fields. Throughout this comprehensive guide, we have explored its mathematical definition, properties, methods of calculation, and decimal approximations. We have also delved into its use in equations, geometry, and various real-world applications.
The square root of 7, approximately \( \sqrt{7} \approx 2.64575 \), is an irrational number that cannot be expressed as a simple fraction. Its non-repeating, non-terminating decimal expansion highlights the complexity and beauty of irrational numbers.
In geometry, the square root of 7 is instrumental in solving problems involving right triangles and circles, among other shapes. It also plays a crucial role in advanced mathematical topics such as algebraic equations and number theory, where it often appears in the solutions to quadratic equations and in the study of quadratic fields.
Moreover, the square root of 7 has practical applications in technology and engineering. For instance, it can be used in calculations for design and construction, where precise measurements are crucial. It also finds applications in physics and computer science, particularly in algorithms that require precise square root computations.
Historically, the significance of the square root of 7 can be traced back to ancient civilizations that recognized the importance of irrational numbers in mathematical theory and practical calculations. Over time, the study of such numbers has led to a deeper understanding of the mathematical landscape and the development of more sophisticated mathematical tools and techniques.
In conclusion, the square root of 7 is not just a mathematical curiosity but a vital component of various mathematical, scientific, and engineering disciplines. Its properties and applications demonstrate the interconnectedness of different areas of study and the ongoing relevance of mathematics in solving real-world problems.
Giải thích về căn bậc hai của số 7 và các tính chất liên quan.
Căn bậc hai của số 7 | Toán học cơ bản
READ MORE:
Tìm căn bậc hai của 7 chính xác đến 3 chữ số thập phân