Topic simplifying radicals with square roots: Understanding how to simplify radicals with square roots is essential for mastering algebra. This guide will help you break down complex expressions into simpler forms, making it easier to solve equations and understand mathematical concepts. Learn step-by-step methods and examples to enhance your mathematical skills and confidence.
Table of Content
- Simplifying Radicals with Square Roots
- Introduction to Simplifying Radicals
- Basic Concepts
- Prime Factorization Method
- Examples of Simplifying Radicals
- Simplifying Radicals with Variables
- Combining Radicals
- Simplifying Fractions with Radicals
- Advanced Techniques and Examples
- Common Mistakes to Avoid
- Practice Problems and Solutions
- Conclusion and Summary
- YOUTUBE: Video này sẽ hướng dẫn bạn cách đơn giản hóa căn thức với biến số, số mũ, phân số và căn bậc ba trong đại số.
Simplifying Radicals with Square Roots
Understanding how to simplify radicals with square roots is a fundamental concept in algebra that helps in reducing expressions to their simplest form. This guide provides detailed steps and examples for simplifying square roots.
Basic Steps to Simplify Square Roots
- Factor the number under the square root into prime factors.
- Group the prime factors into pairs.
- Take one number out of each pair outside the square root.
- Multiply the numbers outside the square root and simplify the remaining number inside the square root.
Examples
Example 1: Simplify √12
- Factor 12 into prime factors: 12 = 2 × 2 × 3
- Group the pairs: (2 × 2) × 3
- Take 2 out of the pair: 2√3
So, √12 simplifies to 2√3.
Example 2: Simplify √45
- Factor 45 into prime factors: 45 = 3 × 3 × 5
- Group the pairs: (3 × 3) × 5
- Take 3 out of the pair: 3√5
So, √45 simplifies to 3√5.
Fractions and Square Roots
When dealing with fractions under a square root, the process involves simplifying both the numerator and the denominator separately.
Example: Simplify √(30/10)
- Simplify the fraction inside the square root: 30/10 = 3
- So, √(30/10) = √3
More Complex Examples
Example: Simplify √(12x⁶y³)
- Factor each term: 12 = 2² × 3, x⁶ = (x³)², y³ = y² × y
- Apply the product rule for radicals: √(2² × 3 × (x³)² × y² × y)
- Simplify: 2x³y√(3y)
So, √(12x⁶y³) simplifies to 2x³y√(3y).
Additional Tips
- Always look for the largest perfect square factor.
- Simplify radicals by combining like terms whenever possible.
- Remember the rule: √a × √b = √(ab).
Practicing these steps with various examples will help solidify your understanding of simplifying square roots.

READ MORE:
Introduction to Simplifying Radicals
Simplifying radicals involves reducing the expression under the square root sign to its simplest form. This process often includes factoring the number into prime factors and then applying the property of square roots to simplify the expression. Understanding how to simplify radicals is a crucial skill in algebra, as it helps in solving equations and simplifying complex mathematical expressions.
Here are the key steps involved in simplifying radicals:
- Identify and list the prime factors of the number inside the square root.
- Group the prime factors into pairs.
- For each pair of factors, "take one out" of the square root sign.
- Multiply the factors outside the square root sign together.
- The remaining factors inside the square root sign are multiplied together and kept under the radical.
Let's look at a few examples to illustrate these steps:
| Example | Simplification Steps |
| √12 |
|
| √45 |
|
When simplifying more complex radicals or fractions under the square root, similar steps are followed. The goal is to reduce the expression to its simplest form, making it easier to work with in further calculations or in solving equations.
Basic Concepts
Before delving into the specifics of simplifying radicals with square roots, it's essential to understand some fundamental concepts:
- Radicals: Radicals, often represented by the √ symbol, are mathematical expressions that denote the square root of a number. For instance, √9 represents the square root of 9, which is 3.
- Index: The index of a radical indicates the root to be taken. For square roots, the index is 2, but for other roots like cube roots or fourth roots, the index would be 3 and 4, respectively.
- Radical Expression: A radical expression consists of a radical symbol (√) and a radicand, which is the number under the radical symbol. For example, in √25, 25 is the radicand.
- Perfect Squares: Numbers that are the squares of integers are called perfect squares. For instance, 1, 4, 9, 16, and 25 are perfect squares because they are the squares of 1, 2, 3, 4, and 5, respectively.
- Principal Square Root: For every positive real number, there exists a positive and a negative square root. However, the principal square root refers to the positive square root of a number. It is denoted by √x, where x is a non-negative real number.
Understanding these concepts lays the groundwork for effectively simplifying radicals with square roots.
Prime Factorization Method
The prime factorization method is a systematic approach used to simplify radicals with square roots. Here's how it works:
- Identify the Radicand: Begin by identifying the number under the square root symbol, known as the radicand.
- Prime Factorization: Find the prime factorization of the radicand. This involves breaking down the number into its prime factors, which are the smallest prime numbers that multiply together to give the original number.
- Pairing Factors: After obtaining the prime factors, group them into pairs of identical factors. For each pair, one factor comes out of the radical symbol, leaving the other inside.
- Product of Square Roots: Multiply the factors that remain inside the radical symbol. This yields a simplified expression.
The prime factorization method is particularly useful when dealing with complex radicals or radicals involving large numbers.
Examples of Simplifying Radicals
Let's explore some examples to illustrate the process of simplifying radicals with square roots:
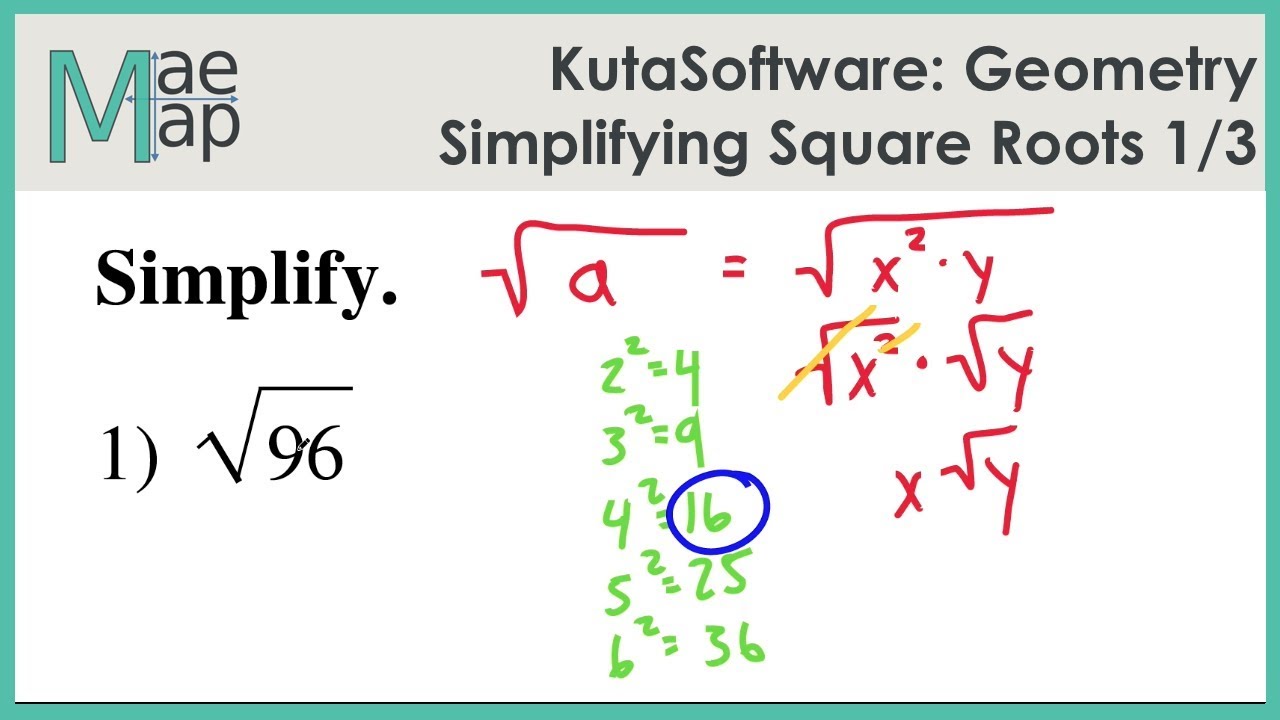
- Example 1: Simplify √32.
- Example 2: Simplify √75.
- Example 3: Simplify √18.
We start by finding the prime factorization of 32, which is 2 * 2 * 2 * 2 * 2. Grouping them into pairs, we have √(2 * 2 * 2 * 2 * 2) = 2√(2 * 2). Multiplying the factors inside the radical gives us 2 * 2 = 4. Therefore, √32 simplifies to 4√2.
The prime factorization of 75 is 3 * 5 * 5. Pairing the factors, we get √(3 * 5 * 5) = 5√3. Hence, √75 simplifies to 5√3.
The prime factorization of 18 is 2 * 3 * 3. Grouping them into pairs, we have √(2 * 3 * 3) = 3√2. Therefore, √18 simplifies to 3√2.
These examples demonstrate the application of the simplification process, providing clarity on how to handle various types of radicals with square roots.

Simplifying Radicals with Variables
When dealing with radicals containing variables, the simplification process follows similar principles as with numerical radicals. Here's how to simplify radicals with variables:
- Identify the Radicand: Start by identifying the expression under the square root symbol, which includes variables.
- Factorization: If possible, factorize the radicand into its prime factors. This might involve factoring out perfect square factors.
- Apply Radical Rules: Use the properties of radicals to simplify the expression. For instance, if a variable raised to an even exponent is inside the radical, half of the exponent can be brought out of the radical.
- Combine Like Terms: If there are multiple radicals with similar variables, combine them by adding or subtracting their coefficients.
Let's illustrate this process with an example:
Example: Simplify √(16x^2y^3).
First, we factorize the expression as √(4 * 4 * x * x * y * y * y). Pairing the perfect square factors, we get 4xy√y. Thus, √(16x^2y^3) simplifies to 4xy√y.
By following these steps, radicals containing variables can be simplified efficiently, aiding in various mathematical calculations and problem-solving.
Combining Radicals
Combining radicals involves simplifying expressions containing multiple radical terms. Here's a step-by-step guide:
- Identify Like Radicals: Look for radicals with the same root and radicand.
- Add or Subtract Coefficients: If the radicals are being added or subtracted, combine their coefficients by adding or subtracting them.
- Maintain Individual Radicals: Keep the individual radicals unchanged after combining coefficients.
Let's see an example to understand the process:
Example: Combine √3 + 2√3 - √12.
First, identify the radicals with the same radicand, which are √3 and 2√3. Adding their coefficients, we get 3√3. Then, we subtract √12, as it cannot be simplified further. Thus, the expression simplifies to 3√3 - √12.
By following these steps, expressions containing multiple radicals can be combined efficiently, leading to simplified and more manageable mathematical expressions.
Simplifying Fractions with Radicals
When dealing with fractions containing radicals, simplifying them involves rationalizing the denominator to remove the radical. Here's how to simplify fractions with radicals:
- Rationalize the Denominator: Multiply both the numerator and denominator of the fraction by a suitable expression to eliminate the radical from the denominator.
- Apply Radical Rules: Simplify the resulting expression by applying the properties of radicals and combining like terms.
Let's illustrate this process with an example:
Example: Simplify the fraction (3√2)/(√6).
To rationalize the denominator, we multiply both the numerator and denominator by √6 to get (3√2 * √6)/(√6 * √6) = (3√12)/6. Next, we simplify the expression inside the radical to get (3 * 2√3)/6 = √3/2. Therefore, the fraction simplifies to √3/2.
By rationalizing the denominator and simplifying the expression, fractions containing radicals can be expressed in a more concise and understandable form.
Advanced Techniques and Examples
Mastering simplification of radicals involves employing advanced techniques to handle complex expressions. Here are some advanced techniques along with examples:
- Conjugate Method: Utilize the conjugate of a binomial expression to rationalize denominators containing radicals. This technique involves multiplying the expression by its conjugate to eliminate radicals.
- Higher Index Radicals: Extend the simplification process to radicals with indices higher than 2, such as cube roots or fourth roots. Apply similar principles of factorization and simplification.
- Rational Exponents: Convert radicals to expressions with rational exponents for easier manipulation and calculation.
Let's delve into an example to showcase these techniques:
Example: Simplify (√5 - √3)/(√5 + √3).
To rationalize the denominator using the conjugate method, we multiply both the numerator and denominator by the conjugate of the denominator: (√5 - √3) * (√5 - √3) / ((√5 + √3) * (√5 - √3)). This yields ((√5)^2 - 2 * √5 * √3 + (√3)^2) / (5 - 3) = (5 - 2√15 + 3) / 2 = 8/2 = 4.
By employing advanced techniques like the conjugate method, handling higher index radicals, and utilizing rational exponents, even intricate radical expressions can be simplified effectively.

Common Mistakes to Avoid
While simplifying radicals with square roots, it's important to be mindful of common mistakes that can lead to errors. Here are some mistakes to avoid:
- Incorrect Factorization: Mistakes in identifying and factorizing the radicand can lead to incorrect simplification. Ensure accurate factorization to obtain the correct simplified form.
- Forgetting to Rationalize Denominators: Neglecting to rationalize denominators containing radicals can result in incomplete simplification. Always rationalize the denominator to ensure the expression is fully simplified.
- Misapplying Radical Rules: Applying radical rules incorrectly, such as distributing exponents or combining unlike radicals, can lead to inaccuracies in the simplification process. Understand and apply the rules correctly to avoid errors.
- Overlooking Like Terms: Failure to identify and combine like terms within the expression can result in unnecessary complexity. Always check for like terms and simplify accordingly.
- Skipping Steps: Rushing through the simplification process without performing each step diligently can lead to oversight and mistakes. Take time to systematically simplify the expression to ensure accuracy.
By being aware of these common mistakes and taking precautionary measures to avoid them, you can enhance the accuracy and efficiency of simplifying radicals with square roots.
Practice Problems and Solutions
Practice problems are an excellent way to reinforce your understanding of simplifying radicals with square roots. Here are some practice problems along with their solutions:
- Problem 1: Simplify √50.
- Problem 2: Simplify √(18x^2).
- Problem 3: Simplify (2√3 + √6)/(√2 - √3).
Solution: The prime factorization of 50 is 2 * 5 * 5. Grouping them into pairs, we have √(2 * 5 * 5) = 5√2. Therefore, √50 simplifies to 5√2.
Solution: The prime factorization of 18 is 2 * 3 * 3. Pairing them, we have √(2 * 3 * 3 * x * x) = 3x√2. Therefore, √(18x^2) simplifies to 3x√2.
Solution: To rationalize the denominator, we multiply both the numerator and denominator by the conjugate of the denominator: (√2 + √3) * (√2 + √3) / ((√2 - √3) * (√2 + √3)). This simplifies to (2√6 + 6)/(2 - 3) = -(2√6 + 6).
By practicing these problems and understanding the solutions, you can strengthen your skills in simplifying radicals with square roots.
Conclusion and Summary
Simplifying radicals with square roots is an essential skill in mathematics, often encountered in algebra and calculus. Through this article, we have explored various techniques and methods for simplifying radicals effectively. Here's a summary of key points:
- Basic Concepts: Understanding the fundamentals of radicals, including radicands, indices, and radical expressions, lays the foundation for simplification.
- Prime Factorization Method: Utilizing prime factorization to simplify radicals enables breaking down complex expressions into simpler forms.
- Examples of Simplifying Radicals: Illustrated examples demonstrate the application of simplification techniques in real scenarios.
- Simplifying Radicals with Variables: Extending simplification techniques to radicals containing variables involves factorization and applying radical rules.
- Combining Radicals: Combining like radicals involves identifying similar terms and combining coefficients while maintaining individual radicals.
- Simplifying Fractions with Radicals: Rationalizing denominators containing radicals enables complete simplification of fractions.
- Advanced Techniques and Examples: Employing advanced techniques like the conjugate method and rational exponents facilitates handling complex expressions.
- Common Mistakes to Avoid: Being mindful of common mistakes, such as incorrect factorization and misapplying radical rules, helps in achieving accurate simplification.
- Practice Problems and Solutions: Practicing problems with solutions reinforces understanding and enhances proficiency in simplifying radicals.
By mastering the techniques outlined in this article and practicing regularly, you can confidently simplify radicals with square roots, contributing to your mathematical skills and problem-solving abilities.
Video này sẽ hướng dẫn bạn cách đơn giản hóa căn thức với biến số, số mũ, phân số và căn bậc ba trong đại số.
Đơn Giản Hóa Căn Thức Với Biến Số, Số Mũ, Phân Số, Căn Bậc Ba - Đại Số
READ MORE:
Video này sẽ hướng dẫn bạn cách đơn giản hóa căn bậc hai, số mũ, căn thức, và ký hiệu khoa học trong tiền đại số từ Khan Academy.
Đơn Giản Hóa Căn Bậc Hai | Số Mũ, Căn Thức, và Ký Hiệu Khoa Học | Tiền Đại Số | Khan Academy