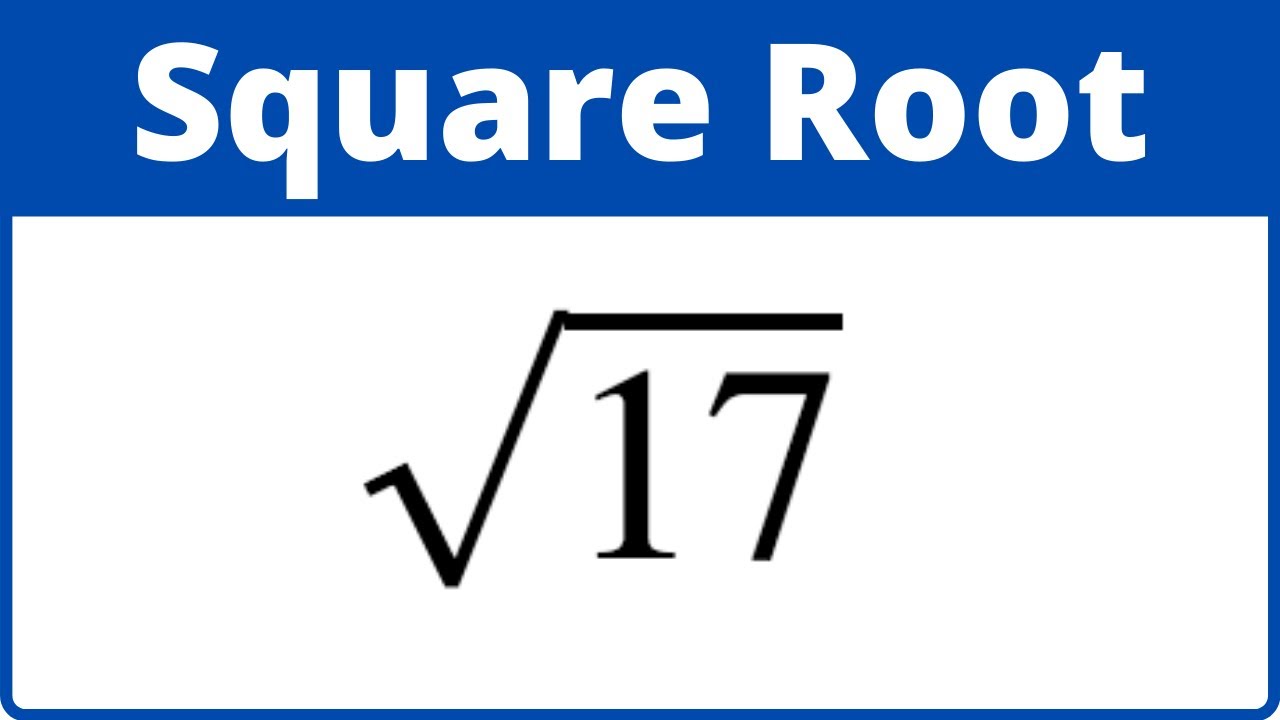
Topic simplify square root 64: Learn how to simplify the square root of 64 with this comprehensive guide. We'll walk you through the easy steps to understand and simplify square roots, making math more approachable and enjoyable. Whether you're a student or just curious, this article will help you master the concept effortlessly.
Table of Content
- Simplifying the Square Root of 64
- Introduction to Square Roots
- Understanding Perfect Squares
- Basic Concepts of Square Roots
- What is the Square Root of 64?
- Step-by-Step Process to Simplify Square Roots
- Mathematical Explanation of \(\sqrt{64}\)
- Examples of Perfect Squares
- Why 64 is a Perfect Square?
- Alternative Methods to Simplify Square Roots
- Common Mistakes to Avoid
- Applications of Square Roots in Real Life
- Frequently Asked Questions About Square Roots
- YOUTUBE:
Simplifying the Square Root of 64
Simplifying square roots is a fundamental concept in mathematics. In this example, we will simplify the square root of 64.
Step-by-Step Solution
- Identify the number under the square root: \(\sqrt{64}\).
- Determine if 64 is a perfect square. A perfect square is an integer that can be expressed as the square of another integer.
- Find the square root of 64. Since 64 is a perfect square, we can express it as \(8 \times 8\).
- Thus, \(\sqrt{64} = 8\).
Conclusion
Therefore, the simplified form of \(\sqrt{64}\) is 8.

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics, often encountered in algebra and geometry. The square root of a number \( x \) is a value that, when multiplied by itself, gives the number \( x \). This can be expressed as:
\( \sqrt{x} \times \sqrt{x} = x \)
For instance, the square root of 64 is 8 because \( 8 \times 8 = 64 \).
Square roots can be applied to both positive and negative numbers, although the square root of a negative number is a complex number, not a real number. In real numbers, every positive number has two square roots: one positive (principal square root) and one negative.
Mathematically, this is represented as:
\( \sqrt{64} = 8 \) and \( -\sqrt{64} = -8 \)
Properties of Square Roots
- Non-Negative: The principal square root of any non-negative number is non-negative.
- Perfect Squares: Numbers like 1, 4, 9, 16, and 64 are called perfect squares because their square roots are whole numbers.
- Product Rule: The square root of a product is equal to the product of the square roots of the factors. For example, \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \) for non-negative numbers \( a \) and \( b \).
- Quotient Rule: The square root of a quotient is the quotient of the square roots of the numerator and denominator. For instance, \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \) where \( b \neq 0 \).
Visualizing Square Roots
Square roots can be visualized geometrically. If you have a square with an area of \( x \), the side length of the square is \( \sqrt{x} \). For example, a square with an area of 64 square units has side lengths of 8 units because \( \sqrt{64} = 8 \).
This property is useful in various fields, such as in calculating the dimensions of a square plot of land or determining the side length of a square-shaped object from its area.
Practical Applications
Square roots are widely used in science, engineering, and everyday life. For instance:
- Physics: In calculating the root-mean-square speed of gas molecules.
- Finance: In assessing the standard deviation of returns in investment portfolios.
- Geometry: In finding the length of the hypotenuse in right-angled triangles using the Pythagorean theorem.
- Construction: In determining the diagonal length of square or rectangular components.
Understanding square roots enhances problem-solving skills and provides a deeper comprehension of various mathematical and real-world concepts.
Understanding Perfect Squares
A perfect square is a number that can be expressed as the product of an integer with itself. In mathematical terms, a number \( n \) is a perfect square if there exists an integer \( m \) such that:
\( n = m \times m \) or \( n = m^2 \)
For example, 64 is a perfect square because it can be written as \( 8 \times 8 \) or \( 8^2 \).
Characteristics of Perfect Squares
- Whole Numbers: Perfect squares are always non-negative whole numbers. Examples include 1, 4, 9, 16, 25, and 64.
- Even and Odd Properties: The square of an even number is even, and the square of an odd number is odd. For instance, 4 is even, and its square 16 is also even. Similarly, 5 is odd, and its square 25 is odd.
- Factor Pairs: The number of factors of a perfect square is always odd. For example, the factors of 36 (a perfect square) are 1, 2, 3, 4, 6, 9, 12, 18, and 36, totaling nine factors.
List of Perfect Squares
Below is a list of the first ten perfect squares:
| Number | Perfect Square |
|---|---|
| 1 | \( 1^2 = 1 \) |
| 2 | \( 2^2 = 4 \) |
| 3 | \( 3^2 = 9 \) |
| 4 | \( 4^2 = 16 \) |
| 5 | \( 5^2 = 25 \) |
| 6 | \( 6^2 = 36 \) |
| 7 | \( 7^2 = 49 \) |
| 8 | \( 8^2 = 64 \) |
| 9 | \( 9^2 = 81 \) |
| 10 | \( 10^2 = 100 \) |
Finding Perfect Squares
To determine if a number is a perfect square, find its prime factorization and check if all the prime factors have even exponents. For instance, the prime factorization of 64 is:
\( 64 = 2^6 \)
Since the exponent 6 is even, 64 is a perfect square.
Perfect Squares in Geometry
In geometry, the concept of perfect squares is essential. For example, if you have a square with an area of 64 square units, the side length of this square is 8 units because \( \sqrt{64} = 8 \).
This principle is widely used in various fields, including architecture, design, and engineering, to determine dimensions and proportions accurately.
Real-Life Applications
- Area Calculation: Perfect squares are used to find the areas of squares and rectangular plots where length and width are equal.
- Pythagorean Theorem: Perfect squares are crucial in finding the sides of right-angled triangles.
- Optimization Problems: They are often used in maximizing or minimizing the use of space and materials.
Understanding perfect squares helps in simplifying complex mathematical problems and finding solutions efficiently.
Basic Concepts of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. For instance, the square root of 64 is 8 because \(8 \times 8 = 64\). This concept is fundamental in various areas of mathematics and has practical applications in many fields.
Here are some basic properties and rules related to square roots:
- Non-negative numbers: The square root function is defined only for non-negative numbers. The square root of a negative number is not a real number.
- Perfect squares: Numbers like 1, 4, 9, 16, 25, 36, 49, 64, 81, and 100 are perfect squares because they can be expressed as the square of integers. For example, 64 is a perfect square because \(8 \times 8 = 64\).
- Product property: The square root of a product is equal to the product of the square roots of the factors. Mathematically, this is expressed as \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \). For example, \( \sqrt{64} = \sqrt{8 \times 8} = \sqrt{8} \times \sqrt{8} = 8 \).
- Quotient property: The square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator. This is written as \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \). For example, \( \sqrt{\frac{64}{16}} = \frac{\sqrt{64}}{\sqrt{16}} = \frac{8}{4} = 2 \).
To simplify a square root, follow these steps:
- Factorize the number: Break down the number inside the square root into its prime factors. For example, the prime factorization of 64 is \(2 \times 2 \times 2 \times 2 \times 2 \times 2\).
- Pair the factors: Group the prime factors into pairs. Each pair of identical factors can be taken out of the square root. For instance, \(64 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 = (2 \times 2) \times (2 \times 2) \times (2 \times 2)\).
- Simplify the expression: Take one factor from each pair out of the square root and multiply them together. In the case of 64, you get \(2 \times 2 \times 2 = 8\). Therefore, \( \sqrt{64} = 8 \).
Understanding these basic concepts and properties will help you simplify square roots effectively and recognize when a number is a perfect square.
What is the Square Root of 64?
The square root of a number is a value that, when multiplied by itself, gives the original number. In mathematical terms, if \(x^2 = y\), then \(x\) is the square root of \(y\). The square root is represented by the radical symbol \(\sqrt{}\).
Let's find the square root of 64:
64 is a perfect square because it can be expressed as the product of an integer with itself. Specifically, \(64 = 8 \times 8\). Therefore, the square root of 64 is 8.
We can also verify this using the prime factorization method:
- First, we factorize 64 into its prime factors: \(64 = 2 \times 2 \times 2 \times 2 \times 2 \times 2\).
- Next, we group the factors into pairs of the same number: \( (2 \times 2) \times (2 \times 2) \times (2 \times 2) \).
- Each pair of twos gives us a 2 outside the radical: \(\sqrt{(2 \times 2) \times (2 \times 2) \times (2 \times 2)} = 2 \times 2 \times 2 = 8\).
Thus, the simplified form of \(\sqrt{64}\) is 8.
In mathematical notation, we write this as:
\[\sqrt{64} = 8\]
Alternatively, using the property of exponents, we know that the square root of a number can be expressed as that number raised to the power of \(\frac{1}{2}\). Therefore:
\[64^{\frac{1}{2}} = 8\]
To summarize, the square root of 64 is 8, which means \( \sqrt{64} = 8 \). This result can be obtained through direct calculation, prime factorization, or by recognizing that 64 is a perfect square.

Step-by-Step Process to Simplify Square Roots
Simplifying square roots involves breaking down the number under the radical sign into its prime factors and then simplifying. Here is a detailed step-by-step process to simplify square roots:
-
Identify the Number: Start with the number under the square root. For example, let's simplify the square root of 64, written as \(\sqrt{64}\).
-
Prime Factorization: Find the prime factors of the number. Prime factorization of 64 is:
\(64 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 = 2^6\)
-
Group the Factors: Pair the prime factors. Since 64 is \(2^6\), we can group the factors in pairs of two:
\((2 \times 2) \times (2 \times 2) \times (2 \times 2) = 2^2 \times 2^2 \times 2^2\)
-
Simplify the Square Root: Take one number from each pair outside the square root:
\(\sqrt{64} = \sqrt{(2^2) \times (2^2) \times (2^2)} = 2 \times 2 \times 2 = 8\)
-
Result: The simplified form of \(\sqrt{64}\) is 8.
Here are more examples to illustrate the process:
Simplify \(\sqrt{27}\): Prime factorization of 27 is \(3 \times 3 \times 3 = 3^3\). Group the factors: \(3^2 \times 3\). Simplify: \(\sqrt{27} = \sqrt{(3^2) \times 3} = 3\sqrt{3}\).
Simplify \(\sqrt{45}\): Prime factorization of 45 is \(3 \times 3 \times 5 = 3^2 \times 5\). Group the factors: \(3^2 \times 5\). Simplify: \(\sqrt{45} = \sqrt{(3^2) \times 5} = 3\sqrt{5}\).
By following these steps, you can simplify any square root. Remember to look for pairs of factors and take one number from each pair outside the square root.
Mathematical Explanation of \(\sqrt{64}\)
The square root of a number is a value that, when multiplied by itself, gives the original number. For the number 64, we seek a value that satisfies this condition:
\[
x^2 = 64
\]
To solve for \(x\), we take the square root of both sides:
\[
\sqrt{x^2} = \sqrt{64}
\]
This simplifies to:
\[
x = \pm 8
\]
Thus, the square roots of 64 are 8 and -8. Here, we typically consider the principal (positive) square root unless otherwise specified.
Let's break down the steps to find the square root of 64 using prime factorization:
- Prime factorize 64:
\[
64 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 = 2^6
\] - Apply the property of square roots:
\[
\sqrt{a^2} = a
\] - Since 64 is \(2^6\), pair the factors:
\[
\sqrt{64} = \sqrt{2^6} = \sqrt{(2^3)^2} = 2^3 = 8
\]
Therefore, the square root of 64 is:
\[
\sqrt{64} = 8
\]
This approach confirms that the principal square root of 64 is 8.
Examples of Perfect Squares
Perfect squares are numbers that are the square of an integer. These numbers have integer values as their square roots. Here are some common examples of perfect squares:
- \(1 = 1^2\)
- \(4 = 2^2\)
- \(9 = 3^2\)
- \(16 = 4^2\)
- \(25 = 5^2\)
- \(36 = 6^2\)
- \(49 = 7^2\)
- \(64 = 8^2\)
- \(81 = 9^2\)
- \(100 = 10^2\)
- \(121 = 11^2\)
- \(144 = 12^2\)
- \(169 = 13^2\)
- \(196 = 14^2\)
- \(225 = 15^2\)
These perfect squares are foundational in understanding the concept of square roots. For example, the square root of 64 is 8, because \(8^2 = 64\). Similarly, the square root of 49 is 7, as \(7^2 = 49\).
Perfect squares are particularly useful in various mathematical computations and problem-solving scenarios. Recognizing these numbers can simplify many algebraic processes and calculations.
Why 64 is a Perfect Square?
A perfect square is a number that can be expressed as the product of an integer with itself. In other words, if a number \( n \) is a perfect square, there exists an integer \( m \) such that \( n = m^2 \).
To understand why 64 is a perfect square, let's break it down:
- First, we find the prime factorization of 64. The factorization of 64 is:
- \( 64 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \)
- We can group the factors into pairs of the same number:
- \( 64 = (2 \times 2) \times (2 \times 2) \times (2 \times 2) \)
- Each pair of 2's can be expressed as \( 2^2 \):
- \( 64 = 2^2 \times 2^2 \times 2^2 = (2^3)^2 = 8^2 \)
Since we have expressed 64 as \( 8^2 \), it is clear that 64 is a perfect square, with 8 being its square root.
To further illustrate, let's consider the property of perfect squares in general:
- Perfect squares have an integer as their square root. For 64, the square root is:
- \( \sqrt{64} = 8 \)
- Perfect squares can be visualized as squares with sides of integer length. A square with a side length of 8 units has an area of 64 square units:
- \( \text{Area} = \text{side} \times \text{side} = 8 \times 8 = 64 \)
Thus, 64 meets all the criteria of a perfect square, as it can be expressed both as \( 8^2 \) and as a product of equal integers (8 times 8).

Alternative Methods to Simplify Square Roots
There are several methods to simplify square roots. Here, we will discuss three common methods: prime factorization, using the property of square roots, and estimation. Each method provides a systematic approach to finding the simplest form of a square root.
1. Prime Factorization
Prime factorization involves breaking down the number inside the square root into its prime factors. Here’s a step-by-step process:
- Find the prime factors of the number.
- Group the prime factors into pairs.
- For each pair, take one number out of the square root.
- Multiply the numbers taken out of the square root to get the simplified form.
Example: Simplify \(\sqrt{64}\).
Prime factors of 64: \(2 \times 2 \times 2 \times 2 \times 2 \times 2\).
Grouping into pairs: \((2 \times 2) \times (2 \times 2) \times (2 \times 2)\).
Taking one number from each pair: \(2 \times 2 \times 2 = 8\).
Therefore, \(\sqrt{64} = 8\).
2. Using the Property of Square Roots
This method utilizes the property that the square root of a product is the product of the square roots. Here's how it works:
- Express the number as a product of perfect squares and other numbers.
- Separate the perfect squares and take their square roots.
- Multiply the results.
Example: Simplify \(\sqrt{64}\).
64 can be written as \(8 \times 8\).
Taking the square root of each: \(\sqrt{8 \times 8} = 8\).
Therefore, \(\sqrt{64} = 8\).
3. Estimation Method
The estimation method is useful when dealing with numbers that are not perfect squares. Here’s the process:
- Find two perfect squares between which the number lies.
- Estimate the square root by finding the average of the square roots of these two perfect squares.
- Refine the estimation if needed.
Example: Estimate \(\sqrt{50}\).
50 lies between 49 (\(7^2\)) and 64 (\(8^2\)).
Average: \((7 + 8) / 2 = 7.5\).
Refine: Since 50 is closer to 49, \(\sqrt{50}\) is slightly greater than 7.5 but less than 8.
Therefore, \(\sqrt{50} \approx 7.1\).
These methods provide different approaches to simplifying square roots, each useful depending on the context and the number involved.
Common Mistakes to Avoid
When simplifying square roots, it's essential to avoid common mistakes that can lead to incorrect results. Here are some of the most frequent errors and tips on how to steer clear of them:
-
Forgetting to Check for Perfect Squares:
Always check if the number under the square root is a perfect square. For example, 64 is a perfect square because \( \sqrt{64} = 8 \). Skipping this step can lead to unnecessary complications in the simplification process.
-
Rushing Through the Process:
Simplification should be done methodically. Take your time to ensure each step is performed correctly. This helps avoid mistakes such as incorrect factorization or missing out on further simplifications.
-
Not Breaking Down the Number Correctly:
Ensure that the number is broken down into its prime factors correctly. For example, the prime factorization of 64 is \(2^6\), which helps in simplifying the square root to \(8\).
-
Ignoring the Properties of Radicals:
Remember the properties of square roots, such as \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \). Misapplying these properties can result in incorrect simplifications.
-
Not Simplifying Completely:
Ensure you simplify the expression completely. Sometimes, intermediate steps reveal further simplifications that are easy to miss if you're not thorough.
-
Misidentifying Non-Perfect Squares:
Distinguish between perfect and non-perfect squares correctly. For instance, knowing that \( \sqrt{20} \) simplifies to \( 2\sqrt{5} \), not \( 4.47 \), helps maintain accuracy in your calculations.
Avoiding these mistakes can significantly enhance your ability to simplify square roots accurately and efficiently.
Applications of Square Roots in Real Life
Square roots have a variety of applications in real-life scenarios. Here are a few examples:
-
Construction and Architecture:
In construction, square roots are used to determine the length of the sides of a square area when the area is known. For example, if you have a square patio with an area of 200 square feet, you can find the length of each side by calculating \(\sqrt{200} \approx 14.1\) feet.
-
Physics - Gravity:
Square roots are used in physics to calculate the time it takes for an object to fall to the ground from a certain height. The formula \(\frac{\sqrt{h}}{4}\) is used, where \(h\) is the height in feet. For instance, if an object is dropped from a height of 64 feet, it will take \(\frac{\sqrt{64}}{4} = 2\) seconds to reach the ground.
-
Accident Investigations:
Police officers use square roots to determine the speed of a car before it started to skid. The formula \(\sqrt{24d}\) is used, where \(d\) is the length of the skid marks in feet. For example, if the skid marks measure 190 feet, the speed of the car can be approximated as \(\sqrt{24 \times 190} \approx 67.5\) mph.
-
Finance - Compound Interest:
In finance, square roots are used in the formula for compound interest to determine the growth rate of an investment over time.
-
Medicine - Dosage Calculations:
In medicine, square roots are used to calculate correct dosages based on body surface area. This ensures that patients receive the proper amount of medication.
These examples illustrate how square roots are not just abstract mathematical concepts but practical tools used in various fields to solve real-world problems.
Frequently Asked Questions About Square Roots
Understanding square roots can be challenging, so here are answers to some common questions:
- What is a square root?
A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 64 is 8, because 8 x 8 = 64.
- How do you simplify square roots?
To simplify a square root, factor the number into its prime factors and pair them. For example, to simplify √72:
- Factor 72 into 2 × 2 × 2 × 3 × 3.
- Group the factors into pairs: (2 × 2) and (3 × 3).
- Take one factor from each pair: 2 and 3.
- Multiply these factors: 2 × 3 = 6.
- The simplified form is 6√2.
- Why is the square root of 64 a whole number?
64 is a perfect square because it can be expressed as 8 × 8. Thus, its square root is a whole number, 8.
- What are perfect squares?
Perfect squares are numbers that are the product of an integer multiplied by itself. Examples include 1 (1×1), 4 (2×2), 9 (3×3), and 64 (8×8).
- What are common mistakes to avoid when simplifying square roots?
Common mistakes include not fully factoring the number and missing pairs of factors. Always check for all possible pairs to simplify correctly.
- Are there different methods to simplify square roots?
Yes, there are several methods including the prime factorization method, the repeated subtraction method, and the long division method. Each method involves different steps but aims to simplify the square root to its simplest form.
- How do square roots apply in real life?
Square roots are used in various fields such as engineering, physics, and finance. For example, they are used to calculate areas, solve quadratic equations, and determine standard deviations in statistics.
Căn Bậc Hai Của 64
READ MORE:
Video hướng dẫn cách tính căn bậc hai của 64 (Sqrt(64)). Dễ hiểu và thu hút người xem.
Cách Tính Căn Bậc Hai Của 64: Sqrt(64)