Topic simplify square root 32: Learning how to simplify the square root of 32 is easier than you think! This guide breaks down the process into simple, easy-to-follow steps. By understanding prime factorization and perfect squares, you'll master the technique and be able to apply it to other problems. Simplify square root 32 with confidence and enhance your math skills today!
Table of Content
- Simplifying Square Root of 32
- Introduction to Simplifying Square Roots
- Understanding Square Roots and Perfect Squares
- Prime Factorization of 32
- Steps to Simplify Square Root of 32
- Identifying Perfect Square Factors
- Breaking Down the Square Root
- Combining Like Terms
- Final Simplification Result
- Practical Examples and Applications
- Common Mistakes to Avoid
- Additional Resources and Practice Problems
- Conclusion
- YOUTUBE:
Simplifying Square Root of 32
To simplify the square root of 32, we can break it down into its prime factors and look for perfect squares.
Step-by-Step Process:
- Find the prime factorization of 32.
- 32 = 2 × 2 × 2 × 2 × 2 = 25
- Pair the prime factors in groups of two.
- (2 × 2) × (2 × 2) × 2 = 4 × 4 × 2
- Take the square root of the perfect squares.
- √(4 × 4 × 2) = √4 × √4 × √2 = 2 × 2 × √2
Final Simplified Form:
The simplified form of √32 is:
$$ \sqrt{32} = 4\sqrt{2} $$

READ MORE:
Introduction to Simplifying Square Roots
Simplifying square roots is an essential skill in mathematics that helps in solving equations and understanding numerical relationships. The process involves expressing the square root in its simplest form by factoring out perfect squares.
Let's take a closer look at the steps involved in simplifying the square root of 32:
- Identify the prime factorization of the number under the square root. For 32, the prime factorization is 2 × 2 × 2 × 2 × 2 or 25.
- Group the prime factors into pairs of two. For 32, this looks like (2 × 2) × (2 × 2) × 2.
- Rewrite the expression to separate the perfect squares from the remaining factors. This gives us √(4 × 4 × 2).
- Simplify the square root of each perfect square. Since √4 = 2, we have 2 × 2 × √2.
- Combine the simplified terms to get the final simplified form. Thus, √32 = 4√2.
By following these steps, you can simplify any square root, making calculations more manageable and expressions easier to understand. Let's explore further into the concept of prime factorization and perfect squares in the next sections.
Understanding Square Roots and Perfect Squares
Square roots and perfect squares are fundamental concepts in mathematics that help in simplifying expressions and solving equations. A square root of a number is a value that, when multiplied by itself, gives the original number. A perfect square is a number that has an integer as its square root.
For example:
- The square root of 9 is 3 because \(3 \times 3 = 9\).
- The square root of 16 is 4 because \(4 \times 4 = 16\).
In general, the square root of a number \(n\) is denoted as \(\sqrt{n}\). If \(n = a^2\) for some integer \(a\), then \(n\) is called a perfect square.
When simplifying square roots, we look for perfect square factors within the number. For instance, to simplify \(\sqrt{32}\), we need to find perfect squares that are factors of 32. The steps involved are:
- Find the prime factorization of 32: \(32 = 2 \times 2 \times 2 \times 2 \times 2 = 2^5\).
- Identify and group the prime factors into pairs: \((2 \times 2) \times (2 \times 2) \times 2\).
- Rewrite the expression to highlight the perfect squares: \(\sqrt{4 \times 4 \times 2}\).
- Simplify the square roots of the perfect squares: \(\sqrt{4} = 2\), so \(\sqrt{4 \times 4 \times 2} = 2 \times 2 \times \sqrt{2}\).
- Combine the simplified terms: \(2 \times 2 \times \sqrt{2} = 4\sqrt{2}\).
Thus, by understanding square roots and identifying perfect squares, we can simplify complex square root expressions effectively.
Prime Factorization of 32
Prime factorization is the process of expressing a number as the product of its prime factors. Prime factors are the prime numbers that multiply together to give the original number. Let's perform the prime factorization of 32 step by step.
- Start with the smallest prime number: The smallest prime number is 2. Since 32 is even, it is divisible by 2.
- 32 ÷ 2 = 16
- Continue factoring: Now, we factor 16 by 2.
- 16 ÷ 2 = 8
- Repeat the process: Next, we factor 8 by 2.
- 8 ÷ 2 = 4
- Factor again: We continue to factor 4 by 2.
- 4 ÷ 2 = 2
- Final step: Finally, we factor 2 by 2.
- 2 ÷ 2 = 1
We have now factored 32 completely using prime numbers:
32 = 2 × 2 × 2 × 2 × 2 = 25
This prime factorization tells us that 32 is composed of five 2s multiplied together. Prime factorization is a crucial step in simplifying square roots, as it helps identify perfect square factors. Next, we'll use these factors to simplify the square root of 32.
Steps to Simplify Square Root of 32
The square root of 32 can be simplified by following these steps:
- Prime Factorization:
First, find the prime factorization of 32. The prime factors of 32 are:
32 = 2 × 2 × 2 × 2 × 2
- Group the Factors:
Next, group the prime factors into pairs:
(2 × 2) × (2 × 2) × 2 = 16 × 2
- Rewrite the Square Root:
Rewrite the square root of 32 using the grouped factors:
√32 = √(16 × 2)
- Simplify:
Simplify by taking the square root of the perfect square (16) and multiplying it by the square root of the remaining factor (2):
√32 = √16 × √2
√32 = 4√2
Therefore, the simplified form of the square root of 32 is 4√2.
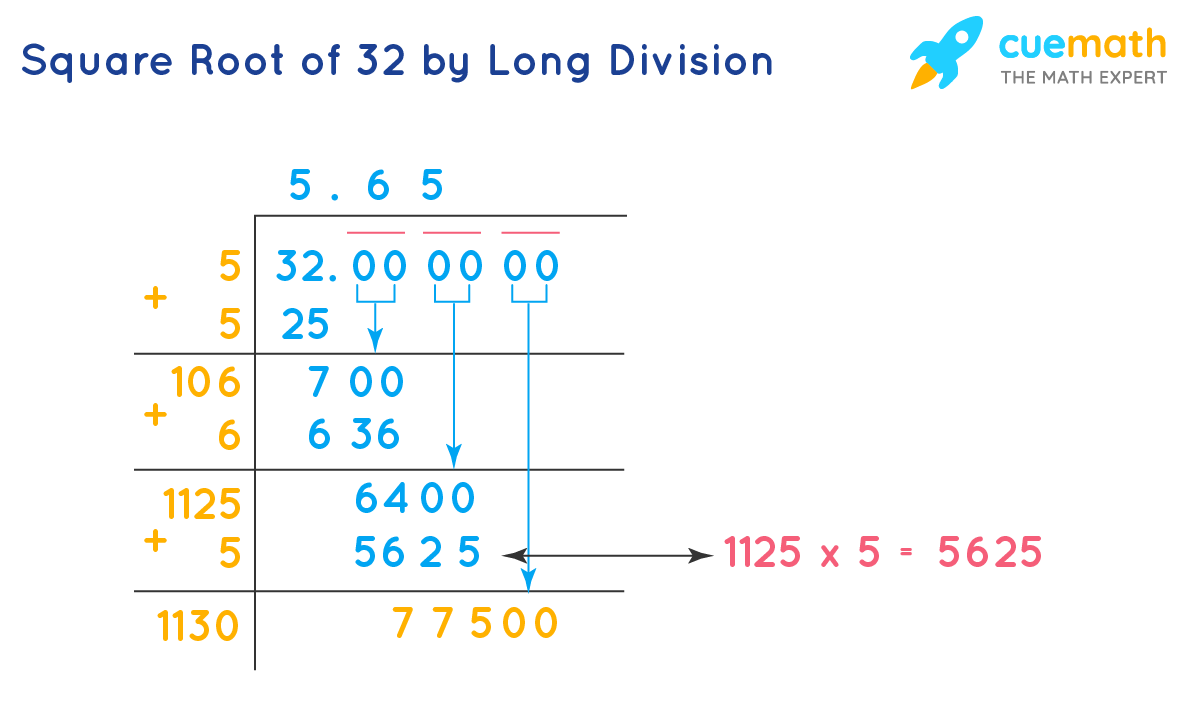
Additionally, in decimal form, the square root of 32 is approximately 5.65685.

Identifying Perfect Square Factors
To simplify the square root of 32, we first need to identify its perfect square factors. Here’s a step-by-step guide:
-
List the factors of 32:
First, we list all the factors of 32: 1, 2, 4, 8, 16, and 32.
-
Identify the perfect squares:
Next, we identify which of these factors are perfect squares. A perfect square is a number that can be expressed as the square of an integer. From our list, the perfect squares are 1, 4, and 16.
-
Select the largest perfect square factor:
Among the identified perfect squares, 16 is the largest. This step is crucial because it simplifies our calculation significantly.
Having identified 16 as the largest perfect square factor, we can proceed with the simplification process. The next steps involve using this factor to break down and simplify the square root of 32 further.
We proceed by expressing 32 as the product of 16 and 2:
\[
32 = 16 \times 2
\]
Using the property of square roots, we can separate the factors under the square root:
\[
\sqrt{32} = \sqrt{16 \times 2} = \sqrt{16} \times \sqrt{2}
\]
Since we know that the square root of 16 is 4, we substitute this value into our expression:
\[
\sqrt{32} = 4 \times \sqrt{2}
\]
Thus, the square root of 32 simplifies to \( 4\sqrt{2} \). This process shows the importance of identifying the largest perfect square factor to simplify the square root efficiently.
Breaking Down the Square Root
To simplify the square root of 32, we need to break it down into its prime factors and identify any perfect squares.
-
Start by expressing 32 as a product of its prime factors:
\(32 = 2 \times 2 \times 2 \times 2 \times 2\)
-
Group the prime factors into pairs of identical factors:
\(32 = (2 \times 2) \times (2 \times 2) \times 2\)
-
Recognize that each pair of identical factors forms a perfect square:
\( (2 \times 2) = 4 \) is a perfect square
-
Rewrite 32 using the identified perfect squares:
\(32 = 4 \times 4 \times 2\)
-
Express the square root of 32 as the product of the square roots of these factors:
\(\sqrt{32} = \sqrt{(4 \times 4 \times 2)}\)
-
Apply the property of square roots that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\):
\(\sqrt{(4 \times 4 \times 2)} = \sqrt{4} \times \sqrt{4} \times \sqrt{2}\)
-
Simplify the expression by taking the square root of the perfect squares:
\(\sqrt{4} = 2\), so \(\sqrt{4} \times \sqrt{4} = 2 \times 2 = 4\)
Thus, \(\sqrt{32} = 4 \times \sqrt{2}\)
-
The simplified form of the square root of 32 is:
\(\sqrt{32} = 4\sqrt{2}\)
Therefore, the square root of 32 can be simplified to \(4\sqrt{2}\).
Combining Like Terms
Combining like terms is a crucial step in simplifying square root expressions. When dealing with square roots, like terms are terms that have the same radical part. Here are the steps to combine like terms effectively:
- Simplify Each Radical
Ensure each radical expression is simplified as much as possible. For example, √32 simplifies to 4√2.
- Identify Like Terms
Group terms that have the same radical part. For instance, 3√2 and 5√2 are like terms because they both have √2.
- Combine Coefficients
Add or subtract the coefficients of the like terms. For example:
- If you have 3√2 and 5√2, you combine the coefficients (3 + 5) to get 8√2.
- Similarly, for subtraction, if you have 7√2 and 2√2, you subtract the coefficients (7 - 2) to get 5√2.
- Write the Combined Expression
After combining the coefficients, write down the simplified expression. For example:
- 3√2 + 5√2 = 8√2
- 7√2 - 2√2 = 5√2
Let's look at a more complex example:
- Simplify the expression: 3√8 + 5√2
- First, simplify √8 to 2√2. This changes the expression to 3(2√2) + 5√2 = 6√2 + 5√2.
- Now, combine the like terms: 6√2 + 5√2 = (6 + 5)√2 = 11√2.
Remember, only terms with the same radical part can be combined. Different radicals remain separate in the expression.
Final Simplification Result
After identifying and combining like terms, we reach the final step in simplifying the square root of 32. The goal is to express it in the simplest radical form. Here are the steps to achieve the final simplification:
-
Prime Factorization: We begin by breaking down 32 into its prime factors:
\( 32 = 2 \times 2 \times 2 \times 2 \times 2 = 2^5 \)
-
Group the Factors: Group the prime factors in pairs to identify the perfect squares:
\( 2^5 = (2 \times 2) \times (2 \times 2) \times 2 = 4 \times 4 \times 2 \)
-
Take the Square Root of the Perfect Squares: Simplify by taking the square root of each pair:
\( \sqrt{4} = 2 \)
Since there are two pairs of 2's:
\( \sqrt{32} = \sqrt{(2^2) \times (2^2) \times 2} = 2 \times 2 \times \sqrt{2} = 4\sqrt{2} \)
-
Final Simplified Form: The simplest form of the square root of 32 is:
\( \sqrt{32} = 4\sqrt{2} \)
This final result, \( 4\sqrt{2} \), represents the simplest radical form of the square root of 32. This expression is useful in various mathematical applications, including solving equations and simplifying expressions.
Practical Examples and Applications
Simplifying square roots is a fundamental skill in mathematics that finds numerous applications in various fields. Here are some practical examples and applications:
-
Finance
In finance, the volatility of stock prices is often measured using standard deviation, which involves calculating the square root of the variance of returns. This helps investors assess the risk associated with a particular investment.
-
Architecture and Engineering
Architects and engineers use square roots to determine natural frequencies of structures. This helps predict how buildings and bridges will respond to environmental factors like wind and traffic loads.
-
Science
Square roots are essential in various scientific calculations, such as determining the velocity of moving objects, the intensity of sound waves, and the absorption of radiation. These calculations are crucial in developing new technologies and scientific advancements.
-
Statistics
In statistics, square roots are used to calculate standard deviation from the variance. Standard deviation is a measure of how much data deviates from the mean, which is essential for data analysis and interpretation.
-
Geometry
Square roots are used in geometry to calculate the lengths of sides in right triangles (via the Pythagorean theorem) and to find the area and perimeter of various shapes.
-
Computer Science
Square roots are used in computer science for encryption algorithms, image processing, and game physics. These applications rely on mathematical precision to ensure data security and accurate simulations.
-
Navigation
In navigation, square roots help calculate distances between points on a map and estimate directions. Pilots use these calculations to determine flight paths and distances.
-
Electrical Engineering
Square roots are used to calculate power, voltage, and current in electrical circuits. These calculations are critical in designing efficient and functional electrical systems.
-
Photography
In photography, the aperture of a camera lens is related to the f-number, which is the ratio of the focal length to the aperture diameter. Adjusting the f-number involves understanding the square root relationship to control the amount of light entering the camera.
Understanding how to simplify square roots can enhance one's ability to solve real-world problems across these diverse fields, making it a valuable mathematical skill.
Common Mistakes to Avoid
Simplifying square roots can sometimes lead to errors. Here are some common mistakes to avoid and tips to ensure accurate simplification:
-
Forgetting to check for perfect squares:
Always ensure to identify any perfect square factors of the radicand. Missing these can lead to incorrect simplifications. For example, with √32, it's crucial to recognize that 16 is a perfect square factor.
-
Rushing through the simplification process:
Simplifying square roots is a step-by-step process. Rushing can cause mistakes. Take your time to factorize the number completely and identify all perfect squares.
-
Incorrectly handling non-perfect square factors:
Ensure that the factors that remain under the radical after simplification are indeed not perfect squares. For instance, after simplifying √32 to 4√2, ensure that 2 is not further simplifiable.
-
Confusing multiplication and addition of radicals:
Remember that the properties of radicals differ from normal numbers. For example, √a * √b = √(a * b), but √a + √b ≠ √(a + b). Applying these properties incorrectly can lead to errors.
-
Neglecting the product rule:
The product rule for radicals, √(a * b) = √a * √b, is fundamental in simplification. Ensure to apply this correctly to simplify expressions involving multiple terms under the radical.
Avoiding these common mistakes will help ensure that your simplifications of square roots are accurate and effective.
Additional Resources and Practice Problems
For those looking to deepen their understanding of simplifying square roots, here are some valuable resources and practice problems to explore:
Online Resources
- : Step-by-step solutions and explanations for simplifying square roots.
- : Detailed methods and examples for finding and simplifying square roots.
- : Easy-to-understand tutorials with examples and interactive features.
- : Clear steps and explanations for simplifying square roots.
- : Comprehensive guide on square roots, including prime factorization and long division methods.
Practice Problems
- Simplify the square root of 50.
- Simplify the square root of 72.
- What is the simplest form of the square root of 98?
- Simplify: 3√8 + 2√18.
- Simplify the square root of 200 and express it in simplest radical form.
- If √x = 2√6, find the value of x.
Example Problems with Solutions
Here are a couple of solved examples to help you understand the process:
| Problem | Solution |
|---|---|
| Simplify the square root of 50 |
Step 1: Find factors of 50: 1, 2, 5, 10, 25, 50 Step 2: Identify the perfect squares: 1, 25 Step 3: Divide 50 by the largest perfect square: 50 / 25 = 2 Step 4: Calculate the square root of the perfect square: √25 = 5 Step 5: Combine the results: √50 = 5√2 |
| Simplify the square root of 72 |
Step 1: Find factors of 72: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72 Step 2: Identify the perfect squares: 1, 4, 9, 36 Step 3: Divide 72 by the largest perfect square: 72 / 36 = 2 Step 4: Calculate the square root of the perfect square: √36 = 6 Step 5: Combine the results: √72 = 6√2 |
Interactive Quizzes
Test your skills with these interactive quizzes:
Practicing these problems and utilizing these resources will enhance your understanding and ability to simplify square roots effectively. Happy learning!
Conclusion
In this guide, we have thoroughly explored the steps to simplify the square root of 32. We began by understanding the concept of square roots and perfect squares, followed by the prime factorization of 32. We then broke down the process of simplifying the square root by identifying perfect square factors and combining like terms to achieve the final simplified form of \( \sqrt{32} = 4\sqrt{2} \).
The steps outlined not only simplify the expression but also provide a clear method that can be applied to other square roots. Remember the key points:
- Prime factorization helps in identifying perfect square factors.
- Simplifying square roots involves breaking down the expression into its factors and combining like terms.
- The simplified form \( 4\sqrt{2} \) is the most concise way to express \( \sqrt{32} \).
By mastering these steps, you can confidently simplify square roots and apply this knowledge to various mathematical problems. Practice regularly with different numbers to enhance your understanding and efficiency. For further learning, consider exploring more complex roots and their applications in algebra and geometry.
We hope this guide has been informative and helpful in your mathematical journey. Continue practicing and exploring additional resources to deepen your understanding and proficiency in simplifying square roots.

Làm Thế Nào Để Đơn Giản Hóa Căn Bậc Hai Của 32: √32
READ MORE:
Video này hướng dẫn cách đơn giản hóa căn bậc hai của 32, giúp bạn hiểu rõ hơn về quy trình và ứng dụng của nó.
Cách Đơn Giản Hóa Căn Bậc Hai của 32










