Topic simplify square roots fractions: Master the art of simplifying square root fractions with our comprehensive guide. Learn step-by-step methods, tips, and examples to effortlessly handle square roots in fractions. Whether you're a student or just looking to refresh your math skills, this guide will make simplifying square root fractions a breeze.
Table of Content
- How to Simplify Square Root Fractions
- Introduction to Simplifying Square Root Fractions
- Understanding Square Roots and Fractions
- Basic Principles of Simplification
- Examples of Simplifying Square Root Fractions
- Rationalizing the Denominator
- Combining Square Roots in Fractions
- Simplifying Complex Fractions with Square Roots
- Common Mistakes and How to Avoid Them
- Practice Problems and Solutions
- Advanced Techniques for Simplification
- Applications of Simplified Square Root Fractions
- Summary and Key Takeaways
- Additional Resources and References
- YOUTUBE:
How to Simplify Square Root Fractions
Simplifying square root fractions involves reducing the fraction to its simplest form while dealing with the square roots in both the numerator and the denominator. Here are the steps to simplify square root fractions:
Steps to Simplify Square Root Fractions
- Identify the square root in the fraction.
- Rationalize the denominator if needed.
- Reduce the fraction to its simplest form.
Step-by-Step Example
Consider the fraction \(\frac{\sqrt{8}}{\sqrt{2}}\).
- First, simplify the square roots if possible. Since \(8 = 4 \times 2\), we can write:
- \(\sqrt{8} = \sqrt{4 \times 2} = \sqrt{4} \times \sqrt{2} = 2\sqrt{2}\)
- The fraction becomes:
- \(\frac{2\sqrt{2}}{\sqrt{2}}\)
- Next, divide the terms with square roots:
- \(\frac{2\sqrt{2}}{\sqrt{2}} = 2\)
Another Example
Let's simplify the fraction \(\frac{\sqrt{50}}{\sqrt{2}}\).
- Simplify the square root in the numerator. Since \(50 = 25 \times 2\), we have:
- \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}\)
- The fraction is now:
- \(\frac{5\sqrt{2}}{\sqrt{2}}\)
- Divide the terms with square roots:
- \(\frac{5\sqrt{2}}{\sqrt{2}} = 5\)
General Rule
For a fraction of the form \(\frac{\sqrt{a}}{\sqrt{b}}\), you can simplify by combining the square roots:
- \(\frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}}\)
If the denominator is not a perfect square, rationalize by multiplying the numerator and the denominator by the square root of the denominator:
- \(\frac{\sqrt{a}}{\sqrt{b}} \times \frac{\sqrt{b}}{\sqrt{b}} = \frac{\sqrt{a} \cdot \sqrt{b}}{b} = \frac{\sqrt{ab}}{b}\)
Practice Problems
Try simplifying these fractions:
- \(\frac{\sqrt{12}}{\sqrt{3}}\)
- \(\frac{\sqrt{18}}{\sqrt{2}}\)
- \(\frac{\sqrt{45}}{\sqrt{5}}\)
By following these steps and practicing, you can master simplifying square root fractions.

READ MORE:
Introduction to Simplifying Square Root Fractions
Simplifying square root fractions involves reducing fractions that contain square roots in the numerator, the denominator, or both, to their simplest form. This process is essential in mathematics as it makes complex expressions more manageable and easier to work with. Let's break down the steps and principles involved in this process.
Here are the key steps to simplify square root fractions:
- Identify and simplify the square roots in the fraction.
- Rationalize the denominator if it contains a square root.
- Combine and reduce the fraction to its simplest form.
To understand these steps better, let's delve into each one in detail:
- Identifying and Simplifying Square Roots: Look at the numerator and the denominator separately. Simplify any square roots that can be reduced. For example,
\(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\). - Rationalizing the Denominator: If the denominator contains a square root, multiply the numerator and the denominator by the square root to eliminate it. For example,
\(\frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}\). - Combining and Reducing: Combine the terms and simplify the fraction. For example,
\(\frac{2\sqrt{3}}{\sqrt{3}} = 2\).
By following these steps, you can simplify square root fractions effectively and efficiently. Practice with different fractions to gain confidence and improve your skills.
Understanding Square Roots and Fractions
Square roots and fractions are fundamental concepts in mathematics, and understanding how to work with them together is crucial for simplifying square root fractions. Here, we will break down these concepts and explain their properties step-by-step.
What is a Square Root?
A square root of a number \(x\) is a number \(y\) such that \(y^2 = x\). For example, the square root of 9 is 3 because \(3^2 = 9\). Square roots can be denoted using the radical symbol \(\sqrt{}\).
What is a Fraction?
A fraction represents a part of a whole and is written in the form \(\frac{a}{b}\), where \(a\) is the numerator and \(b\) is the denominator. Fractions can be simplified if the numerator and denominator have common factors.
Square Roots of Fractions
The square root of a fraction \(\frac{a}{b}\) can be expressed as a fraction of square roots: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\). This property allows us to simplify the square roots of fractions more easily.
Example:
Let's simplify \(\sqrt{\frac{4}{9}}\):
- \(\sqrt{\frac{4}{9}} = \frac{\sqrt{4}}{\sqrt{9}}\)
- \(\sqrt{4} = 2\) and \(\sqrt{9} = 3\)
- So, \(\sqrt{\frac{4}{9}} = \frac{2}{3}\)
Rationalizing the Denominator
Sometimes, it is necessary to eliminate the square root from the denominator of a fraction. This process is known as rationalizing the denominator. To do this, multiply both the numerator and the denominator by the square root in the denominator.
Example:
Simplify \(\frac{5}{\sqrt{2}}\):
- Multiply the numerator and the denominator by \(\sqrt{2}\): \(\frac{5}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{5\sqrt{2}}{2}\)
Combining Square Roots in Fractions
When dealing with fractions that include square roots in both the numerator and the denominator, you can often simplify by combining the square roots before simplifying the fraction.
Example:
Simplify \(\frac{\sqrt{50}}{\sqrt{2}}\):
- Combine the square roots: \(\frac{\sqrt{50}}{\sqrt{2}} = \sqrt{\frac{50}{2}}\)
- Simplify the fraction under the radical: \(\sqrt{\frac{50}{2}} = \sqrt{25}\)
- Since \(\sqrt{25} = 5\), the simplified form is 5.
By understanding these concepts and practicing these steps, you can become proficient in simplifying square root fractions, making it easier to work with these expressions in more complex mathematical problems.
Basic Principles of Simplification
Simplifying square root fractions involves a few fundamental principles that make the process manageable and intuitive. By understanding and applying these principles, you can simplify complex expressions into their simplest forms.
-
Simplify the Square Root
Start by simplifying any square roots in the fraction. This means finding the largest perfect square that can be factored out of the square root.
- Example: Simplify \(\sqrt{72}\)
- \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\)
-
Rationalize the Denominator
If the fraction has a square root in the denominator, rationalize it by multiplying both the numerator and denominator by the conjugate of the denominator. This eliminates the square root from the denominator.
- Example: Rationalize \(\frac{5}{\sqrt{3}}\)
- \(\frac{5}{\sqrt{3}} = \frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
-
Combine Like Terms
After simplifying and rationalizing, combine any like terms. This often involves simplifying further or factoring out common terms.
- Example: Simplify \(\frac{\sqrt{18}}{3}\)
- \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
- \(\frac{\sqrt{18}}{3} = \frac{3\sqrt{2}}{3} = \sqrt{2}\)
By following these principles, you can simplify square root fractions effectively. Practice with various examples to become more comfortable with these techniques.
Examples of Simplifying Square Root Fractions
Here are some examples to help illustrate the process of simplifying square root fractions:
Example 1: Simplify \(\sqrt{\frac{18}{50}}\)
- Factor both the numerator and the denominator to identify any perfect squares:
\(\sqrt{\frac{18}{50}} = \sqrt{\frac{9 \times 2}{25 \times 2}} = \sqrt{\frac{9}{25}}\)
- Simplify the square roots of the perfect squares:
\(\sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5}\)
- Therefore, \(\sqrt{\frac{18}{50}} = \frac{3}{5}\).
Example 2: Simplify \(\sqrt{\frac{27}{75}}\)
- Factor both the numerator and the denominator to identify any perfect squares:
\(\sqrt{\frac{27}{75}} = \sqrt{\frac{9 \times 3}{25 \times 3}} = \sqrt{\frac{9}{25}}\)
- Simplify the square roots of the perfect squares:
\(\sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5}\)
- Therefore, \(\sqrt{\frac{27}{75}} = \frac{3}{5}\).
Example 3: Simplify \(\sqrt{\frac{50}{128}}\)
- Reduce the fraction by dividing both numerator and denominator by their greatest common divisor:
\(\frac{50}{128} = \frac{25}{64}\)
- Separate the square root into the numerator and denominator:
\(\sqrt{\frac{25}{64}} = \frac{\sqrt{25}}{\sqrt{64}}\)
- Simplify the square roots:
\(\frac{\sqrt{25}}{\sqrt{64}} = \frac{5}{8}\)
- Therefore, \(\sqrt{\frac{50}{128}} = \frac{5}{8}\).
Example 4: Simplify \(\frac{\sqrt{32}}{\sqrt{8}}\)
- Combine the square roots:
\(\frac{\sqrt{32}}{\sqrt{8}} = \sqrt{\frac{32}{8}} = \sqrt{4}\)
- Simplify the square root:
\(\sqrt{4} = 2\)
- Therefore, \(\frac{\sqrt{32}}{\sqrt{8}} = 2\).
Example 5: Simplify \(\frac{2\sqrt{18}}{3\sqrt{2}}\)
- Simplify the square roots in the numerator and the denominator:
\(\frac{2\sqrt{18}}{3\sqrt{2}} = \frac{2 \times 3\sqrt{2}}{3\sqrt{2}} = \frac{6\sqrt{2}}{3\sqrt{2}}\)
- Cancel out the common terms:
\(\frac{6\sqrt{2}}{3\sqrt{2}} = 2\)
- Therefore, \(\frac{2\sqrt{18}}{3\sqrt{2}} = 2\).

Rationalizing the Denominator
Rationalizing the denominator is a process used to eliminate radicals from the denominator of a fraction. This is often done to simplify expressions and make them easier to work with. Here are the steps to rationalize the denominator:
-
Identify the Radical in the Denominator:
If the denominator contains a square root, we need to eliminate it by multiplying both the numerator and the denominator by a suitable expression.
-
Multiply by the Conjugate (for Binomial Denominators):
For denominators that are binomials (expressions with two terms), multiply the numerator and the denominator by the conjugate of the denominator. The conjugate is formed by changing the sign between the terms in the denominator.
Example: To rationalize \(\frac{2}{3 + \sqrt{3}}\), multiply by the conjugate \(\frac{3 - \sqrt{3}}{3 - \sqrt{3}}\).
Perform the multiplication:
- Numerator: \(2 \cdot (3 - \sqrt{3}) = 6 - 2\sqrt{3}\)
- Denominator: \((3 + \sqrt{3})(3 - \sqrt{3}) = 9 - (\sqrt{3})^2 = 9 - 3 = 6\)
Result: \(\frac{6 - 2\sqrt{3}}{6} = 1 - \frac{\sqrt{3}}{3}\)
-
Multiply by the Same Radical (for Monomial Denominators):
For denominators that are single terms, multiply both the numerator and the denominator by the same radical.
Example: To rationalize \(\frac{1}{\sqrt{2}}\), multiply by \(\frac{\sqrt{2}}{\sqrt{2}}\).
Perform the multiplication:
- Numerator: \(1 \cdot \sqrt{2} = \sqrt{2}\)
- Denominator: \(\sqrt{2} \cdot \sqrt{2} = 2\)
Result: \(\frac{\sqrt{2}}{2}\)
-
Simplify the Resulting Expression:
After rationalizing the denominator, simplify the fraction if possible by reducing it to its lowest terms.
By following these steps, you can rationalize the denominator of any fraction containing square roots, making the expression simpler and more standardized.
Combining Square Roots in Fractions
Combining square roots in fractions involves the use of various mathematical properties and rules. Here, we will explore how to combine square roots in fractions step-by-step.
Steps to Combine Square Roots in Fractions
- Simplify Individual Radicals:
Before combining, simplify each square root separately if possible. For example, \(\sqrt{8}\) can be simplified to \(2\sqrt{2}\).
- Apply the Quotient Property of Square Roots:
The quotient property states that \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\), where \(a\) and \(b\) are non-negative numbers and \(b \neq 0\).
For example, \(\sqrt{\frac{18}{50}}\) can be written as \(\frac{\sqrt{18}}{\sqrt{50}}\).
- Simplify the Fraction:
Simplify the fraction inside the square root if possible. This can make the square root easier to manage.
For example, \(\sqrt{\frac{18}{50}}\) simplifies to \(\sqrt{\frac{9}{25}}\) because both the numerator and the denominator have common factors.
- Combine Like Terms:
If you have multiple terms with square roots, combine like terms.
For example, \(\frac{\sqrt{3}}{2} + \frac{2\sqrt{3}}{2}\) combines to \(\frac{3\sqrt{3}}{2}\).
- Rationalize the Denominator if Needed:
If the denominator contains a square root, you may need to rationalize it by multiplying the numerator and the denominator by the same square root.
For example, \(\frac{1}{\sqrt{2}}\) can be rationalized to \(\frac{\sqrt{2}}{2}\).
Examples
- Example 1: Simplify \(\sqrt{\frac{8}{50}}\)
Step 1: Simplify the fraction inside the square root: \(\frac{8}{50} = \frac{4}{25}\).
Step 2: Apply the quotient property: \(\sqrt{\frac{4}{25}} = \frac{\sqrt{4}}{\sqrt{25}} = \frac{2}{5}\).
- Example 2: Simplify \(\sqrt{\frac{18}{50}}\)
Step 1: Simplify the fraction inside the square root: \(\frac{18}{50} = \frac{9}{25}\).
Step 2: Apply the quotient property: \(\sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5}\).
- Example 3: Combine \(\frac{\sqrt{2}}{3} + \frac{\sqrt{2}}{6}\)
Step 1: Find a common denominator: \(\frac{2\sqrt{2}}{6} + \frac{\sqrt{2}}{6} = \frac{3\sqrt{2}}{6} = \frac{\sqrt{2}}{2}\).
By following these steps, you can effectively combine square roots in fractions, simplifying them to their most reduced form.
Simplifying Complex Fractions with Square Roots
Simplifying complex fractions with square roots involves a series of steps that make the process systematic and straightforward. Here are the detailed steps:
-
Identify the Numerator and Denominator:
Start by clearly identifying the numerator and denominator of the complex fraction. For example, consider the complex fraction \(\frac{\sqrt{8}}{\frac{\sqrt{2}}{3}}\).
-
Combine the Fractions:
Combine the fractions into a single fraction by multiplying by the reciprocal of the denominator. For instance, \(\frac{\sqrt{8}}{\frac{\sqrt{2}}{3}} = \sqrt{8} \times \frac{3}{\sqrt{2}}\).
-
Simplify the Square Roots:
Simplify the square roots in the fraction. In our example, \(\sqrt{8} = 2\sqrt{2}\), so the fraction becomes \(2\sqrt{2} \times \frac{3}{\sqrt{2}}\).
-
Multiply the Numerator and Denominator:
Multiply the numerators and denominators. Here, \(2\sqrt{2} \times \frac{3}{\sqrt{2}} = \frac{6\sqrt{2}}{\sqrt{2}} = 6\).
-
Simplify the Result:
Finally, simplify the result to its lowest terms. If there are any common factors, divide them out.
Let's look at some examples to illustrate these steps:
-
Example 1: Simplify \(\frac{\sqrt{18}}{3}\)
- Simplify the square root: \(\sqrt{18} = 3\sqrt{2}\)
- Combine the fractions: \(\frac{3\sqrt{2}}{3}\)
- Simplify: \(\sqrt{2}\)
The simplified form is \(\sqrt{2}\).
-
Example 2: Simplify \(\frac{2}{\sqrt{12}}\)
- Simplify the square root: \(\sqrt{12} = 2\sqrt{3}\)
- Rationalize the denominator: \(\frac{2}{2\sqrt{3}} = \frac{2}{2\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{2\sqrt{3}}{6} = \frac{\sqrt{3}}{3}\)
The simplified form is \(\frac{\sqrt{3}}{3}\).
-
Example 3: Simplify \(\frac{\sqrt{6}}{\sqrt{2}}\)
- Simplify the square roots: \(\sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \times \sqrt{3}\)
- Combine the fractions: \(\frac{\sqrt{2} \times \sqrt{3}}{\sqrt{2}}\)
- Simplify: \(\sqrt{3}\)
The simplified form is \(\sqrt{3}\).
By following these steps, you can simplify complex fractions with square roots effectively and efficiently.
Common Mistakes and How to Avoid Them
When simplifying square root fractions, there are several common mistakes that students often make. Being aware of these pitfalls can help you avoid them and achieve accurate results. Here are the most frequent errors and tips on how to steer clear of them:
- Not Simplifying the Square Root Completely:
Ensure that the square root is simplified as much as possible. Use prime factorization to identify the largest perfect square factor. For example, simplify √72 as follows:
√72 = √(36 × 2) = √36 × √2 = 6√2
- Incorrectly Rationalizing the Denominator:
When rationalizing the denominator, multiply both the numerator and the denominator by the necessary square root to eliminate the square root from the denominator. For instance:
5/√3 = (5/√3) × (√3/√3) = 5√3/3
- Forgetting to Simplify the Fraction:
After simplifying the square root and rationalizing the denominator, always check if the fraction itself can be further reduced. For example:
√18/3 = (3√2)/3 = √2
- Mixing Up Operations:
Always simplify square roots before performing operations with fractions. Be careful with multiplication and division steps. For example, simplify √50 to 5√2 before dividing by √2:
√50/√2 = (5√2)/√2 = 5
- Overlooking Negative Signs:
Negative signs can significantly affect the outcome. Always pay close attention to them, especially when dealing with square roots. For instance:
√(-4)/2 is not the same as -√4/2 = -2/2 = -1.
By being mindful of these common mistakes and applying the correct techniques, you can confidently simplify square root fractions and tackle more complex mathematical problems.
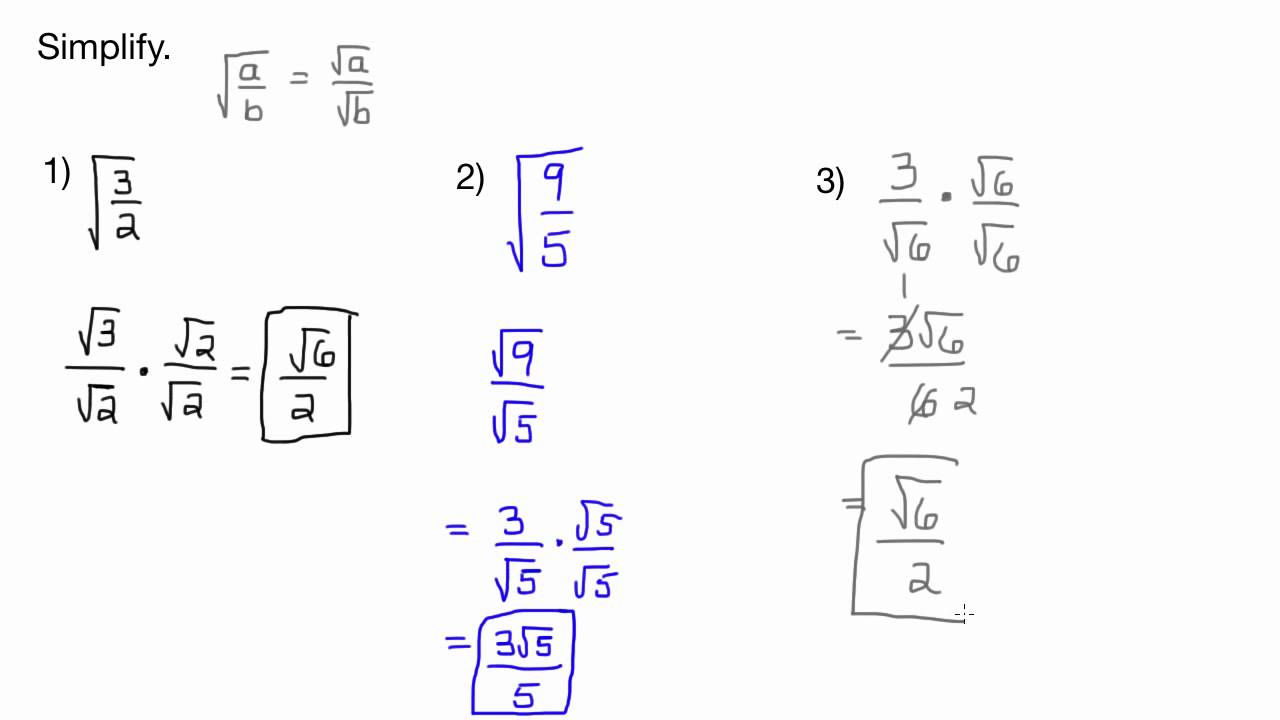
Practice Problems and Solutions
Sharpen your skills in simplifying square root fractions with these practice problems and detailed solutions:
-
Problem 1: Simplify \( \frac{\sqrt{50}}{\sqrt{2}} \)
Solution: First, factor \( \sqrt{50} \) into \( \sqrt{25 \cdot 2} = 5\sqrt{2} \). Then, \( \frac{5\sqrt{2}}{\sqrt{2}} = 5 \).
-
Problem 2: Simplify \( \frac{1}{\sqrt{3}} \)
Solution: Rationalize the denominator by multiplying both the numerator and the denominator by \( \sqrt{3} \):
\( \frac{1}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{3}}{3} \). -
Problem 3: Simplify \( \frac{\sqrt{18} + \sqrt{2}}{\sqrt{9}} \)
Solution: Simplify \( \sqrt{18} \) to \( 3\sqrt{2} \) and \( \sqrt{9} \) to 3:
\( \frac{3\sqrt{2} + \sqrt{2}}{3} = \frac{4\sqrt{2}}{3} = \frac{4\sqrt{2}}{3} \). -
Problem 4: Simplify \( \frac{\sqrt{4/9}} \)
Solution: The square root of 4 is 2, and the square root of 9 is 3:
\( \sqrt{\frac{4}{9}} = \frac{2}{3} \). -
Problem 5: Simplify \( \sqrt{\frac{25}{49}} \)
Solution: The square root of 25 is 5, and the square root of 49 is 7:
\( \sqrt{\frac{25}{49}} = \frac{5}{7} \). -
Problem 6: Simplify \( \sqrt{\frac{2}{3}} \)
Solution: Since 2 and 3 are not perfect squares, the expression remains as:
\( \sqrt{\frac{2}{3}} \) or rationalized to \( \frac{\sqrt{2}}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{6}}{3} \). -
Problem 7: Simplify \( \frac{4}{\sqrt{18}} \)
Solution: Simplify \( \sqrt{18} \) to \( 3\sqrt{2} \), then rationalize the denominator:
\( \frac{4}{3\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{4\sqrt{2}}{6} = \frac{2\sqrt{2}}{3} \). -
Problem 8: Simplify \( \frac{3\sqrt{5}}{5} \)
Solution: Simplify directly:
\( \frac{3\sqrt{5}}{5} \).
Practice these problems to become more comfortable with simplifying square root fractions. With time and practice, you will develop a deeper understanding and become proficient in this area.
Advanced Techniques for Simplification
Simplifying square root fractions, especially those involving complex expressions, requires advanced techniques. Here are some strategies to master:
- Prime Factorization: Break down both the numerator and the denominator into their prime factors. This makes it easier to identify and simplify square roots within the fraction.
- Using Conjugates for Rationalization: If the denominator contains a square root, multiply both the numerator and the denominator by the conjugate of the denominator. For example, if the denominator is \( \sqrt{a} + \sqrt{b} \), its conjugate is \( \sqrt{a} - \sqrt{b} \).
- Applying the Distributive Property: For fractions involving algebraic expressions, apply the distributive property to simplify the expression inside the square root before simplifying the fraction.
- Leveraging the Pythagorean Identity: In cases involving trigonometric functions, use the Pythagorean identity to simplify square roots of fractions by converting them into trigonometric terms.
- Utilizing Complex Numbers: For square roots of negative fractions, use complex numbers. Represent the square root of a negative number as an imaginary number (i).
Below are detailed steps to apply these techniques:
1. Prime Factorization
- Find the prime factors of both the numerator and the denominator.
- Identify and extract the largest perfect square factor from both.
- Simplify the square roots accordingly.
Example:
Simplify \( \sqrt{\frac{50}{8}} \).
- Prime factors of 50: \( 2 \times 5^2 \).
- Prime factors of 8: \( 2^3 \).
- Simplify: \( \sqrt{\frac{2 \times 5^2}{2^3}} = \sqrt{\frac{25}{4}} = \frac{5}{2} \).
2. Using Conjugates for Rationalization
- Identify the conjugate of the denominator.
- Multiply both the numerator and the denominator by the conjugate.
- Apply the distributive property to simplify.
Example:
Simplify \( \frac{1}{\sqrt{3} + \sqrt{2}} \).
- Conjugate: \( \sqrt{3} - \sqrt{2} \).
- Multiply: \( \frac{1}{\sqrt{3} + \sqrt{2}} \times \frac{\sqrt{3} - \sqrt{2}}{\sqrt{3} - \sqrt{2}} = \frac{\sqrt{3} - \sqrt{2}}{(\sqrt{3})^2 - (\sqrt{2})^2} = \frac{\sqrt{3} - \sqrt{2}}{3 - 2} = \sqrt{3} - \sqrt{2} \).
3. Applying the Distributive Property
- Distribute the terms inside the square root.
- Simplify the resulting expression.
Example:
Simplify \( \sqrt{\frac{a^2 + 2ab + b^2}{c^2}} \).
- Distribute: \( \sqrt{\frac{(a + b)^2}{c^2}} \).
- Simplify: \( \frac{a + b}{c} \).
4. Leveraging the Pythagorean Identity
- Convert square root expressions into trigonometric terms using the Pythagorean identity.
- Simplify the resulting trigonometric expressions.
Example:
Simplify \( \sqrt{1 - \sin^2(x)} \).
- Use the identity \( \cos^2(x) = 1 - \sin^2(x) \).
- Simplify: \( \sqrt{\cos^2(x)} = \cos(x) \).
5. Utilizing Complex Numbers
- Recognize square roots of negative numbers and represent them as imaginary numbers.
- Simplify using properties of imaginary numbers.
Example:
Simplify \( \sqrt{\frac{-4}{9}} \).
- Rewrite: \( \sqrt{\frac{-4}{9}} = \frac{\sqrt{-4}}{\sqrt{9}} = \frac{2i}{3} \).
Mastering these advanced techniques will significantly enhance your ability to simplify complex square root fractions.
Applications of Simplified Square Root Fractions
Simplified square root fractions have numerous applications across various fields. Here are some key examples:
1. Physics
Square root fractions are often used in physics, especially in equations involving gravity and motion. For instance, the time \( t \) it takes for an object to fall from a height \( h \) can be calculated using the formula:
This application helps determine the duration of free fall, which is crucial in experiments and safety calculations.
2. Engineering
In engineering, simplified square root fractions are used in structural analysis. For example, the natural frequency \( f \) of a vibrating system can be determined using the formula:
where \( k \) is the stiffness and \( m \) is the mass of the system. Simplifying these square root fractions ensures accurate and efficient design.
3. Mathematics
Simplified square root fractions are foundational in various mathematical concepts and proofs. They appear in quadratic equations, trigonometric identities, and calculus problems, making the process of finding solutions more straightforward.
4. Computer Science
Algorithms involving computational geometry often use square root fractions. For example, calculating the Euclidean distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) involves the formula:
Simplifying these expressions improves the efficiency and accuracy of algorithms.
5. Everyday Life
Square root fractions are used in everyday measurements and conversions. For example, converting between different units of area or volume often requires simplification for practical use.
6. Finance
In finance, square root fractions are used in risk assessment models, such as calculating the standard deviation of investment returns. Simplifying these calculations helps in making informed financial decisions.
7. Statistics
Square root fractions appear in various statistical formulas, including the calculation of confidence intervals and the standard error of the mean:
where \( \sigma \) is the standard deviation and \( n \) is the sample size. Simplification aids in clearer interpretation of data.
These examples illustrate the broad utility of simplified square root fractions in enhancing understanding and solving real-world problems efficiently.
Summary and Key Takeaways
Understanding how to simplify square root fractions is an essential skill in algebra and higher-level mathematics. Here are the key takeaways from our comprehensive guide:
- Basics of Square Roots: A square root of a number is a value that, when multiplied by itself, gives the original number. The notation is √, and for any non-negative number \(a\), \( \sqrt{a} \) is a number \( b \) such that \( b^2 = a \).
- Fractions Involving Square Roots: When working with fractions that include square roots, you can simplify them by applying the property \( \sqrt{a/b} = \sqrt{a} / \sqrt{b} \).
- Rationalizing the Denominator: This process involves eliminating the square root from the denominator by multiplying both the numerator and the denominator by the square root present in the denominator.
- Simplification Techniques: Simplifying square roots often involves factoring the number inside the square root into its prime factors and then applying the property \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \).
- Combining Square Roots: When adding or subtracting square roots, they must be like terms. For example, \( 2\sqrt{3} + 3\sqrt{3} = 5\sqrt{3} \).
- Common Mistakes: Avoid errors such as not simplifying fully, failing to rationalize the denominator, or incorrectly combining non-like terms.
- Advanced Techniques: These include dealing with higher-order roots, using conjugates for complex rationalizations, and applying algebraic identities to simplify expressions.
- Practical Applications: Simplified square root fractions are used in various fields such as physics, engineering, and computer science, where precise calculations are crucial.
By mastering these principles, you'll be able to handle square root fractions with confidence and accuracy. Regular practice with a variety of problems will reinforce your understanding and proficiency in these techniques.

Additional Resources and References
For those who wish to deepen their understanding of simplifying square root fractions, here are some valuable resources and references:
-
This site offers comprehensive explanations and examples for simplifying fractions with square roots, including detailed steps for rationalizing the denominator and simplifying complex fractions.
-
Online Math Learning provides video lessons and examples on simplifying square roots that include fractions. It covers various techniques such as reducing fractions to perfect squares and using the quotient rule.
-
This resource explains the quotient property of square roots and provides numerous examples to help learners practice and master the simplification process.
-
Socratic offers community-driven explanations and step-by-step solutions to various mathematical problems, including simplifying fractions with square roots and rationalizing denominators.
These resources will provide you with a deeper understanding and additional practice to confidently tackle any square root fraction simplification problems you encounter.
Đơn Giản Hóa Phân Số Dưới Dấu Căn - Bí Quyết Toán Học Hợp Pháp
READ MORE:
Đơn Giản Hóa Căn Bậc Hai Của Phân Số: sqrt(a/b), a/sqrt(b)