Topic simplify a square root fraction: Learning how to simplify a square root fraction is a vital math skill that can make complex equations easier to solve. This guide provides clear, step-by-step instructions to help you master this technique, ensuring you can tackle square root fractions with confidence and precision.
Table of Content
- Simplifying a Square Root Fraction
- Introduction to Simplifying Square Root Fractions
- Understanding Square Roots and Fractions
- Basic Steps to Simplify Square Root Fractions
- Examples of Simplifying Square Root Fractions
- Simplifying Fractions with Square Roots in the Numerator
- Simplifying Fractions with Square Roots in the Denominator
- Rationalizing the Denominator
- Advanced Techniques for Simplifying Square Root Fractions
- Common Mistakes and How to Avoid Them
- Practice Problems and Solutions
- Applications of Simplified Square Root Fractions
- Conclusion and Summary
- YOUTUBE: Xem video này để học cách giản lược căn bậc hai của phân số và áp dụng vào các bài tập và ví dụ thực tế.
Simplifying a Square Root Fraction
Understanding how to simplify square root fractions is an essential skill in algebra. This process involves two main steps: simplifying the square root and rationalizing the denominator. Below, we will outline the detailed steps along with examples to help you master this concept.
Step-by-Step Guide
-
Simplify the Square Root
Identify the largest perfect square that factors into the number under the square root.
- For example, \( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \).
-
Rationalize the Denominator
To eliminate the square root from the denominator, multiply both the numerator and denominator by the conjugate of the denominator.
- For instance, to simplify \( \frac{5}{\sqrt{3}} \), multiply by \( \frac{\sqrt{3}}{\sqrt{3}} \):
- \[ \frac{5}{\sqrt{3}} = \frac{5 \times \sqrt{3}}{\sqrt{3} \times \sqrt{3}} = \frac{5\sqrt{3}}{3} \]
Examples of Simplifying Fractions with Square Roots
| Expression | Steps | Result |
|---|---|---|
| \( \frac{\sqrt{18}}{3} \) |
|
\( \sqrt{2} \) |
| \( \frac{2}{\sqrt{12}} \) |
|
\( \frac{\sqrt{3}}{3} \) |
| \( \frac{\sqrt{6}}{\sqrt{2}} \) |
|
\( \sqrt{3} \) |
With practice, simplifying fractions involving square roots becomes more intuitive. Remember to first simplify the square root if possible and then eliminate any square roots from the denominator by rationalizing it.
Practice Problems
- Simplify \( \frac{3\sqrt{5}}{5} \)
- Simplify \( \frac{4}{\sqrt{18}} \)
Attempt these problems to solidify your understanding of simplifying square root fractions.

READ MORE:
Introduction to Simplifying Square Root Fractions
Simplifying square root fractions involves reducing the fraction to its simplest form where the numerator and the denominator are as simplified as possible. This process can make complex equations more manageable and is an essential skill in algebra and higher-level math. Follow these steps to simplify square root fractions effectively:
- Identify the square root fraction: Recognize the fraction that contains a square root in either the numerator, denominator, or both.
- Simplify the square roots: Simplify the square roots in the numerator and the denominator separately, if possible. For example, \(\sqrt{50}\) can be simplified to \(5\sqrt{2}\).
- Rationalize the denominator: If the square root is in the denominator, multiply both the numerator and the denominator by the square root in the denominator to remove it. For example, to simplify \(\frac{1}{\sqrt{2}}\), multiply by \(\frac{\sqrt{2}}{\sqrt{2}}\) to get \(\frac{\sqrt{2}}{2}\).
- Simplify the resulting fraction: Reduce the resulting fraction to its simplest form by dividing the numerator and the denominator by their greatest common divisor (GCD).
Let's look at an example:
| Example: | \(\frac{\sqrt{18}}{\sqrt{2}}\) |
| Simplify the square roots: | \(\frac{\sqrt{18}}{\sqrt{2}} = \frac{3\sqrt{2}}{\sqrt{2}}\) |
| Rationalize the denominator: | \(\frac{3\sqrt{2}}{\sqrt{2}} = 3\) |
By following these steps, you can simplify any square root fraction, making your mathematical work easier and more accurate.
Understanding Square Roots and Fractions
To simplify square root fractions effectively, it's essential to have a clear understanding of both square roots and fractions. This section will provide a foundational overview of these concepts and how they interact.
Square Roots
A square root of a number \( x \) is a number \( y \) such that \( y^2 = x \). In other words, when \( y \) is multiplied by itself, it equals \( x \). The square root is denoted as \( \sqrt{x} \).
- Perfect Squares: Numbers like 1, 4, 9, 16, and 25 are perfect squares because their square roots are whole numbers (e.g., \( \sqrt{25} = 5 \)).
- Non-Perfect Squares: Numbers like 2, 3, 5, 10, and 20 are not perfect squares. Their square roots are irrational numbers (e.g., \( \sqrt{2} \approx 1.414 \)).
Fractions
A fraction represents a part of a whole and is expressed as \( \frac{a}{b} \), where \( a \) is the numerator and \( b \) is the denominator. The numerator indicates how many parts are being considered, while the denominator indicates the total number of equal parts in the whole.
- Simplifying Fractions: A fraction is simplified by dividing the numerator and the denominator by their greatest common divisor (GCD). For example, \( \frac{8}{12} \) can be simplified to \( \frac{2}{3} \).
- Rational and Irrational Fractions: Fractions can be rational (both numerator and denominator are integers) or can involve irrational numbers (e.g., \( \frac{\sqrt{3}}{2} \)).
Combining Square Roots and Fractions
When dealing with square roots in fractions, the same principles of simplifying fractions apply, but additional steps may be needed to handle the square roots:
- Identify Square Roots: Locate the square root in the numerator, denominator, or both.
- Simplify Square Roots: Simplify the square roots where possible. For example, \( \sqrt{50} \) can be simplified to \( 5\sqrt{2} \).
- Rationalize the Denominator: If the square root is in the denominator, multiply both the numerator and the denominator by the square root in the denominator to eliminate it. For instance, \( \frac{1}{\sqrt{2}} \) is rationalized to \( \frac{\sqrt{2}}{2} \).
By understanding these fundamental concepts, you can approach simplifying square root fractions with confidence and clarity.
Basic Steps to Simplify Square Root Fractions
To simplify square root fractions, follow these basic steps:
-
Simplify the Square Root:
Identify and simplify the square roots in the fraction. Look for the largest perfect square factor within the square root and simplify. For example:
\(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\)
-
Simplify the Fraction (if possible):
If both the numerator and the denominator are square roots, you can simplify them. For example:
\(\frac{\sqrt{8}}{\sqrt{2}} = \sqrt{\frac{8}{2}} = \sqrt{4} = 2\)
-
Rationalize the Denominator:
If there is a square root in the denominator, rationalize it by multiplying both the numerator and the denominator by the conjugate or by the square root itself to eliminate the square root in the denominator. For example:
- Simple case:
- Binomial denominator:
\(\frac{5}{\sqrt{3}} = \frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
\(\frac{2}{3+\sqrt{3}} = \frac{2}{3+\sqrt{3}} \times \frac{3-\sqrt{3}}{3-\sqrt{3}} = \frac{2(3-\sqrt{3})}{(3+\sqrt{3})(3-\sqrt{3})} = \frac{6-2\sqrt{3}}{9-3} = \frac{6-2\sqrt{3}}{6} = 1-\frac{\sqrt{3}}{3}\)
By following these steps, you can simplify square root fractions effectively.
Examples of Simplifying Square Root Fractions
Simplifying square root fractions involves several steps, including finding common factors, rationalizing the denominator, and simplifying the resulting expressions. Below are some detailed examples:
Example 1: Simplify \(\frac{3\sqrt{7}}{\sqrt{14}}\)
Find the Greatest Common Factor (GCF): The GCF between the numerator \(3\sqrt{7}\) and the denominator \(\sqrt{14}\) is \(\sqrt{7}\).
Divide the numerator and the denominator by \(\sqrt{7}\):
\[
\frac{3\sqrt{7}}{\sqrt{14}} = \frac{3\sqrt{7}}{\sqrt{7} \cdot \sqrt{2}} = \frac{3}{\sqrt{2}}
\]Rationalize the denominator: Multiply both the numerator and the denominator by \(\sqrt{2}\).
\[
\frac{3}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{3\sqrt{2}}{2}
\]
Therefore, \(\frac{3\sqrt{7}}{\sqrt{14}}\) simplifies to \(\frac{3\sqrt{2}}{2}\).
Example 2: Simplify \(\frac{\sqrt{18}}{6}\)
Factorize the radicand: \(\sqrt{18} = \sqrt{9 \cdot 2} = \sqrt{9} \cdot \sqrt{2} = 3\sqrt{2}\).
Simplify the fraction:
\[
\frac{3\sqrt{2}}{6} = \frac{3}{6} \cdot \sqrt{2} = \frac{1}{2} \cdot \sqrt{2} = \frac{\sqrt{2}}{2}
\]
Therefore, \(\frac{\sqrt{18}}{6}\) simplifies to \(\frac{\sqrt{2}}{2}\).
Example 3: Simplify \(\frac{\sqrt{50}}{\sqrt{2}}\)
Combine the radicals: Use the quotient property of radicals \(\frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}}\).
\[
\frac{\sqrt{50}}{\sqrt{2}} = \sqrt{\frac{50}{2}} = \sqrt{25} = 5
\]
Therefore, \(\frac{\sqrt{50}}{\sqrt{2}}\) simplifies to \(5\).
Example 4: Simplify \(\frac{\sqrt{8}}{4}\)
Factorize the radicand: \(\sqrt{8} = \sqrt{4 \cdot 2} = \sqrt{4} \cdot \sqrt{2} = 2\sqrt{2}\).
Simplify the fraction:
\[
\frac{2\sqrt{2}}{4} = \frac{2}{4} \cdot \sqrt{2} = \frac{1}{2} \cdot \sqrt{2} = \frac{\sqrt{2}}{2}
\]
Therefore, \(\frac{\sqrt{8}}{4}\) simplifies to \(\frac{\sqrt{2}}{2}\).
These examples illustrate the process of simplifying square root fractions by finding common factors, rationalizing the denominator, and using properties of radicals to simplify the expressions further.

Simplifying Fractions with Square Roots in the Numerator
Simplifying fractions with square roots in the numerator involves reducing the square root expression and the fraction to their simplest forms. Below are the steps to simplify such fractions:
-
Simplify the square root in the numerator: Break down the square root into its prime factors and simplify. For instance, if the square root in the numerator is \( \sqrt{18} \), you can simplify it as follows:
\[
\sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2}
\] -
Reduce the fraction if possible: If there is a common factor between the numerator and the denominator, divide both by that factor. For example:
\[
\frac{3\sqrt{2}}{6} = \frac{3}{6} \times \sqrt{2} = \frac{1}{2} \times \sqrt{2} = \frac{\sqrt{2}}{2}
\]
Let's look at some specific examples:
-
Example 1: Simplify \( \frac{\sqrt{50}}{5} \)
Step 1: Simplify the square root:
\[
\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}
\]Step 2: Reduce the fraction:
\[
\frac{5\sqrt{2}}{5} = \sqrt{2}
\] -
Example 2: Simplify \( \frac{\sqrt{32}}{8} \)
Step 1: Simplify the square root:
\[
\sqrt{32} = \sqrt{16 \times 2} = 4\sqrt{2}
\]Step 2: Reduce the fraction:
\[
\frac{4\sqrt{2}}{8} = \frac{1}{2}\sqrt{2} = \frac{\sqrt{2}}{2}
\]
These steps and examples illustrate the process of simplifying fractions with square roots in the numerator. Remember to always look for ways to simplify the square root first and then reduce the fraction to its simplest form.
Simplifying Fractions with Square Roots in the Denominator
Simplifying fractions that have square roots in the denominator involves a process called rationalizing the denominator. This technique eliminates the square root from the denominator, making the fraction easier to work with. Here are the detailed steps to simplify such fractions:
-
Identify the fraction: Consider a fraction of the form \( \frac{a}{\sqrt{b}} \), where \( a \) and \( b \) are real numbers, and \( \sqrt{b} \) is the square root in the denominator.
-
Multiply by the conjugate: To rationalize the denominator, multiply both the numerator and the denominator by the conjugate of the denominator. In this case, the conjugate of \( \sqrt{b} \) is \( \sqrt{b} \) itself. Therefore, multiply the fraction by \( \frac{\sqrt{b}}{\sqrt{b}} \).
For example: \( \frac{a}{\sqrt{b}} \times \frac{\sqrt{b}}{\sqrt{b}} \).
-
Perform the multiplication: Multiply the numerators together and the denominators together:
\[
\frac{a \cdot \sqrt{b}}{\sqrt{b} \cdot \sqrt{b}} = \frac{a \cdot \sqrt{b}}{b}
\] -
Simplify the fraction: If possible, simplify the resulting fraction by reducing it to its lowest terms.
For example, if \( a = 2 \) and \( b = 3 \), the simplified fraction would be:
\[
\frac{2 \cdot \sqrt{3}}{3}
\]
Here are some specific examples to illustrate the process:
-
Example 1: Simplify \( \frac{5}{\sqrt{2}} \)
- Multiply by the conjugate: \( \frac{5}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} \)
- Perform the multiplication: \( \frac{5 \cdot \sqrt{2}}{2} = \frac{5\sqrt{2}}{2} \)
-
Example 2: Simplify \( \frac{7}{\sqrt{5}} \)
- Multiply by the conjugate: \( \frac{7}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} \)
- Perform the multiplication: \( \frac{7 \cdot \sqrt{5}}{5} = \frac{7\sqrt{5}}{5} \)
By following these steps, you can simplify fractions with square roots in the denominator, making them easier to work with in further calculations.
Rationalizing the Denominator
Rationalizing the denominator involves eliminating any square roots or other irrational numbers from the denominator of a fraction. This process helps to simplify the fraction and make it easier to work with in further calculations. Follow these steps to rationalize the denominator:
-
Identify the Irrational Denominator:
If you have a fraction with a square root in the denominator, such as \( \frac{1}{\sqrt{2}} \), note that the square root is the irrational component that needs to be removed.
-
Multiply by the Conjugate:
For a denominator with a single square root, multiply both the numerator and the denominator by that square root. For example:
\[
\frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}
\]If the denominator is a binomial involving a square root, such as \(3 - \sqrt{2}\), multiply by its conjugate \(3 + \sqrt{2}\). The conjugate is formed by changing the sign between the terms. For example:
\[
\frac{1}{3 - \sqrt{2}} \times \frac{3 + \sqrt{2}}{3 + \sqrt{2}} = \frac{3 + \sqrt{2}}{(3 - \sqrt{2})(3 + \sqrt{2})} = \frac{3 + \sqrt{2}}{9 - 2} = \frac{3 + \sqrt{2}}{7}
\] -
Simplify the Fraction:
Ensure that the new fraction is in its simplest form. If necessary, simplify the numerator and the denominator further by factoring out common terms or performing additional arithmetic operations.
Here is another example to illustrate the process:
-
Consider the fraction \( \frac{5}{\sqrt{3}} \).
To rationalize the denominator, multiply both the numerator and the denominator by \( \sqrt{3} \):
\[
\frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}
\] -
For a more complex example, consider \( \frac{2}{4 + \sqrt{5}} \).
Multiply by the conjugate of the denominator, \(4 - \sqrt{5}\):
\[
\frac{2}{4 + \sqrt{5}} \times \frac{4 - \sqrt{5}}{4 - \sqrt{5}} = \frac{2(4 - \sqrt{5})}{(4 + \sqrt{5})(4 - \sqrt{5})} = \frac{8 - 2\sqrt{5}}{16 - 5} = \frac{8 - 2\sqrt{5}}{11}
\]
By following these steps, you can ensure that your fractions are in their simplest form, making them easier to use in calculations and further mathematical operations.
Advanced Techniques for Simplifying Square Root Fractions
When simplifying square root fractions, advanced techniques can help to further simplify complex expressions. Here are some detailed methods:
-
Prime Factorization:
Decompose both the numerator and the denominator into their prime factors. This helps in identifying and simplifying the square roots more efficiently.
- Example: Simplify \(\sqrt{\frac{72}{18}}\).
- Step 1: Prime factorize: \(\sqrt{\frac{36 \cdot 2}{9 \cdot 2}} = \sqrt{\frac{36}{9} \cdot \frac{2}{2}}\).
- Step 2: Simplify inside the square roots: \(\frac{\sqrt{36}}{\sqrt{9}}\).
- Step 3: Calculate the square roots: \(\frac{6}{3} = 2\).
-
Using Conjugates for Rationalization:
To remove a square root from the denominator, multiply the numerator and the denominator by the conjugate of the denominator.
- Example: Simplify \(\frac{3}{\sqrt{2} + 1}\).
- Step 1: Multiply by the conjugate: \(\frac{3}{\sqrt{2} + 1} \cdot \frac{\sqrt{2} - 1}{\sqrt{2} - 1}\).
- Step 2: Apply the distributive property: \(\frac{3(\sqrt{2} - 1)}{(\sqrt{2})^2 - 1^2} = \frac{3\sqrt{2} - 3}{2 - 1}\).
- Step 3: Simplify: \(3\sqrt{2} - 3\).
-
Applying the Distributive Property:
For expressions involving algebra, apply the distributive property inside the square root before simplifying the fraction.
- Example: Simplify \(\sqrt{4x^2 + 4x + 1}\).
- Step 1: Recognize the perfect square trinomial: \(\sqrt{(2x + 1)^2}\).
- Step 2: Simplify the square root: \(2x + 1\).
-
Leveraging the Pythagorean Identity:
In cases involving trigonometric functions, use the Pythagorean identity to simplify square roots by converting them into trigonometric terms.
- Example: Simplify \(\sqrt{\sin^2 \theta + \cos^2 \theta}\).
- Step 1: Apply the Pythagorean identity: \(\sin^2 \theta + \cos^2 \theta = 1\).
- Step 2: Simplify the square root: \(\sqrt{1} = 1\).
-
Utilizing Complex Numbers:
For square roots of negative fractions, represent the square root of a negative number as an imaginary number (i).
- Example: Simplify \(\sqrt{\frac{-4}{9}}\).
- Step 1: Separate the square root: \(\frac{\sqrt{-4}}{\sqrt{9}}\).
- Step 2: Simplify using imaginary numbers: \(\frac{2i}{3}\).
These advanced techniques can significantly streamline the process of simplifying square root fractions, making complex expressions more manageable.
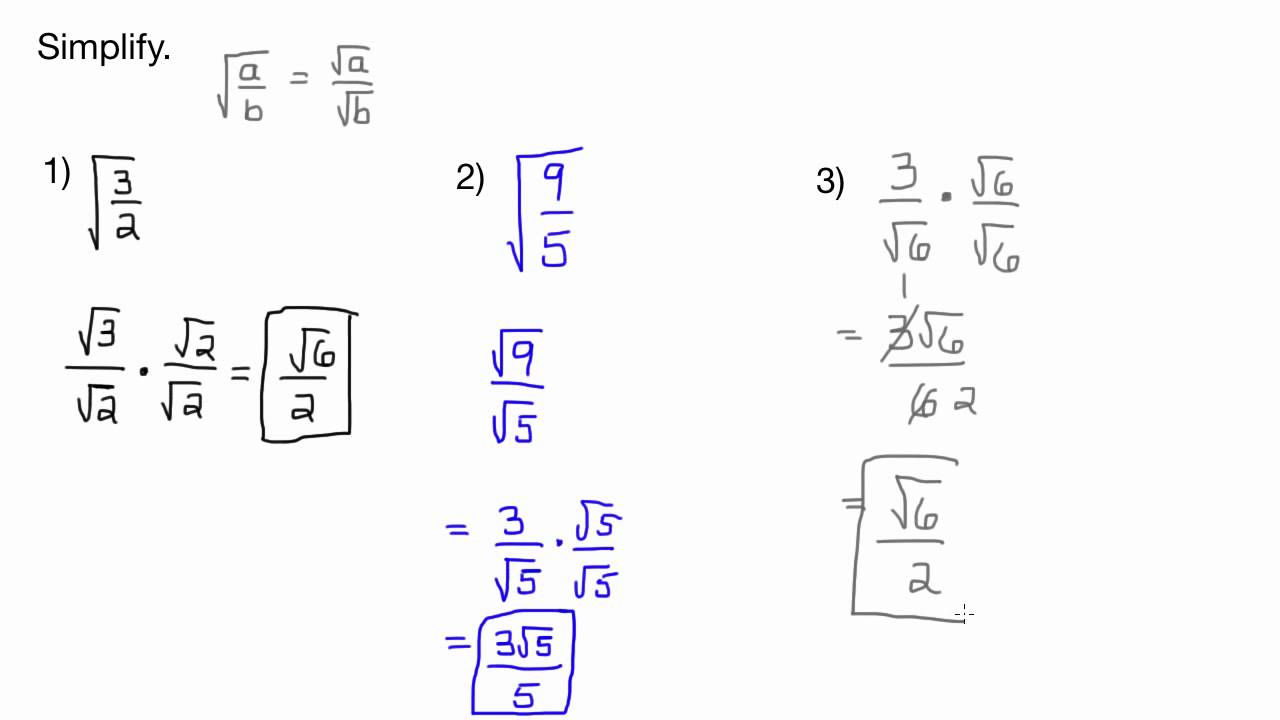
Common Mistakes and How to Avoid Them
When simplifying square root fractions, it's common to encounter pitfalls that can lead to errors. Here are some common mistakes to watch out for and tips on how to avoid them:
- Forgetting to Simplify Radicals: Always check if you can simplify the square roots in the numerator and denominator further before performing any operations. Simplifying radicals can often lead to simpler fractions.
- Ignoring the Rationalizing Technique: Sometimes, leaving a radical in the denominator can make calculations more complicated. Remember to use the rationalizing technique when necessary to eliminate radicals from the denominator.
- Incorrectly Distributing Exponents: When dealing with fractions containing square roots, be cautious when distributing exponents. Make sure to apply the exponent rules correctly to avoid errors in simplification.
- Misapplying Addition and Subtraction: Adding or subtracting fractions with square roots requires a common denominator. Ensure that you find the least common multiple of the denominators before performing these operations.
- Overlooking Conjugate Pairs: Utilizing conjugate pairs can simplify fractions with square roots in the denominator. Don't forget to identify and exploit these pairs to streamline your calculations.
By being mindful of these common mistakes and employing the recommended strategies, you can simplify square root fractions accurately and efficiently.
Practice Problems and Solutions
Here are some practice problems along with step-by-step solutions to help you master simplifying square root fractions:
- Problem 1: Simplify the fraction $\frac{\sqrt{18}}{\sqrt{8}}$.
- Solution:
First, factor the radicands:
$\sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2}$ $\sqrt{8} = \sqrt{4 \times 2} = \sqrt{4} \times \sqrt{2} = 2\sqrt{2}$ Now, divide the numerator and denominator by the greatest common factor:
$\frac{3\sqrt{2}}{2\sqrt{2}} = \frac{3}{2}$
So, the simplified form of $\frac{\sqrt{18}}{\sqrt{8}}$ is $\frac{3}{2}$.
- Problem 2: Simplify the expression $\frac{5\sqrt{12}}{\sqrt{27}}$.
- Solution:
Begin by simplifying the square roots:
$\sqrt{12} = \sqrt{4 \times 3} = \sqrt{4} \times \sqrt{3} = 2\sqrt{3}$ $\sqrt{27} = \sqrt{9 \times 3} = \sqrt{9} \times \sqrt{3} = 3\sqrt{3}$ Now, substitute the simplified radicals into the fraction:
$\frac{5 \times 2\sqrt{3}}{3\sqrt{3}}$
Cancel out the common factors:
$\frac{10}{3}$
Therefore, $\frac{5\sqrt{12}}{\sqrt{27}}$ simplifies to $\frac{10}{3}$.
- Problem 3: Simplify the fraction $\frac{\sqrt{75}}{\sqrt{27}}$.
- Solution:
First, factor the radicands:
$\sqrt{75} = \sqrt{25 \times 3} = \sqrt{25} \times \sqrt{3} = 5\sqrt{3}$ $\sqrt{27} = \sqrt{9 \times 3} = \sqrt{9} \times \sqrt{3} = 3\sqrt{3}$ Now, simplify the fraction:
$\frac{5\sqrt{3}}{3\sqrt{3}} = \frac{5}{3}$
Thus, $\frac{\sqrt{75}}{\sqrt{27}}$ simplifies to $\frac{5}{3}$.
Practice these problems to enhance your skills in simplifying square root fractions.
Applications of Simplified Square Root Fractions
Simplified square root fractions find numerous applications in various fields, showcasing their practical significance:
- Engineering: In engineering disciplines such as civil, mechanical, and electrical engineering, simplified square root fractions are commonly encountered in calculations involving measurements, dimensions, and complex equations.
- Physics: Simplified square root fractions are extensively used in physics, especially in equations related to waveforms, harmonic motion, and quantum mechanics. They help in simplifying complex mathematical expressions in theoretical physics and experimental data analysis.
- Finance: In financial analysis and modeling, simplified square root fractions are utilized in calculations involving interest rates, risk assessment, and investment portfolios. They enable analysts and investors to make informed decisions by simplifying complex financial formulas.
- Computer Science: Simplified square root fractions play a role in algorithms, data analysis, and computational geometry within the field of computer science. They are employed in optimizing code efficiency, designing algorithms, and solving computational problems.
- Statistics: Statistical analysis often involves computations with square root fractions, particularly in calculating measures of dispersion, standard deviation, and variance. Simplifying these fractions aids in interpreting and drawing conclusions from statistical data.
- Education: In educational settings, simplified square root fractions are taught as fundamental mathematical concepts. They help students understand mathematical principles, problem-solving techniques, and real-world applications across various academic disciplines.
By mastering the simplification of square root fractions, individuals can enhance their proficiency in these diverse fields and effectively tackle complex problems with confidence.
Conclusion and Summary
In conclusion, simplifying square root fractions is a valuable skill with widespread applications across various disciplines. Through this comprehensive guide, we have explored the fundamentals of simplifying square root fractions, including basic techniques, advanced strategies, common mistakes to avoid, practice problems with solutions, and real-world applications.
By understanding the principles outlined in this guide and practicing the provided examples, individuals can enhance their mathematical proficiency and problem-solving abilities. Whether in engineering, physics, finance, computer science, statistics, or education, the ability to simplify square root fractions is indispensable.
Remember to approach each problem systematically, carefully applying the techniques learned, and verifying solutions for accuracy. With dedication and practice, mastering the simplification of square root fractions will empower you to tackle complex mathematical challenges with confidence and precision.

Xem video này để học cách giản lược căn bậc hai của phân số và áp dụng vào các bài tập và ví dụ thực tế.
Giản Lược Căn Bậc Hai của Phân Số: $\sqrt{\frac{a}{b}}$, $\frac{a}{\sqrt{b}}$
READ MORE:
Hãy xem cách dễ dàng đơn giản hóa phân số dưới dấu căn mà không vi phạm luật trong toán học.
Đơn giản hóa phân số dưới dấu căn - Cách giúp việc toán học dễ dàng hơn






