Topic 7 square root 2: Discover the fascinating world of 7 square root 2, a mathematical expression that opens up new insights into geometry, algebra, and real-world applications. This article delves into the significance of 7√2, exploring its calculation, properties, and uses, providing a comprehensive understanding for math enthusiasts and students alike.
Table of Content
- Mathematical Expression: \(7 \sqrt{2}\)
- Introduction to Square Roots
- The Mathematical Meaning of 7 Square Root 2
- Calculation Methods for 7 Square Root 2
- Properties and Characteristics of Square Roots
- Applications of Square Roots in Real Life
- Simplifying Expressions Involving Square Roots
- Common Mistakes in Square Root Calculations
- Advanced Techniques for Working with Square Roots
- Square Roots in Geometry and Trigonometry
- Historical Development of Square Roots
- Visualizing 7 Square Root 2 with Graphs
- Conclusion and Further Reading
- YOUTUBE: Video hướng dẫn cách tìm căn bậc hai của (7+2√10) để thu hút người xem và giải thích rõ ràng bằng tiếng Việt.
Mathematical Expression: \(7 \sqrt{2}\)
The expression \(7 \sqrt{2}\) involves a multiplication of the number 7 with the square root of 2. This can be understood in various mathematical contexts and has applications in different fields.
Understanding \( \sqrt{2} \)
The square root of 2, denoted as \( \sqrt{2} \), is an irrational number approximately equal to 1.414213562. It cannot be expressed as a simple fraction, and its decimal representation goes on infinitely without repeating.
Value of \(7 \sqrt{2}\)
To calculate the value of \(7 \sqrt{2}\), you multiply 7 by the approximate value of \( \sqrt{2} \):
\[
7 \times \sqrt{2} \approx 7 \times 1.414213562 = 9.899494937
\]
Properties and Applications
- Geometry: In geometry, \(7 \sqrt{2}\) can represent the length of the diagonal of a square with side length 7. This is derived from the Pythagorean theorem.
- Trigonometry: It can appear in various trigonometric functions and identities.
- Physics: The expression is used in calculations involving wave functions and other physical phenomena where root values are significant.
Example Calculations
Here are a few example problems involving \(7 \sqrt{2}\):
- Geometry Problem: Find the diagonal of a square with a side length of 7 units.
- Solution: The diagonal \(d\) is given by \(d = 7 \sqrt{2} \approx 9.899494937\) units.
- Scaling Problem: If an object is scaled by \( \sqrt{2} \) and its original measurement is 7 units, find the new measurement.
- Solution: The new measurement is \(7 \sqrt{2} \approx 9.899494937\) units.
Graphical Representation
The value \(7 \sqrt{2}\) can be represented graphically on a number line or within geometric shapes to illustrate its magnitude and relationship to other numbers.
Using \(7 \sqrt{2}\) in various mathematical contexts helps in understanding the interplay between whole numbers and irrational numbers, enhancing problem-solving skills in both theoretical and practical scenarios.

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, gives the original number. The square root symbol is \( \sqrt{} \), and it is used to denote the principal (non-negative) square root of a number.
For example:
- The square root of 9 is 3, since \( 3 \times 3 = 9 \).
- The square root of 16 is 4, since \( 4 \times 4 = 16 \).
Square roots are used in various fields, including algebra, geometry, and calculus. They are essential for solving quadratic equations, analyzing geometric shapes, and understanding various natural phenomena.
Consider the expression \( 7 \sqrt{2} \). This means 7 times the square root of 2. The square root of 2 is an irrational number, approximately equal to 1.414. Therefore:
\[ 7 \sqrt{2} \approx 7 \times 1.414 = 9.898 \]
Here is a step-by-step explanation of how to calculate the square root of a number:
- Identify the number you want to find the square root of.
- Use a calculator or estimation method to find the square root. For example, the square root of 25 is 5.
- If the number is not a perfect square, use a calculator to get a decimal approximation. For example, the square root of 2 is approximately 1.414.
Square roots also have properties that simplify calculations:
- \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \)
- \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \) for \( b \neq 0 \)
- \( (\sqrt{a})^2 = a \)
Understanding square roots helps in solving equations and understanding the nature of numbers. Whether dealing with simple arithmetic or advanced calculus, square roots play a crucial role in mathematical problem-solving.
The Mathematical Meaning of 7 Square Root 2
The expression \( 7 \sqrt{2} \) combines a whole number and an irrational number, creating a product that holds significance in various mathematical contexts. To fully understand this expression, let's break it down step by step:
1. Understanding the Components:
- 7: A whole number, also known as an integer.
- \( \sqrt{2} \): The square root of 2, which is an irrational number approximately equal to 1.414. An irrational number cannot be expressed as a simple fraction and has an infinite, non-repeating decimal expansion.
2. Multiplication of Components:
When you multiply a whole number by an irrational number, you get a product that is also irrational. Therefore:
\[ 7 \sqrt{2} \approx 7 \times 1.414 \approx 9.898 \]
This approximation helps us understand the magnitude of the product, but the exact value remains in its simplest form as \( 7 \sqrt{2} \).
3. Mathematical Properties:
- \( 7 \sqrt{2} \) can be simplified in various mathematical operations, such as algebraic expressions and equations.
- The expression retains the properties of square roots and multiplication, such as:
- \( 7 \sqrt{2} \times \sqrt{2} = 7 \times 2 = 14 \)
- \( 7 \sqrt{2} \div 7 = \sqrt{2} \)
4. Applications in Geometry and Algebra:
In geometry, \( 7 \sqrt{2} \) can represent lengths, areas, or other measurements that involve the square root of 2. For example, in a right-angled isosceles triangle with legs of length 7, the hypotenuse would be \( 7 \sqrt{2} \).
In algebra, \( 7 \sqrt{2} \) might appear in solutions to equations or in simplifying expressions, demonstrating the versatility of combining whole numbers with irrational numbers.
Understanding \( 7 \sqrt{2} \) provides insight into how mathematical operations extend beyond integers and rational numbers, bridging the gap to more complex and intriguing numerical relationships.
Calculation Methods for 7 Square Root 2
Calculating \( 7 \sqrt{2} \) involves understanding both the multiplication of numbers and the properties of square roots. Here, we will explore different methods to calculate this expression step by step.
1. Direct Multiplication Method:
- Identify the value of \( \sqrt{2} \), which is approximately 1.414.
- Multiply this value by 7.
\[ 7 \times 1.414 \approx 9.898 \]
2. Using a Calculator:
- Enter the value 2 into the calculator.
- Press the square root function to get \( \sqrt{2} \).
- Multiply the result by 7 to get the final value.
\[ 7 \sqrt{2} \approx 7 \times 1.414 \approx 9.898 \]
3. Estimation Method:
If you don't have a calculator, you can estimate the square root of 2 using simple arithmetic:
- Know that \( \sqrt{2} \) is between 1 and 2.
- Try squaring numbers between 1 and 2 to narrow down the value:
- \( 1.4^2 = 1.96 \) (close to 2)
- \( 1.5^2 = 2.25 \) (greater than 2)
- Use 1.414 as a close approximation and multiply by 7.
\[ 7 \times 1.414 \approx 9.898 \]
4. Algebraic Method:
- Express \( \sqrt{2} \) as an exponent:
\[ \sqrt{2} = 2^{0.5} \]
- Multiply by 7:
\[ 7 \sqrt{2} = 7 \times 2^{0.5} \]
5. Utilizing a Table of Square Roots:
- Find the value of \( \sqrt{2} \) from a table of square roots (typically found in math textbooks or online resources).
- Multiply the tabulated value by 7.
\[ 7 \times 1.414 \approx 9.898 \]
6. Computer Software:
- Use mathematical software like MATLAB, Python, or even a spreadsheet program to calculate:
- In Python:
\[ \text{import math} \]
\[ \text{result = 7 * math.sqrt(2)} \]
\[ \text{print(result)} \approx 9.898 \]
- In Python:
By understanding these various methods, you can accurately calculate \( 7 \sqrt{2} \) using tools and techniques suitable for your needs, whether through direct calculation, estimation, or the use of technology.
Properties and Characteristics of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. Square roots have several important properties and characteristics that are fundamental in mathematics.
- Non-Negative Numbers: The square root of a non-negative number is always non-negative. For example, √4 = 2.
- Principal Square Root: The principal square root is the non-negative square root of a number. For instance, the principal square root of 9 is 3, denoted as √9 = 3.
- Zero: The square root of zero is zero, which is unique: √0 = 0.
- Irrational Numbers: The square root of a non-perfect square is an irrational number. For example, √2 is irrational and cannot be expressed as a simple fraction.
- Multiplicative Property: The square root of a product is the product of the square roots of the factors:
\[
For example, \(\sqrt{36} = \sqrt{4 \cdot 9} = \sqrt{4} \cdot \sqrt{9} = 2 \cdot 3 = 6\).
\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}
\] - Quotient Property: The square root of a quotient is the quotient of the square roots:
\[
For example, \(\sqrt{\frac{9}{4}} = \frac{\sqrt{9}}{\sqrt{4}} = \frac{3}{2}\).
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\] - Exponential Form: The square root of a number can be written in exponential form as:
\[
\sqrt{a} = a^{\frac{1}{2}}
\] - Square of Square Root: The square of the square root of a number returns the original number:
\[
For instance, \((\sqrt{5})^2 = 5\).
(\sqrt{a})^2 = a
\] - Sum and Difference: The square root of a sum or difference does not equal the sum or difference of the square roots:
\[
\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}
\]\[
\sqrt{a - b} \neq \sqrt{a} - \sqrt{b}
\]
| Number | Square Root |
|---|---|
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
Understanding these properties helps in simplifying expressions and solving equations involving square roots. They are fundamental in various areas of mathematics, including algebra, geometry, and calculus.
Applications of Square Roots in Real Life
Square roots are used in various fields and everyday scenarios, making them a fundamental mathematical concept. Here are some notable applications:
-
Engineering and Architecture:
In engineering, square roots are used to calculate stresses, strains, and loads on structures. Architects use square roots to determine the dimensions and stability of buildings and bridges. For instance, the Pythagorean theorem, which involves square roots, is crucial in determining the lengths of sides in right-angled triangles, helping in the design of structures and ensuring their stability.
-
Finance:
Square roots are essential in finance, especially in calculating stock market volatility. The standard deviation, a measure of investment risk, is derived by taking the square root of the variance of stock returns. This helps investors make informed decisions by assessing the risk associated with different investments.
-
Science:
Square roots are used in various scientific calculations, such as determining the intensity of sound waves, the velocity of moving objects, and the absorption of radiation by materials. These applications help scientists understand natural phenomena and develop new technologies.
-
Statistics:
In statistics, square roots are used to calculate standard deviation, which measures how spread out the values in a data set are. This is crucial for data analysis, helping statisticians interpret data and make sound conclusions.
-
Geometry:
Square roots are fundamental in geometry for calculating areas and perimeters of shapes and solving problems involving right triangles. The Pythagorean theorem, which involves square roots, is widely used to find the lengths of sides in triangles.
-
Computer Science:
Square roots are used in algorithms for encryption, image processing, and game physics. For example, encryption algorithms use square roots to generate secure keys, ensuring data privacy and security.
-
Navigation:
In navigation, square roots are used to calculate distances between points on a map or globe. This is essential for determining the shortest path and estimating travel time, aiding pilots and sailors in their navigation tasks.
-
Electrical Engineering:
Square roots are used in electrical engineering to calculate power, voltage, and current in circuits. These calculations are vital for designing and analyzing electrical systems, ensuring their efficiency and safety.
-
Photography:
In photography, the f-number, which controls the aperture of a camera lens, is related to the square root of the area of the aperture. Adjusting the f-number changes the amount of light entering the camera, affecting the exposure and depth of field in photographs.
Square roots, therefore, play a crucial role in diverse fields, aiding in practical problem-solving and technological advancements.
Simplifying Expressions Involving Square Roots
Simplifying expressions involving square roots is a fundamental skill in algebra. Here are some key properties and steps to simplify such expressions:
Product Rule
The product rule states that the square root of a product is the product of the square roots:
\[\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\]
Quotient Rule
The quotient rule states that the square root of a quotient is the quotient of the square roots:
\[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}, \quad b \neq 0\]
Steps to Simplify Square Roots
- Identify perfect square factors within the radicand (the number inside the square root).
- Apply the product rule to split the square root into simpler parts.
- Simplify each part separately.
Examples
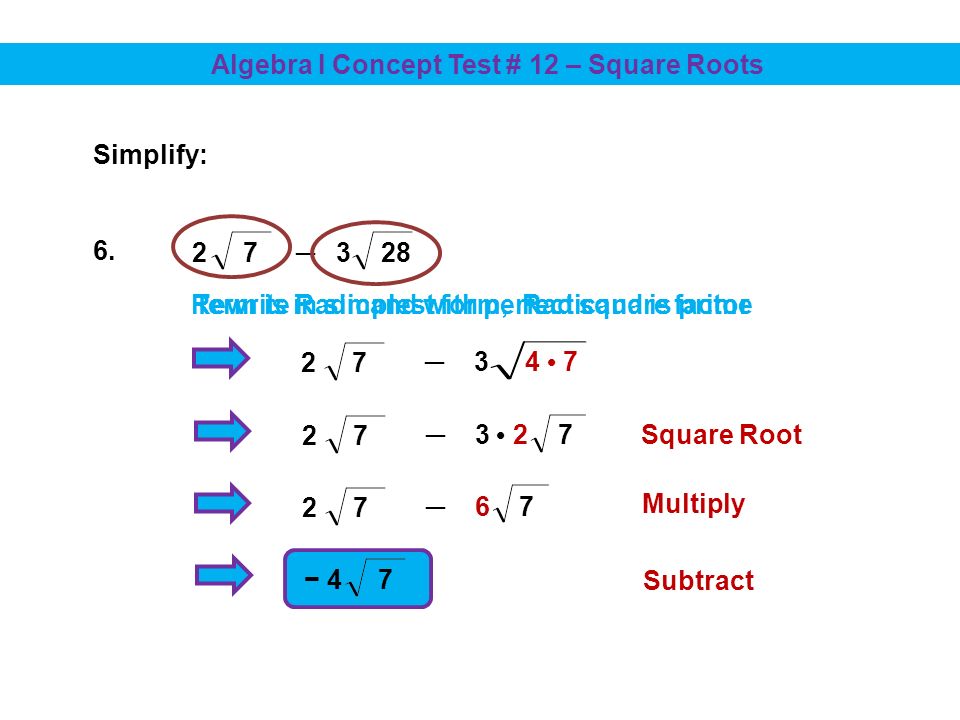
- Simplify \(\sqrt{72}\):
First, find the largest perfect square factor of 72, which is 36.
\[\sqrt{72} = \sqrt{36 \cdot 2} = \sqrt{36} \cdot \sqrt{2} = 6\sqrt{2}\]
- Simplify \(\sqrt{50}\):
The largest perfect square factor of 50 is 25.
\[\sqrt{50} = \sqrt{25 \cdot 2} = \sqrt{25} \cdot \sqrt{2} = 5\sqrt{2}\]
- Simplify \(\sqrt{32}\):
The largest perfect square factor of 32 is 16.
\[\sqrt{32} = \sqrt{16 \cdot 2} = \sqrt{16} \cdot \sqrt{2} = 4\sqrt{2}\]
Handling Variables
When the radicand includes variables, apply the product and quotient rules to both the numerical and variable parts:
- Simplify \(\sqrt{50x^4}\):
Break down the expression using the product rule:
\[\sqrt{50x^4} = \sqrt{25 \cdot 2 \cdot x^4} = \sqrt{25} \cdot \sqrt{2} \cdot \sqrt{x^4}\]
Then simplify each part:
\[\sqrt{25} = 5, \quad \sqrt{x^4} = x^2\]
So, \(\sqrt{50x^4} = 5x^2\sqrt{2}\)
- Simplify \(\sqrt{72a^3}\):
Identify the largest perfect square factor and apply the product rule:
\[\sqrt{72a^3} = \sqrt{36 \cdot 2 \cdot a^2 \cdot a} = 6a\sqrt{2a}\]
Advanced Example
Simplify \(\sqrt{200x^8y^3}\):
Identify and separate the perfect square factors:
\[\sqrt{200x^8y^3} = \sqrt{100 \cdot 2 \cdot x^8 \cdot y^2 \cdot y} = 10x^4y\sqrt{2y}\]
By breaking down the radicand into its prime factors or perfect squares, you can simplify complex square root expressions efficiently.
Common Mistakes in Square Root Calculations
Understanding square roots is crucial in mathematics, but there are common mistakes that learners often make. Identifying and correcting these mistakes can help in mastering square root calculations.
Incorrectly Simplifying Square Roots
One frequent mistake is failing to properly simplify square roots. Remember, you can only simplify a square root by factoring out perfect squares.
- Mistake: \(\sqrt{50} = 5\sqrt{2}\)
- Correction: \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \cdot \sqrt{2} = 5\sqrt{2}\)
Forgetting the ± Symbol
When solving equations involving square roots, it's essential to remember that both positive and negative roots are valid solutions.
- Mistake: \(x = \sqrt{25}\)
- Correction: \(x = \pm \sqrt{25} = \pm 5\)
Confusing the Product and Sum Rules
It's important not to mix up the rules for multiplying and adding square roots.
- Mistake: \(\sqrt{a + b} = \sqrt{a} + \sqrt{b}\)
- Correction: \(\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\)
- Correct Rule: \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\)
Ignoring the Domain of the Function
Square roots are only defined for non-negative numbers in the real number system.
- Mistake: Trying to find \(\sqrt{-4}\) in the real numbers.
- Correction: \(\sqrt{-4}\) is not defined in the real numbers. It is \(2i\) in the complex number system.
Misapplying the Quotient Rule
The quotient rule states that the square root of a quotient is the quotient of the square roots, provided the denominator is not zero.
- Mistake: \(\sqrt{\frac{a}{b}} = \frac{a}{\sqrt{b}}\)
- Correction: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}, \quad b \neq 0\)
Advanced Mistake: Simplifying Nested Radicals Incorrectly
Nested radicals can be tricky, and incorrect simplification is a common error.
- Mistake: \(\sqrt{\sqrt{16}}\)
- Correction: \(\sqrt{\sqrt{16}} = \sqrt{4} = 2\)
Examples
- Incorrect: \(\sqrt{18} = 3\sqrt{2}\)
- Correct: \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
By being aware of these common mistakes and understanding the correct methods, you can improve your accuracy in calculating square roots.
Advanced Techniques for Working with Square Roots
Square roots are fundamental in various fields of mathematics and science. Advanced techniques for working with square roots can help simplify calculations and solve complex problems efficiently. Here are some of the advanced methods:
- Babylonian Method (Heron's Method)
- Start with an initial guess \( x_0 \) for the square root of a number \( S \).
- Iteratively update the guess using the formula: \[ x_{n+1} = \frac{1}{2} \left( x_n + \frac{S}{x_n} \right) \]
- Continue the iterations until the difference between consecutive guesses is less than a predetermined tolerance.
- Simplifying Radical Expressions
- Factorize the radicand into its prime factors.
- Group the factors into pairs of identical factors.
- Take one factor from each pair out of the square root.
- Approximation Techniques
- Identify two integers between which the square root lies.
- Compute the arithmetic mean of these two integers as an approximation.
- Using Mathematical Software
- Import the necessary libraries.
- Use functions like
numpy.sqrt()for calculations. - Visualize the square root function using
matplotlibto plot the curve.
The Babylonian method is an ancient algorithm for finding the square root of a number. It is based on iterative averaging and can be implemented as follows:
This method converges quickly to the accurate value of the square root.
Simplifying square roots involves expressing the radicand as a product of prime factors and then applying the square root to each factor:
For example, to simplify \( \sqrt{72} \):
\[
\sqrt{72} = \sqrt{2^3 \cdot 3^2} = \sqrt{2^2 \cdot 2 \cdot 3^2} = 3 \cdot \sqrt{2} \cdot 3 = 6 \sqrt{2}
\]
When an exact square root is not necessary, approximation techniques can be used. One common method is to use the mean of two close integers:
For example, to approximate \( \sqrt{2.5} \):
\[
\sqrt{2.5} \approx \frac{1.5 + 2}{2} = 1.75
\]
Modern mathematical software, such as Python with libraries like NumPy and Matplotlib, can be used to compute and visualize square roots:
This approach provides precise and quick computations, especially for large datasets.
By mastering these advanced techniques, you can handle complex square root problems more effectively and gain deeper insights into their applications in various mathematical contexts.
Square Roots in Geometry and Trigonometry
Square roots play a crucial role in both geometry and trigonometry, helping to solve various problems related to lengths, areas, and angles. Here are some key applications:
- Pythagorean Theorem: In any right triangle, the length of the hypotenuse \( c \) can be found using the formula \( c = \sqrt{a^2 + b^2} \), where \( a \) and \( b \) are the lengths of the other two sides.
- Distance Formula: The distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) in the Cartesian plane is given by \( d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \).
- Area of a Triangle: For a triangle with sides of lengths \( a \), \( b \), and \( c \), and semi-perimeter \( s \), the area \( A \) can be found using Heron's formula: \( A = \sqrt{s(s-a)(s-b)(s-c)} \).
In trigonometry, square roots frequently appear in various identities and calculations:
- Trigonometric Ratios: In a right triangle, the sine, cosine, and tangent functions can involve square roots when dealing with special angles. For example, \( \sin(45^\circ) = \cos(45^\circ) = \frac{\sqrt{2}}{2} \).
- Unit Circle: The coordinates of points on the unit circle often involve square roots, such as \( \left(\frac{\sqrt{3}}{2}, \frac{1}{2}\right) \) for \( 30^\circ \) or \( \left(\frac{1}{2}, \frac{\sqrt{3}}{2}\right) \) for \( 60^\circ \).
- Special Right Triangles: In a 45-45-90 triangle, the lengths of the legs are equal, and the hypotenuse is \( \sqrt{2} \) times the length of a leg. In a 30-60-90 triangle, the hypotenuse is twice the length of the shorter leg, and the longer leg is \( \sqrt{3} \) times the length of the shorter leg.
Square roots are fundamental in understanding and solving geometric and trigonometric problems, providing a mathematical basis for many practical and theoretical applications.
Historical Development of Square Roots
The concept of square roots has a rich and diverse history, spanning several millennia and various cultures. Understanding the historical development of square roots provides insight into the evolution of mathematical thought and its practical applications.
- Ancient Civilizations:
The earliest known references to square roots date back to ancient Babylon and Egypt. Babylonian mathematicians around 2000 BCE used square roots in solving quadratic equations, with clay tablets demonstrating their methods. The Egyptians, particularly in the Rhind Mathematical Papyrus (circa 1650 BCE), also showed an understanding of square roots in their geometric calculations.
- Greek Contributions:
Greek mathematicians, including Pythagoras (circa 570-495 BCE) and Euclid (circa 300 BCE), made significant advancements in the understanding of square roots. Pythagoras's study of right triangles and the Pythagorean theorem inherently involved square roots. Euclid's "Elements" included propositions on square roots, establishing a foundation for future work in number theory and geometry.
- Indian and Islamic Scholars:
Indian mathematicians such as Aryabhata (476-550 CE) and Brahmagupta (598-668 CE) made notable contributions to the understanding and calculation of square roots. Aryabhata's works included algorithms for finding square roots, while Brahmagupta provided methods for solving quadratic equations involving square roots. Islamic scholars later translated and expanded upon these works during the Islamic Golden Age (8th to 14th centuries), preserving and enhancing the knowledge of square roots.
- Medieval Europe:
During the medieval period, European scholars gained access to ancient Greek and Islamic texts, leading to a revival of mathematical studies. Fibonacci (1170-1250 CE), an Italian mathematician, introduced the use of square roots in Europe through his book "Liber Abaci." The text presented the Hindu-Arabic numeral system and methods for arithmetic operations, including square roots.
- Modern Era:
In the Renaissance and beyond, mathematicians like René Descartes (1596-1650) and Isaac Newton (1643-1727) furthered the study of square roots. Descartes' development of analytical geometry utilized square roots in the context of Cartesian coordinates. Newton's work on calculus and numerical methods provided more sophisticated techniques for approximating square roots, such as the Newton-Raphson method.
Today, square roots are a fundamental concept in mathematics, with applications across various fields including engineering, physics, and computer science. Their historical development highlights the continuous and collaborative effort of mathematicians from different cultures and eras in advancing mathematical knowledge.
Visualizing 7 Square Root 2 with Graphs
Visualizing square roots, particularly complex expressions like \(7\sqrt{2}\), can be greatly facilitated using graphs. Below, we explore different ways to graphically represent the expression \(7\sqrt{2}\).
Graphing the Basic Square Root Function
The basic square root function \(y = \sqrt{x}\) can be visualized as a curve that starts at the origin (0,0) and increases slowly as \(x\) increases.
- The domain is \(x \geq 0\).
- The range is \(y \geq 0\).
- The function is undefined for negative values of \(x\).
The graph of \(y = \sqrt{x}\) looks like this:
Transformations of the Square Root Function
To visualize \(7\sqrt{2}\), we apply transformations to the basic square root function:
- Scaling: Multiplying by 7 scales the function vertically.
- Constant Multiplication: Incorporating \(\sqrt{2}\) affects the amplitude of the function.
So, the function \(y = 7\sqrt{2}\sqrt{x}\) becomes \(y = 7\sqrt{2x}\).
This transformation makes the graph steeper compared to the basic square root function:
Plotting Specific Points
To better understand the behavior of the function, let's plot some specific points. For example:
| x | y = 7\sqrt{2x} |
|---|---|
| 1 | 7\sqrt{2} \approx 9.899 |
| 4 | 7\sqrt{8} \approx 19.799 |
| 9 | 7\sqrt{18} \approx 29.698 |
These points confirm that as \(x\) increases, the value of \(y\) increases significantly due to the \(7\sqrt{2}\) factor.
Combining Graphs for Visualization
To fully appreciate the impact of the transformation, it can be helpful to overlay the graph of \(y = 7\sqrt{2}\) with the graph of \(y = \sqrt{x}\). This comparison clearly shows how the function is stretched and scaled.
Using graphing tools, such as graphing calculators or software like Desmos, can help create these visualizations more accurately and explore further transformations and their impacts on the graph.
Conclusion and Further Reading
The exploration of square roots, particularly in understanding and calculating expressions like \(7 \sqrt{2}\), reveals the depth and utility of this fundamental mathematical concept. Square roots are not only vital in theoretical mathematics but also have extensive applications in various fields such as geometry, trigonometry, physics, and engineering. By mastering the techniques for simplifying and manipulating square root expressions, one gains a powerful tool for solving complex mathematical problems.
In conclusion, square roots are an essential part of mathematics, offering both practical applications and deep theoretical insights. The journey from understanding the basic properties to applying advanced techniques showcases the versatility and importance of square roots in mathematical computations.
For further reading, consider exploring the following topics and resources:
- Books:
- The Square Root of 2: A Dialogue Concerning a Number and a Sequence by David Flannery
- How to Solve It by George Pólya - a classic book that delves into problem-solving strategies, including those involving square roots.
- Online Resources:
- - Comprehensive lessons and practice problems on square roots.
- - In-depth explanations and advanced problems involving square roots.
- Research Papers:
- Articles on the historical development of square roots and their applications in modern mathematics.
- Studies on the use of square roots in computational algorithms and numerical methods.
By continuing to explore these resources, you can deepen your understanding of square roots and their wide-ranging applications, enhancing both your theoretical knowledge and practical problem-solving skills.

Video hướng dẫn cách tìm căn bậc hai của (7+2√10) để thu hút người xem và giải thích rõ ràng bằng tiếng Việt.
Cách tìm căn bậc hai của (7+2√10)
READ MORE:
Khái Niệm Về Căn Bậc Hai | Toán Học Cùng Thầy J