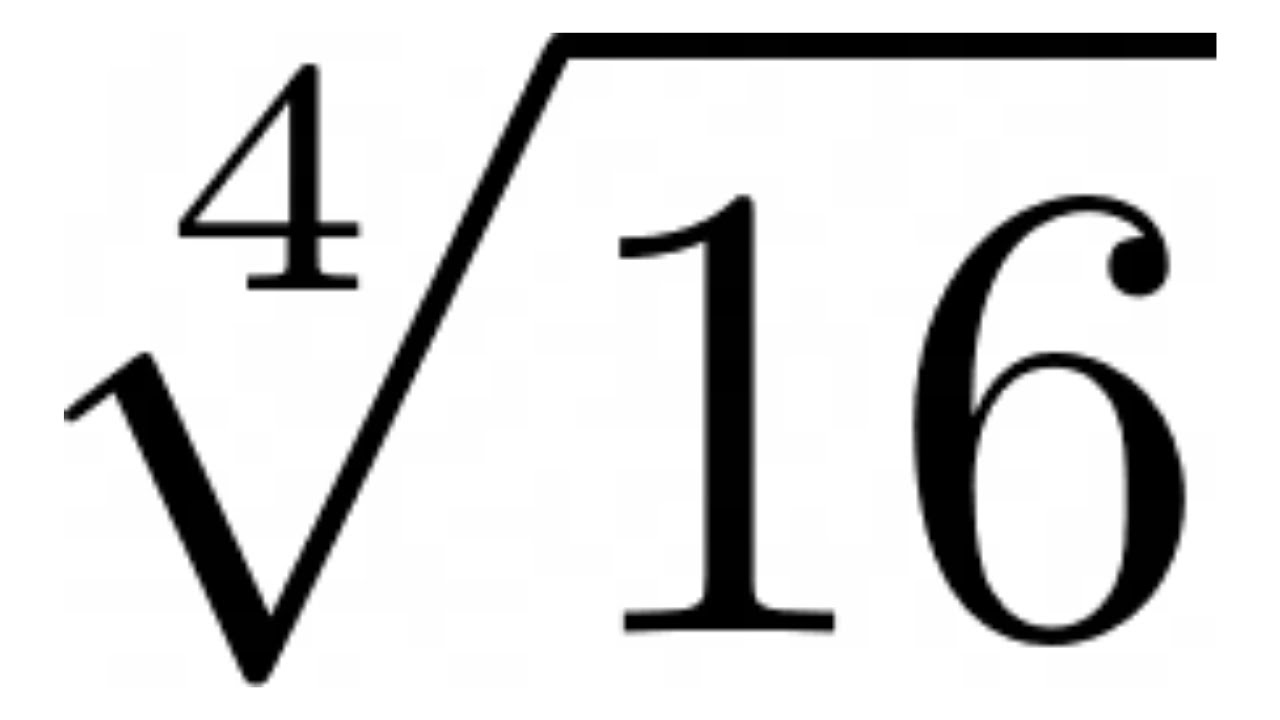Negative Square Root of 16: Understanding the Concept

The concept of square roots often brings up interesting discussions, especially when dealing with negative numbers. Let's dive into the details of finding the negative square root of 16.

Mathematical Definition

The square root of a number \( x \) is a number \( y \) such that \( y^2 = x \). When we talk about the square root of a negative number, we enter the realm of complex numbers.

For example, the square root of 16 is given by:

\(\sqrt{16} = 4\)

However, when we deal with the square root of -16, we need to use the imaginary unit \( i \), where \( i = \sqrt{-1} \).

Therefore, the square root of -16 is:

\(\sqrt{-16} = 4i\)

Additionally, \(-4i\) is also a square root of -16 since:

\((-4i)^2 = (-4)^2 \cdot i^2 = 16 \cdot (-1) = -16\)

Calculating Negative Square Roots

1. Rewrite the square root of the negative number using the imaginary unit \( i \).
2. Calculate the square root of the positive counterpart.
3. Combine the result with \( i \) to get the complex number.

Examples

• \(\sqrt{-4} = 2i\)
• \(\sqrt{-9} = 3i\)
• \(\sqrt{-25} = 5i\)

To sum up, the negative square root of 16 can be expressed as:

\(\sqrt{-16} = 4i \text{ or } -4i\)

These complex numbers are essential in various fields of science and engineering, particularly in solving equations that do not have real solutions.

Further Reading

The concept of square roots is fundamental in mathematics. The square root of a number \( x \) is a number \( y \) such that \( y^2 = x \). This means \( y \) when multiplied by itself gives \( x \). For example, both \( 4 \) and \( -4 \) are square roots of \( 16 \) because \( 4^2 = 16 \) and \( (-4)^2 = 16 \).

Square roots can be classified into two categories: principal and negative. The principal square root is the nonnegative root, denoted as \( \sqrt{x} \), while the negative square root is denoted as \( -\sqrt{x} \). For instance, the principal square root of 16 is \( \sqrt{16} = 4 \), and the negative square root of 16 is \( -\sqrt{16} = -4 \).

When dealing with negative numbers under the square root, the concept of imaginary numbers comes into play. The square root of a negative number is not a real number. Instead, it is an imaginary number. The imaginary unit \( i \) is defined as \( i = \sqrt{-1} \). Therefore, the square root of \( -16 \) can be expressed as \( \sqrt{-16} = 4i \), since \( i^2 = -1 \).

Here are some key points about square roots:

• Every positive real number has two square roots: one positive and one negative.
• The principal square root of a non-negative number is always non-negative.
• Imaginary numbers are used to represent the square roots of negative numbers.

Understanding square roots is essential for solving quadratic equations, simplifying expressions, and in various applications in science and engineering.

The concept of the square root of a negative number often puzzles many, but it becomes clearer with the introduction of complex numbers. Let's delve into the details.

In mathematics, the square root of a negative number cannot be represented as a real number because the square of any real number is always non-negative. However, using complex numbers, we can find the square root of negative numbers. The imaginary unit, denoted as \( i \), is defined by the property \( i^2 = -1 \). This allows us to express the square root of -16 in terms of \( i \).

• To find the square root of -16, we start by recognizing that: \[ \sqrt{-16} = \sqrt{16 \times (-1)} \]
• We can then separate this into: \[ \sqrt{16} \times \sqrt{-1} \]
• Since \(\sqrt{16} = 4\) and \(\sqrt{-1} = i\), we have: \[ \sqrt{-16} = 4i \]

Thus, the square root of -16 is \( 4i \). It's important to note that both \( 4i \) and \( -4i \) are solutions because:
\[
(4i)^2 = 16 \cdot i^2 = 16 \cdot (-1) = -16
\]
and
\[
(-4i)^2 = 16 \cdot (-1) = -16
\]

In summary, the square root of -16 is expressed in the form of complex numbers, with the principal square roots being \( \pm 4i \). This exploration not only helps in understanding the square roots of negative numbers but also introduces the fundamental concept of imaginary numbers, expanding the horizons of conventional arithmetic.

When dealing with square roots of negative numbers, we enter the realm of complex numbers. In mathematics, a complex number is a number that comprises both a real part and an imaginary part. The imaginary unit, denoted by \( i \), is defined as the square root of -1.

To understand the square root of -16, let's first express it as \( \sqrt{-16} \). Since the square root of a negative number is not a real number, we introduce the imaginary unit. Thus, \( \sqrt{-16} \) can be rewritten as \( \sqrt{16} \cdot i \).

Now, let's calculate the square root of 16, which is 4. So, \( \sqrt{-16} = 4i \).

Here, \( i \) represents the imaginary unit. It's important to note that complex numbers have both real and imaginary parts, with the imaginary part being multiplied by \( i \).

To calculate the square root of -16 and simplify it, we can follow these steps:

1. Express the square root of -16 as \( \sqrt{-16} \).
2. Recognize that the square root of a negative number involves complex numbers and the imaginary unit, denoted as \( i \).
3. Apply the property that \( \sqrt{-1} = i \).
4. Factor out the square root of 16, which equals 4.
5. Combine the real part (4) with the imaginary unit (i) to obtain the simplified form.

By following these steps, we find that the square root of -16 simplifies to \( 4i \).

Let's explore some examples of negative square roots:

1. Consider the square root of -16. We can express it as \( \sqrt{-16} \).
2. Apply the definition of the imaginary unit, denoted as \( i \), where \( i = \sqrt{-1} \).
3. Factor out the square root of 16, which is 4.
4. Combine the real part (4) with the imaginary unit (i) to get the simplified form, \( 4i \).

Similarly, other negative square roots follow this pattern of involving complex numbers and the imaginary unit.

Negative square roots, involving complex numbers and the imaginary unit, find applications in various mathematical concepts and fields:

• Complex Analysis: Negative square roots are fundamental in complex analysis, where complex numbers play a crucial role in understanding functions of a complex variable.
• Physics: In certain physical phenomena and equations, negative square roots arise, particularly in areas like quantum mechanics and electrical engineering.
• Engineering: Engineers often encounter negative square roots when dealing with signals, vibrations, and other dynamic systems that involve complex numbers.
• Computer Graphics: Negative square roots are utilized in computer graphics to manipulate images and render three-dimensional scenes, especially in calculations involving rotations and transformations.

Understanding negative square roots is essential for tackling advanced mathematical problems and applications across various disciplines.

In conclusion, exploring the negative square root of 16 unveils the fascinating realm of complex numbers and imaginary units. By understanding the properties of complex numbers and the role of the imaginary unit \( i \), we can navigate through mathematical challenges involving negative square roots.

From calculation and simplification steps to real-world applications in mathematics, the journey through negative square roots enriches our understanding of various mathematical concepts and their practical implications.

Embracing the complexities of negative square roots opens doors to deeper insights in mathematics, physics, engineering, and computer science, paving the way for innovative solutions and advancements in diverse fields.