Is the Square Root of 10 a Rational Number? Discover the Truth!

The square root of 10, denoted as √10, is a well-known mathematical concept. This article provides a detailed explanation of whether the square root of 10 is a rational number and the methods used to determine its value.

Definition of Rational and Irrational Numbers

A rational number is a number that can be expressed in the form of a fraction \( \frac{p}{q} \), where \( p \) and \( q \) are integers and \( q \) is not zero. Rational numbers include integers, finite decimals, and repeating decimals.

An irrational number, on the other hand, cannot be expressed as a simple fraction. Its decimal representation is non-terminating and non-repeating.

Is the Square Root of 10 Rational?

The square root of 10 is an irrational number. It cannot be expressed as a fraction of two integers. When we calculate the square root of 10, we get a non-repeating and non-terminating decimal:

\[
\sqrt{10} \approx 3.16227766017...
\]

Methods to Find the Square Root of 10

• Prime Factorization Method

  
  Decompose 10 into its prime factors: \( 10 = 2 \times 5 \). The square root can be expressed as:
  \[
  \sqrt{10} = \sqrt{2 \times 5} = \sqrt{2} \times \sqrt{5}
  \]
  However, neither \( \sqrt{2} \) nor \( \sqrt{5} \) are integers, reinforcing that √10 is irrational.

• Estimation Method

  
  Since 10 is between 9 (which is \( 3^2 \)) and 16 (which is \( 4^2 \)), √10 is between 3 and 4. A closer estimation can be refined using average techniques:
  \[
  3.162 \approx \left(\frac{3 + 3.33}{2}\right)
  \]

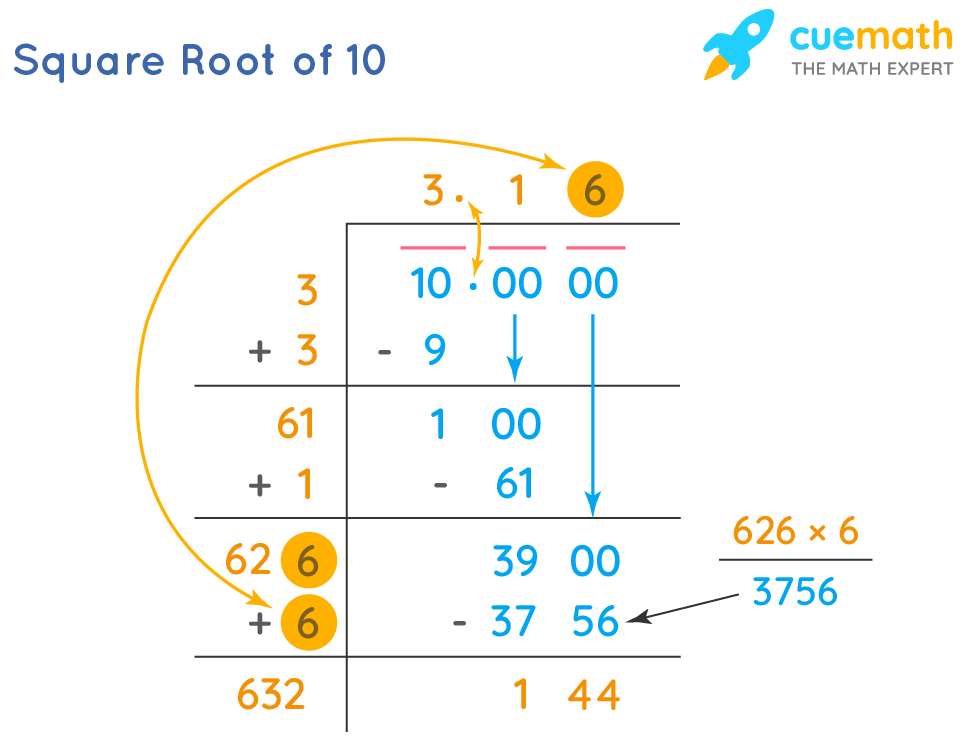
• Long Division Method

  
  This iterative process involves guessing, dividing, and averaging to find increasingly accurate approximations of √10. Starting with an initial guess (e.g., 3), the process is repeated until a satisfactory approximation is achieved.

• Using Calculators or Software

  
  Modern calculators and software can quickly compute the value of √10 to many decimal places, confirming its irrational nature.

Conclusion

The square root of 10 is an irrational number. Despite being an important mathematical concept, it cannot be precisely expressed as a fraction, making its decimal representation infinite and non-repeating.

Additional Resources

The square root of 10 is a fascinating mathematical concept. Understanding whether it is a rational or irrational number requires a deep dive into the properties of numbers and their classifications. In this section, we will explore the definition of rational and irrational numbers, and establish why the square root of 10 falls into one of these categories.

Rational numbers are numbers that can be expressed as the ratio of two integers, \( \frac{p}{q} \), where \( p \) and \( q \) are integers and \( q \neq 0 \). Examples include 1/2, 3, and 0.75. On the other hand, irrational numbers cannot be expressed as such a ratio. They include non-repeating, non-terminating decimals like π and \( \sqrt{2} \).

To determine the nature of \( \sqrt{10} \), consider its decimal form, which is approximately 3.16227766017. This decimal does not terminate or repeat, indicating that it cannot be expressed as a fraction of two integers. Therefore, \( \sqrt{10} \) is classified as an irrational number.

The methods to approximate the value of \( \sqrt{10} \) include the prime factorization method, estimation using nearby perfect squares, iterative methods like Newton-Raphson, and using calculators or mathematical software. These approaches highlight the complex nature of irrational numbers and the need for precise computation.

Understanding the classification and properties of numbers like \( \sqrt{10} \) enhances our comprehension of the mathematical world, providing a clear distinction between rational and irrational numbers and their significance in various mathematical applications.

Numbers can be broadly classified into two categories: rational and irrational. Understanding the differences between these two types of numbers is fundamental in mathematics.

Rational numbers are those that can be expressed as a fraction, where both the numerator and the denominator are integers, and the denominator is not zero. These numbers include integers, fractions, and terminating or repeating decimals.

Examples of rational numbers include:

• 3 (which can be written as 3/1)
• -2 (which can be written as -2/1)
• 1/2
• 0.75 (which can be written as 3/4)
• 0.333... (which can be written as 1/3)

Irrational numbers, on the other hand, cannot be expressed as a fraction. These numbers have non-terminating, non-repeating decimal expansions. Examples include the square roots of non-perfect squares, such as √2, √3, and √10, as well as famous constants like π (pi).

To determine whether the square root of a number is rational or irrational, consider the following steps:

1. Check if the number is a perfect square. If it is, the square root is rational. For example, √9 = 3, and 3 is a rational number.
2. If the number is not a perfect square, the square root will be irrational. For example, 10 is not a perfect square, so √10 is irrational.

Thus, the square root of 10, denoted as √10, is an irrational number because it cannot be expressed as a fraction and its decimal representation is non-terminating and non-repeating.

Understanding these concepts helps in distinguishing between different types of numbers and their properties, which is crucial for various mathematical applications and problem-solving.

Understanding the calculation of the square root of 10 involves recognizing its irrational nature. Below are detailed steps and examples to approximate the square root of 10:

• Prime Factorization Method
1. Decompose 10 into prime factors: \(2 \times 5\).
2. Find the square roots of each factor: \(\sqrt{2}\) and \(\sqrt{5}\).
3. Multiply the square roots: \(\sqrt{2} \times \sqrt{5} = \sqrt{10} \approx 3.162\).
• Estimation Method
1. Identify the nearest perfect squares: \( \sqrt{9} = 3\) and \(\sqrt{16} = 4\).
2. Estimate between these values: Since 10 is closer to 9, \(\sqrt{10} \approx 3.1\).
3. Refine the estimate: \(\sqrt{10} \approx 3.16\).
• Long Division Method
1. Pair the digits: Since 10 is a whole number, consider it as 10.
2. Initial guess: Start with 3 (since \(3^2 = 9\)).
3. Divide and average: \( \frac{10}{3} = 3.33\), average with 3: \(\frac{3 + 3.33}{2} \approx 3.165\).
4. Repeat until stable: Continue the process to get \(\sqrt{10} \approx 3.162\).

The square root of 10, denoted as \( \sqrt{10} \), is classified as an irrational number. This classification arises from its decimal representation, which is non-terminating and non-repeating. To understand why, we need to delve into the characteristics of rational and irrational numbers.

A rational number can be expressed as a fraction \( \frac{p}{q} \) where both \( p \) and \( q \) are integers and \( q \) is not zero. Rational numbers include integers, finite decimals, and repeating decimals.

Conversely, an irrational number cannot be expressed as a simple fraction. Its decimal representation neither terminates nor repeats, which is a key feature of irrational numbers.

Let’s consider \( \sqrt{10} \). When calculated, its value is approximately 3.16227766017... and continues indefinitely without repeating. This non-terminating and non-repeating nature confirms that \( \sqrt{10} \) cannot be written as \( \frac{p}{q} \), where \( p \) and \( q \) are integers. Therefore, it is an irrational number.

Mathematically, if \( \sqrt{10} \) were rational, we could write it as \( \frac{a}{b} \) in simplest form, where \( a \) and \( b \) have no common factors other than 1. Squaring both sides, we would get \( 10 = \frac{a^2}{b^2} \) implying \( 10b^2 = a^2 \). This equation suggests \( a^2 \) is a multiple of 10, so \( a \) must also be a multiple of 10, say \( a = 10k \). Substituting back, we get \( 10b^2 = (10k)^2 \), leading to \( b^2 = 10k^2 \). This implies \( b \) is also a multiple of 10, contradicting our assumption that \( \frac{a}{b} \) is in simplest form. Hence, \( \sqrt{10} \) cannot be rational.

In conclusion, the square root of 10 is an irrational number because it does not conform to the characteristics of rational numbers. Its infinite, non-repeating decimal expansion signifies its place among irrational numbers.

Here are some common questions and answers related to the square root of 10:

• Is the square root of 10 a rational number?

  No, the square root of 10 is an irrational number. It cannot be expressed as a simple fraction of two integers because its decimal representation is non-terminating and non-repeating.

• What is the approximate value of the square root of 10?

  The square root of 10 is approximately 3.162. This value can be determined using various methods such as estimation, long division, or a calculator.

• How can I find the square root of 10 using the long division method?

  The long division method involves dividing 10 by a series of approximations and averaging the results until a satisfactory level of precision is achieved. This iterative process provides a step-by-step approach to find the square root.

• Is the square root of 10 a real number?

  Yes, the square root of 10 is a real number. It lies between the integers 3 and 4 on the number line.

• What is the radical form of the square root of 10?

  The square root of 10 in radical form is expressed as √10.

• Can the square root of 10 be simplified?

  No, √10 cannot be simplified further because 10 is not a perfect square and does not have a square factor other than 1.