Topic half square root: The concept of the half square root often involves simplifying the square root of fractions like 1/2. Understanding how to calculate and rationalize these square roots is essential in various mathematical applications. This introduction provides a concise overview of simplifying square roots, their properties, and practical examples to enhance your mathematical skills.
Table of Content
Half Square Root
The concept of the "half square root" can be explained using properties of square roots in mathematics. Understanding these properties is essential in various mathematical computations and problem-solving.
Definition
The square root of a number \( x \) is a value \( y \) such that \( y^2 = x \). When dealing with square roots, we often come across the term "half-power," which is expressed as:
\[
\sqrt{x} = x^{1/2}
\]
This notation signifies that the square root of \( x \) is equivalent to raising \( x \) to the power of 1/2.
Simplifying Square Roots
To simplify a square root, look for perfect square factors within the number. For example:
- \(\sqrt{45} = \sqrt{9 \times 5} = \sqrt{9} \times \sqrt{5} = 3\sqrt{5}\)
- \(\sqrt{27} = \sqrt{9 \times 3} = \sqrt{9} \times \sqrt{3} = 3\sqrt{3}\)
- \(\sqrt{8} = \sqrt{4 \times 2} = \sqrt{4} \times \sqrt{2} = 2\sqrt{2}\)
These examples demonstrate how to break down a square root into more manageable parts.
Square Root of a Fraction
The square root of a fraction can be computed by taking the square root of the numerator and the denominator separately. For instance:
\[
\sqrt{\frac{1}{2}} = \frac{\sqrt{1}}{\sqrt{2}} = \frac{1}{\sqrt{2}}
\]
To rationalize the denominator, multiply by \(\sqrt{2}/\sqrt{2}\):
\[
\frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}
\]
Important Properties
Here are some essential properties of square roots:
- \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
- \((\sqrt{a})^2 = a\)
Applications
Square roots are widely used in geometry, algebra, and calculus. They are essential for solving quadratic equations, finding distances, and working with areas and volumes in geometric problems.
Online Calculators
Several online calculators can help with computing square roots and understanding their properties:

READ MORE:
Introduction to Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. For any non-negative real number \( a \), the principal square root is denoted by \( \sqrt{a} \), where \( \sqrt{} \) is the radical sign. For example, the principal square root of 9 is 3, denoted as \( \sqrt{9} = 3 \).
Square roots have important properties and applications in various fields, including algebra, geometry, and calculus. Understanding these properties is crucial for solving equations and simplifying expressions involving radicals.
The principal square root is always non-negative, but every positive number actually has two square roots: a positive and a negative one. These can be written as \( \pm \sqrt{x} \). For example, the square roots of 4 are \( \pm 2 \).
Here are some key points about square roots:
- Square roots can be simplified by factoring out perfect squares.
- Rationalizing the denominator is a common technique used to eliminate radicals from the denominator of a fraction.
- Square roots of fractions can be simplified using the property \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
In addition to their basic properties, square roots can be used to solve quadratic equations and model real-world phenomena. Mastery of square roots is a fundamental skill in mathematics.
Properties of Square Roots
Square roots have unique properties that make them fundamental in various mathematical operations and applications. Here are some key properties:
- Nonnegative Real Numbers: The square root of a nonnegative real number is also a nonnegative real number. For example, the principal square root of 16 is 4.
- Product Property: The square root of a product is the product of the square roots of the factors. This is expressed as:
- Quotient Property: The square root of a quotient is the quotient of the square roots of the numerator and the denominator. This is expressed as:
- Power of One-Half: A square root can be expressed as a number raised to the power of one-half:
- Simplification: A square root can be simplified if the radicand has a factor that is a perfect square. For example:
These properties are crucial for simplifying expressions involving square roots and for performing arithmetic operations on them.
Principal Square Root
The principal square root of a nonnegative real number \(a\) is the nonnegative number \(b\) such that \(b^2 = a\). This is denoted as \(\sqrt{a}\), where the symbol \(\sqrt{}\) is called the radical sign. The principal square root focuses on the positive root, disregarding the negative counterpart.
For example, the principal square root of 9 is 3, which we denote as:
\[\sqrt{9} = 3\]
The process of finding the principal square root involves several steps, particularly when dealing with non-perfect squares or more complex numbers. Here’s a detailed explanation:
- Identify whether the number is a perfect square.
- If the number is a perfect square, its square root is an integer. For example, \(\sqrt{16} = 4\) because \(4^2 = 16\).
- If the number is not a perfect square, proceed to the next steps to simplify.
- Factorize the number to identify any perfect square factors.
- For example, for \(\sqrt{18}\), we can factorize 18 into \(9 \times 2\).
- Apply the property of square roots: \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\).
- Using the example above, \(\sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2}\).
- Combine and simplify the expression to get the final result.
- The simplified form of \(\sqrt{18}\) is \(3\sqrt{2}\).
Understanding the principal square root is crucial in various mathematical contexts, from solving quadratic equations to applying it in geometry and calculus.
Here's a table summarizing some principal square roots for quick reference:
| Number | Principal Square Root |
|---|---|
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
| 36 | 6 |
When dealing with negative numbers, the principal square root does not apply within the real number system because there is no real number whose square is negative. Instead, the concept of imaginary numbers is used, where \(\sqrt{-1} = i\).
For example, the principal square root of -16 is represented as:
\[\sqrt{-16} = 4i\]
Understanding the principal square root and its properties helps in various fields, including algebra, engineering, and physics, making it a fundamental concept in mathematics.
Negative Square Roots
When dealing with square roots, a negative number under the radical sign presents unique challenges. Let's explore the concept of negative square roots and their implications in mathematics.
In general, the square root of a number \(x\) is a number \(y\) such that \(y^2 = x\). For positive numbers, this is straightforward, but for negative numbers, we encounter a problem:
\[
y^2 = -x
\]
where \(x > 0\). There is no real number \(y\) that can satisfy this equation because the product of two real numbers is always non-negative.
Therefore, the square root of a negative number does not exist within the set of Real Numbers. To address this issue, mathematicians introduced the concept of imaginary numbers. The imaginary unit is defined as:
\[
i = \sqrt{-1}
\]
Using this, we can express the square root of any negative number. For example:
\[
\sqrt{-x} = \sqrt{x} \cdot \sqrt{-1} = \sqrt{x} \cdot i
\]
where \(x\) is a positive real number.
Let's look at some examples to clarify this:
- \[ \sqrt{-4} = \sqrt{4} \cdot \sqrt{-1} = 2i \]
- \[ \sqrt{-9} = \sqrt{9} \cdot \sqrt{-1} = 3i \]
Imaginary numbers, when combined with real numbers, form Complex Numbers. A complex number is written in the form:
\[
a + bi
\]
where \(a\) and \(b\) are real numbers, and \(i\) is the imaginary unit.
Imaginary numbers play a crucial role in various fields such as electrical engineering, quantum mechanics, and applied mathematics. Their introduction helped fill a critical gap in mathematical theory and allowed for the solution of equations that were previously unsolvable within the real number system.
To summarize, negative square roots do not exist in the realm of real numbers, but with the invention of the imaginary unit \(i\), we can handle these values effectively in the complex number system.

Calculating Square Roots
Calculating square roots can be approached in various ways, from simple methods for perfect squares to more complex algorithms for non-perfect squares. Here, we will explore some common techniques:
1. Using Prime Factorization
This method works well for perfect squares. Follow these steps:
- Factorize the number into its prime factors.
- Pair the prime factors.
- Take one factor from each pair and multiply them together.
Example: To find the square root of 144:
- Prime factorization of 144: \(144 = 2^4 \times 3^2\)
- Pairing the factors: \((2^2) \times (2^2) \times (3^2)\)
- Taking one factor from each pair: \(2 \times 2 \times 3 = 12\)
Thus, \(\sqrt{144} = 12\).
2. Estimation and Approximation
For non-perfect squares, we can estimate and refine our answer:
- Find two consecutive perfect squares between which the number lies.
- Estimate the square root by averaging the two bounding square roots.
- Refine the estimate by comparing the square of the estimate with the original number and adjusting accordingly.
Example: To estimate \(\sqrt{10}\):
- 10 lies between 9 (\(3^2\)) and 16 (\(4^2\)), so \(\sqrt{10}\) is between 3 and 4.
- Estimate: \(3.5\). Check: \(3.5 \times 3.5 = 12.25\) (too high).
- Adjust estimate: \(3.2\). Check: \(3.2 \times 3.2 = 10.24\) (closer).
3. Using a Calculator
The easiest and most accurate method is to use a calculator:
- Enter the number and press the square root button (√).
- The calculator will display the square root, often to many decimal places.
Example: Using a calculator to find \(\sqrt{10}\), we get approximately 3.1623.
4. Newton's Method (Iterative Approach)
This is an iterative method that improves the estimate with each iteration:
- Start with an initial guess \(x_0\).
- Use the formula \(x_{n+1} = \frac{1}{2} \left( x_n + \frac{S}{x_n} \right)\), where \(S\) is the number.
- Repeat until the desired accuracy is achieved.
Example: To find \(\sqrt{10}\) with an initial guess \(x_0 = 3\):
- First iteration: \(x_1 = \frac{1}{2} \left( 3 + \frac{10}{3} \right) = 3.1667\)
- Second iteration: \(x_2 = \frac{1}{2} \left( 3.1667 + \frac{10}{3.1667} \right) \approx 3.1623\)
Continuing this process will yield more accurate results.
5. Special Cases
For perfect squares, such as 16, 25, or 36, the square roots are integers: 4, 5, and 6, respectively. For negative numbers, the square root involves imaginary numbers (i.e., \(\sqrt{-1} = i\)).
Using these methods, you can calculate square roots for a variety of numbers, from simple integers to complex decimals.
Square Roots in History
The concept of square roots has a rich history that spans many cultures and centuries. Understanding its historical development helps appreciate its significance in mathematics today.
Square roots were known to ancient civilizations. The earliest records date back to the Babylonians around 2000 BCE, who approximated the square root of 2. This shows their advanced understanding of mathematics, as they used this knowledge for architectural and astronomical calculations.
In ancient Greece, mathematicians such as Pythagoras and Euclid furthered the study of square roots. Euclid's work "Elements" laid the foundation for modern geometry, including methods for finding square roots of numbers through geometric constructions.
The term "square root" comes from the geometrical concept of the area of a square. If a square has an area of \( A \), the side length of the square is \( \sqrt{A} \). The Latin word "radix," meaning root, was used in medieval translations of Arabic mathematical texts, and this term stuck in modern usage.
In the 9th century, the Persian mathematician Al-Khwarizmi wrote a book titled "Al-Kitab al-Mukhtasar fi Hisab al-Jabr wal-Muqabala," which translates to "The Compendious Book on Calculation by Completion and Balancing." This book introduced algebra and included methods for solving quadratic equations, indirectly dealing with square roots.
The Bakhshali Manuscript, an ancient Indian mathematical text, contains the earliest known use of a symbol for zero and sophisticated methods for arithmetic operations, including square roots. This manuscript, dating back to between the 3rd and 7th centuries, demonstrates the advanced level of mathematical understanding in ancient India.
During the Renaissance, European mathematicians such as Leonardo of Pisa (Fibonacci) reintroduced the knowledge of square roots to Europe through translations of Arabic works. The development of symbolic algebra by mathematicians like François Viète and later René Descartes formalized the use of symbols for operations, including square roots, in equations.
In summary, the concept of square roots has evolved through contributions from various cultures, including Babylonian, Greek, Indian, Arabic, and European scholars. Each civilization built upon the knowledge of their predecessors, leading to the comprehensive understanding of square roots we have today.
Practical Applications of Square Roots
Square roots are utilized in various practical applications across different fields. Here are some key examples:
-
Geometry and Construction:
Square roots help in calculating the dimensions of geometric shapes. For instance, to find the side length of a square given its area, you use the square root of the area. If the area \(A\) is 200 square feet, the side length is \(\sqrt{200} \approx 14.1\) feet.
-
Physics and Engineering:
In physics, the square root function is used to determine time, speed, and other dynamic quantities. For example, the time \(t\) it takes for an object to fall from height \(h\) can be calculated using the formula \(t = \frac{\sqrt{h}}{4}\). If an object is dropped from 64 feet, it takes \(t = \frac{\sqrt{64}}{4} = 2\) seconds to hit the ground.
-
Distance Calculation:
The distance \(D\) between two points in a plane with coordinates \((x_1, y_1)\) and \((x_2, y_2)\) is found using the distance formula \(D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\). This is derived from the Pythagorean theorem.
-
Accident Investigations:
Police officers use square roots to determine the speed of vehicles involved in accidents. The speed \(s\) can be found using the length of skid marks \(d\) with the formula \(s = \sqrt{24d}\). If skid marks are 190 feet long, the speed is approximately \( \sqrt{24 \times 190} \approx 67.5 \) mph.
-
Finance:
In finance, square roots are used to calculate standard deviations, which measure the volatility or risk of an investment. The formula involves taking the square root of the variance of asset returns.
These applications illustrate the importance of square roots in simplifying complex problems and providing accurate results in various practical scenarios.
Square Roots and Complex Numbers
Square roots of complex numbers are crucial in various mathematical and engineering applications. Calculating the square root of a complex number can be approached using both rectangular and polar coordinate systems.
Rectangular Coordinates
For a complex number \( z = a + ib \), the square root can be found using the formula:
\[
\sqrt{a + ib} = \pm \left( \sqrt{\frac{|z| + a}{2}} + i \cdot \text{sgn}(b) \sqrt{\frac{|z| - a}{2}} \right)
\]
Where \( |z| = \sqrt{a^2 + b^2} \) is the magnitude of \( z \), and \( \text{sgn}(b) \) is the sign of \( b \).
- If \( b < 0 \), then \( \text{sgn}(b) = -1 \), and \( x \) and \( y \) have opposite signs.
- If \( b > 0 \), then \( \text{sgn}(b) = 1 \), and \( x \) and \( y \) have the same signs.
Example Calculation
To find the square root of \( 3 + 4i \):
- Calculate the magnitude: \( |3 + 4i| = \sqrt{3^2 + 4^2} = 5 \).
- Use the formula: \[ \sqrt{3 + 4i} = \pm \left( \sqrt{\frac{5 + 3}{2}} + i \cdot \sqrt{\frac{5 - 3}{2}} \right) \]
- Simplify: \[ \sqrt{3 + 4i} = \pm \left( \sqrt{4} + i \cdot \sqrt{1} \right) = \pm (2 + i) \]
Hence, the square roots are \( 2 + i \) and \( -2 - i \).
Polar Coordinates
Using polar coordinates, a complex number \( z = re^{i\theta} \) has a square root given by:
\[
\sqrt{z} = \sqrt{r} \left( \cos \frac{\theta}{2} + i \sin \frac{\theta}{2} \right)
\]
Where \( r \) is the magnitude and \( \theta \) is the argument (angle) of the complex number.
Example Calculation
To find the square root of \( z = 2[\cos(\pi/4) + i \sin(\pi/4)] \):
- Identify \( r = 2 \) and \( \theta = \pi/4 \).
- Use the formula: \[ \sqrt{z} = \sqrt{2} \left( \cos \frac{\pi/8} + i \sin \frac{\pi/8} \right) \]
- The roots are: \[ z_1 = \sqrt{2} \left( \cos \frac{\pi/8} + i \sin \frac{\pi/8} \right) \] and \[ z_2 = \sqrt{2} \left( \cos \frac{9\pi/8} + i \sin \frac{9\pi/8} \right) \]
These methods provide a systematic approach to finding the square roots of complex numbers, which can be applied to various practical and theoretical problems.

Advanced Topics in Square Roots
Square roots are a fundamental concept in mathematics with various advanced applications and properties. This section delves into some of these advanced topics to provide a deeper understanding of square roots.
Square Roots of Negative Numbers
In the real number system, the square root of a negative number is not defined. However, in the complex number system, we use the imaginary unit i, where i is defined as the square root of -1. Therefore, for any negative number -a, the square root can be expressed as:
\(\sqrt{-a} = \sqrt{a} \cdot i\)
For example, \(\sqrt{-4} = \sqrt{4} \cdot i = 2i\).
Rational and Irrational Square Roots
Square roots can be either rational or irrational. A rational square root is a square root that can be expressed as a fraction, while an irrational square root cannot be expressed as a simple fraction.
- Rational example: \(\sqrt{4} = 2\)
- Irrational example: \(\sqrt{2} \approx 1.414213\ldots\)
Properties of Square Roots
- Product Property: \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\), provided \(a \geq 0\) and \(b \geq 0\).
- Quotient Property: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\), provided \(a \geq 0\) and \(b > 0\).
- Power Property: \(\left(\sqrt{a}\right)^2 = a\).
Square Roots in Higher Dimensions
In advanced mathematics, the concept of square roots extends beyond real and complex numbers. For example, in linear algebra, the square root of a matrix is a matrix that, when multiplied by itself, yields the original matrix. Not all matrices have square roots, and those that do may have more than one.
If \(A\) is a square matrix, a matrix \(B\) is called a square root of \(A\) if \(B^2 = A\).
Applications in Differential Equations
Square roots frequently appear in solutions to differential equations. For instance, the general solution to the second-order linear differential equation:
\(\frac{d^2y}{dx^2} + ay = 0\)
depends on the square root of the parameter \(a\). The characteristic equation for this differential equation is:
\(r^2 + a = 0 \implies r = \pm \sqrt{-a} = \pm \sqrt{a} \cdot i\)
This leads to solutions involving exponential functions and trigonometric functions of complex arguments.
Computational Methods
Several algorithms exist for calculating square roots, including:
- Newton's Method (or Heron's Method): An iterative method to approximate square roots.
- Bisection Method: A bracketing method that repeatedly bisects an interval and then selects a subinterval in which a root exists.
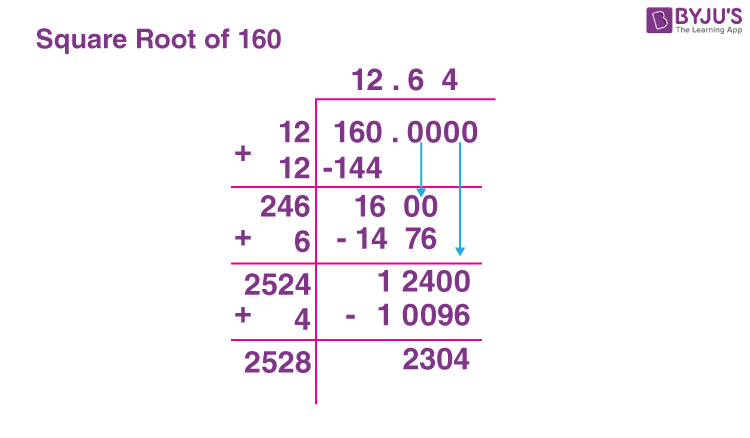
- Digit-by-Digit Calculation: A manual method that is analogous to long division.
These methods are implemented in various computer algorithms to efficiently calculate square roots to a high degree of precision.
Exploring these advanced topics provides a greater appreciation for the depth and utility of square roots in mathematics.
Tại sao "x mũ một phần hai" có nghĩa là căn bậc hai?
READ MORE:
Tại sao Căn Bậc Hai là Mũ Một Phần Hai