Topic what is the square root of 161: Understanding the square root of 161 can enhance your mathematical knowledge and problem-solving skills. In this article, we will delve into the calculation, properties, and applications of the square root of 161, providing you with a comprehensive and engaging exploration of this fascinating mathematical concept.
Table of Content
- Square Root of 161
- Introduction to Square Roots
- What is the Square Root of 161?
- Calculating the Square Root of 161
- Methods for Finding Square Roots
- Square Root Calculation Using a Calculator
- Manual Calculation of Square Roots
- Square Root Approximation Techniques
- Properties of Square Roots
- Applications of Square Roots in Real Life
- Frequently Asked Questions About Square Roots
- Conclusion
- YOUTUBE: Khám phá căn bậc hai của 161 và cách tính toán chi tiết. Video này sẽ hướng dẫn bạn hiểu rõ hơn về khái niệm và ứng dụng của căn bậc hai.
Square Root of 161
The square root of 161 is approximately 12.6885775404. This value is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating.
Mathematical Explanation
The square root of a number \( x \) is a value that, when multiplied by itself, equals \( x \). For 161, this can be represented as:
\[ \sqrt{161} = 12.6885775404 \]
Methods to Calculate the Square Root
- Prime Factorization: This method is ideal for perfect squares, but since 161 is not a perfect square, it is less effective here.
- Long Division: This is a more suitable method for finding the square root of 161 as it provides a decimal result.
Square Root Table
Below is a table showing the nth roots of 161:
| Index (n) | Root Symbol | Value |
|---|---|---|
| 2 | \(\sqrt{161}\) | 12.6885775404 |
| 3 | \(\sqrt[3]{161}\) | 5.4401218254 |
| 4 | \(\sqrt[4]{161}\) | 3.562102966 |
| 5 | \(\sqrt[5]{161}\) | 2.762900056 |
| 6 | \(\sqrt[6]{161}\) | 2.3324068739 |
| 7 | \(\sqrt[7]{161}\) | 2.066621006 |
| 8 | \(\sqrt[8]{161}\) | 1.887353429 |
| 9 | \(\sqrt[9]{161}\) | 1.7587449973 |
| 10 | \(\sqrt[10]{161}\) | 1.6621973577 |
Additional Information
For more details on the square root of 161 and other related mathematical concepts, you can explore additional resources and calculators online to deepen your understanding.

READ MORE:
Introduction to Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. It is an essential concept in mathematics, frequently used in various fields such as engineering, physics, and finance. Square roots help in simplifying equations and solving quadratic equations.
For instance, the square root of 9 is 3, because \(3 \times 3 = 9\). This can be represented as:
\(\sqrt{9} = 3\)
Similarly, the square root of 161 is the number that, when multiplied by itself, equals 161.
Square roots are denoted by the radical symbol \(\sqrt{}\). The number under the radical is called the radicand. For example, in \(\sqrt{161}\), 161 is the radicand.
There are several properties of square roots that are useful to know:
- Non-negative Output: The principal square root (the most common square root) is always non-negative.
- Perfect Squares: If a number is a perfect square, its square root will be an integer.
- Product Property: The square root of a product is equal to the product of the square roots of each factor: \(\sqrt{ab} = \sqrt{a} \times \sqrt{b}\).
- Quotient Property: The square root of a quotient is equal to the quotient of the square roots: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\).
Understanding square roots is fundamental in mathematics, as it provides a basis for more advanced topics such as algebra, calculus, and beyond. The square root function can be extended to complex numbers, where it becomes even more powerful and intriguing.
What is the Square Root of 161?
The square root of 161, represented as √161, is a value which, when multiplied by itself, equals 161. The square root of 161 is an irrational number, meaning it cannot be expressed exactly as a simple fraction. The approximate value of the square root of 161 is:
√161 ≈ 12.6885775404
This value can be used in various calculations and can be expressed in different forms:
- Exact Form: √161
- Decimal Form: 12.6885775404
For practical purposes, the square root of 161 can be rounded to different decimal places:
- To the nearest tenth: 12.7
- To the nearest hundredth: 12.69
- To the nearest thousandth: 12.689
The square root of 161 can also be expressed using exponents:
√161 = 1611/2
Since 161 is not a perfect square (a number whose square root is an integer), its square root is irrational. This means the decimal representation is non-terminating and non-repeating.
If you need to find the square root of 161 using a calculator, simply input 161 and press the square root (√) button to get the result. This can also be done using spreadsheet software like Excel by entering the formula =SQRT(161).
In summary, the square root of 161 is an important mathematical constant that is used in various applications, from simple arithmetic to more complex calculations in geometry and algebra.
Calculating the Square Root of 161
The square root of 161 is a value which, when multiplied by itself, gives the number 161. This value is not a whole number, and therefore, 161 is not a perfect square. The square root of 161 can be expressed in several ways and calculated using different methods. Below, we detail these methods step-by-step.
Using a Calculator
The most straightforward method to find the square root of 161 is to use a calculator. On most calculators, you can simply enter 161 and press the √ (square root) button to get the result:
Using Long Division Method
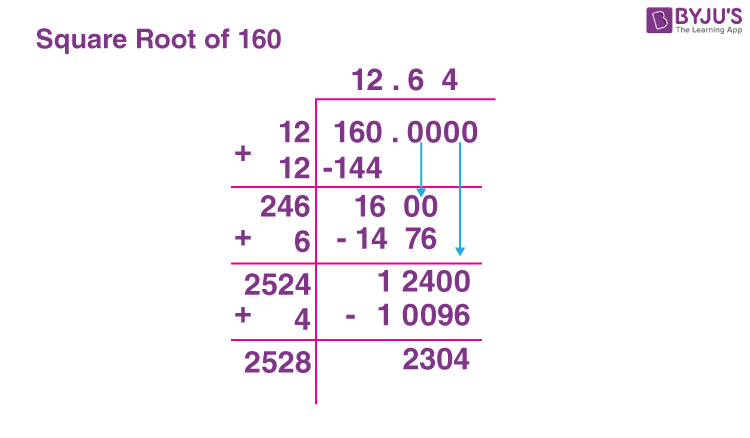
The long division method is a manual technique to calculate square roots. Here’s how you can do it:
- Set up 161 in pairs of digits from right to left and add a decimal point and pairs of zeros. For 161, you write it as 1 61.00 00.
- Find the largest square less than or equal to the first set (1). In this case, 1 is the largest perfect square, so we place 1 on top and subtract it from 1, leaving 0.
- Bring down the next pair of digits (61), making it 61.
- Double the number on top (which is 1) to get 2. Find a digit (X) such that 2X times X is less than or equal to 61. The digit is 2 because 22*2 = 44.
- Subtract 44 from 61, giving 17. Bring down the next pair of zeros to make it 1700.
- Double the number on top (12) to get 24. Find a digit (Y) such that 24Y times Y is less than or equal to 1700. The digit is 7 because 247*7 = 1729.
- Repeat the process as needed for more decimal places.
The result is approximately 12.6886.
Approximating the Square Root
Another method is to approximate the square root by identifying two perfect squares between which 161 lies. These are 144 (12²) and 169 (13²).
- The whole number part of the square root of 161 is between 12 and 13.
- Use the formula for approximation:
\[ \text{Square root} \approx \text{Lower bound} + \frac{\text{Difference between the number and the lower perfect square}}{\text{Difference between the upper and lower perfect squares}} \] \[ \sqrt{161} \approx 12 + \frac{161 - 144}{169 - 144} \] \[ \sqrt{161} \approx 12 + \frac{17}{25} \approx 12.68 \]
Properties of the Square Root of 161
- The square root of 161 is an irrational number, meaning it cannot be expressed as a simple fraction.
- The approximate value of the square root of 161 is 12.6886.
- The square root of 161 can be expressed using exponents: \(161^{1/2}\).
By understanding these methods, you can calculate or approximate the square root of 161 accurately.
Methods for Finding Square Roots
Finding the square root of a number can be approached using various methods. Here, we outline some of the most common techniques:
-
Prime Factorization Method:
- Identify the prime factors of the given number.
- Form factor pairs such that each pair’s two factors are equal.
- Select one number from each pair.
- Multiply the selected numbers to find the square root.
For example, to find the square root of 324:
- Prime factors of 324: \( 2 \times 2 \times 3 \times 3 \times 3 \times 3 \)
- Form pairs: \( (2 \times 2) \times (3 \times 3) \times (3 \times 3) \)
- Select one number from each pair: \( 2 \times 3 \times 3 \)
- Multiply: \( 2 \times 3 \times 3 = 18 \)
Thus, \( \sqrt{324} = 18 \).
-
Repeated Subtraction Method:This method involves subtracting consecutive odd numbers from the original number until the result is zero. The number of subtractions performed is the square root of the number.
For example, to find the square root of 36:
- 36 - 1 = 35
- 35 - 3 = 32
- 32 - 5 = 27
- 27 - 7 = 20
- 20 - 9 = 11
- 11 - 11 = 0
Thus, \( \sqrt{36} = 6 \).
-
Long Division Method:
- Divide the number into pairs of digits starting from the decimal point.
- Find the largest number whose square is less than or equal to the first pair.
- Subtract and bring down the next pair of digits.
- Double the quotient and find the next digit of the quotient.
- Continue until all pairs have been processed.
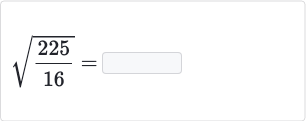
For example, to find the square root of 7225:
- Pairs: 72 and 25
- Find the largest square less than or equal to 72: \( 8^2 = 64 \)
- Subtract and bring down the next pair: \( 7225 - 6400 = 825 \)
- Double the quotient (8) and find the next digit: \( 16_5 \) fits into 825, \( 5 \times 165 = 825 \)
- The quotient is 85, so \( \sqrt{7225} = 85 \).
-
Estimation and Approximation:This method involves approximating the square root by finding the nearest perfect squares and interpolating between them.
For example, to find \( \sqrt{20} \):
- Find the nearest perfect squares: \( 16 \) and \( 25 \)
- \( \sqrt{16} = 4 \) and \( \sqrt{25} = 5 \)
- Since 20 is closer to 16, start with \( 4.5 \) and refine the estimate to \( 4.47 \).
Thus, \( \sqrt{20} \approx 4.47 \).
These methods provide different ways to calculate or approximate the square root of a number, depending on the context and the precision required.

Square Root Calculation Using a Calculator
Calculating the square root of 161 using a calculator is straightforward and can be done in a few simple steps. Here’s how you can do it:
- Turn on your calculator: Ensure that your calculator is in good working condition and has power.
- Locate the square root function: The square root function is usually represented by the symbol
√. On most scientific calculators, this function can be found on the keypad or as a secondary function (accessed by pressing theShiftor2ndkey). - Input the number: Enter
161into the calculator. Make sure you input the number correctly. - Apply the square root function: Press the
√button. If it’s a secondary function, press theShiftor2ndkey first, then press the button with the√symbol. - Read the result: The calculator will display the square root of 161. The result should be approximately
12.6886.
Here is a summary of the steps in tabular form:
| Step | Action |
|---|---|
| 1 | Turn on the calculator |
| 2 | Locate the square root function (√) |
| 3 | Enter the number 161 |
| 4 | Press the square root button (√) |
| 5 | Read the result: approximately 12.6886 |
Using these steps, you can easily find the square root of any number using a calculator.
Manual Calculation of Square Roots
Calculating the square root of a number manually can be done using a method similar to long division. Here's a step-by-step guide on how to manually calculate the square root of 161:
- Separate the Digits into Pairs:
Start by grouping the digits of the number from right to left into pairs. For 161, this would be written as 1 61.
- Find the Largest Integer:
Find the largest integer whose square is less than or equal to the leftmost number. For 1, this is 1, since 12 = 1.
- Subtract and Bring Down the Next Pair:
Subtract the square of this integer (12 = 1) from the leftmost number (1), resulting in 0. Bring down the next pair of digits (61), making the new number 61.
- Double the Current Root:
Double the integer found in step 2 (1), giving 2. Write this as the first part of the new divisor, which is now 2_.
- Find the Next Digit:
Find the largest digit (x) to fill in the blank so that (2x) * x is less than or equal to 61. In this case, 23 * 3 = 69 is too large, but 22 * 2 = 44 fits. So, 2 is the next digit of the root.
- Subtract and Repeat:
Subtract 44 from 61 to get 17. Bring down pairs of zeros to continue the process for decimal places, if needed. Repeat steps 4-6 for each pair of digits.
Continuing this process will refine the accuracy of the square root. For 161, the square root is approximately 12.688.
Square Root Approximation Techniques
Approximating square roots can be done using various methods, each with different levels of precision and complexity. Below are some common techniques for approximating the square root of 161:
1. Long Division Method
This is a manual method that provides a way to find the square root of any number, especially useful for numbers that are not perfect squares.
- Pair the digits of the number starting from the decimal point. For 161, we pair as (1, 61).
- Find the largest number whose square is less than or equal to the first pair. Here, it is 1, because 12 = 1.
- Subtract this square from the first pair and bring down the next pair of digits. This gives us 61.
- Double the quotient obtained so far (1) and place it next to the remainder as 2_. Now, find a digit to replace the underscore (_) that, when multiplied by the new number, is less than or equal to 61. The digit here is 6 because 26 * 6 = 156.
- Continue this process to find the decimal places. This method gives us an approximation: √161 ≈ 12.688.
2. Babylonian Method (Heron's Method)
This iterative method is also known as Heron's method. It is very efficient for manual calculations.
- Start with a guess value, say \( x_0 \). For 161, let \( x_0 = 12 \).
- Compute a new guess using the formula: \[ x_{n+1} = \frac{1}{2} \left( x_n + \frac{161}{x_n} \right) \]
- Repeat the process until the difference between successive values is very small. For example: \[ x_1 = \frac{1}{2} \left( 12 + \frac{161}{12} \right) \approx 12.67 \] \[ x_2 = \frac{1}{2} \left( 12.67 + \frac{161}{12.67} \right) \approx 12.688 \]
- After a few iterations, the value stabilizes to √161 ≈ 12.688.
3. Using Continued Fractions
Continued fractions can provide a very accurate approximation for irrational numbers.
The square root of 161 can be represented as a continued fraction:
\[
\sqrt{161} = [12; \overline{1, 1, 24}]
\]
This means:
\[
\sqrt{161} = 12 + \frac{1}{1 + \frac{1}{1 + \frac{1}{24 + \cdots}}}
\]
4. Newton-Raphson Method
This is another iterative method similar to the Babylonian method but more generalized. It uses the function:
\[
f(x) = x^2 - 161
\]
and its derivative:
\[
f'(x) = 2x
\]
The iterative formula is:
\[
x_{n+1} = x_n - \frac{f(x_n)}{f'(x_n)} = x_n - \frac{x_n^2 - 161}{2x_n}
\]
Starting with \( x_0 = 12 \):
\[
x_1 = 12 - \frac{12^2 - 161}{2 \cdot 12} \approx 12.67
\]
\[
x_2 = 12.67 - \frac{12.67^2 - 161}{2 \cdot 12.67} \approx 12.688
\]
5. Estimation Using Perfect Squares
Since 144 (122) and 169 (132) are close to 161, we know that √161 is between 12 and 13. Using finer approximations, we can narrow it down to √161 ≈ 12.688.
Each of these methods provides a way to approximate the square root of 161 with varying levels of precision and complexity, allowing for flexibility based on the tools and time available.
Properties of Square Roots
The square root of a number has several important properties that are useful in various mathematical contexts. Here are some key properties of square roots:
- Non-negativity: The square root of a non-negative number is also non-negative. For any non-negative number \( x \), \( \sqrt{x} \geq 0 \).
- Product Property: The square root of a product is equal to the product of the square roots of the factors. For any non-negative numbers \( a \) and \( b \), \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \).
- Quotient Property: The square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator. For any non-negative numbers \( a \) and \( b \) where \( b \neq 0 \), \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
- Power Property: The square root of a number raised to a power is the same as the number raised to half of that power. For any non-negative number \( x \) and any real number \( n \), \( (\sqrt{x})^n = x^{\frac{n}{2}} \).
- Square Property: The square of the square root of a number returns the original number. For any non-negative number \( x \), \( (\sqrt{x})^2 = x \).
- Additive Property: The square root of a sum of squares is not necessarily equal to the sum of the square roots. For example, \( \sqrt{a^2 + b^2} \neq \sqrt{a^2} + \sqrt{b^2} \).
To further understand these properties, consider the square root of 161:
- Non-negativity: Since 161 is a positive number, its square root is also positive, i.e., \( \sqrt{161} \approx 12.688 \).
- Product Property: \( \sqrt{161} = \sqrt{7 \cdot 23} = \sqrt{7} \cdot \sqrt{23} \).
- Quotient Property: For example, \( \sqrt{\frac{161}{23}} = \frac{\sqrt{161}}{\sqrt{23}} \).
- Power Property: \( (\sqrt{161})^2 = 161 \).
These properties are foundational in algebra and are widely used in solving equations and understanding the behavior of functions involving square roots.

Applications of Square Roots in Real Life
Square roots are fundamental in various fields, demonstrating their versatility and importance in everyday life and advanced sciences. Here are some key applications of square roots:
- Geometry and Construction: Square roots are used to calculate distances, areas, and volumes. For example, in the Pythagorean theorem, the square root function helps find the length of a side in a right triangle when the lengths of the other two sides are known.
- Physics and Engineering: Many physical laws and engineering formulas involve square roots. For instance, in kinematics, the displacement of an object can be calculated using the square root when dealing with uniformly accelerated motion.
- Probability and Statistics: In statistics, the standard deviation is computed using the square root of the variance. This helps in understanding the dispersion of a dataset.
- Finance: Square roots are used in calculating various financial metrics, such as the volatility of stock prices, which is derived from the standard deviation of returns.
- Astronomy: Astronomers use square roots to determine distances between celestial bodies. For example, the inverse-square law, which describes the intensity of gravitational or electromagnetic forces, involves square root calculations.
- Technology: In digital signal processing, algorithms often require the computation of square roots to normalize signals or compute magnitudes of vectors.
Understanding and applying square roots is crucial in these fields, enabling precise calculations and fostering advancements in technology and science.
Frequently Asked Questions About Square Roots
Square roots are a fundamental concept in mathematics, and they often raise several questions. Below are some frequently asked questions about square roots, along with detailed answers.
-
What is a square root?
A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 161 is a value \( x \) such that \( x \times x = 161 \). The principal square root of 161 is approximately 12.6886.
-
Is 161 a perfect square?
No, 161 is not a perfect square because its square root (approximately 12.6886) is not an integer. Perfect squares are numbers like 1, 4, 9, 16, etc., whose square roots are whole numbers.
-
Is the square root of 161 rational or irrational?
The square root of 161 is an irrational number. This is because it cannot be expressed as a fraction of two integers, and its decimal form is non-terminating and non-repeating.
-
How can you calculate the square root of 161 manually?
There are several methods to calculate the square root of a number manually, such as the long division method and approximation techniques. For the square root of 161, you can use long division or iterative methods to approximate its value.
-
What are the properties of square roots?
- Non-negativity: The principal square root of a non-negative number is also non-negative.
- Product Property: The square root of a product is the product of the square roots: \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \).
- Quotient Property: The square root of a quotient is the quotient of the square roots: \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
- Power of Two: The square root of a number squared is the absolute value of the number: \( \sqrt{x^2} = |x| \).
-
Can the square root of 161 be simplified?
No, the square root of 161 cannot be simplified further because 161 does not have any square factors other than 1.
Understanding these fundamental aspects of square roots can help in various mathematical applications and problem-solving scenarios.
Conclusion
Understanding the square root of 161 offers valuable insights into various mathematical concepts and practical applications. The square root of 161 is approximately 12.6886, a non-terminating decimal which makes it an irrational number. This value is not a perfect square, indicating its complexity in both calculation and application.
Throughout this exploration, we have delved into the properties of square roots, their methods of calculation, and their significant applications in real life, such as in geometry, physics, and various fields requiring precise measurements. Whether using calculators, manual methods, or approximation techniques, knowing how to find and utilize square roots enhances our problem-solving abilities and mathematical literacy.
By recognizing the importance of square roots, we appreciate their role in everyday life and advanced mathematical theories. This knowledge not only aids in academic pursuits but also equips us with tools to tackle real-world problems efficiently.
In conclusion, the study of the square root of 161, and square roots in general, underscores the beauty and utility of mathematics in both theoretical and practical realms. Embracing these concepts can lead to greater appreciation and proficiency in various aspects of mathematics and its applications.
Khám phá căn bậc hai của 161 và cách tính toán chi tiết. Video này sẽ hướng dẫn bạn hiểu rõ hơn về khái niệm và ứng dụng của căn bậc hai.
Căn Bậc Hai Của 161
READ MORE:
Video giải thích về cách tìm căn bậc hai của số 161 một cách chi tiết và dễ hiểu. Thích hợp cho học sinh và những người yêu thích toán học.
Căn bậc hai của 161