Square Root of 7 Times Square Root of 7: Simplified Explanation

The expression √7 × √7 can be simplified using basic properties of square roots and exponents. Here's a detailed explanation of the concept:

Mathematical Expression

The product of the square root of 7 and itself can be written and simplified as:

\[
\sqrt{7} \times \sqrt{7} = \sqrt{7 \times 7} = \sqrt{49} = 7
\]

This shows that the square root of a number multiplied by itself gives the original number.

Steps to Simplify

1. Combine the radicals: \( \sqrt{7} \times \sqrt{7} = \sqrt{7 \times 7} \)
2. Simplify inside the radical: \( \sqrt{7 \times 7} = \sqrt{49} \)
3. Calculate the square root: \( \sqrt{49} = 7 \)

Practical Examples

• When dealing with areas: If a square has a side length of \( \sqrt{7} \), its area is \( (\sqrt{7})^2 = 7 \) square units.
• In geometry: The length of the diagonal of a square with side length \( \sqrt{7} \) is \( 7 \).

Additional Resources

For more detailed explanations and examples, you can explore the following resources:

The square root of 7 times the square root of 7 might seem like a complex mathematical expression, but it's actually quite simple when broken down. In mathematical terms, the square root of a number is a value that, when multiplied by itself, gives the original number. Therefore, the square root of 7 times the square root of 7 equals 7.

To understand this better, consider the properties of square roots and how they can be simplified. The expression √7 * √7 can be rewritten using the property of radicals: √a * √a = a. Applying this property, we get √7 * √7 = 7.

This concept can be illustrated through various methods including the product rule for radicals, using exponents, and through practical examples. Let's delve deeper into these methods to gain a comprehensive understanding.

The concept of square roots is fundamental in mathematics, often used to simplify expressions and solve equations. The square root of a number \( n \) is a value that, when multiplied by itself, equals \( n \). The square root of 7, denoted as \( \sqrt{7} \), is approximately 2.6457513110645906.

Mathematically, this can be expressed as:

\[
\sqrt{7} \times \sqrt{7} = 7
\]

This principle follows the basic property of square roots where \( \sqrt{a} \times \sqrt{a} = a \). Applying this to our example:

\[
\sqrt{7} \times \sqrt{7} = 7
\]

Calculating square roots involves different methods, such as the prime factorization, the approximation method, or the long division method. The square root of 7 is an irrational number, which means it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating.

Here is a step-by-step approach to understand the calculation:

• Prime Factorization: Since 7 is a prime number, it cannot be factorized further. Therefore, its square root is a non-reducible surd.
• Approximation Method: By identifying that 7 is between the perfect squares of 4 (2^2) and 9 (3^2), we can approximate that \( \sqrt{7} \) is between 2 and 3. Further approximation gives us a more precise value of around 2.645.
• Long Division Method: This method provides a systematic approach to finding the square root to several decimal places by repeatedly dividing and averaging.

Understanding the concept of square roots is crucial as it extends to various mathematical applications, including solving quadratic equations, working with exponents, and more complex mathematical functions.

The mathematical properties of the square root of 7 can be explored through various mathematical operations and concepts. Here are the key properties and their explanations:

• Basic Definition:

  The square root of 7, denoted as \( \sqrt{7} \), is a number which, when multiplied by itself, equals 7. This can be written as:

  \[ \sqrt{7} \times \sqrt{7} = 7 \]

• Approximate Value:

  The value of \( \sqrt{7} \) is approximately 2.64575131106. This value is non-repeating and non-terminating, indicating that \( \sqrt{7} \) is an irrational number.

• Positive and Negative Roots:

  Both \( \sqrt{7} \) and \( -\sqrt{7} \) are square roots of 7. This can be shown as:

  \[ \sqrt{7} \times \sqrt{7} = 7 \quad \text{and} \quad (-\sqrt{7}) \times (-\sqrt{7}) = 7 \]

• Radical and Exponential Forms:

  The square root of 7 can be expressed in radical form as \( \sqrt{7} \) and in exponential form as \( 7^{1/2} \).

• Use in Equations:

  The square root of 7 is often used in various mathematical equations and problems. For example, solving for \( x \) in the equation \( x^2 = 7 \) involves finding \( \sqrt{7} \).

  \[ x = \pm \sqrt{7} \]

• Long Division Method:

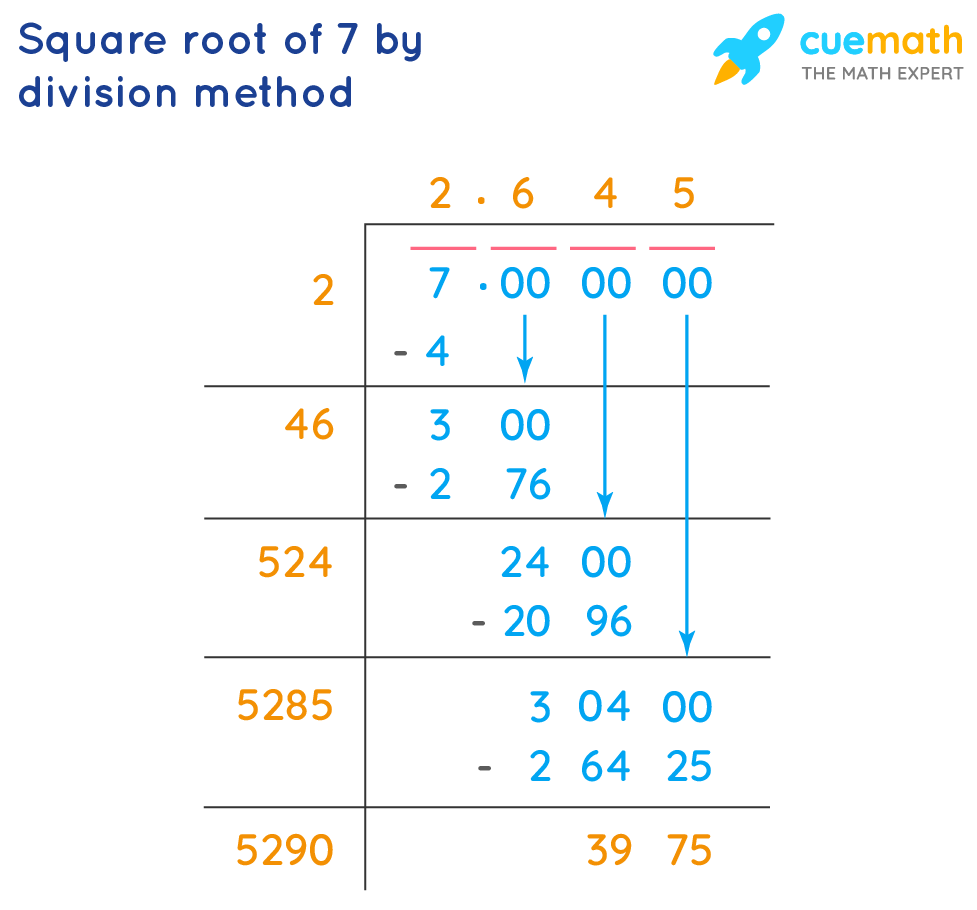
  To find \( \sqrt{7} \) using the long division method, follow these steps:

  1. Write 7 as 7.0000.
  2. Find a number whose square is less than or equal to 7 (e.g., 2).
  3. Subtract the square of this number (4) from 7 to get the remainder (3).
  4. Bring down a pair of zeros and double the quotient (2) to get the new divisor (4).
  5. Find a digit (6) such that (40 + 6) × 6 is less than or equal to 300.
  6. Repeat the process to find the value up to the desired decimal places.

  This process yields an approximate value of \( \sqrt{7} \) as 2.645.

The calculation of the square root of 7 can be approached through several methods, ensuring precision and understanding of the underlying mathematical concepts.

• Long Division Method:
  1. Write the number 7 in decimal form as 7.0000.
  2. Determine the largest integer whose square is less than or equal to 7, which is 2 (since 2^2 = 4).
  3. Subtract 4 from 7 to get a remainder of 3.
  4. Double the quotient (2) to get 4, then determine the new divisor by finding a number that, when added to 4 and multiplied by itself, remains less than 300. This gives us 46 (since 46*6 = 276).
  5. Subtract 276 from 300 to get 24, then bring down the next pair of zeros and repeat the process to refine the result up to the desired decimal places.

  The approximate value of the square root of 7 using the long division method is 2.645751311.

• Guess and Check Method:
  1. Make an initial guess, such as 2.5.
  2. Square the guess and compare it to 7.
  3. Adjust the guess based on whether the squared result is too high or too low.
  4. Repeat until the guess is sufficiently close to the actual square root of 7.
• Estimation Method:
  1. Recognize that the square root of 7 is between the square roots of 4 (2) and 9 (3).
  2. Make an educated guess and refine it through trial and error.
• Pattern Recognition Method:
  1. Use knowledge of perfect squares to guide the estimation.
  2. Start with a guess closer to 2 than to 3 and refine it.
• Visual Representation Method:
  1. Draw a number line between 2 and 3.
  2. Estimate where the square root of 7 might fall based on its position between 4 and 9.
• Binomial Expansion:

  The binomial expansion formula can also be used, although it is more complex:

  \[
  (1 + x)^n = 1 + nx + \frac{n(n-1)}{2!}x^2 + \frac{n(n-1)(n-2)}{3!}x^3 + \ldots
  \]

  Using this expansion, the value of \(\sqrt{7}\) can be approximated.

Each method has its unique advantages and can be chosen based on the required accuracy and the available tools.

The square root of 7, approximately 2.6457513110645906, has several practical applications across various fields. Understanding these applications provides insight into how mathematical concepts are utilized in real-world scenarios.

• Geometry: The square root of 7 appears in geometric constructions and problems. For instance, it is the length of the diagonal of a rectangle with sides of 1 and √6 units. Additionally, it is involved in calculating distances in certain geometric shapes, such as the minimal enclosing rectangle of an equilateral triangle.
• Physics: The square root of 7 can be found in various physics formulas, especially those involving wave frequencies and oscillations. Understanding this root helps in precise calculations required for engineering and scientific research.
• Engineering: Engineers often encounter the square root of 7 when dealing with complex structures and mechanical systems. It is essential for accurately determining stress and strain in materials, ensuring safety and reliability in construction projects.
• Mathematical Puzzles and Games: The square root of 7 is used in mathematical puzzles and games, challenging individuals to apply their understanding of roots and exponents. These puzzles enhance problem-solving skills and mathematical intuition.
• Computer Science: In algorithms and computational mathematics, the square root of 7 is used in various numerical methods and optimizations. Efficiently computing this root is crucial for performance in software applications.
• Finance: The concept of square roots, including the square root of 7, is applied in financial calculations such as determining compound interest, risk assessment, and financial modeling.

Calculating the square root of 7 times the square root of 7 involves understanding basic multiplication of square roots. Here are some detailed examples to help illustrate the process:

• Basic Example: The square root of 7 multiplied by the square root of 7 is written as √7 × √7. According to the property of square roots, this is equivalent to the square root of (7 × 7), which simplifies to the square root of 49. Since the square root of 49 is 7, we have:

  \[
  \sqrt{7} \times \sqrt{7} = \sqrt{49} = 7
  \]

• Using Prime Factorization: The square root of a number can also be found using its prime factorization. For example, the square root of 49 is determined by breaking 49 into its prime factors:
  1. 49 = 7 × 7
  2. Taking the square root of both sides, we get:

     \[
     \sqrt{49} = \sqrt{7 \times 7} = 7
     \]

• Decimal Numbers: If the number is not a perfect square, finding its square root involves a bit more calculation. For instance, the square root of 7 is approximately 2.64575. Multiplying this by itself yields:

  \[
  2.64575 \times 2.64575 \approx 7
  \]

  This shows that:

  \[
  \sqrt{7} \times \sqrt{7} \approx 7
  \]

• Negative Numbers: The square root of a negative number involves imaginary numbers. For instance, the square root of -7 times the square root of -7 is:

  \[
  \sqrt{-7} \times \sqrt{-7} = \sqrt{49} \cdot (-1) = 7i^2 = -7
  \]

For further understanding and detailed steps regarding the calculation and properties of the square root of 7, consider exploring the following resources:

• Long Division Method:

  The long division method is a step-by-step approach to finding the square root of non-perfect squares like 7. This method involves dividing, averaging, and iterating to reach the approximate value of √7. You can find detailed steps on platforms like Cuemath and BYJUS.

  1. Write 7 as 7.0000.
  2. Divide 7 by a number whose square is less than or equal to 7. The quotient is 2 and remainder is 3.
  3. Double the quotient and use it as the new divisor. Continue the process by bringing down pairs of zeros and finding suitable divisors until the desired accuracy is reached.
  4. This method yields √7 ≈ 2.6457513111.

• Babylonian Method (Newton's Method):

  This iterative method starts with an initial guess and improves the approximation of the square root through successive iterations. Each step involves averaging the current guess with the quotient of dividing 7 by the current guess. This method quickly converges to the accurate value of √7.

• Geometric Interpretation:

  The square root of 7 has interesting geometric properties. For example, it can be visualized as the diagonal of a specific rectangle, which provides insight into its spatial characteristics. This approach is often illustrated in mathematical geometry resources.

• Applications:

  • In geometry, the square root of 7 is used in calculating the diagonal lengths of rectangles and other geometric shapes.
  • In physics, √7 appears in formulas involving root calculations, demonstrating its practical significance in scientific computations.

• Practical Examples:

  • Finding the radius of a circular area: If a pizza has an area of 22 square units, its radius would be √(22/π) which simplifies to √7, approximately 2.645 units.
  • Simplifying expressions involving square roots: For instance, simplifying 2√7 + 5√7 results in 7√7.

For more detailed explanations, examples, and practice problems, visit educational websites such as Cuemath, BYJUS, Mathway, and Omnicalculator.