Topic is the square root of 7 a rational number: Discover the intriguing nature of the square root of 7 and whether it qualifies as a rational number. Explore the mathematical principles and proofs that reveal why the square root of 7 cannot be expressed as a simple fraction, highlighting its status as an irrational number with fascinating properties.
Table of Content
- Is the Square Root of 7 a Rational Number?
- Introduction to Square Roots
- Understanding Rational and Irrational Numbers
- Prime Numbers and Their Properties
- Why is the Square Root of 7 Irrational?
- Mathematical Proofs of Irrationality
- Methods to Calculate the Square Root of 7
- Applications of Square Root of 7
- Constructing the Square Root of 7 on a Number Line
- Historical Approaches to Square Roots
- Frequently Asked Questions
- YOUTUBE:
Is the Square Root of 7 a Rational Number?
The square root of 7 (\(\sqrt{7}\)) is an important concept in mathematics, especially when discussing rational and irrational numbers. Here, we explore why \(\sqrt{7}\) is considered irrational and provide some related information and examples.
Definition of Rational and Irrational Numbers
- A rational number can be expressed as a fraction \(\frac{p}{q}\), where \(p\) and \(q\) are integers and \(q \neq 0\).
- An irrational number cannot be expressed as a simple fraction. It has a non-repeating, non-terminating decimal expansion.
Why is \(\sqrt{7}\) Irrational?
To understand why \(\sqrt{7}\) is irrational, let's examine the nature of its decimal expansion and some mathematical proofs:
- Prime Number Consideration: The number 7 is a prime number, meaning it has no divisors other than 1 and itself. This fact is a basis for proving the irrationality of its square root.
- Non-Terminating Decimal: The decimal expansion of \(\sqrt{7}\) is approximately 2.6457513110645906... and it continues without repeating, which is characteristic of irrational numbers.
- Proof by Contradiction: Assume \(\sqrt{7}\) is rational, meaning it can be written as \(\frac{p}{q}\) in lowest terms. Then \(p^2 = 7q^2\). This implies \(p^2\) is divisible by 7, hence \(p\) must be divisible by 7. Let \(p = 7k\). Substituting, we get \(49k^2 = 7q^2\), or \(q^2 = 7k^2\), implying \(q\) is also divisible by 7, which contradicts the assumption that \(\frac{p}{q}\) is in lowest terms. Thus, \(\sqrt{7}\) cannot be rational.
Examples and Applications
| Example | Calculation |
|---|---|
| Area of a Square | If the area of a square is 7 square units, the side length is \(\sqrt{7}\) units, which is approximately 2.645 units. |
| Right Triangle Hypotenuse | In a right triangle with legs of lengths \(\sqrt{3}\) and 2, the hypotenuse is \(\sqrt{(\sqrt{3})^2 + 2^2} = \sqrt{3 + 4} = \sqrt{7}\). |
Representation on the Number Line
The construction of \(\sqrt{7}\) on the number line involves several steps using the Pythagorean theorem, progressively building upon known lengths (\(\sqrt{1}, \sqrt{2}, \sqrt{3}, \sqrt{4}, \sqrt{5}, \sqrt{6}\)) to finally construct \(\sqrt{7}\).
Conclusion
In summary, \(\sqrt{7}\) is an irrational number as it cannot be expressed as a fraction and its decimal expansion is non-terminating and non-repeating. This characteristic is shared by the square roots of all prime numbers.
Understanding the nature of \(\sqrt{7}\) helps in appreciating the distinction between rational and irrational numbers, and is fundamental in various mathematical applications.
READ MORE:
Introduction to Square Roots
The concept of square roots is fundamental in mathematics, playing a crucial role in various calculations and problem-solving scenarios. A square root of a number is a value that, when multiplied by itself, gives the original number. For instance, the square root of 9 is 3, as 3 squared equals 9. Square roots are often used in algebra, geometry, and various fields of science and engineering.
Mathematically, the square root of a number \( n \) is represented as \( \sqrt{n} \) or \( n^{1/2} \). Square roots can be both positive and negative because both positive and negative numbers, when squared, yield a positive product. For example, both \( \sqrt{9} = 3 \) and \( \sqrt{9} = -3 \) are valid, as \( 3^2 = 9 \) and \( (-3)^2 = 9 \).
However, not all numbers have a neat integer as their square root. Numbers like 2, 3, 5, and 7 do not have integer square roots, making their square roots irrational numbers. An irrational number cannot be expressed as a simple fraction; it has a non-repeating, non-terminating decimal expansion. The square root of 7, for instance, is approximately 2.645751311, which cannot be accurately represented as a fraction.
To understand the nature of square roots more deeply, consider the methods of calculating them:
- Guess and Check Method: Start with an initial guess and refine it through iteration until the result is sufficiently accurate.
- Estimation Method: Use knowledge of perfect squares to narrow down the range within which the square root lies.
- Pattern Recognition Method: Recognize patterns in square roots of close numbers to guide your estimation.
- Visual Representation Method: Draw a number line to visually estimate where the square root falls.
One common manual method is the long division method, which provides a step-by-step approach to finding the square root of a number to a desired degree of accuracy. This method involves dividing and averaging steps to hone in on the correct value.
Understanding square roots and their properties is essential for solving equations, analyzing geometric shapes, and more. They are a key element in the mathematical toolkit, enabling precise and varied computations.
Understanding Rational and Irrational Numbers
In mathematics, numbers are classified into different categories based on their properties. One of the primary classifications is between rational and irrational numbers. Understanding the differences between these types of numbers is essential for grasping more advanced mathematical concepts.
Rational Numbers: A rational number is any number that can be expressed as a fraction \(\frac{p}{q}\), where \(p\) and \(q\) are integers and \(q \neq 0\). Rational numbers can be finite decimals, repeating decimals, or whole numbers. For example:
- \(\frac{1}{2}\)
- \(0.75\) (which is \(\frac{3}{4}\))
- \(-5\)
Irrational Numbers: An irrational number, on the other hand, cannot be expressed as a simple fraction. These numbers have non-terminating and non-repeating decimal expansions. Common examples of irrational numbers include:
- \(\sqrt{2}\)
- \(\pi\)
- \(e\) (the base of the natural logarithm)
One of the defining characteristics of irrational numbers is that their decimal form never ends and never forms a repeating pattern. For instance, \(\sqrt{7}\) is an irrational number because it cannot be precisely written as a fraction and its decimal expansion goes on infinitely without repeating.
The distinction between rational and irrational numbers is crucial in many areas of mathematics. Together, rational and irrational numbers form the set of real numbers. This classification helps in understanding the properties of numbers and their behavior in different mathematical operations.
| Type of Number | Examples |
| Rational Numbers | \(\frac{1}{2}, 0.75, -5\) |
| Irrational Numbers | \(\sqrt{2}, \pi, e\) |
In conclusion, while rational numbers are easily expressible as fractions and have predictable decimal expansions, irrational numbers are more complex with infinite, non-repeating decimals. Both types of numbers are fundamental to the broader understanding of the number system and real numbers.
Prime Numbers and Their Properties
Prime numbers are natural numbers greater than 1 that have no positive divisors other than 1 and themselves. They are the building blocks of the number system, playing a crucial role in number theory and various mathematical applications.
Here are some key properties of prime numbers:
- Unique Factorization: Every natural number greater than 1 can be uniquely represented as a product of prime numbers. This is known as the Fundamental Theorem of Arithmetic.
- Infinitude: There are infinitely many prime numbers. This was first proven by the ancient Greek mathematician Euclid.
- Prime Density: As numbers get larger, primes become less frequent. The distribution of prime numbers among the natural numbers is a central topic in number theory.
- Prime Gaps: The difference between consecutive prime numbers is called a prime gap. There is no largest prime gap, and they can vary significantly.
- Prime Testing: Various algorithms exist to test if a number is prime, such as trial division, the Sieve of Eratosthenes, and more advanced methods like the Miller-Rabin primality test.
- Applications: Prime numbers are used in cryptography, computer science, and for creating random number generators, among other applications.
Prime numbers are also closely related to the concept of irrational numbers. While prime numbers themselves are integers, they help in understanding the properties of irrational numbers. For instance, if a prime number's square root is not a perfect square, then it is an irrational number.
Here is a table summarizing some small prime numbers and their properties:
| Prime Number | Square | Square Root (Approx.) | Remarks |
|---|---|---|---|
| 2 | 4 | 1.414 | Smallest prime number |
| 3 | 9 | 1.732 | Next prime number after 2 |
| 5 | 25 | 2.236 | Prime, larger than 2 |
| 7 | 49 | 2.646 | Related to the topic |
Why is the Square Root of 7 Irrational?
The square root of 7, denoted as √7, is considered irrational. An irrational number is a number that cannot be expressed as a simple fraction of two integers. To understand why √7 is irrational, let's delve into a mathematical proof using contradiction.
Assume, for the sake of contradiction, that √7 is rational. This means we can express it as a fraction in its simplest form, p/q, where p and q are coprime integers (i.e., they have no common factors other than 1), and q is not zero.
So, we have:
Squaring both sides of the equation, we get:
Multiplying both sides by q2 to clear the fraction, we get:
This implies that p2 is divisible by 7. Therefore, p must also be divisible by 7 (since 7 is a prime number). Let's write p as:
for some integer k. Substituting back into the equation, we get:
This equation shows that q2 is also divisible by 7, implying that q must be divisible by 7. Therefore, both p and q have a common factor of 7. This contradicts our initial assumption that p and q are coprime. Hence, our original assumption that √7 is rational must be false.
Therefore, we conclude that √7 is irrational, meaning it cannot be expressed as a fraction of two integers. Its decimal representation is non-terminating and non-repeating, confirming its irrationality.

Mathematical Proofs of Irrationality
The square root of 7 is irrational, meaning it cannot be expressed as a fraction of two integers. Here's a detailed proof using the method of contradiction:
- Assume that
\(\sqrt{7}\) is rational. This means it can be written as\(\frac{p}{q}\) , where\(p\) and\(q\) are coprime integers (having no common factors other than 1) and\(q \neq 0\) . - According to our assumption:
\(\sqrt{7} = \frac{p}{q}\) . - Squaring both sides, we get:
\(7 = \frac{p^2}{q^2}\) . - Rewriting the equation, we have:
\(p^2 = 7q^2\) . - This implies that
\(p^2\) is divisible by 7, so\(p\) must also be divisible by 7 (since 7 is a prime number). - Let
\(p = 7k\) for some integer\(k\) . Substituting this back into the equation, we get:\((7k)^2 = 7q^2\) . - This simplifies to:
\(49k^2 = 7q^2\) , and further to:\(q^2 = 7k^2\) . - Thus,
\(q^2\) is also divisible by 7, meaning\(q\) must be divisible by 7. - Therefore, both
\(p\) and\(q\) are divisible by 7, which contradicts our initial assumption that they are coprime. - Hence, our initial assumption that
\(\sqrt{7}\) is rational must be false. Therefore,\(\sqrt{7}\) is irrational.
This contradiction proves that the square root of 7 cannot be expressed as a fraction of two integers, confirming its irrationality.
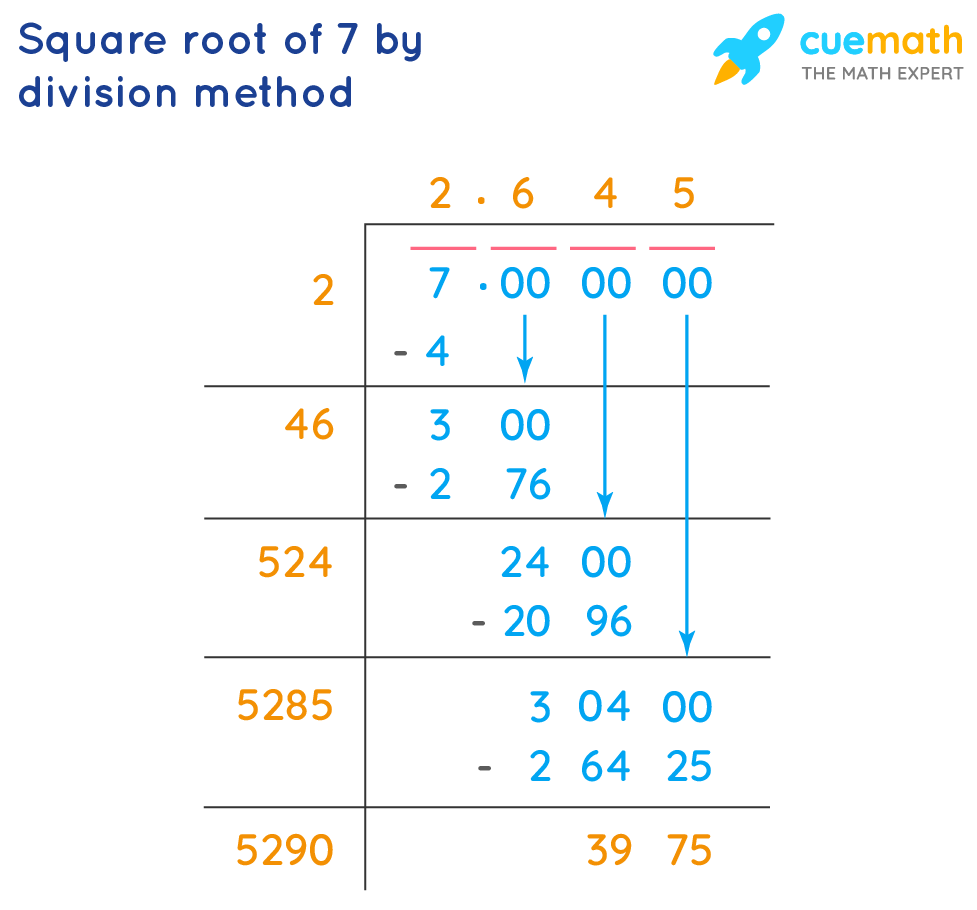
Methods to Calculate the Square Root of 7
Calculating the square root of 7 involves several methods, each with its own level of precision and complexity. Here are a few common methods:
1. Long Division Method
The long division method provides a systematic approach to finding the square root of a non-perfect square like 7.
- Start by writing 7 as 7.0000 to handle decimal places.
- Find a number whose square is less than or equal to 7. In this case, 22 = 4, so 2 is the initial quotient.
- Subtract 4 from 7, leaving a remainder of 3. Bring down two zeros, making it 300.
- Double the quotient (2), giving 4. Use 40 as the new divisor and find the largest digit (x) such that (40 + x)x is less than or equal to 300. Here, 46 x 6 = 276.
- Subtract 276 from 300 to get 24. Bring down another pair of zeros, making it 2400.
- Double the quotient part (26) to get 52, and find the new digit (y) such that (520 + y)y is less than or equal to 2400. Repeat this process to obtain more decimal places.
Following these steps, the square root of 7 is approximately 2.645.
2. Newton's Method (Newton-Raphson Method)
This iterative method starts with an initial guess and refines it using the formula:
\( x_{n+1} = \frac{1}{2} \left( x_n + \frac{7}{x_n} \right) \)
Steps:
- Choose an initial guess (e.g., \( x_0 = 2.5 \)).
- Apply the formula iteratively:
- \( x_1 = \frac{1}{2} \left( 2.5 + \frac{7}{2.5} \right) = 2.65 \)
- \( x_2 = \frac{1}{2} \left( 2.65 + \frac{7}{2.65} \right) \approx 2.645 \)
- Continue until the values stabilize to a desired precision.
This method converges quickly to the square root value.
3. Using a Calculator
Modern calculators and online tools can quickly provide the square root of 7. For example:
- Enter the number 7 into the calculator.
- Press the square root (√) button.
- The display will show approximately 2.6457513110645906.
4. Approximation Methods
For rough estimates, you can use simpler arithmetic techniques:
- Recognize that \( 2^2 = 4 \) and \( 3^2 = 9 \). Since 7 is closer to 9, start with a value slightly less than 3.
- Refine the approximation using fractional values, such as 2.6 or 2.65, to get closer to the true value.
These methods provide various ways to calculate the square root of 7, each suited for different levels of precision and computational resources.
Applications of Square Root of 7
The square root of 7, represented as \( \sqrt{7} \), has several interesting applications in various fields, including mathematics, engineering, and science. Here are some notable uses:
-
Mathematical Problem Solving:
The value of \( \sqrt{7} \) is used in solving algebraic equations and inequalities where the presence of irrational numbers is crucial. For instance, it appears in quadratic equations and in the simplification of expressions involving radicals.
-
Geometry and Trigonometry:
In geometry, \( \sqrt{7} \) can be used to determine lengths and distances that are not easily expressed as rational numbers. For example, in the Pythagorean theorem, finding the hypotenuse of a right triangle with sides of certain lengths might involve \( \sqrt{7} \).
-
Physics and Engineering:
The square root of 7 is used in various physics equations and engineering calculations. It can appear in formulas related to wave frequencies, signal processing, and other areas where precise measurements are required.
-
Computer Science:
In computer algorithms, especially those involving computational geometry and numerical methods, \( \sqrt{7} \) may be used in calculations that require high precision. It helps in the approximation of certain values and in the development of algorithms that handle irrational numbers efficiently.
-
Construction and Architecture:
Architects and construction engineers use \( \sqrt{7} \) when designing structures that involve non-standard angles and dimensions. It helps in creating blueprints that require precise measurements and calculations.
-
Astronomy:
In astronomy, \( \sqrt{7} \) might be used in calculating distances between celestial bodies or in the analysis of orbital mechanics where precise measurements are essential.
Overall, the square root of 7 is a fundamental irrational number that plays a critical role in both theoretical and practical applications across multiple disciplines.
Constructing the Square Root of 7 on a Number Line
To represent the square root of 7 on a number line, we can use a geometric approach involving the Pythagorean theorem. Here are the steps:
-
Draw a horizontal line and mark a point \( O \) as the origin.
-
Mark a point \( A \) on the line such that \( OA = 1 \).
-
Draw a perpendicular line at point \( A \) and mark a point \( B \) on it such that \( AB = 1 \).
-
Use the Pythagorean theorem to find the hypotenuse \( OB \):
\[
OB^2 = OA^2 + AB^2 = 1^2 + 1^2 = 2
\]
Hence, \( OB = \sqrt{2} \). -
With \( O \) as the center and \( OB \) as the radius, draw an arc to intersect the horizontal line at point \( C \). Now \( OC = \sqrt{2} \).
-
From point \( C \), draw another perpendicular line and mark a point \( D \) such that \( CD = 1 \).
-
Again, use the Pythagorean theorem to find \( OD \):
\[
OD^2 = OC^2 + CD^2 = 2 + 1 = 3
\]
Hence, \( OD = \sqrt{3} \). -
With \( O \) as the center and \( OD \) as the radius, draw an arc to intersect the horizontal line at point \( E \). Now \( OE = \sqrt{3} \).
-
From point \( E \), draw another perpendicular line and mark a point \( F \) such that \( EF = 2 \).
-
Use the Pythagorean theorem to find \( OF \):
\[
OF^2 = OE^2 + EF^2 = 3 + 4 = 7
\]
Hence, \( OF = \sqrt{7} \). -
With \( O \) as the center and \( OF \) as the radius, draw an arc to intersect the horizontal line at point \( G \). Now \( OG = \sqrt{7} \).
Thus, point \( G \) represents \( \sqrt{7} \) on the number line.

Historical Approaches to Square Roots
The concept and calculation of square roots have evolved significantly throughout history, influenced by various cultures and mathematical advancements. Here are some key historical approaches:
-
Babylonian Method (circa 1800 BCE):
The Babylonians used an iterative method to approximate square roots. Their approach is similar to what is now known as Newton's method. They would start with an initial guess and refine it using the formula:
\[
x_{n+1} = \frac{1}{2} \left( x_n + \frac{S}{x_n} \right)
\]
where \(S\) is the number for which the square root is being calculated, and \(x_n\) is the current approximation. -
Greek Geometry (circa 300 BCE):
Greek mathematicians like Euclid approached square roots through geometric means. They used the Pythagorean theorem and geometric constructions to find square roots, especially within the context of solving quadratic equations.
-
Indian Mathematicians (circa 500 CE):
Hindu mathematicians, such as Aryabhata and later Brahmagupta, made significant contributions. They used methods involving algebraic manipulation and provided rules for finding square roots and solving quadratic equations.
-
Islamic Scholars (circa 800 CE):
Persian mathematicians like Al-Khwarizmi further developed algebra and introduced systematic methods for solving quadratic equations, which included finding square roots. They translated and expanded upon Greek and Indian works, preserving and enhancing mathematical knowledge.
-
European Renaissance (circa 1500 CE):
During the Renaissance, European mathematicians like Cardano and Newton made significant advancements. Newton's method, derived from the earlier Babylonian method, provided a powerful iterative approach to approximating roots. Newton's formulation:
\[
x_{n+1} = x_n - \frac{f(x_n)}{f'(x_n)}
\]
became a fundamental tool in numerical analysis.
These historical approaches highlight the diverse and rich development of mathematical techniques for calculating square roots, reflecting the contributions of many different cultures and eras.
Frequently Asked Questions
-
What is the square root of 7?
The square root of 7 is an irrational number, approximately equal to 2.64575. It cannot be expressed as a simple fraction.
-
Is the square root of 7 a rational number?
No, the square root of 7 is not a rational number. It cannot be written as a ratio of two integers.
-
How can we prove that the square root of 7 is irrational?
One common proof involves assuming that \( \sqrt{7} \) is rational, meaning it can be written as \( \frac{p}{q} \) where \( p \) and \( q \) are coprime integers. Squaring both sides leads to a contradiction, proving \( \sqrt{7} \) is irrational.
-
What are the uses of the square root of 7?
The square root of 7 is used in various mathematical and scientific calculations, including geometry, algebra, and physics, especially in problems involving roots and irrational numbers.
-
Can the square root of 7 be simplified?
The square root of 7 cannot be simplified further as it is already in its simplest form.
-
What are the properties of the square root of 7?
The square root of 7 is an irrational number, meaning it has a non-repeating, non-terminating decimal expansion. It is also a real number and can be located on the number line.
-
How do we approximate the square root of 7?
We can approximate the square root of 7 using methods like the Newton-Raphson method, long division, or using a calculator. The value is approximately 2.64575.
-
What is the historical significance of the square root of 7?
The concept of square roots, including that of 7, dates back to ancient civilizations where mathematicians explored irrational numbers and their properties.
Khái niệm Căn bậc hai | Toán học với thầy J
READ MORE:
Video chứng minh rằng căn bậc hai của bất kỳ số nguyên nào cũng là vô tỷ, ngoại trừ các số chính phương. Nội dung giúp bạn hiểu rõ hơn về tính chất của các số này.
Chứng minh rằng căn bậc hai của bất kỳ số nguyên nào cũng là vô tỷ (trừ các số chính phương)