Square Root of 7 Simplified: Understanding and Calculation

The square root of 7, denoted as √7, is an irrational number. Here is a detailed explanation on how to simplify and calculate the square root of 7 using various methods.

Decimal Form

The square root of 7 in decimal form is approximately:

2.6457513110645907

Exponential Form

The square root of 7 can also be expressed in exponential form:

7^0.5 or 7^1/2

Radical Form

The radical form of the square root of 7 is:

√7

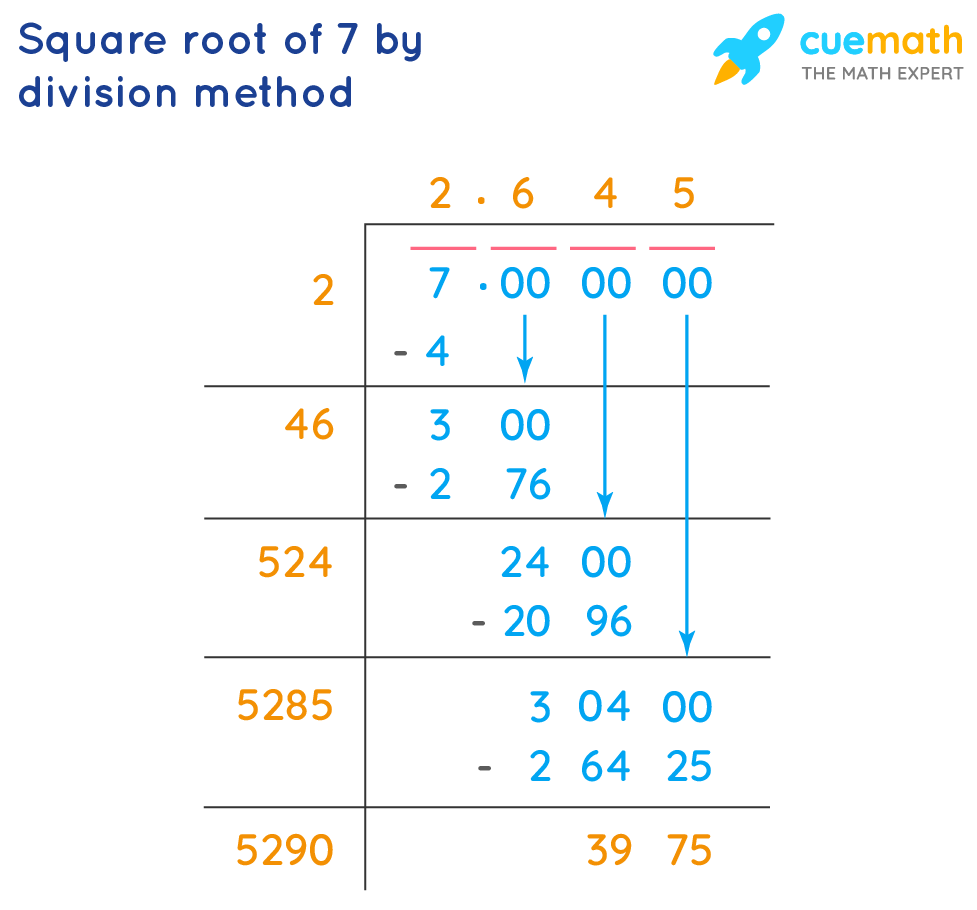
Long Division Method

1. Write the number 7 in decimal form (7.0000).
2. Divide 7 by a number such that the product of the number with itself is less than or equal to 7. We choose 2 because 2 × 2 = 4, which is less than 7.
3. Subtract 4 from 7 to get a remainder of 3. Bring down a pair of zeros to make it 300.
4. Double the quotient (2), making it 4. Use 40 as the new divisor.
5. Find a number that, when added to 40 and multiplied by itself, is less than or equal to 300. The number is 6, making it 46. So, 46 × 6 = 276.
6. Subtract 276 from 300 to get a remainder of 24 and bring down the next pair of zeros, making it 2400.
7. Double the quotient (26), making it 52. Use 520 as the new divisor and find the number to complete the division process. Repeat the steps until you reach the desired accuracy.
8. The approximate value of √7 is 2.645.

Simplifying Square Roots

To simplify square roots, look for perfect square factors. Here are some examples:

• √27 can be simplified to 3√3 because 27 = 9 × 3 and √27 = √(9 × 3) = √9 × √3 = 3√3
• √45 can be simplified to 3√5 because 45 = 9 × 5 and √45 = √(9 × 5) = √9 × √5 = 3√5
• √8 can be simplified to 2√2 because 8 = 4 × 2 and √8 = √(4 × 2) = √4 × √2 = 2√2

Special Cases

When adding or subtracting square roots, combine like terms:

For example, 2√7 + 5√7 = 7√7

Applications

Here are some solved examples involving the square root of 7:

1. Example 1: If the area of a pizza is 22 square units, the radius is √(22/π) ≈ √7 = 2.645 units.
2. Example 2: In a right-angled triangle with legs √3 and 2, the hypotenuse is √(3+4) = √7 = 2.645 units.

Understanding Irrational Numbers

The square root of 7 is irrational because it cannot be expressed as a fraction of two integers. This is due to the fact that 7 is a prime number and does not have a pair.

We hope this detailed guide helps you understand the concept of the square root of 7 and how to work with it in different mathematical contexts.

The concept of square roots is fundamental in mathematics, often used in various calculations and problem-solving scenarios. A square root of a number is a value that, when multiplied by itself, gives the original number. For instance, the square root of 9 is 3 because 3 multiplied by 3 equals 9. Similarly, the square root of 7 is denoted as √7, and its approximate value is 2.6457513110645907.

Square roots are essential in algebra and geometry, helping solve equations and understand geometric properties. They appear in quadratic equations, Pythagorean theorem applications, and more. Understanding how to simplify square roots, especially for non-perfect squares like 7, can make complex mathematical problems more manageable.

Here are the steps to simplify a square root:

1. Identify if the number is a perfect square. If it is, find the integer value. For example, √16 = 4.
2. If the number is not a perfect square, factorize it into its prime factors.
3. Look for pairs of prime factors. Each pair can be taken out of the square root symbol.
4. Multiply the numbers outside the square root together and keep the remaining number inside the square root.

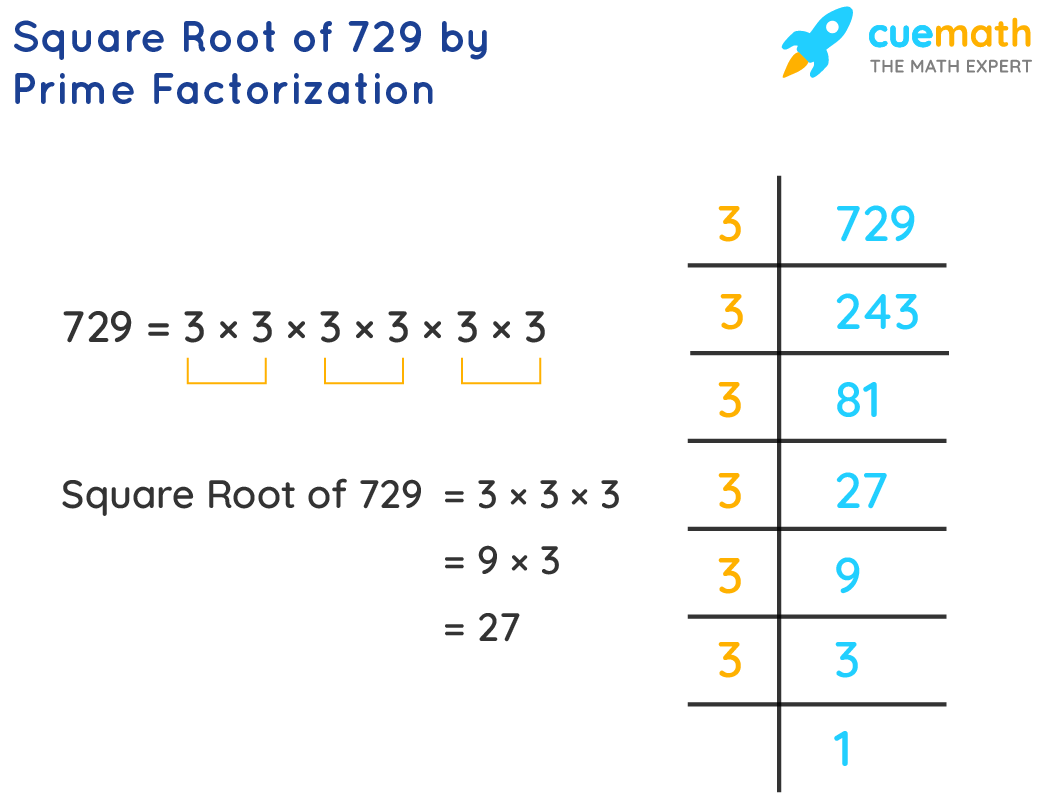
Let's take an example of simplifying the square root of 45:

• 45 can be factorized into 9 and 5 (since 45 = 9 * 5).
• We know that 9 is a perfect square (√9 = 3).
• Thus, √45 = √(9 * 5) = √9 * √5 = 3√5.

For the square root of 7, which is already in its simplest form (since 7 is a prime number), the simplification process is straightforward:

√7 ≈ 2.6457513110645907

Square roots can also be expressed in exponential form, where √x = x^(1/2). This notation is particularly useful in higher mathematics, including calculus and algebra.

The square root of 7, denoted as √7, is an irrational number, meaning it cannot be expressed as a simple fraction. Its decimal form is approximately 2.6457513110645907. This value is crucial in various mathematical computations and can be found using methods like long division.

Here's a detailed step-by-step process to understand and calculate the square root of 7:

1. First, represent the number 7 in decimal form: 7.0000.
2. Find the largest square number less than or equal to 7. In this case, 2 × 2 = 4.
3. Subtract 4 from 7 to get the remainder 3. Write down the quotient 2.
4. Bring down a pair of zeros to the right of the remainder, making it 300.
5. Double the quotient (2), which gives 4, and use 40 as the new divisor.
6. Find a number that, when multiplied by 40 plus itself, gives a product less than or equal to 300. Here, it is 6 (46 × 6 = 276).
7. Subtract 276 from 300 to get 24, and bring down another pair of zeros to make it 2400.
8. Repeat this process to achieve the desired precision. The next steps involve using 520 as the new divisor, finding a suitable digit, and so on, until the square root is approximated to the required decimal places.

Through this method, the approximate value of √7 is found to be 2.645. This method ensures accuracy and is often used for finding the square roots of numbers that are not perfect squares.

Expressing √7 in different forms:

• Radical Form: √7
• Decimal Form: 2.6457513110645907
• Exponential Form: 7^1/2 or 7^0.5

Understanding and calculating the square root of 7 is fundamental in various fields of mathematics, including algebra and geometry. Whether you are solving quadratic equations or working on geometric problems, knowing how to simplify and compute square roots is an essential skill.

Calculating the square root of 7 involves several methods depending on the desired precision and form of the result:

1. Estimation Method: Start with an approximate guess, refine using successive approximations until desired precision is achieved.
2. Long Division Method: Similar to traditional long division, iteratively determine each digit of the square root until sufficient decimal places are obtained.
3. Newton's Method: Use the iterative formula \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{7}{x_n} \right) \) to converge towards the square root of 7.
4. Using a Calculator: Utilize a scientific calculator or computer software to obtain an accurate decimal approximation or the exact radical form of \( \sqrt{7} \).

The square root of 7 can be expressed in both decimal and radical forms:

• Decimal Form: \( \sqrt{7} \approx 2.6457513110645906 \)
• Exact Radical Form: \( \sqrt{7} \) is an irrational number and cannot be expressed exactly as a simple fraction or decimal. It remains in its radical form as \( \sqrt{7} \).

In mathematics, understanding the distinction between perfect squares and imperfect squares is essential when working with square roots.

Perfect Squares: A perfect square is a number that is the square of an integer. For example, the numbers 1, 4, 9, 16, 25, and 36 are all perfect squares because they can be written as:

• 1 = 1²
• 4 = 2²
• 9 = 3²
• 16 = 4²
• 25 = 5²
• 36 = 6²

Perfect squares have whole numbers as their square roots, making them straightforward to simplify. For instance, \(\sqrt{25} = 5\) and \(\sqrt{36} = 6\).

Imperfect Squares: An imperfect square, on the other hand, is a number that does not have an integer as its square root. Examples of imperfect squares include numbers like 2, 3, 5, 7, 8, and 10. These numbers cannot be expressed as the square of an integer and their square roots are irrational numbers.

• \(\sqrt{2} \approx 1.414\)
• \(\sqrt{3} \approx 1.732\)
• \(\sqrt{5} \approx 2.236\)
• \(\sqrt{7} \approx 2.646\)
• \(\sqrt{8} \approx 2.828\)
• \(\sqrt{10} \approx 3.162\)

Since imperfect squares are not exact squares of integers, their square roots are often left in the radical form or approximated to a certain number of decimal places. For example, the square root of 7 is represented as \(\sqrt{7}\) or approximately 2.646.

To simplify imperfect squares, we sometimes express them in terms of simpler radicals if possible. For example, \(\sqrt{8}\) can be simplified as \(\sqrt{4 \times 2} = \sqrt{4} \times \sqrt{2} = 2\sqrt{2}\).

Understanding the difference between perfect and imperfect squares helps in the simplification and computation of square roots in various mathematical contexts.

The square root of 7, denoted as \( \sqrt{7} \), is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal form goes on indefinitely without repeating. Here are some key properties and rules related to the square root of 7:

1. Approximation: \( \sqrt{7} \approx 2.6457513110645906 \)
2. Decimal Representation: \( \sqrt{7} \) in decimal form is approximately 2.6457513110645906.
3. Prime Factorization: The square root of 7 is related to its prime factorization as \( \sqrt{7} = \sqrt{7} \).
4. Calculation Methods: There are various methods to approximate or calculate the square root of 7, including long division, iterative methods, and using scientific calculators.
5. Simplification: \( \sqrt{7} \) cannot be simplified further because 7 is a prime number.
6. Properties:
   • Multiplication Property: For any real numbers \( a \geq 0 \) and \( b \geq 0 \), \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \).
   • Addition Property: There is no simple addition property for square roots like \( \sqrt{a+b} \).
   • Division Property: For \( b \neq 0 \), \( \frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}} \).
   • Exponentiation Property: \( (\sqrt{a})^2 = a \).
7. Applications: Square roots, including \( \sqrt{7} \), are used extensively in mathematics, physics, engineering, and various scientific fields for calculations involving distances, areas, volumes, and more.

When simplifying the square root of 7, we consider methods to express \( \sqrt{7} \) in a more manageable form. Here are some examples:

1. Rationalizing the Denominator: \( \sqrt{7} \) cannot be simplified into a rational number since 7 is a prime number. Therefore, \( \sqrt{7} \) remains \( \sqrt{7} \).
2. Approximation: \( \sqrt{7} \approx 2.6457513110645906 \) is a useful decimal approximation for practical calculations.
3. Using Factorization: Recognizing that 7 is a prime number, we understand that \( \sqrt{7} \) does not simplify further beyond \( \sqrt{7} \).
4. Calculation Methods: Various techniques, including long division or iterative approaches, can be applied to estimate \( \sqrt{7} \).
5. Real-life Applications: \( \sqrt{7} \) is frequently encountered in geometry, physics, and engineering for calculating distances, areas, and other measurements.

The square root of 7, \( \sqrt{7} \), finds practical applications in various fields where precise calculations are essential. Here are some examples:

1. Geometry: In geometry, \( \sqrt{7} \) helps calculate the diagonal of a square whose side length is 7 units.
2. Physics: In physics, \( \sqrt{7} \) appears in equations related to wave propagation, such as calculating wavelengths or frequencies.
3. Engineering: Engineers use \( \sqrt{7} \) in structural analysis to determine stresses and strains, as well as in designing mechanical components.
4. Finance: Financial analysts may use \( \sqrt{7} \) in risk assessment models or when analyzing market volatility.
5. Computer Graphics: In computer graphics, \( \sqrt{7} \) helps determine pixel distances and aspect ratios in digital imaging and display technologies.

Here are some frequently asked questions about the square root of 7:

1. What is the square root of 7?

   The square root of 7, denoted as \( \sqrt{7} \), is an irrational number approximately equal to 2.6457513110645906.

2. Can the square root of 7 be simplified?

   No, \( \sqrt{7} \) cannot be simplified further because 7 is a prime number.

3. What are the practical uses of \( \sqrt{7} \)?

   \( \sqrt{7} \) is used in various applications such as geometry, physics, engineering, finance, and computer graphics for precise calculations involving distances, areas, frequencies, and more.

4. How do you calculate \( \sqrt{7} \)?

   There are several methods to calculate \( \sqrt{7} \), including iterative methods, using scientific calculators, or approximating through long division.

5. Is \( \sqrt{7} \) an integer?

   No, \( \sqrt{7} \) is not an integer; it is an irrational number.

6. Where does \( \sqrt{7} \) fall on the number line?

   \( \sqrt{7} \) falls between 2 and 3 on the number line, closer to 2.6457513110645906.

In conclusion, the square root of 7, \( \sqrt{7} \), is an important mathematical constant with several notable characteristics:

• It is an irrational number, meaning its decimal representation is non-repeating and goes on indefinitely.
• \( \sqrt{7} \) cannot be simplified further because 7 is a prime number.
• It has various practical applications in fields such as geometry, physics, engineering, finance, and computer graphics.
• Calculating \( \sqrt{7} \) can be done using iterative methods, scientific calculators, or approximating through long division.

Understanding \( \sqrt{7} \) not only enhances mathematical knowledge but also facilitates accurate calculations in real-world scenarios.