Topic is the square root of 7 a real number: The square root of 7 is indeed a real number. In mathematics, it is an irrational number that cannot be expressed as a simple fraction. This article explores the properties, calculation methods, and significance of the square root of 7, providing a comprehensive understanding of this fascinating mathematical concept.
Table of Content
- Understanding the Square Root of 7
- Introduction
- Definition and Properties of Square Roots
- Understanding the Square Root of 7
- Methods to Calculate the Square Root of 7
- Long Division Method
- Newton's Method
- Approximation Techniques
- Mathematical Proofs and Examples
- Geometric Representation
- Historical Context
- Applications in Real Life
- Frequently Asked Questions
- Related Topics
- YOUTUBE: Tìm hiểu về căn bậc hai cùng thầy J. Video này sẽ giúp bạn hiểu rõ khái niệm căn bậc hai và cách áp dụng nó trong toán học.
Understanding the Square Root of 7
The square root of 7 is an interesting number with various properties and applications in mathematics. Below, we explore its characteristics, how to find its value, and its significance in different contexts.
Mathematical Properties
- Radical Form: √7
- Exponential Form: 71/2
- Decimal Approximation: ±2.6457513111
Rationality
The square root of 7 is an irrational number. This means it cannot be expressed as a simple fraction of two integers. In other words, there are no integers p and q such that √7 = p/q. The decimal expansion of √7 is non-terminating and non-repeating.
How to Calculate
Several methods can be used to calculate √7:
- Babylonian Method: An iterative process that converges to the square root by averaging guesses.
- Newton's Method: Another iterative method that refines guesses using derivatives.
- Calculator: Most modern calculators and online tools can provide a precise value of √7.
Geometric Interpretation
In geometry, the square root of 7 can be visualized as the length of the diagonal of a rectangle with sides 1 and √6. This geometric approach provides a visual and intuitive understanding of the number.
Applications and Examples
| Example | Solution |
|---|---|
| Pizza Radius: The area of a pizza is 22 square units. What is the radius? | Area = πr2 = 22. Solving for r gives r = √(22/π) ≈ 2.645. |
| Right Triangle: In a right-angled triangle with legs √3 and 2, find the hypotenuse. | Hypotenuse = √( (√3)2 + 22 ) = √(3 + 4) = √7 ≈ 2.645. |
FAQs
- Is the square root of 7 a real number? Yes, √7 is a real number as it exists on the number line.
- Is the square root of 7 a natural number? No, it is not a natural number as it is not a whole number.
- Is the square root of 7 a whole number? No, it is not a whole number as it is not an integer.
Understanding the properties and applications of the square root of 7 enhances our knowledge of irrational numbers and their role in various mathematical and real-world contexts.

READ MORE:
Introduction
The square root of 7, denoted as \( \sqrt{7} \), is a significant number in mathematics. It is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating. In this section, we will explore the properties of \( \sqrt{7} \), its classification among different sets of numbers, and methods to approximate its value.
Definition and Properties of Square Roots
The square root of a number \(x\) is a value that, when multiplied by itself, gives \(x\). It is denoted as \(\sqrt{x}\). For example, the square root of 9 is 3 because \(3 \times 3 = 9\). The square root function is a type of radical function.
Square roots have several important properties:
- Positive and Negative Roots: Every positive real number \(x\) has two square roots: \(\sqrt{x}\) (positive root) and \(-\sqrt{x}\) (negative root). However, when we refer to "the square root," we typically mean the principal (positive) square root.
- Perfect Squares: If \(x\) is a perfect square (e.g., 1, 4, 9, 16), its square root is an integer. If \(x\) is not a perfect square, its square root is an irrational number.
- Irrational Numbers: The square root of a number that is not a perfect square is irrational. This means it cannot be expressed as a ratio of two integers and its decimal expansion is non-terminating and non-repeating. For example, \(\sqrt{7}\) is irrational.
- Real Numbers: The square root of any non-negative real number is also a real number. This includes both rational and irrational numbers.
For instance, \(\sqrt{7}\) is an irrational number because it cannot be expressed as a fraction of two integers. It is approximately equal to 2.6457513110645906...
Another important aspect of square roots involves solving quadratic equations. The solutions to the equation \(x^2 = a\) are \(x = \pm \sqrt{a}\), where \(a\) is a positive real number.
In summary, the square root function plays a crucial role in mathematics, especially in the fields of algebra and geometry, and understanding its properties is fundamental to grasping more complex mathematical concepts.
Understanding the Square Root of 7
The square root of 7, denoted as \( \sqrt{7} \), is a fascinating number that holds a unique place in mathematics. Here, we will delve into its properties and significance.
Real Number:
The square root of 7 is a real number. Real numbers include all the rational and irrational numbers, and \( \sqrt{7} \) fits into this category because it can be placed on the number line.
Irrational Number:
\( \sqrt{7} \) is also an irrational number. This means it cannot be expressed as a simple fraction \( \frac{p}{q} \), where \( p \) and \( q \) are integers with \( q \neq 0 \). The decimal expansion of \( \sqrt{7} \) is non-repeating and non-terminating, approximately 2.6457513111...
Mathematical Proof of Irrationality:
To understand why \( \sqrt{7} \) is irrational, assume the contrary, that \( \sqrt{7} \) is rational and can be expressed as \( \frac{p}{q} \) in its simplest form. Then, we can write:
\[
\sqrt{7} = \frac{p}{q}
\]
Squaring both sides gives:
\[
7 = \frac{p^2}{q^2} \implies p^2 = 7q^2
\]
This implies \( p^2 \) is divisible by 7, and hence \( p \) must also be divisible by 7. Let \( p = 7k \) for some integer \( k \). Substituting back, we get:
\[
(7k)^2 = 7q^2 \implies 49k^2 = 7q^2 \implies q^2 = 7k^2
\]
This implies \( q^2 \) is divisible by 7, and hence \( q \) must also be divisible by 7. This contradicts the assumption that \( \frac{p}{q} \) is in simplest form. Therefore, \( \sqrt{7} \) cannot be rational and must be irrational.
Geometric Representation:
Geometrically, \( \sqrt{7} \) can be visualized as the length of the diagonal of a rectangle with sides of length \( \sqrt{6} \) and 1, based on the Pythagorean theorem.
Value and Approximation:
The principal square root of 7 is approximately 2.6457513111. This value can be approximated using various numerical methods such as the Babylonian method or Newton's method.
Properties:
- Non-terminating and non-repeating decimal: The decimal form of \( \sqrt{7} \) goes on forever without repeating.
- Positive and negative roots: While \( \sqrt{7} \) typically refers to the positive root (principal square root), mathematically, \( \sqrt{7} \) has both positive and negative roots: \( \pm 2.6457513111 \).
Understanding \( \sqrt{7} \) helps in appreciating the broader concepts of real numbers and their properties in mathematics.
Methods to Calculate the Square Root of 7
To calculate the square root of 7, several methods can be employed:
- Estimation by Hand: Start with an initial guess close to the actual value of √7 and refine it using approximation techniques.
- Long Division Method: Divide 7 by increasingly accurate approximations of the square root until the desired precision is achieved.
- Newton's Method: Iteratively refine an initial guess based on the function f(x) = x^2 - 7 until the root is found.
- Approximation Techniques: Utilize methods like the Babylonian method or continued fractions to approximate √7 with increasing precision.
Each method has its advantages depending on the required accuracy and computational resources available.
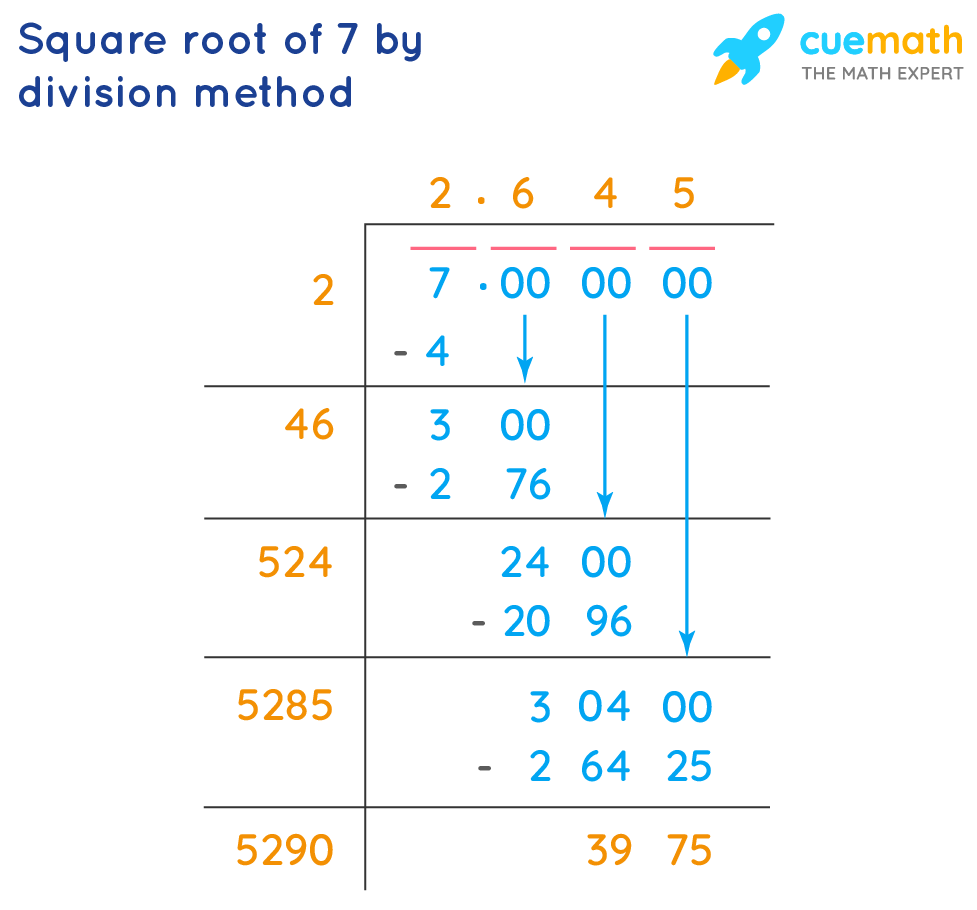
Long Division Method
The long division method is a manual technique for finding the square root of a number. Below are the steps to calculate the square root of 7 using the long division method:
Pair the digits: Write the number 7 and pair the digits starting from the decimal point. Since 7 is a single digit, we can consider it as 7.000000 for our calculations.
Determine the largest number: Find the largest number whose square is less than or equal to 7. This number is 2 because \(2^2 = 4\) and \(3^2 = 9\).
Set up the division: Place 2 as the first digit of the quotient. Subtract \(2^2 = 4\) from 7, leaving a remainder of 3. Bring down two zeros to get 300.
Double the quotient: Double the quotient (which is 2) to get 4. This will be used as part of the divisor for the next step.
Find the next digit: Find a digit (let's call it X) such that \(4X \times X\) is less than or equal to 300. The digit 7 works because \(47 \times 7 = 329\) is too large, but \(46 \times 6 = 276\) is acceptable.
Subtract and bring down zeros: Place 6 in the quotient, making it 2.6. Subtract 276 from 300 to get 24. Bring down two more zeros to get 2400.
Repeat the process: Continue the process by doubling the quotient (now 26) to get 52, and find a new digit X such that \(520X \times X\) is less than or equal to 2400.
Continue the steps: Repeat the above steps to get more digits of the square root. Each time, double the current quotient, find the appropriate digit, subtract, and bring down the next pair of zeros.
Following these steps, we can approximate the square root of 7 to several decimal places. The more steps we follow, the more accurate the result.
| Step | Quotient | Divisor | Remainder | Next Digits |
|---|---|---|---|---|
| 1 | 2 | 4 | 3 | 300 |
| 2 | 2.6 | 46 | 24 | 2400 |
| 3 | 2.64 | 528 | 192 | 19200 |
| 4 | 2.645 | 5290 | 610 | 61000 |
This method demonstrates how the long division technique can be used to manually approximate the square root of a number, such as 7, to any desired level of precision.
Newton's Method
Newton's Method, also known as the Newton-Raphson Method, is an efficient iterative approach to finding successively better approximations to the roots (or zeroes) of a real-valued function. This method can be effectively used to approximate the square root of 7.
To apply Newton's Method for finding \(\sqrt{7}\), we start with an initial guess and iteratively improve this guess using the formula:
\( x_{n+1} = \frac{1}{2} \left( x_n + \frac{7}{x_n} \right) \)
Here's a step-by-step outline of the process:
- Initial Guess: Start with an initial guess \(x_0\). A reasonable starting point is \(x_0 = 2.5\) since \(2.5^2 = 6.25\), which is close to 7.
- First Iteration: Apply the Newton's Method formula to get a better approximation.
\( x_1 = \frac{1}{2} \left( 2.5 + \frac{7}{2.5} \right) = \frac{1}{2} \left( 2.5 + 2.8 \right) = \frac{1}{2} \left( 5.3 \right) = 2.65 \)
- Second Iteration: Use \(x_1 = 2.65\) and apply the formula again.
\( x_2 = \frac{1}{2} \left( 2.65 + \frac{7}{2.65} \right) = \frac{1}{2} \left( 2.65 + 2.6415 \right) = \frac{1}{2} \left( 5.2915 \right) \approx 2.64575 \)
- Third Iteration: Use \(x_2 = 2.64575\) for further refinement.
\( x_3 = \frac{1}{2} \left( 2.64575 + \frac{7}{2.64575} \right) \approx \frac{1}{2} \left( 2.64575 + 2.6457513 \right) \approx 2.6457513111 \)
After three iterations, we find that \(x_3 \approx 2.6457513111\), which is a very close approximation to \(\sqrt{7}\). With each iteration, the value of \(x_n\) becomes closer to the actual square root of 7.
Newton's Method is particularly useful because it converges quickly. Each iteration roughly doubles the number of correct digits, making it a powerful technique for finding square roots and solving other mathematical problems.
Approximation Techniques
Approximating the square root of 7 involves several techniques, each providing a different level of accuracy and computational effort. Here are some commonly used methods:
1. Estimation using Nearby Perfect Squares
To begin, identify the perfect squares closest to 7. These are 4 (since \(2^2 = 4\)) and 9 (since \(3^2 = 9\)). Thus, the square root of 7 lies between 2 and 3.
2. Average Method
The average method involves finding a better approximation by averaging two estimates:
- Start with two initial guesses. For example, take 2 and 3.
- Calculate the average: \(\frac{2 + 3}{2} = 2.5\).
- Square 2.5: \(2.5^2 = 6.25\). Since 6.25 is less than 7, take the higher end of the range for the next guess.
- Average 2.5 and 3: \(\frac{2.5 + 3}{2} = 2.75\).
- Square 2.75: \(2.75^2 = 7.5625\). Since 7.5625 is more than 7, take the lower end of the range for the next guess.
- Continue this process until the desired precision is reached. This iterative method narrows down the estimate effectively.
3. Long Division Method
The long division method is a manual technique that provides a more precise result:
- Start by pairing the digits of the number from the decimal point. For 7, it’s written as 7.0000...
- Determine the largest number whose square is less than or equal to the first pair (7 in this case). The closest is 2 since \(2^2 = 4\).
- Write 2 as the first digit of the square root. Subtract 4 from 7 to get a remainder of 3, then bring down the next pair of zeros, making it 300.
- Double the current result (2), getting 4. Use 40 as the new divisor and find the largest digit \(x\) such that \(40x \cdot x \leq 300\). This digit is 6, as \(46 \cdot 6 = 276\).
- Subtract 276 from 300 to get 24, then bring down the next pair of zeros, making it 2400.
- Repeat the process, doubling the quotient (2.6) to get 52, and using 520 as the new divisor. Find the digit \(x\) such that \(520x \cdot x \leq 2400\). Continue until the desired precision is reached.
- The quotient gives the approximate value of the square root of 7. Following these steps yields approximately 2.645.
4. Using a Calculator
For a quick and accurate result, a calculator can be used. Enter the number 7 and use the square root function to obtain a result of approximately 2.64575.
5. Continued Fractions
Another advanced technique is using continued fractions. The square root of 7 can be expressed as a continued fraction which provides an increasingly accurate approximation with each step.
Conclusion
By using these approximation techniques, one can find a value close to the actual square root of 7. Each method varies in complexity and accuracy, allowing for flexibility based on the required precision.
Mathematical Proofs and Examples
The square root of 7, denoted as \( \sqrt{7} \), is a real number. This section provides mathematical proofs and examples to illustrate its properties and the methods to calculate it.
Proof that \( \sqrt{7} \) is a Real Number:
- Definition of Real Numbers: Real numbers include all the numbers that can be found on the number line. This includes both rational numbers (such as integers and fractions) and irrational numbers (numbers that cannot be expressed as a simple fraction).
- Square Roots of Positive Numbers: Any positive real number has a real square root. Since 7 is a positive real number, \( \sqrt{7} \) must also be a real number.
- Irrationality of \( \sqrt{7} \): \( \sqrt{7} \) is irrational because it cannot be expressed as a ratio of two integers. This can be proven using a proof by contradiction:
- Assume \( \sqrt{7} \) is rational, meaning \( \sqrt{7} = \frac{a}{b} \) for some integers \( a \) and \( b \) with no common factors other than 1.
- Then \( 7 = \frac{a^2}{b^2} \), leading to \( a^2 = 7b^2 \).
- This implies \( a^2 \) is a multiple of 7, so \( a \) must also be a multiple of 7 (since 7 is prime).
- Let \( a = 7k \) for some integer \( k \). Then \( (7k)^2 = 49k^2 \), so \( 49k^2 = 7b^2 \) leading to \( 7k^2 = b^2 \).
- This implies \( b^2 \) is also a multiple of 7, so \( b \) must be a multiple of 7.
- But this contradicts our assumption that \( a \) and \( b \) have no common factors other than 1. Thus, \( \sqrt{7} \) is irrational.
Examples of Calculating \( \sqrt{7} \):
- Using Approximation Techniques: By estimation or numerical methods, we can find that:
- \( \sqrt{7} \approx 2.6457513110645906 \).
- Using the Long Division Method: This method can be used to find more precise decimal values of \( \sqrt{7} \):
- Pair the digits of 7 from right to left. Since 7 has only one digit, we treat it as 07.
- Find the largest number whose square is less than or equal to 7. This is 2, because \( 2^2 = 4 \).
- Subtract 4 from 7, leaving a remainder of 3. Bring down two zeros to make 300.
- Double the 2 to get 4. Find the largest digit \( x \) such that \( 40x \times x \) is less than or equal to 300. This digit is 6, because \( 406 \times 6 = 2436 \), which is less than 3000.
- Continue the process to obtain more digits of \( \sqrt{7} \).
- Using Newton's Method: This iterative method provides an efficient way to approximate \( \sqrt{7} \):
- Start with an initial guess, \( x_0 \). A reasonable guess is \( x_0 = 3 \).
- Apply the formula \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{7}{x_n} \right) \).
- For \( x_0 = 3 \):
- First iteration: \( x_1 = \frac{1}{2} \left( 3 + \frac{7}{3} \right) = 2.8333 \).
- Second iteration: \( x_2 = \frac{1}{2} \left( 2.8333 + \frac{7}{2.8333} \right) \approx 2.6458 \).
- Continue iterating to reach a desired level of accuracy.
Geometric Interpretation: The value of \( \sqrt{7} \) can also be visualized geometrically as the length of the diagonal in a rectangle with side lengths of 1 and \( \sqrt{7} \), which is a solution to the Pythagorean theorem for right triangles.
Geometric Representation
The geometric representation of the square root of 7 can be visualized using the properties of right triangles and the Pythagorean theorem. Let's explore a couple of methods to understand this concept.
1. Right Triangle Method
We can represent \( \sqrt{7} \) geometrically by constructing a right triangle. In a right triangle, the lengths of the sides are related by the Pythagorean theorem:
Here, \( c \) is the hypotenuse, and \( a \) and \( b \) are the legs of the triangle. To represent \( \sqrt{7} \), consider a triangle where one leg is 1 and the other is \( \sqrt{6} \). The hypotenuse of this triangle will be:
Therefore, the hypotenuse is \( \sqrt{7} \).
2. Number Line Representation
Another way to visualize \( \sqrt{7} \) is by placing it on a number line. To do this, we can use a geometric compass:
- Draw a line segment \( AB \) of length 7 units on a number line.
- Construct a perpendicular line at point \( A \) and mark a point \( C \) such that \( AC = 1 \) unit.
- Draw a circle with center \( C \) and radius \( AC \). This circle will intersect the line \( AB \) at a point \( D \).
- The length of the segment \( AD \) will be \( \sqrt{7} \).
3. Visualizing in Coordinate System
We can also represent \( \sqrt{7} \) using a circle in a coordinate system. Draw a circle with a radius of \( \sqrt{7} \) centered at the origin (0,0). The distance from the center to any point on the circumference will be \( \sqrt{7} \).
For example, the point ( \( \sqrt{7}, 0 \) ) lies on this circle.
4. Spiral Method
A more complex but interesting geometric representation is through the use of a spiral. By constructing a spiral with right triangles, each subsequent triangle's hypotenuse represents the square root of an incrementing integer. The hypotenuse of the fourth triangle in this series will give us \( \sqrt{7} \).
Start with a right triangle with legs 1 and 2, then build subsequent triangles on each hypotenuse:
- First triangle: sides 1 and 2, hypotenuse \( \sqrt{3} \).
- Second triangle: legs \( \sqrt{3} \) and 2, hypotenuse \( \sqrt{7} \).
Historical Context
The concept of square roots, including the square root of 7, has a rich historical background that dates back to ancient civilizations. Understanding how these concepts evolved provides a deeper appreciation of their significance in mathematics.
1. Ancient Civilizations
- Babylonian Mathematics:
The Babylonians, around 2000 BCE, were among the first to develop methods for approximating square roots. Using a numerical algorithm similar to the modern-day Newton's method, they could find close approximations for square roots, including the square root of non-perfect squares like 7.
Babylonian tablets show approximations of square roots using a base-60 (sexagesimal) system. For example, they might have approximated \( \sqrt{7} \) by a series of calculations involving fractions of their base unit.
- Ancient Greeks:
The Greeks, particularly through the work of Pythagoras and Euclid, advanced the understanding of irrational numbers, which include \( \sqrt{7} \). Euclid's "Elements" provided a formal proof that not all square roots are rational, laying the groundwork for later mathematical exploration of irrational numbers.
Euclid's geometric approach to square roots involved constructing lengths using compass and straightedge, showing that the diagonal of a square with side length \( \sqrt{7} \) is indeed an irrational number.
2. Middle Ages and Islamic Mathematics
- Islamic Golden Age:
During the Islamic Golden Age (8th to 14th centuries), mathematicians like Al-Khwarizmi expanded on Greek and Indian mathematical concepts, including the work on square roots. They developed more sophisticated methods for calculating square roots and dealing with irrational numbers.
Al-Khwarizmi's work in algebra, from which the word 'algorithm' is derived, provided systematic methods for solving quadratic equations, which inherently involve square roots.
- European Renaissance:
Mathematicians in the Renaissance period, such as Fibonacci, continued to build on these earlier works. Fibonacci’s "Liber Abaci" introduced the Hindu-Arabic numeral system to Europe and included methods for calculating square roots.
The development of algebra and analytic geometry during this time further solidified the understanding and calculation of irrational square roots like \( \sqrt{7} \).
3. Modern Era
- Advancements in Calculus:
With the advent of calculus in the 17th century by Newton and Leibniz, mathematicians gained powerful tools to approximate square roots with great precision. Newton's method, for instance, iteratively refines estimates of square roots, making it possible to compute \( \sqrt{7} \) to many decimal places efficiently.
- Computational Methods:
In the 20th and 21st centuries, computational advances have allowed for the precise calculation of square roots. Computers can now calculate \( \sqrt{7} \) to millions of decimal places within seconds. This precision is crucial in fields like cryptography, numerical analysis, and scientific computing.
4. Cultural and Mathematical Significance
- In Mathematical Theory:
The study of square roots, including \( \sqrt{7} \), continues to play a fundamental role in number theory, algebra, and geometry. Understanding the properties of irrational numbers is essential in various branches of mathematics and its applications.
- In Education:
The concept of square roots is a key topic in mathematics education worldwide. It introduces students to the idea of irrational numbers and serves as a stepping stone to more advanced topics in mathematics.
Applications in Real Life
The square root of 7 (\( \sqrt{7} \)) is not just an abstract mathematical concept; it has various applications in real-world scenarios. From geometry and engineering to natural phenomena and technology, understanding and using \( \sqrt{7} \) is crucial in several fields.
1. Geometry and Construction
In geometry, the square root of 7 often appears in calculations involving distances and angles:
- Diagonals and Areas: When calculating the diagonal of a rectangular prism or a multi-dimensional object, \( \sqrt{7} \) can emerge naturally. For instance, in a right triangle with legs of 1 and \( \sqrt{6} \), the hypotenuse is \( \sqrt{7} \).
- Architectural Design: Certain design elements and structural components may involve lengths that simplify to \( \sqrt{7} \) when considering proportions and symmetry. Understanding these lengths helps in precise measurements and construction.
2. Engineering and Physics
In engineering and physics, the square root of 7 is used in various computations and analyses:
- Wave Mechanics: In the study of wave frequencies and their harmonics, \( \sqrt{7} \) can appear in equations modeling the behavior of waves under specific conditions. It helps in understanding phenomena like resonance and standing waves.
- Structural Engineering: When analyzing forces and stresses in structures, engineers often use principles that involve irrational numbers like \( \sqrt{7} \). For instance, the bending of beams or the dynamics of oscillating systems may require such calculations.
3. Natural Phenomena
The square root of 7 also appears in natural patterns and formations:
- Crystal Structures: Certain crystals and minerals exhibit atomic spacing or angles that involve \( \sqrt{7} \). Understanding these structures aids in the study of material properties and their applications in technology.
- Fractal Geometry: In the study of fractals and natural patterns, \( \sqrt{7} \) can be part of the scaling factors or dimensions that describe the complexity of these shapes.
4. Technology and Computing
In technology, the square root of 7 plays a role in algorithms and data analysis:
- Algorithm Design: In computational algorithms, especially those involving numerical methods and optimizations, \( \sqrt{7} \) might be used to find solutions or to simplify complex calculations.
- Cryptography: The principles involving irrational numbers and their properties, including \( \sqrt{7} \), are foundational in developing secure cryptographic systems. These principles help in creating robust encryption methods.
5. Art and Music
Even in the realms of art and music, the square root of 7 has its place:
- Musical Scales: In the tuning and spacing of musical scales, mathematical ratios involving square roots help in defining intervals and harmonics. The use of \( \sqrt{7} \) contributes to the creation of unique tonal qualities and tuning systems.
- Visual Art: Artists and designers often use geometric principles that involve irrational numbers like \( \sqrt{7} \) to create aesthetically pleasing and balanced compositions.
In summary, \( \sqrt{7} \) is more than just a mathematical curiosity. Its applications span various domains, providing solutions and insights that are integral to both theoretical and practical endeavors in our daily lives.
Frequently Asked Questions
Below are some commonly asked questions about the square root of 7 (\( \sqrt{7} \)) and its properties. These questions help to clarify the nature and applications of this mathematical constant.
- Is the square root of 7 a real number?
Yes, the square root of 7 is a real number. In mathematics, a real number is any value that can represent a distance along a continuous number line. Since 7 is a positive number, its square root is also a real number.
- Is the square root of 7 a rational number?
No, the square root of 7 is not a rational number. A rational number is any number that can be expressed as a ratio of two integers (e.g., \( \frac{a}{b} \) where \( a \) and \( b \) are integers and \( b \neq 0 \)). Since \( \sqrt{7} \) cannot be expressed as such a ratio, it is classified as an irrational number.
- How can we approximate the square root of 7?
There are several methods to approximate \( \sqrt{7} \):
- Decimal Approximation: Using a calculator, \( \sqrt{7} \) can be approximated as 2.6457513110645906...
- Long Division Method: This manual method involves a step-by-step process of estimating and refining the value of \( \sqrt{7} \).
- Newton's Method: Also known as the Newton-Raphson method, it provides an iterative way to approach the square root by using the formula:
- Why is the square root of 7 important in mathematics?
The square root of 7 is important because it is an example of an irrational number that often appears in various mathematical contexts, such as geometry and number theory. Understanding such numbers helps in studying more complex mathematical structures and concepts.
- How is the square root of 7 used in geometry?
In geometry, \( \sqrt{7} \) can be used to find the lengths of sides in right triangles, the diagonal of rectangles, or in constructing certain geometric shapes. For example, if one side of a right triangle is 1 and another is \( \sqrt{6} \), the hypotenuse would be \( \sqrt{7} \).
- Can the square root of 7 be expressed as a fraction?
No, \( \sqrt{7} \) cannot be exactly expressed as a fraction. It is an irrational number, meaning it cannot be written as a simple fraction. However, it can be approximated by fractions to a desired level of precision.
- How do you represent the square root of 7 on a number line?
To represent \( \sqrt{7} \) on a number line, you can start at 0 and move right to approximately 2.645, placing a point at this position. Alternatively, using geometric tools, you can construct a segment whose length is \( \sqrt{7} \) and place it on the number line.
- Are there any applications of the square root of 7 in physics?
Yes, \( \sqrt{7} \) appears in various physics problems, particularly in wave mechanics and quantum mechanics where certain equations involve square roots of numbers. It helps in describing properties such as energy levels, wave frequencies, and distances in physical systems.
- What role does the square root of 7 play in higher mathematics?
In higher mathematics, \( \sqrt{7} \) is used in algebraic equations, calculus, and complex number theory. It serves as an example to illustrate the properties of irrational numbers and helps in solving polynomial equations and understanding functions that involve square roots.
- How do you find the square root of 7 without a calculator?
Finding \( \sqrt{7} \) without a calculator can be done using methods like:
- Long Division Method: This involves estimating and refining the square root step by step.
- Newton's Method: This iterative method starts with a guess and improves the approximation using the formula:

Related Topics
Exploring the square root of 7 opens up a variety of related mathematical topics and concepts. Here are some key areas that are closely linked to the square root of 7:
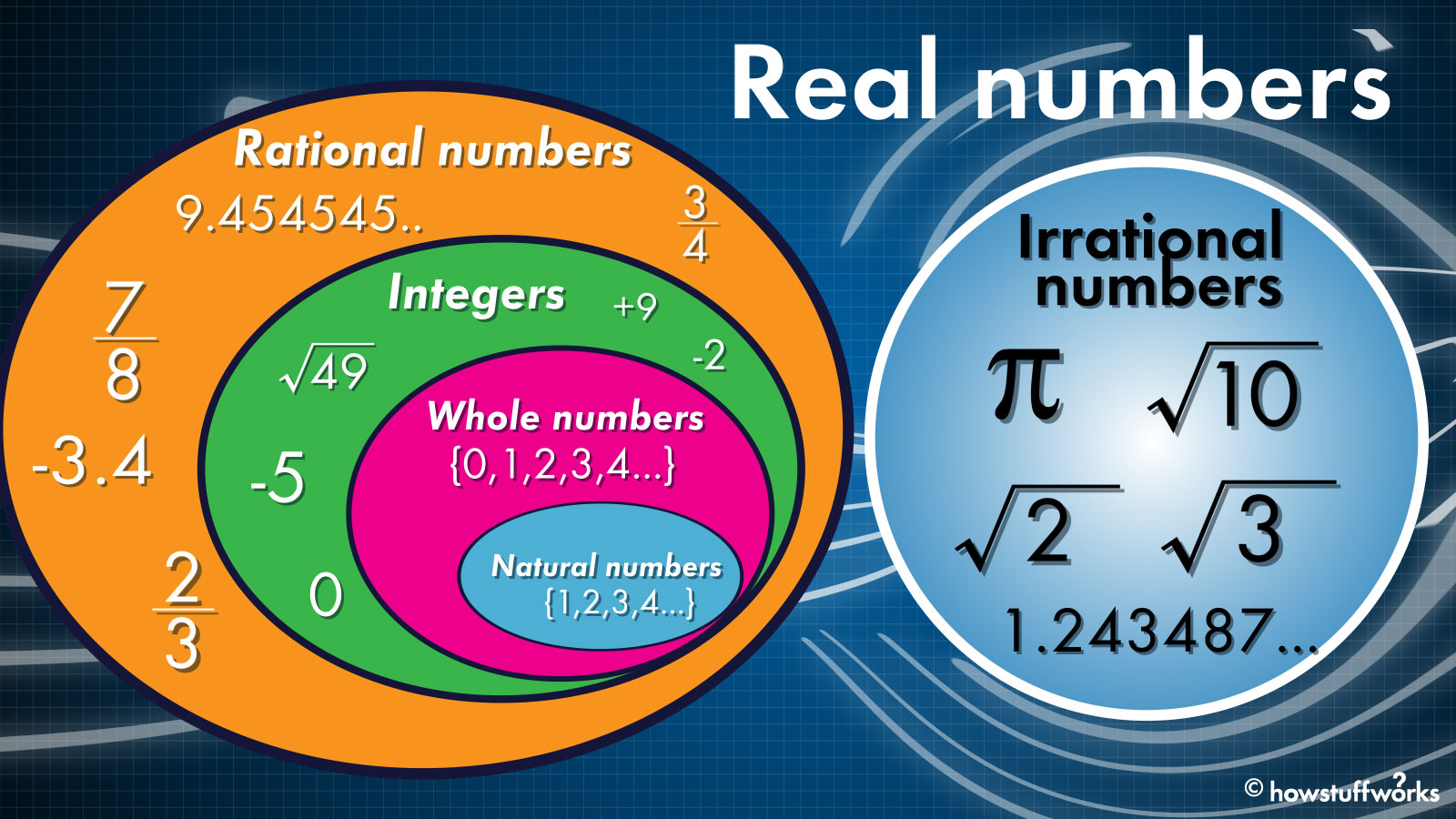
- Real Numbers: The square root of 7 is a real number. Real numbers include both rational and irrational numbers, and they form the continuum of numbers that we use in everyday life.
- Irrational Numbers: The square root of 7 is an irrational number, which means it cannot be expressed as a simple fraction. Other examples of irrational numbers include √2, √3, and π.
- Square Roots: Understanding the properties and calculation methods of square roots is essential for grasping the concept of the square root of 7. This includes methods such as long division, Newton's method, and approximation techniques.
- Algebraic Numbers: The square root of 7 is also an algebraic number, as it is a root of the polynomial x² - 7 = 0.
- Decimal Expansion: The square root of 7, like other irrational numbers, has a non-repeating and non-terminating decimal expansion. For example, √7 ≈ 2.6457513110645906...
- Pythagorean Theorem: The concept of square roots is deeply connected to the Pythagorean theorem, which relates the sides of a right triangle. The square root of 7 can be part of a Pythagorean triplet in geometric problems.
- Complex Numbers: While the square root of 7 is a real number, the study of square roots in general leads to the concept of complex numbers, where square roots of negative numbers are considered.
- Number Theory: The properties and classifications of numbers, such as prime numbers, composite numbers, and their factors, are closely related to the study of square roots.
- Historical Context: The understanding and calculation of square roots have a rich history in mathematics, dating back to ancient civilizations that developed methods to approximate these values.
- Applications in Real Life: Square roots, including the square root of 7, have numerous applications in science, engineering, and everyday problem-solving.
Each of these topics provides a deeper insight into the mathematical principles and applications of the square root of 7, highlighting its significance and the broader context in which it exists.
Tìm hiểu về căn bậc hai cùng thầy J. Video này sẽ giúp bạn hiểu rõ khái niệm căn bậc hai và cách áp dụng nó trong toán học.
Căn Bậc Hai Là Gì? | Toán Học Với Thầy J
READ MORE:
Khám phá về số thực và cách chúng được sử dụng trong toán học. Video này sẽ giúp bạn hiểu rõ về khái niệm số thực.
Số Thực