Topic square root of 2 x square root of 2: The square root of 2 multiplied by the square root of 2 yields the number 2. This fascinating property has significant implications in various fields of mathematics and its practical applications. Understanding this basic yet intriguing mathematical fact can provide deeper insights into algebra and geometry, and help solve complex mathematical problems more efficiently.
Table of Content
- Understanding the Square Root of 2
- Introduction
- Basic Concept
- Calculation Methods
- Properties of Square Roots
- Mathematical Applications
- Examples and Problem Solving
- FAQs on Square Roots
- Additional Resources
- YOUTUBE: Video này giới thiệu về cách tính toán căn bậc hai của 2x^2 và 10x, cùng với việc nhân chúng lại với nhau. Hãy cùng tìm hiểu cách giải quyết bài toán này một cách chi tiết.
Understanding the Square Root of 2
The mathematical expression "square root of 2 x square root of 2" is a fundamental concept that often appears in various mathematical problems and solutions. This article aims to provide a comprehensive explanation of this expression, using MathJax for clarity.
Mathematical Expression
The expression can be simplified as follows:
Steps to Simplify
- Identify the square roots: and .
- Multiply the values under the square roots: .
- Take the square root of the result: .
Applications
This concept is not only important in basic arithmetic but also in more advanced fields like algebra and calculus. Understanding how to simplify square roots is crucial for solving quadratic equations, integrating functions, and more.
Interactive Example
Here is an example to help illustrate the concept:
Consider the expression Following the steps above:
- Multiply the values: .
- Find the square root of the result: .
Conclusion
Understanding how to simplify the expression is fundamental in mathematics. This concept is widely used across different mathematical fields and provides a basis for more complex calculations and problem-solving techniques.
Explore more about square roots and their applications through various online calculators and interactive tools available on educational websites.
READ MORE:
Introduction
The calculation of the square root of 2 times the square root of 2 is a fundamental mathematical concept that serves as an excellent example of the properties of square roots. Mathematically, this expression can be simplified using basic principles of algebra and square roots.
To begin with, the square root function has a key property: for any non-negative numbers a and b, the square root of their product is the product of their square roots. This is expressed as:
\[ \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \]
Applying this property to our expression, we have:
\[ \sqrt{2} \times \sqrt{2} \]
Using the property, we can combine the square roots:
\[ \sqrt{2} \times \sqrt{2} = \sqrt{2 \times 2} = \sqrt{4} \]
Since the square root of 4 is 2, we obtain:
\[ \sqrt{2} \times \sqrt{2} = 2 \]
This simple yet powerful example demonstrates how understanding the properties of square roots can simplify expressions and solve mathematical problems efficiently. Whether you are a student learning basic algebra or someone revisiting these concepts, mastering the manipulation of square roots is a valuable skill.
Basic Concept
The basic concept of multiplying square roots can be understood using the properties of square roots and multiplication. Specifically, the square root of a product is equal to the product of the square roots. This principle can be applied to simplify the expression √2 × √2.
Let's break down the steps:
- Step 1: Identify the expression to be simplified: \( \sqrt{2} \times \sqrt{2} \).
- Step 2: Apply the property of square roots: \( \sqrt{a} \times \sqrt{b} = \sqrt{a \times b} \). In this case, \( a = 2 \) and \( b = 2 \).
- Step 3: Simplify the expression: \( \sqrt{2 \times 2} = \sqrt{4} \).
- Step 4: Calculate the square root of the resulting number: \( \sqrt{4} = 2 \).
Thus, \( \sqrt{2} \times \sqrt{2} = 2 \).
| Expression | Simplification | Result |
| √2 × √2 | √(2 × 2) | √4 |
| √4 | 2 | 2 |
This process demonstrates that multiplying two square roots of the same number results in the original number, emphasizing the fundamental property of square roots in algebra.
Calculation Methods
Calculating the square root of a number can be done using various methods. Below, we detail some common techniques for finding the value of √2 x √2:
-
Prime Factorization Method: This method involves breaking down the number into its prime factors and then simplifying. For example, the prime factorization of 2 is already a prime number, so we consider it as √2 directly. When √2 is multiplied by itself, the result is 2:
\[
\sqrt{2} \times \sqrt{2} = \sqrt{2 \times 2} = \sqrt{4} = 2
\] -
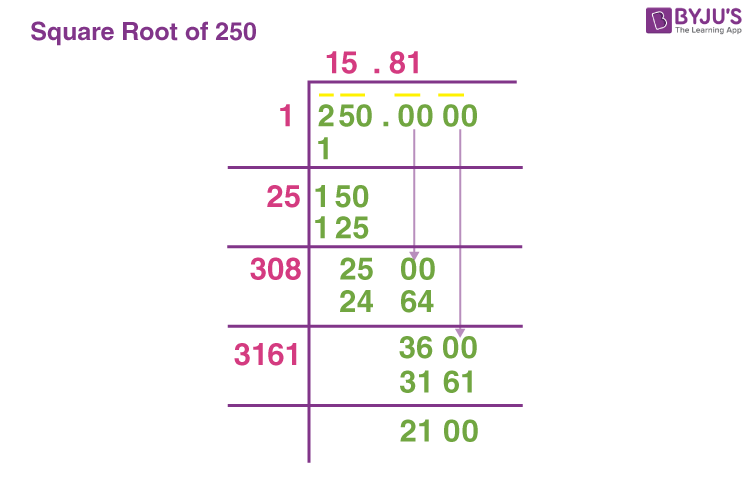
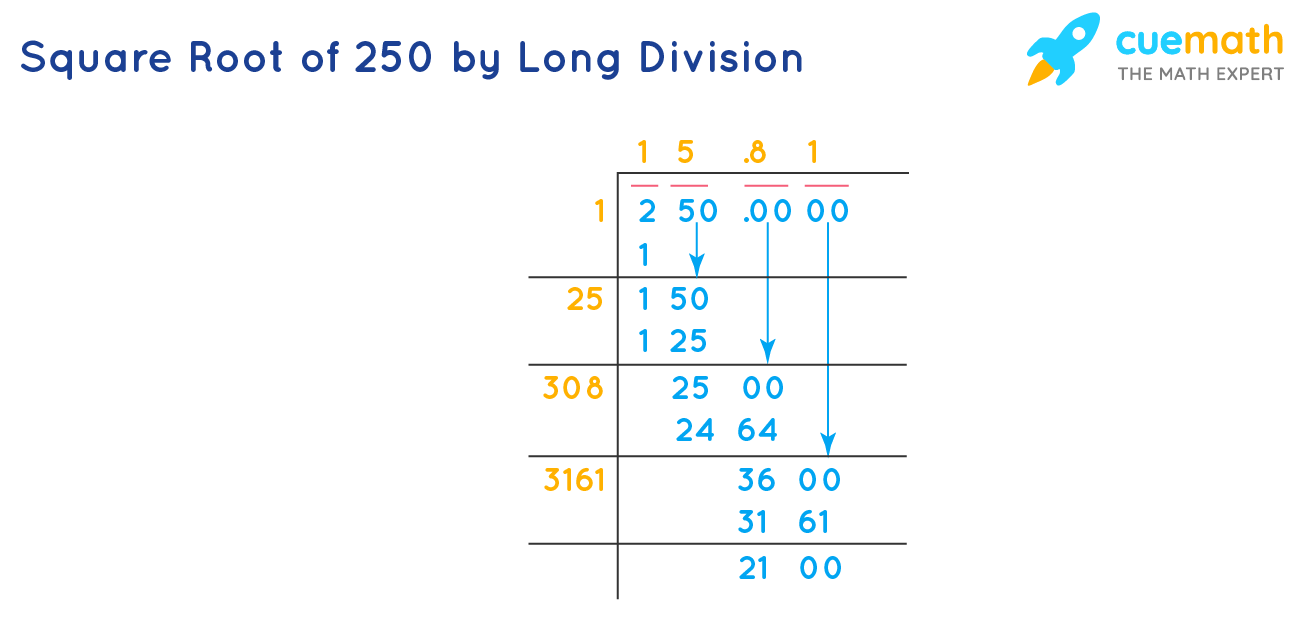
Long Division Method: This is a more traditional method where the number is divided into pairs of digits from right to left, and the square root is calculated digit by digit. This method is generally used for more complex numbers and less often for simple cases like √2:
The steps involve estimating, dividing, and averaging to refine the guess for the square root. For example, finding the square root of 2 through long division will ultimately show that:
\[
\sqrt{2} \times \sqrt{2} = 2
\] -
Estimation Method: This method involves making an initial guess and refining it. For instance, starting with a guess close to 1.4 for √2 and squaring it to check if the result approaches 2:
\[
\text{Initial guess: } 1.4 \\
1.4 \times 1.4 = 1.96 \approx 2
\]
Using any of these methods will demonstrate that multiplying √2 by √2 yields the value 2, confirming the consistency across different calculation approaches.
Properties of Square Roots
The properties of square roots are essential for understanding various mathematical concepts. Here are some key properties:
- Non-Negativity: The square root of any non-negative number is also non-negative. For any \( a \geq 0 \), \( \sqrt{a} \geq 0 \).
- Product Property: The square root of a product is the product of the square roots of each factor. Mathematically, \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \) for \( a \geq 0 \) and \( b \geq 0 \).
- Quotient Property: The square root of a quotient is the quotient of the square roots. This is expressed as \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \) for \( a \geq 0 \) and \( b > 0 \).
- Square Property: The square of a square root returns the original value. That is, \( (\sqrt{a})^2 = a \) for any \( a \geq 0 \).
- Addition and Subtraction: Unlike multiplication and division, the square root of a sum or difference does not equal the sum or difference of the square roots. For example, \( \sqrt{a + b} \ne \sqrt{a} + \sqrt{b} \).
- Even and Odd Properties: The square root of a perfect square number (e.g., 4, 9, 16) is always an integer.
These properties form the foundation for more advanced operations involving square roots and are crucial for simplifying expressions and solving equations.
Mathematical Applications
The square root of 2 multiplied by the square root of 2 is a fundamental mathematical concept with various applications in different fields. This operation is often represented as \( \sqrt{2} \times \sqrt{2} \) and simplifies to 2. Here, we will explore some of the significant applications of this operation in mathematics.
- Geometry: The operation \( \sqrt{2} \times \sqrt{2} = 2 \) is crucial in geometry, especially in determining the lengths of the sides of squares and triangles. For example, in a right-angled triangle with both legs of length 1, the hypotenuse is \( \sqrt{2} \). Multiplying this by \( \sqrt{2} \) confirms the Pythagorean theorem, giving a hypotenuse length of 2.
- Algebra: Understanding \( \sqrt{2} \times \sqrt{2} = 2 \) helps in solving equations involving square roots. This operation is a key step in simplifying and solving radical expressions.
- Physics: In physics, square roots frequently appear in formulas, such as those involving energy, wave functions, and distances. Simplifying expressions with square roots is essential for accurate calculations and predictions.
- Engineering: Engineers use square roots to solve problems related to signal processing, electrical circuits, and material properties. Simplifying square roots ensures precise measurements and calculations in design and analysis.
- Statistics: In statistics, the square root is used to compute standard deviations and variances. Understanding the properties of square roots helps in accurately interpreting data distributions and variability.
Overall, the operation \( \sqrt{2} \times \sqrt{2} = 2 \) is not only a simple mathematical fact but also a fundamental tool used in various applications across multiple disciplines.
Examples and Problem Solving
In this section, we will explore practical examples and problem-solving techniques involving the multiplication of the square root of 2 by itself.
Example 1: Multiplying Square Roots
Let's start with a straightforward example to understand the multiplication of square roots:
- Problem: Find the result of multiplying the square root of 2 by itself.
- Solution:
Using the property of square roots, we have:
\[
\sqrt{2} \times \sqrt{2} = (\sqrt{2})^2 = 2
\]
Thus, \(\sqrt{2} \times \sqrt{2} = 2\).
Example 2: Solving a Quadratic Equation
Consider solving a quadratic equation involving square roots:
- Problem: Solve the equation \(x^2 - 2 = 0\).
- Solution:
- Isolate the quadratic term:
\[
x^2 = 2
\] - Apply the square root property:
\[
x = \pm \sqrt{2}
\]
Therefore, the solutions are \(x = \sqrt{2}\) and \(x = -\sqrt{2}\).
Example 3: Finding the Length of a Diagonal
In geometry, the diagonal of a square can be calculated using the Pythagorean theorem:
- Problem: Find the diagonal of a square with side length 1.
- Solution:
- Apply the Pythagorean theorem:
\[
d = \sqrt{1^2 + 1^2} = \sqrt{2}
\] - Multiply by the square root of 2 to find the length of the diagonal:
\[
d = \sqrt{2}
\]
The length of the diagonal is \(\sqrt{2}\).
Example 4: Practical Application in Geometry
Consider a real-world application where you need to find the diagonal of a rectangle:
- Problem: Find the diagonal of a rectangle with length 3 and width 2.
- Solution:
- Apply the Pythagorean theorem:
\[
d = \sqrt{3^2 + 2^2} = \sqrt{9 + 4} = \sqrt{13}
\] - Simplify if necessary. In this case, \(\sqrt{13}\) cannot be simplified further.
The length of the diagonal is \(\sqrt{13}\).
Example 5: Solving Equations with Radicals
Finally, let's solve an equation involving radicals:
- Problem: Solve the equation \(\sqrt{2x + 3} = 3\).
- Solution:
- Square both sides to eliminate the square root:
\[
2x + 3 = 9
\] - Simplify and solve for \(x\):
\[
2x = 6 \\
x = 3
\]
Thus, the solution is \(x = 3\).
FAQs on Square Roots
-
What is the value of the square root of 2?
The value of the square root of 2 (√2) is approximately 1.414213562373095. It is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal form goes on forever without repeating.
-
Why is the square root of 2 considered irrational?
The square root of 2 is considered irrational because it cannot be written as a fraction of two integers. This was proven by the ancient Greeks who showed that no fraction exactly equals the square root of 2.
-
How to calculate the square root of negative numbers?
To calculate the square root of negative numbers, we use the concept of imaginary numbers. The square root of -1 is denoted as 'i', so the square root of any negative number can be expressed as the square root of the positive number times 'i'. For example, √(-4) = 2i.
-
Is 2 a perfect square?
No, 2 is not a perfect square. A perfect square is a number that can be expressed as the square of an integer. Since there is no integer that, when squared, equals 2, it is not a perfect square.
Additional Resources
To deepen your understanding of the square root of 2 and its multiplication, here are some valuable resources:
-
Khan Academy - Solving Square Root Equations: A comprehensive guide on solving equations involving square roots, including step-by-step tutorials and practice problems. Perfect for building a solid foundation in algebraic principles.
-
Calculator Soup - Square Root Calculator: This online tool helps you calculate the square root of any number, providing both principal and negative roots. Additionally, it offers insights into perfect squares and complex solutions.
-
Math is Fun - Square Root: An educational resource that explains the concept of square roots in a simple and engaging manner, with interactive examples and exercises. Ideal for learners of all ages.
-
Wolfram Alpha - Square Roots: A powerful computational engine that provides detailed solutions and visual representations of square root calculations, making complex math more accessible.
-
Math World - Square Root: An in-depth article from Wolfram's Math World, covering the mathematical theory behind square roots, including proofs, properties, and applications.
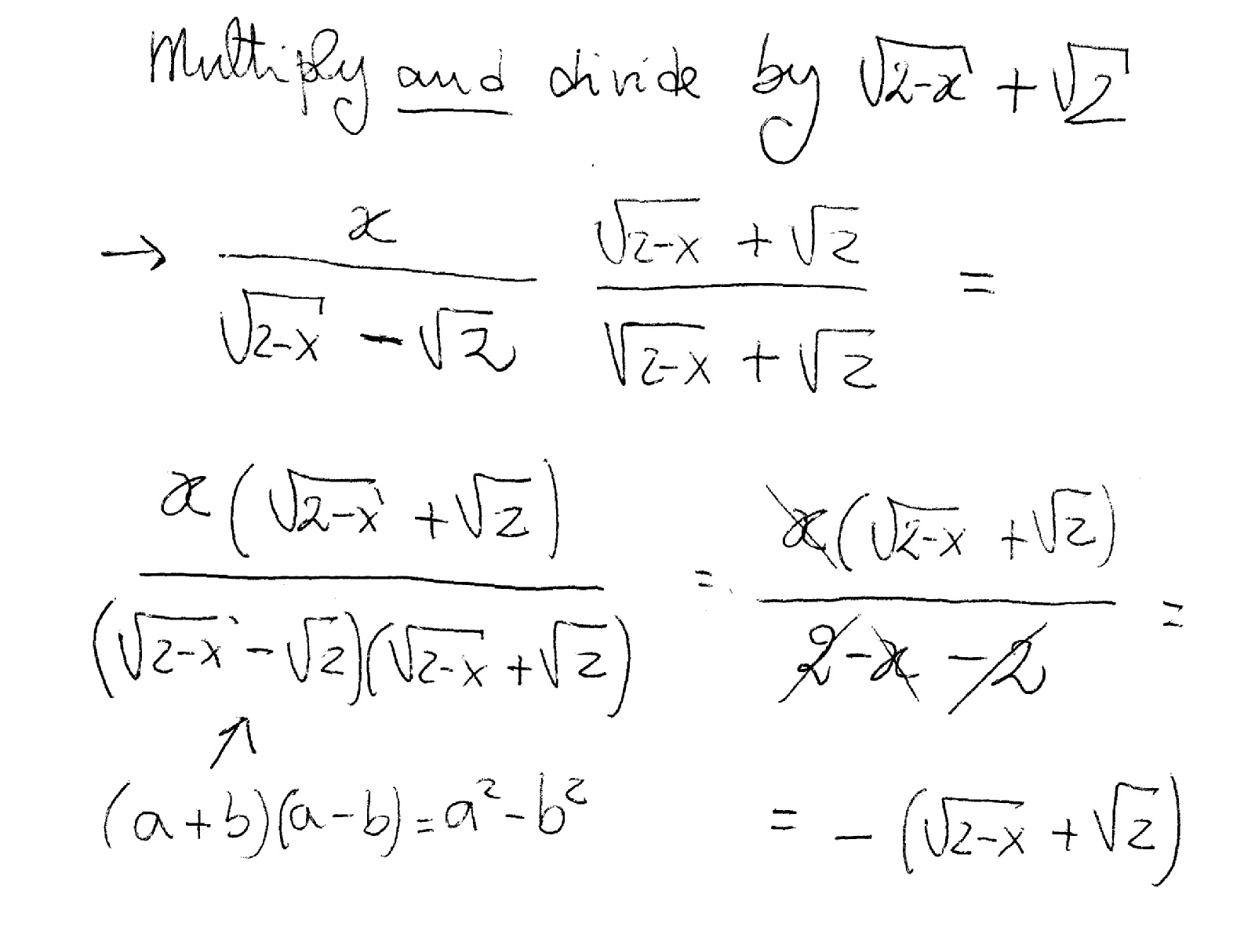
Video này giới thiệu về cách tính toán căn bậc hai của 2x^2 và 10x, cùng với việc nhân chúng lại với nhau. Hãy cùng tìm hiểu cách giải quyết bài toán này một cách chi tiết.
sqrt(2x^2) * sqrt(10x), căn bậc hai của 2x^2 và căn bậc hai của 10x
READ MORE:
Video này giới thiệu về cách tính căn bậc hai của 2, giúp bạn hiểu rõ hơn về khái niệm và tính chất của căn bậc hai của 2 trong toán học.
Căn bậc hai của 2