Topic 1 square root of 2: The concept of "1 square root of 2" is fundamental in mathematics, symbolizing an irrational number with an infinite decimal expansion. Understanding its calculation, historical significance, and applications in various fields can enhance mathematical comprehension and appreciation.
Table of Content
Understanding 1 Over the Square Root of 2
The expression is a common mathematical concept that appears in various fields such as algebra, trigonometry, and geometry. Here's a detailed explanation and various examples related to this expression:
Mathematical Simplification
To simplify , we can rationalize the denominator:
- Multiply both the numerator and the denominator by :
- The simplified form is .
Trigonometric Applications
The value often appears in trigonometry, specifically in the context of sine and cosine functions for 45-degree angles:
- For a 45° angle, the sine and cosine values are both , which is derived from .
- In general, results in ° or ° for x.
Geometric Context
In geometry, this expression can describe the relationship between the sides of a 45°-45°-90° triangle:
- The legs of the triangle are equal, and the hypotenuse is times the length of each leg.
- If each leg is 1 unit, then the hypotenuse is units, resulting in the sides being expressed as .
Example Problems
| Expression | Solution |
|---|---|
| Simplify | |
| Trigonometric value of |
Further Resources
- For detailed step-by-step solutions, visit .
- Explore more about simplifying radicals at .

READ MORE:
Introduction
The concept of the square root of 2, denoted as \( \sqrt{2} \), has intrigued mathematicians and scholars for centuries. This irrational number, approximately 1.414213562373095, is the positive solution to the equation \( x^2 = 2 \). It emerges naturally in various geometric and algebraic contexts, such as calculating the diagonal of a square with unit side lengths. Understanding the properties and methods of calculating \( \sqrt{2} \) involves delving into historical, theoretical, and practical aspects of mathematics.
-
Historical Background: The discovery of \( \sqrt{2} \) is often attributed to the ancient Greeks, specifically the Pythagoreans. The realization that \( \sqrt{2} \) is an irrational number, which cannot be expressed as a simple fraction, marked a significant moment in the history of mathematics.
-
Geometric Interpretation: In geometry, \( \sqrt{2} \) is known as the length of the diagonal of a square with side length 1. This result is derived from the Pythagorean theorem and has important implications in various fields such as architecture and design.
-
Methods of Calculation: Several algorithms exist to compute \( \sqrt{2} \) with high precision, including the Babylonian method and Newton's method. These iterative techniques improve the approximation of \( \sqrt{2} \) by repeatedly refining initial guesses.
-
Applications: The value of \( \sqrt{2} \) is used in various real-world applications, including engineering, physics, and computer science. It plays a crucial role in calculations involving roots and exponents, as well as in the design of algorithms and systems.
Historical Significance
The square root of 2, also known as √2, has played a pivotal role in the history of mathematics and has profound historical significance. It was the first number to be identified as irrational, a discovery attributed to the ancient Greeks. This realization marked a critical point in mathematical history, as it challenged the previously held belief that all numbers could be expressed as a ratio of integers.
Here are some key points outlining the historical significance of √2:
- Ancient Greeks: The Pythagoreans discovered that √2 could not be expressed as a fraction, leading to the concept of irrational numbers. This was a groundbreaking discovery that expanded the understanding of number theory.
- Geometric Interpretation: √2 represents the length of the diagonal of a square with unit side lengths, as described by the Pythagorean theorem. This geometric relationship was crucial in the study of geometry and influenced many subsequent mathematical theories.
- Babylonian Mathematics: Ancient Babylonian mathematicians developed methods to approximate √2, showcasing their advanced mathematical skills and the significance they placed on this number.
- Symbol of Intellectual Pursuit: Over centuries, √2 has symbolized the intellectual pursuit of knowledge and the understanding of the natural world. It has appeared in various mathematical problems and theories, highlighting its enduring legacy.
The continuous study and application of √2 in different cultures and mathematical contexts underscore its importance and the universal fascination with this unique and fundamental constant.
Mathematical Representation
The square root of 2, often denoted as or √2, is a crucial mathematical constant with various representations and applications. Here, we explore its mathematical significance step by step.
- Algebraic Definition: The square root of 2 is defined as the positive number that, when squared, equals 2. Mathematically, this can be expressed as:
where
- Decimal Representation: The value of is approximately 1.41421356237, but it is an irrational number, meaning it cannot be precisely expressed as a fraction and its decimal representation is non-terminating and non-repeating.
- Geometric Representation: In geometry, represents the length of the diagonal of a square with side length 1. Using the Pythagorean theorem:
which simplifies to .
- Rational Approximations: While is irrational, it can be approximated by fractions such as 99/70, which provides a close estimate of 1.4142857.
- Trigonometric Applications: The value of or frequently appears in trigonometry, particularly in the sine and cosine of 45 degrees (π/4 radians), which are both equal to .
Understanding the mathematical representation of provides a foundation for deeper exploration into various fields of mathematics, from algebra and geometry to calculus and beyond.
Calculation Methods
Calculating the square root of 2, an irrational number, involves several methods, each with varying levels of precision. Here are some common methods:
Estimation Method
- Start with an initial guess between 1 and 2 since 12 = 1 and 22 = 4.
- Refine your guess. For example, 1.52 = 2.25, which is greater than 2, so try a lower number.
- Continue narrowing the range. For instance, 1.42 = 1.96, which is closer but still less than 2.
- Keep refining until the desired precision is reached, such as 1.41, 1.414, etc.
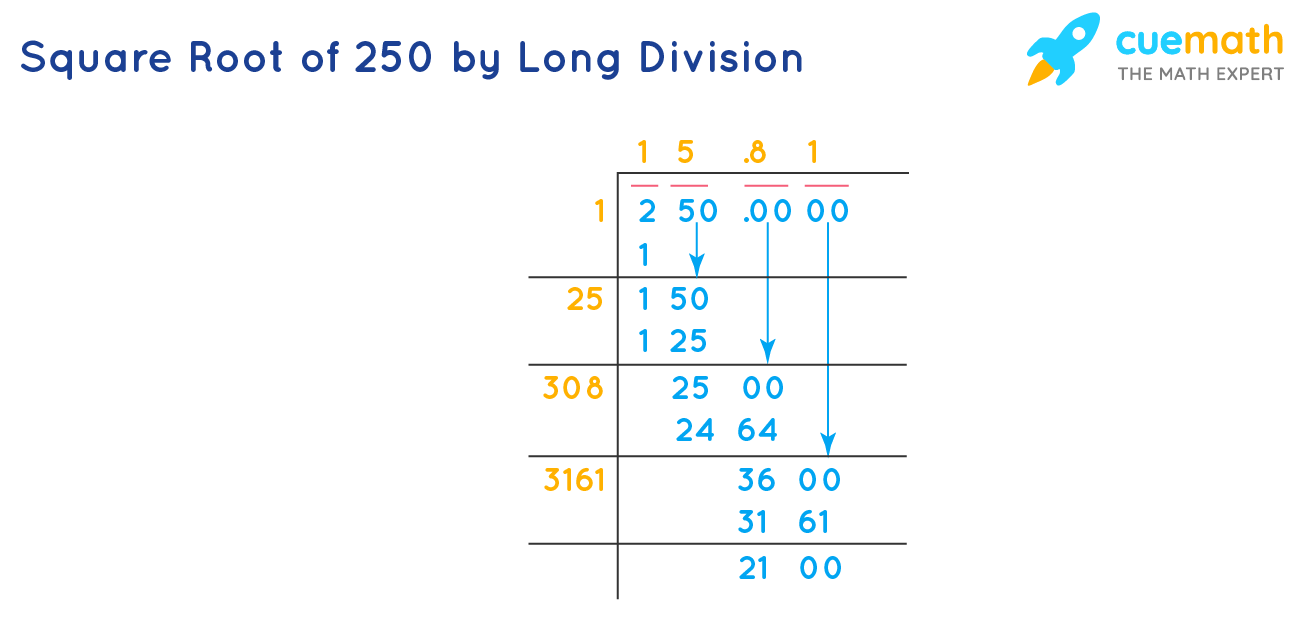
Long Division Method
This method provides a step-by-step approach to calculating the square root:
- Pair the digits of the number starting from the decimal point.
- Find the largest number whose square is less than or equal to the first pair. Use it as both the divisor and quotient.
- Subtract the square of the quotient from the first pair and bring down the next pair of digits.
- Double the quotient and determine the next digit of the quotient such that when appended and multiplied, the product is less than or equal to the current dividend.
- Repeat the process for more decimal places.
Prime Factorization Method
This method is generally useful for finding the square root of perfect squares:
- Express the number as a product of prime factors.
- Group the prime factors in pairs.
- Take one number from each pair and multiply them together to get the square root.
Since 2 is not a perfect square, this method highlights its irrational nature, which cannot be perfectly expressed as a product of integer pairs.

Applications in Geometry
The square root of 2, often represented as
- Diagonal of a Square: In a square with each side of length \(a\), the length of the diagonal is
\(\sqrt{2}a\) . For example, if each side of the square is 1 unit, the diagonal will be\(\sqrt{2}\) units. - Right-Angled Triangles: In a right-angled triangle with both legs of equal length \(a\), the hypotenuse is
\(\sqrt{2}a\) . This is derived using the Pythagorean theorem. - Construction with Compass and Straightedge: Geometrical methods allow the construction of a segment of length
\(\sqrt{2}\) using a compass and straightedge. This involves creating a right triangle with equal legs of unit length and measuring the hypotenuse. - Tiling and Patterns: The square root of 2 appears in tiling problems, especially those involving diagonal patterns or the construction of octagonal shapes within squares.
Understanding the applications of
Examples and Problems
The square root of 2, denoted as √2, is an irrational number often encountered in various mathematical problems. Below are some examples and problems involving √2, presented in a step-by-step manner for clarity and understanding.
Example 1: Basic Calculation
Calculate the square root of 2 to three decimal places.
- We start with the approximation method or a calculator.
- The square root of 2 is approximately 1.414.
Thus, √2 ≈ 1.414.
Example 2: Solving Equations
Solve the equation x² = 2 for x.
- Taking the square root on both sides: x = ±√2.
- Since √2 is approximately 1.414, the solutions are x ≈ ±1.414.
Therefore, x ≈ 1.414 or x ≈ -1.414.
Problem 1: Geometry Application
In a right-angled triangle, if the legs are both 1 unit in length, find the length of the hypotenuse.
- Using the Pythagorean theorem: hypotenuse² = 1² + 1².
- Thus, hypotenuse² = 2.
- Taking the square root on both sides: hypotenuse = √2.
The length of the hypotenuse is √2 or approximately 1.414 units.
Problem 2: Simplifying Expressions
Simplify the expression: 5√2 + 3√2.
- Combine like terms: (5 + 3)√2.
- Thus, the expression simplifies to 8√2.
The simplified expression is 8√2.
Example 3: Comparing Numbers
Determine whether √2 is greater than 1.4.
- We know that √2 ≈ 1.414.
- Comparing 1.414 with 1.4: 1.414 > 1.4.
Therefore, √2 is greater than 1.4.
Problem 3: Rationalizing the Denominator
Rationalize the denominator of the expression 1/√2.
- Multiply the numerator and the denominator by √2: (1/√2) * (√2/√2).
- This simplifies to √2/2.
The rationalized form of 1/√2 is √2/2.
Example 4: Verifying Solutions
Verify if √2 is a solution to the equation x² = 2.
- Substitute √2 into the equation: (√2)² = 2.
- Since (√2)² = 2, the equation holds true.
Thus, √2 is indeed a solution to the equation x² = 2.
Problem 4: Approximation Techniques
Estimate the value of √2 using the method of averaging.
- Choose two close numbers whose product is less than and greater than 2, e.g., 1.4 and 1.5.
- Calculate the average: (1.4 + 1.5) / 2 = 1.45.
- Square 1.45 to check: 1.45² = 2.1025.
- Adjust and repeat until the desired precision is achieved.
The estimated value of √2 using averaging is approximately 1.414.
FAQ
-
What is the Value of √2?
The value of √2 is approximately 1.41421. For more precision, it can be represented as 1.4142135623730950488016887242097..., continuing infinitely without repetition.
-
Why is √2 an Irrational Number?
√2 is an irrational number because it cannot be expressed as a fraction of two integers. Its decimal representation is non-terminating and non-repeating, which means there is no exact fractional equivalent.
-
How to Calculate √2?
There are several methods to calculate √2:
-
Long Division Method: This method involves estimating, dividing, averaging, and repeating until the desired precision is achieved. Here's a step-by-step outline:
- Estimate a number close to √2 (e.g., 1.4).
- Divide 2 by this estimate (2 ÷ 1.4 ≈ 1.428).
- Averaging the result with the estimate ((1.4 + 1.428) / 2 ≈ 1.414).
- Repeat the process with the new estimate until you reach the desired accuracy.
-
Newton's Method: Also known as the Babylonian method, it uses iteration to approximate √2:
- Start with an initial guess (x0).
- Apply the iteration formula: xn+1 = (xn + 2 / xn) / 2.
- Continue iterating until the difference between successive values is within the desired precision.
-
Rational Approximations: For rough estimates, fractions like 99/70 (≈ 1.414285714) can be used to approximate √2.
-
-
How is √2 Used in Geometry?
√2 appears in various geometric contexts, particularly related to right triangles and squares:
- Diagonal of a Square: The length of the diagonal of a square is √2 times the length of one of its sides.
- Isosceles Right Triangle: In a 45-45-90 triangle, the hypotenuse is √2 times the length of each leg.
Chứng Minh: Căn Bậc Hai Của 2 Là Số Vô Tỉ
READ MORE:
Một Chứng Minh Rằng Căn Bậc Hai Của Hai Là Số Vô Tỉ