Square Root of 180 in Simplest Radical Form: Understanding and Calculation

According to mathematical calculations and methods, the simplified square root of 180 is represented as follows: $$\sqrt{180} = 6 \sqrt{5}$$

Explanation:

To simplify the square root of 180, we first find its prime factors. 180 can be expressed as the product of 36 and 5. Since 36 is a perfect square, its square root is 6. Therefore, the simplified form of $$\sqrt{180}$$ is 6 times the square root of 5.

Verification:

Calculating further, $$6 \sqrt{5}$$ squared yields: $$(6 \sqrt{5})^2 = 36 \times 5 = 180$$ Thus, confirming that $$\sqrt{180} = 6 \sqrt{5}$$ is correct.

Conclusion:

Therefore, the simplified square root of 180 is $$\sqrt{180} = 6 \sqrt{5}$$

The square root of 180 in simplest radical form is a fundamental mathematical concept that involves finding a number which, when multiplied by itself, gives the result of 180. In mathematical notation, it is represented as \( \sqrt{180} \). Simplifying \( \sqrt{180} \) involves breaking down the number into its prime factors to reveal the simplest form of the square root. This process is crucial in various fields such as geometry, physics, and engineering, where precise calculations are necessary.

Square roots are a foundational concept in mathematics, specifically dealing with the inverse operation of squaring a number. For the square root of 180, denoted as \( \sqrt{180} \), the goal is to find a number that, when multiplied by itself, equals 180. To simplify \( \sqrt{180} \) into its simplest radical form, one typically factors 180 into prime numbers and extracts pairs of identical factors, leaving any unmatched factor under the square root symbol.

Calculating the square root of 180 in simplest radical form involves several methods:

1. Prime Factorization Method: Decompose 180 into its prime factors: \( 180 = 2^2 \times 3^2 \times 5 \). Identify pairs of identical factors to simplify under the square root: \( \sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} = 6\sqrt{5} \).
2. Estimation Method: Approximate the square root by testing integers close to the expected result. For 180, \( \sqrt{180} \approx 13.42 \). Refine the approximation until reaching the simplest radical form.
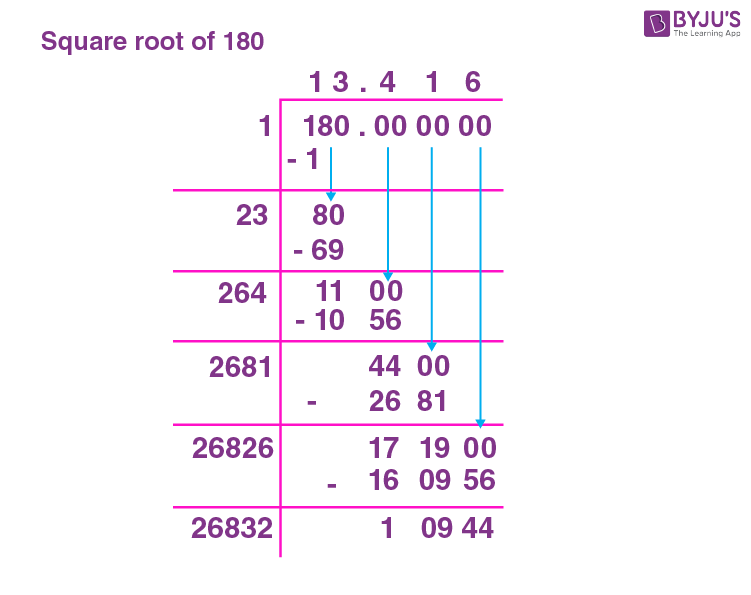
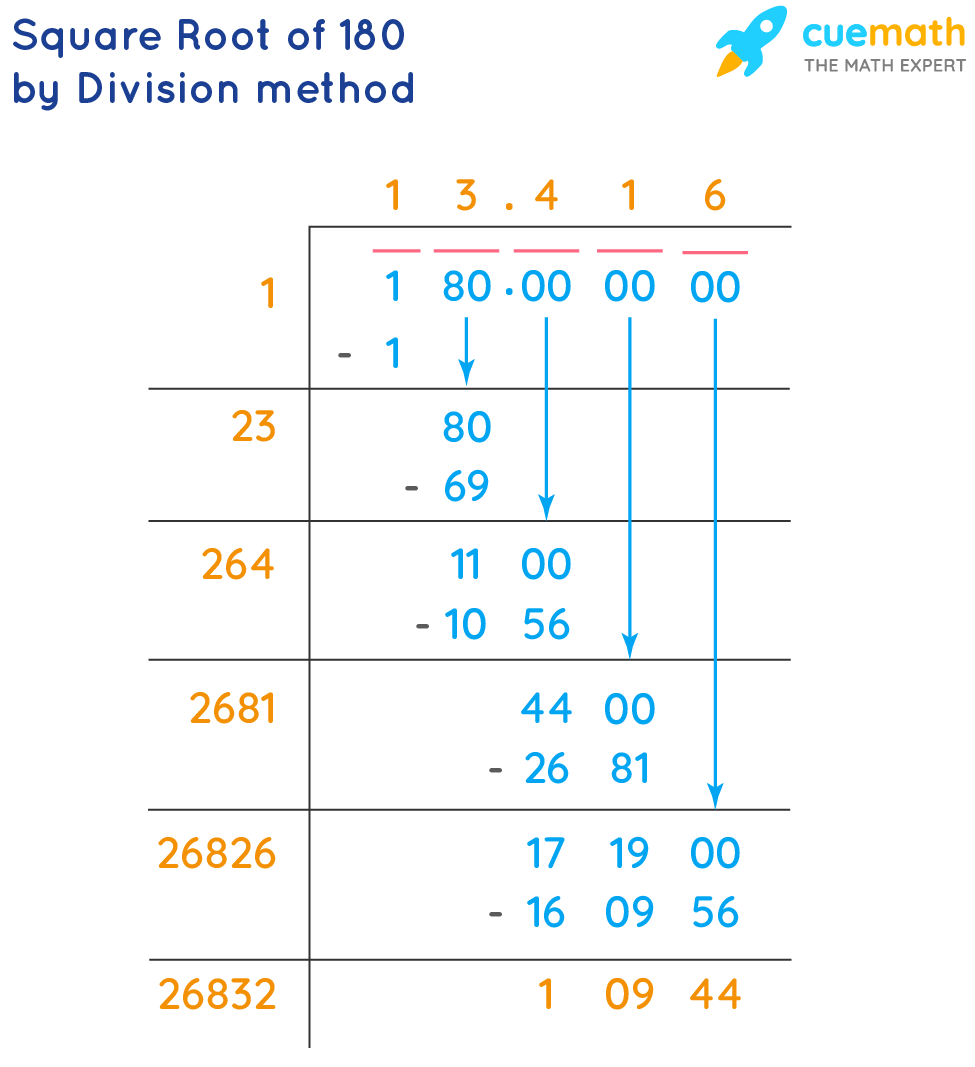
3. Long Division Method: Employ the long division algorithm for square roots to iteratively find the digits of the square root of 180, ensuring precision in calculation.

Simplifying square roots involves finding the prime factorization of the number under the radical sign and then simplifying based on pairs of prime factors. Let's go through an example with the square root of 180.

The steps to simplify \( \sqrt{180} \) are as follows:

1. Find the prime factorization of 180.
   • 180 = 2 × 90
   • 90 = 2 × 45
   • 45 = 3 × 15
   • 15 = 3 × 5
   • So, 180 = 2 × 2 × 3 × 3 × 5
2. Rewrite the square root of 180 using its prime factors.

   \( \sqrt{180} = \sqrt{2 \times 2 \times 3 \times 3 \times 5} \)

3. Group the prime factors into pairs and simplify.
   • Pairs of 2 and 3 can be taken out of the square root.
     • \( \sqrt{2 \times 2} = 2 \)
     • \( \sqrt{3 \times 3} = 3 \)
   • So, \( \sqrt{180} = 2 \times 3 \times \sqrt{5} \)
   • Therefore, \( \sqrt{180} = 6\sqrt{5} \)

Thus, the simplest radical form of \( \sqrt{180} \) is \( 6\sqrt{5} \).

Let's look at another example:

Simplify \( \sqrt{72} \).

1. Find the prime factorization of 72.
   • 72 = 2 × 36
   • 36 = 2 × 18
   • 18 = 2 × 9
   • 9 = 3 × 3
   • So, 72 = 2 × 2 × 2 × 3 × 3
2. Rewrite the square root of 72 using its prime factors.

   \( \sqrt{72} = \sqrt{2 \times 2 \times 2 \times 3 \times 3} \)

3. Group the prime factors into pairs and simplify.
   • Pairs of 2 and 3 can be taken out of the square root.
     • \( \sqrt{2 \times 2} = 2 \)
     • \( \sqrt{3 \times 3} = 3 \)
   • So, \( \sqrt{72} = 2 \times 3 \times \sqrt{2} \)
   • Therefore, \( \sqrt{72} = 6\sqrt{2} \)

Thus, the simplest radical form of \( \sqrt{72} \) is \( 6\sqrt{2} \).

The square root of 180 in its simplest radical form, \(6\sqrt{5}\), has several practical applications in various fields. Here, we explore some key areas where understanding and utilizing this radical expression is beneficial.

• Engineering and Construction:

  In engineering and construction, precise measurements are crucial. When working with geometric shapes and distances, the square root of 180 can come into play. For example, when calculating the diagonal of a rectangular space or when dealing with right-angled triangles where one of the sides is a multiple of \(6\sqrt{5}\), using this simplified radical form makes the calculations more manageable.

• Physics:

  Physics often involves dealing with square roots when calculating distances, speeds, and other physical quantities. The square root of 180 can be used in formulas related to wave mechanics, optics, and even quantum physics. For instance, if an experiment requires setting up an apparatus at a distance proportional to \(6\sqrt{5}\) meters, recognizing the simplest radical form aids in accuracy and simplification.

• Architecture and Design:

  Architects and designers use square roots to determine proportions and spatial relationships in their designs. When designing elements that must maintain specific proportions, such as the height of a building or the dimensions of a garden, the square root of 180 might be used. The simplest radical form helps in maintaining aesthetic harmony and structural integrity.

• Mathematical Problem Solving:

  In mathematics, especially in algebra and geometry, simplifying radicals is a common practice. The square root of 180, when simplified to \(6\sqrt{5}\), is often used in solving equations and proving theorems. This simplification helps in making the problems easier to manage and solve, leading to a deeper understanding of mathematical concepts.

• Computer Graphics:

  In computer graphics, calculations involving square roots are used for rendering images, animations, and simulations. The square root of 180 might be used when calculating the distances between points in a 3D space or when scaling objects. Simplifying this to \(6\sqrt{5}\) can optimize the performance of graphic algorithms and enhance visual accuracy.

In summary, the simplest radical form of the square root of 180, \(6\sqrt{5}\), finds its relevance in various practical scenarios, making complex calculations more approachable and aiding in precision and efficiency across multiple disciplines.

Understanding the square root of 180 in its simplest radical form reveals its properties and applications in mathematics. The square root of 180 simplifies to \( 6\sqrt{5} \), and this process involves breaking down the number into its prime factors and grouping them accordingly.

Throughout this discussion, we explored various methods for simplifying square roots, such as the prime factorization method, which is crucial for dealing with large numbers. For instance, we broke down 180 into \( 2 \times 2 \times 3 \times 3 \times 5 \), allowing us to simplify \( \sqrt{180} \) to \( 6\sqrt{5} \).

We also examined the importance of recognizing irrational numbers. The square root of 180 is irrational because it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating. This understanding is fundamental in higher mathematics and real-world applications.

In conclusion, mastering the simplification of square roots not only enhances mathematical comprehension but also prepares us for more complex problem-solving scenarios. Whether applying these concepts in geometry, algebra, or real-world contexts, the skills developed here are invaluable.