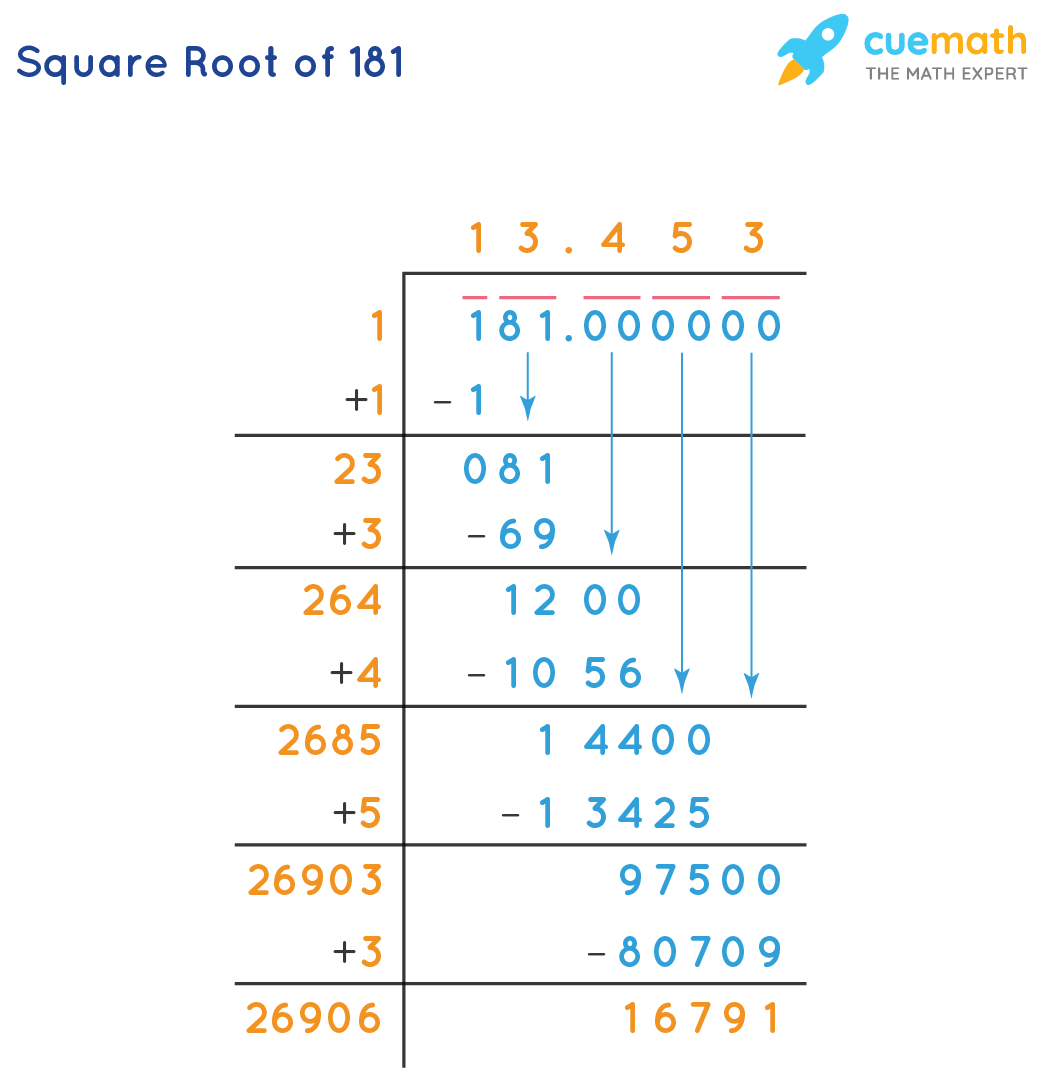
Topic what is the square root of 1/4: Welcome to our comprehensive guide on the square root of 1/4. Whether you're a student or simply curious about fractions and square roots, this article will walk you through the basic definitions, step-by-step calculations, and real-life applications of this fundamental mathematical concept.
Table of Content
- Understanding the Square Root of 1/4
- Introduction to Square Roots
- Basic Definition of Square Roots
- Understanding Fractions
- Square Root of a Fraction
- Step-by-Step Calculation of Square Root of 1/4
- Mathematical Properties of Square Roots
- Visualizing the Square Root of 1/4
- Square Root of 1/4 in Decimal Form
- Applications of Square Roots in Real Life
- Common Misconceptions About Square Roots
- Advanced Topics Related to Square Roots
- Conclusion and Summary
- YOUTUBE: Video này giải thích cách tìm căn bậc hai của 1/4, hướng dẫn chi tiết và dễ hiểu để thu hút người xem.
Understanding the Square Root of 1/4
The square root of a number is a value that, when multiplied by itself, gives the original number. In this case, we are looking for the square root of
Step-by-Step Calculation
- We start with the fraction
\(\frac{1}{4}\) . - Recall that the square root of a fraction is equal to the square root of the numerator divided by the square root of the denominator:
- The square root of 1 is 1:
- The square root of 4 is 2:
- Putting it all together:
Conclusion
The square root of

READ MORE:
Introduction to Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 9 is 3 because 3 multiplied by 3 equals 9.
When dealing with fractions, like the square root of 1/4, it's important to understand that the square root operation involves finding a number that, when squared, results in the given fraction.
The square root of 1/4 can be determined as follows:
- Recognize that 1/4 is a fraction where the numerator (1) is the number whose square root we seek and the denominator (4) is the number we square to obtain the numerator.
- Apply the square root operation separately to the numerator and the denominator. The square root of 1 is 1 and the square root of 4 is 2.
- Therefore, the square root of 1/4 is 1/2.
Understanding square roots is essential in various fields of mathematics and everyday applications, from geometry and physics to finance and engineering.
Basic Definition of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of x is denoted as √x, and it satisfies the equation:
√x × √x = x
This can also be written as:
(√x)2 = x
The square root operation is denoted by the radical symbol (√) followed by the number whose square root is being determined. Here are some key properties of square roots:
- The square root of a positive number is always positive.
- The square root of 0 is 0.
- The square root of a positive number has two solutions: a positive and a negative value. For example, √4 = 2 and -2, since 22 = 4 and (-2)2 = 4.
- Square roots of negative numbers are not real numbers; they are complex numbers.
For fractions, the square root operation can be applied to both the numerator and the denominator individually. For instance, the square root of a fraction ½ can be expressed as:
√(½) = √1 / √2 = 1 / √2 = √2 / 2
Let's consider the square root of a specific fraction, like 1/4:
√(¼) = √1 / √4 = 1 / 2
Thus, the square root of 1/4 is 1/2.
Understanding Fractions
Fractions are a way to represent parts of a whole. They consist of two numbers: the numerator and the denominator, separated by a slash. The numerator (the top number) indicates how many parts we have, while the denominator (the bottom number) shows how many parts the whole is divided into.
For example, in the fraction , the numerator is 1 and the denominator is 4, meaning we have one part out of four equal parts of a whole.
Here are some key points to understand about fractions:
- Proper Fractions: These fractions have numerators smaller than denominators, like .
- Improper Fractions: These fractions have numerators larger than or equal to denominators, like .
- Mixed Numbers: These are combinations of a whole number and a proper fraction, like 1 .
- Equivalent Fractions: Different fractions that represent the same value, such as and .
Understanding how to simplify fractions is also important. Simplifying a fraction involves dividing both the numerator and the denominator by their greatest common divisor (GCD). For example:
- Find the GCD of the numerator and the denominator.
- Divide both the numerator and the denominator by the GCD.
- The result is a simplified fraction.
For instance, to simplify :
- The GCD of 4 and 8 is 4.
- Divide both the numerator and the denominator by 4:
- The simplified fraction is .
Fractions are fundamental in mathematics and understanding them is essential for progressing to more advanced topics such as ratios, proportions, and algebra.
Square Root of a Fraction
Finding the square root of a fraction involves understanding how to handle the numerator and the denominator separately under the square root. Here’s a step-by-step guide:
-
Express the Fraction: Start with the fraction for which you need to find the square root. For example, consider the fraction \( \frac{1}{4} \).
-
Separate the Numerator and Denominator: Use the property of square roots that allows you to split the square root of a fraction into the square root of the numerator divided by the square root of the denominator. This is represented mathematically as:
\[
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\] -
Calculate the Square Roots: Find the square roots of the numerator and the denominator separately.
- The square root of 1 is 1.
- The square root of 4 is 2.
-
Combine the Results: Place the square roots found in the numerator and the denominator back into a fraction:
\[
\frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}
\]
Therefore, the square root of \( \frac{1}{4} \) is \( \frac{1}{2} \).
This method can be applied to any fraction. Just remember to simplify the square roots of the numerator and denominator separately, and then combine them into a simplified fraction.
Step-by-Step Calculation of Square Root of 1/4
To find the square root of \( \frac{1}{4} \), we can follow a step-by-step process that simplifies the calculation:
-
Identify the Fraction: Start with the fraction \( \frac{1}{4} \).
-
Separate the Square Root: Use the property of square roots that allows you to separate the square root of the numerator and the denominator:
\[
\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}}
\] -
Calculate the Square Roots: Find the square root of the numerator and the denominator separately:
- The square root of 1 is 1, since \( 1 \times 1 = 1 \).
- The square root of 4 is 2, since \( 2 \times 2 = 4 \).
-
Combine the Results: Place the square roots found in the numerator and the denominator back into the fraction:
\[
\frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}
\] -
Verify the Result: Multiply the result by itself to ensure it equals the original fraction:
\[
\left(\frac{1}{2}\right)^2 = \frac{1}{4}
\]Since \( \frac{1}{2} \times \frac{1}{2} = \frac{1}{4} \), the calculation is correct.
Therefore, the square root of \( \frac{1}{4} \) is \( \frac{1}{2} \).
This method demonstrates the simplicity of working with square roots of fractions, breaking down the process into manageable steps.
Mathematical Properties of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. Mathematically, the square root of \(x\) is denoted as \(\sqrt{x}\), and can be defined as the number \(y\) such that \(y^2 = x\).
Several important properties and rules govern the behavior of square roots:
- Non-negative Results: For any non-negative number \(x\), \(\sqrt{x}\) is also non-negative. This is because both positive and negative values, when squared, yield positive results.
- Product Property: The square root of a product is the product of the square roots of each factor. Mathematically, \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\).
- Quotient Property: The square root of a quotient is the quotient of the square roots. This can be written as \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\), where \(b \neq 0\).
- Square of a Square Root: The square of a square root returns the original number, i.e., \((\sqrt{x})^2 = x\).
- Addition and Subtraction: Unlike multiplication and division, the square root of a sum or difference is not the sum or difference of the square roots. For example, \(\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\).
- Negative Numbers: The square root of a negative number is not a real number. Instead, it is an imaginary number. For example, \(\sqrt{-1} = i\), where \(i\) is the imaginary unit.
Let's look at a few specific examples to illustrate these properties:
- For the product property: \(\sqrt{16 \cdot 9} = \sqrt{144} = 12\) and \(\sqrt{16} \cdot \sqrt{9} = 4 \cdot 3 = 12\).
- For the quotient property: \(\sqrt{\frac{49}{4}} = \sqrt{12.25} = 3.5\) and \(\frac{\sqrt{49}}{\sqrt{4}} = \frac{7}{2} = 3.5\).
- For a negative number: \(\sqrt{-16} = 4i\) since \(4^2 = 16\) and \(i^2 = -1\).
Understanding these properties is crucial for solving equations involving square roots and for simplifying expressions in algebra.
Visualizing the Square Root of 1/4
Visualizing the square root of a fraction can be an enlightening way to grasp its mathematical properties. Let's explore the square root of \( \frac{1}{4} \) through different visual and graphical methods.
Graphical Representation
One of the most straightforward ways to visualize the square root of a fraction is through a graph. Consider the function \( y = \sqrt{x} \). When we plot this function, we can see how the square root of values between 0 and 1 behave.
For \( x = \frac{1}{4} \):
- The point \( (\frac{1}{4}, \sqrt{\frac{1}{4}}) \) on the graph of \( y = \sqrt{x} \) lies at \( (\frac{1}{4}, \frac{1}{2}) \).
The graph below illustrates this point:
Geometric Visualization
Another approach to visualizing the square root of \( \frac{1}{4} \) is through geometry. Imagine a square with an area of \( \frac{1}{4} \) square units. The side length of this square is the square root of the area:
- If the area is \( \frac{1}{4} \), the side length is \( \sqrt{\frac{1}{4}} = \frac{1}{2} \).
Thus, a square with an area of \( \frac{1}{4} \) has sides that are each \( \frac{1}{2} \) units long.
Unit Circle Representation
The unit circle provides another useful visualization. On the unit circle, the radius is 1, and various fractions can be represented along the circumference. For instance, \( \frac{1}{4} \) of the way around the circle corresponds to an angle of \( \frac{\pi}{2} \) radians. Taking the square root of this fraction involves finding a point where both the x and y coordinates are equal and less than 1:
- The point at \( \frac{1}{4} \) along the unit circle has coordinates that, when squared, sum up to \( \frac{1}{4} \).
- This point would be approximately at \( (\frac{1}{2}, \frac{1}{2}) \), confirming the square root value.
Conclusion
By using graphs, geometric shapes, and unit circles, we can visualize the square root of \( \frac{1}{4} \) as \( \frac{1}{2} \). These visual methods provide intuitive insights into understanding the properties and behavior of square roots in mathematical contexts.
Square Root of 1/4 in Decimal Form
To find the square root of \( \frac{1}{4} \) in decimal form, we can follow these steps:
- Understand the problem: We need to find \( \sqrt{\frac{1}{4}} \).
- Recall the property of square roots for fractions:
\[
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\]
Applying this property to our fraction:
\[
\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}}
\] - Calculate the square roots of the numerator and denominator:
- \( \sqrt{1} = 1 \)
- \( \sqrt{4} = 2 \)
- Combine the results:
\[
\sqrt{\frac{1}{4}} = \frac{1}{2}
\] - Convert the fraction to decimal form:
\[
\frac{1}{2} = 0.5
\]
Therefore, the square root of \( \frac{1}{4} \) in decimal form is 0.5.
| Expression | Calculation | Result |
|---|---|---|
| \( \sqrt{\frac{1}{4}} \) | \( \frac{\sqrt{1}}{\sqrt{4}} \) | \( \frac{1}{2} \) |
| \( \frac{1}{2} \) | Decimal conversion | 0.5 |

Applications of Square Roots in Real Life
Square roots, including the square root of 1/4, find numerous applications in various practical contexts:
- Engineering: Engineers often use square roots in calculations involving electrical power, mechanical forces, and structural stability.
- Finance: In finance, square roots are used to calculate volatility in financial markets, which is crucial for risk management and investment strategies.
- Physics: Square roots appear in physics formulas, such as those related to wave mechanics and quantum theory, where they describe amplitudes and probabilities.
- Statistics: Statisticians use square roots in formulas for standard deviation and variance, essential measures in analyzing data distributions.
- Geometry: Geometrically, square roots help determine distances and dimensions, especially in contexts like area calculations and diagonal lengths of squares.
- Medicine: In medical imaging, square roots are applied in the calculation of image resolution and signal-to-noise ratios, crucial for diagnostic accuracy.
These applications demonstrate the wide-ranging utility of square roots beyond theoretical mathematics, making them indispensable in many fields.
Common Misconceptions About Square Roots
Despite their simplicity, square roots can be misunderstood. Here are some common misconceptions:
- Square roots always result in positive numbers: While the principal (or positive) square root is typically considered, every positive number has both a positive and negative square root.
- Square roots are only applicable to whole numbers: Square roots can be calculated for any real number, including fractions and decimals.
- Square roots of fractions are more complex than whole numbers: The process of finding the square root of a fraction follows the same principles as whole numbers and can be straightforward.
- Square roots are only used in academic mathematics: Square roots find practical applications in fields like engineering, finance, and physics, making them relevant beyond theoretical math.
- Square roots of negative numbers are undefined: While the square root of a negative number is not a real number, it is defined in complex number theory, leading to the concept of imaginary numbers.
Understanding these misconceptions helps clarify the versatility and applicability of square roots in various mathematical contexts.
Advanced Topics Related to Square Roots
Understanding square roots at an advanced level involves exploring various properties, applications, and implications in different mathematical contexts. Here, we delve into some of these advanced topics:
1. Principal and Negative Square Roots
Every positive real number has two square roots: a positive and a negative root. The principal square root is the positive one, represented by the radical symbol (√). For example:
\[
\sqrt{4} = 2 \quad \text{and} \quad -\sqrt{4} = -2
\]
Thus, the square roots of 4 are 2 and -2.
2. Complex Numbers and Imaginary Square Roots
When dealing with negative numbers under the square root, we enter the realm of complex numbers. The imaginary unit \(i\) is defined as the square root of -1. Therefore:
\[
\sqrt{-1} = i
\]
This extends to other negative numbers, e.g., \(\sqrt{-4} = 2i\).
3. Rationalizing the Denominator
When dealing with fractions under a square root, it is often useful to rationalize the denominator. For example:
\[
\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}
\]
4. Properties of Square Roots in Algebraic Manipulations
Square roots follow certain properties that are useful in algebraic manipulations:
-
Product Property:
\[
\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}
\] -
Quotient Property:
\[
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\]
5. Solving Equations Involving Square Roots
Equations that involve square roots often require squaring both sides to eliminate the radical. Consider the equation:
\[
\sqrt{x + 3} = 5
\]
Squaring both sides gives:
\[
x + 3 = 25 \quad \Rightarrow \quad x = 22
\]
6. The Use of Square Roots in Calculus
In calculus, square roots appear in various contexts, including derivatives and integrals. For example, the derivative of the square root function is given by:
\[
\frac{d}{dx} \left( \sqrt{x} \right) = \frac{1}{2\sqrt{x}}
\]
7. Irrational Square Roots
Not all square roots are rational numbers. For instance, \(\sqrt{2}\) is an irrational number, meaning it cannot be expressed as a simple fraction. Its decimal representation is non-repeating and non-terminating.
8. Applications of Square Roots
Square roots have applications in various fields such as physics, engineering, and statistics. They are essential in solving quadratic equations, computing areas and volumes, and analyzing standard deviations in statistics.
9. Visualizing Square Roots
Square roots can be visualized geometrically. For example, the square root of a number represents the length of the side of a square with that area. The square root of 1/4 can be visualized as the side length of a square with an area of 1/4 unit.
Understanding these advanced concepts allows for a deeper appreciation and application of square roots in more complex mathematical problems.
Conclusion and Summary
The square root of \( \frac{1}{4} \) is calculated as follows:
- Recognize that \( \frac{1}{4} \) can be rewritten as \( 1 \div 4 \).
- Apply the square root operation to both the numerator and the denominator separately: \( \sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}} \).
- Since \( \sqrt{1} = 1 \) and \( \sqrt{4} = 2 \), the expression simplifies to \( \frac{1}{2} \).
Therefore, the square root of \( \frac{1}{4} \) is \( \frac{1}{2} \).
Video này giải thích cách tìm căn bậc hai của 1/4, hướng dẫn chi tiết và dễ hiểu để thu hút người xem.
Căn bậc hai của 1/4 | Căn(1/4)
READ MORE:
Khám phá câu hỏi: 'Căn bậc hai của 1/4 là gì?' qua video này. Cùng tìm hiểu và làm sáng tỏ khái niệm toán học thú vị này.
Căn bậc hai của 1/4 là gì?