Topic simplify square root with fractions: Learn how to simplify square root with fractions easily with our comprehensive guide. Whether you're a student or a math enthusiast, this article will provide you with step-by-step instructions, practical examples, and advanced techniques to master this essential mathematical skill. Simplify complex fractions and square roots with confidence and precision.
Table of Content
- Simplifying Square Roots with Fractions
- Introduction
- Understanding Square Roots
- Basics of Fractions
- Combining Square Roots and Fractions
- Simplifying Square Roots
- Rationalizing the Denominator
- Simplifying Fractions with Square Roots
- Step-by-Step Examples
- Special Cases
- Square Roots of Improper Fractions
- Mixed Numbers and Square Roots
- Quotient Rule for Square Roots
- Common Mistakes to Avoid
- Practice Problems
- Advanced Techniques
- Applications in Real Life
- Frequently Asked Questions
- Conclusion
- YOUTUBE: Xem video này để học cách đơn giản hóa phân số dưới dấu căn bậc hai một cách dễ dàng. Hãy cùng khám phá phương pháp đơn giản hóa phân số với căn bậc hai.
Simplifying Square Roots with Fractions
To simplify square roots involving fractions, we typically follow these steps:
Steps to Simplify Fractions with Square Roots
- Simplify the Square Root:
Factor the number inside the square root to identify and simplify any perfect squares. For example:
\(\sqrt{72} = \sqrt{36 \cdot 2} = 6\sqrt{2}\)
- Rationalize the Denominator:
Eliminate the square root in the denominator by multiplying both the numerator and the denominator by the conjugate of the denominator if necessary.
For example, to simplify \(\frac{5}{\sqrt{3}}\):
\(\frac{5}{\sqrt{3}} \cdot \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
Examples
Example 1: Simplify \(\frac{\sqrt{18}}{3}\)
- Simplify the square root:
\(\sqrt{18} = \sqrt{9 \cdot 2} = 3\sqrt{2}\)
- Simplify the fraction:
\(\frac{3\sqrt{2}}{3} = \sqrt{2}\)
Example 2: Simplify \(\frac{2}{\sqrt{12}}\)
- Simplify the square root:
\(\sqrt{12} = \sqrt{4 \cdot 3} = 2\sqrt{3}\)
- Rationalize the denominator:
\(\frac{2}{\sqrt{12}} \cdot \frac{\sqrt{12}}{\sqrt{12}} = \frac{2\sqrt{12}}{12} = \frac{2 \cdot 2\sqrt{3}}{12} = \frac{\sqrt{3}}{3}\)
Example 3: Simplify \(\frac{\sqrt{6}}{\sqrt{2}}\)
- Simplify the square roots:
\(\sqrt{6} = \sqrt{2 \cdot 3} = \sqrt{2} \cdot \sqrt{3}\)
- Simplify the fraction:
\(\frac{\sqrt{2} \cdot \sqrt{3}}{\sqrt{2}} = \sqrt{3}\)
Additional Examples for Practice
- Simplify \(\frac{3\sqrt{5}}{5}\):
\(\frac{3\sqrt{5}}{5}\) remains the same as it cannot be simplified further.
- Simplify \(\frac{4}{\sqrt{18}}\):
\(\sqrt{18} = 3\sqrt{2}\)
\(\frac{4}{\sqrt{18}} \cdot \frac{\sqrt{18}}{\sqrt{18}} = \frac{4\sqrt{18}}{18} = \frac{4 \cdot 3\sqrt{2}}{18} = \frac{2\sqrt{2}}{3}\)
Summary
Fractions with square roots can be simplified by first simplifying the square root and then rationalizing the denominator. Practice with various examples to master the technique.

READ MORE:
Introduction
Simplifying square roots with fractions can initially seem challenging, but with a step-by-step approach, it becomes manageable. The process often involves simplifying the fraction, breaking it down into smaller parts, and using properties of square roots to simplify further. This section will guide you through various methods to simplify square roots that contain fractions.
-
Basic Principle: The square root of a fraction is the fraction of the square roots.
\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
-
Reducing Fractions: Simplify the fraction by factoring both the numerator and the denominator into their prime factors.
\(\sqrt{\frac{18}{50}} = \sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5}\)
-
Rationalizing the Denominator: To remove a square root from the denominator, multiply both the numerator and the denominator by the square root in the denominator.
\(\frac{5}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{5\sqrt{2}}{2}\)
-
Mixed Numbers: Convert mixed numbers to improper fractions before simplifying.
\(\sqrt{1 \frac{13}{36}} = \sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} = 1 \frac{1}{6}\)
Understanding these foundational techniques is crucial for simplifying square roots with fractions. By practicing these methods, you can master the skill and apply it to more complex mathematical problems.
Understanding Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 9 is 3, because \(3 \times 3 = 9\). When working with square roots, it's important to simplify them to their simplest form.
Square roots can be applied to both whole numbers and fractions. Simplifying square roots involves breaking down the number inside the root into its prime factors. For instance, to simplify \(\sqrt{12}\), we factorize 12 into 4 and 3:
- \(\sqrt{12} = \sqrt{4 \times 3}\)
- Using the rule \(\sqrt{ab} = \sqrt{a} \times \sqrt{b}\), we get \(\sqrt{4 \times 3} = \sqrt{4} \times \sqrt{3}\)
- The square root of 4 is 2, so we have \(2\sqrt{3}\)
When dealing with fractions, the same principles apply. The square root of a fraction can be expressed as the square root of the numerator divided by the square root of the denominator. For example:
- \(\sqrt{\frac{25}{36}} = \frac{\sqrt{25}}{\sqrt{36}} = \frac{5}{6}\)
To simplify more complex expressions involving square roots, consider the following examples:
- Simplify \(\sqrt{\frac{18}{50}}\):
- Reduce the fraction: \(\frac{18}{50} = \frac{9}{25}\)
- Find the square root: \(\sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5}\)
- Simplify \(\sqrt{1\frac{13}{36}}\):
- Convert to an improper fraction: \(1\frac{13}{36} = \frac{49}{36}\)
- Find the square root: \(\sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} = 1\frac{1}{6}\)
By following these steps and applying the rules for square roots, you can simplify both whole numbers and fractions effectively.
Basics of Fractions
Fractions represent a part of a whole and consist of two main components: the numerator and the denominator. The numerator is the top number and signifies how many parts we have, while the denominator is the bottom number and indicates the total number of equal parts the whole is divided into.
To better understand fractions, let's break down some key concepts:
- Proper Fractions: These have a numerator smaller than the denominator, such as \( \frac{3}{4} \).
- Improper Fractions: These have a numerator greater than or equal to the denominator, such as \( \frac{7}{4} \).
- Mixed Numbers: These consist of a whole number and a fraction, like \( 1 \frac{3}{4} \).
- Equivalent Fractions: Different fractions that represent the same value, such as \( \frac{1}{2} \) and \( \frac{2}{4} \).
Fractions can also be simplified to their lowest terms by dividing the numerator and denominator by their greatest common divisor (GCD). For example, to simplify \( \frac{8}{12} \):
- Find the GCD of 8 and 12, which is 4.
- Divide both the numerator and the denominator by the GCD: \( \frac{8 \div 4}{12 \div 4} = \frac{2}{3} \).
Understanding these basics is crucial for working with more complex mathematical operations involving fractions, such as simplifying square roots within fractions.
Combining Square Roots and Fractions
Combining square roots and fractions involves several steps to simplify the expression effectively. Here, we will explore these steps in detail:
-
Simplify the Square Root:
First, identify and simplify the square root in both the numerator and the denominator, if possible. For example:
\(\sqrt{\frac{18}{50}} = \frac{\sqrt{18}}{\sqrt{50}}\)
Simplify each square root:
\(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
\(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\)
Thus, \(\frac{\sqrt{18}}{\sqrt{50}} = \frac{3\sqrt{2}}{5\sqrt{2}} = \frac{3}{5}\)
-
Rationalize the Denominator:
When the denominator contains a square root, rationalize it by multiplying both the numerator and the denominator by the conjugate or the square root in the denominator. For example:
\(\frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
-
Convert Mixed Numbers to Improper Fractions:
If dealing with mixed numbers, convert them to improper fractions before simplifying. For instance:
\(\sqrt{1\frac{13}{36}} = \sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} = 1\frac{1}{6}\)
By following these steps, you can simplify any fraction that contains square roots. Practice with various examples will help solidify these techniques.

Simplifying Square Roots
Understanding how to simplify square roots is crucial in mathematics, especially when dealing with fractions. Simplifying square roots involves a few key steps:
- Simplify the square root.
- Rationalize the denominator.
Let's explore these steps in detail:
Simplifying the Square Root
To simplify a square root, look for the largest perfect square factor of the number inside the square root.
- For example, \(\sqrt{72}\) can be simplified as follows:
\[\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\]
Rationalizing the Denominator
To rationalize the denominator, you need to eliminate the square root from the denominator. This is done by multiplying both the numerator and the denominator by the conjugate of the denominator.
- For example, to rationalize \(\frac{5}{\sqrt{3}}\):
\[\frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\]
Examples
Here are some examples to illustrate the process:
| Example 1: | Simplify \(\frac{\sqrt{18}}{3}\) |
| Step 1: | \[\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\] |
| Step 2: | \[\frac{3\sqrt{2}}{3} = \sqrt{2}\] |
| Example 2: | Simplify \(\frac{2}{\sqrt{12}}\) |
| Step 1: | \[\sqrt{12} = \sqrt{4 \times 3} = 2\sqrt{3}\] |
| Step 2: | \[\frac{2}{2\sqrt{3}} = \frac{1}{\sqrt{3}} = \frac{\sqrt{3}}{3}\] |
Rationalizing the Denominator
Rationalizing the denominator involves eliminating any square roots or irrational numbers from the denominator of a fraction. This is often done to simplify the expression and make it easier to understand and use in further calculations. Here are the steps to rationalize the denominator:
- Identify the square root in the denominator.
- Multiply the numerator and the denominator by the square root found in the denominator. This process will eliminate the square root from the denominator.
- Simplify the fraction if possible.
For example, to rationalize the denominator of the fraction \(\frac{1}{\sqrt{2}}\), you multiply both the numerator and the denominator by \(\sqrt{2}\):
- \(\frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}\)
Now, the denominator is rationalized, and the fraction is \(\frac{\sqrt{2}}{2}\).
Consider another example, \(\frac{3}{\sqrt{5}}\):
- \(\frac{3}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} = \frac{3\sqrt{5}}{5}\)
Again, the denominator is now rationalized, and the fraction is \(\frac{3\sqrt{5}}{5}\).
Rationalizing the denominator can also involve more complex expressions such as binomials. For instance, to rationalize \(\frac{2}{1+\sqrt{3}}\), follow these steps:
- Multiply the numerator and the denominator by the conjugate of the denominator, which is \(1-\sqrt{3}\).
- \(\frac{2}{1+\sqrt{3}} \times \frac{1-\sqrt{3}}{1-\sqrt{3}} = \frac{2(1-\sqrt{3})}{(1+\sqrt{3})(1-\sqrt{3})}\)
- In the denominator, use the difference of squares formula: \((a+b)(a-b) = a^2 - b^2\).
- \(\frac{2(1-\sqrt{3})}{1-3} = \frac{2(1-\sqrt{3})}{-2}\)
- Simplify the expression: \(\frac{2-2\sqrt{3}}{-2} = -1+\sqrt{3}\)
Through rationalizing, the denominator is simplified to a rational number or an integer, making the fraction easier to work with.
Simplifying Fractions with Square Roots
Simplifying fractions with square roots involves two main steps: simplifying the square root and rationalizing the denominator. Let's go through these steps in detail.
Step 1: Simplify the Square Root
First, simplify any square roots in the fraction. This involves finding the largest perfect square factor of the number under the square root. For example:
\(\sqrt{72}\) can be simplified as follows:
- \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\)
Step 2: Rationalize the Denominator
If the denominator contains a square root, we need to rationalize it. This means eliminating the square root by multiplying the numerator and denominator by the conjugate of the denominator. The conjugate of a binomial is formed by changing the sign between two terms. For instance, the conjugate of \(\sqrt{3}\) is \(\sqrt{3}\), and for \(\sqrt{3} + 2\), it is \(\sqrt{3} - 2\).
For example:
\(\frac{5}{\sqrt{3}}\) can be rationalized as follows:
- \(\frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
Examples
Let's look at some specific examples:
Example 1: Simplify \(\frac{\sqrt{18}}{3}\)
- Simplify the square root: \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
- Then simplify the fraction: \(\frac{3\sqrt{2}}{3} = \sqrt{2}\)
Therefore, \(\frac{\sqrt{18}}{3}\) simplifies to \(\sqrt{2}\).
Example 2: Simplify \(\frac{2}{\sqrt{12}}\)
- Simplify the square root: \(\sqrt{12} = \sqrt{4 \times 3} = 2\sqrt{3}\)
- Rationalize the denominator: \(\frac{2}{\sqrt{12}} \times \frac{\sqrt{12}}{\sqrt{12}} = \frac{2\sqrt{12}}{12} = \frac{2 \times 2\sqrt{3}}{12} = \frac{2\sqrt{3}}{6} = \frac{\sqrt{3}}{3}\)
Therefore, \(\frac{2}{\sqrt{12}}\) simplifies to \(\frac{\sqrt{3}}{3}\).
Example 3: Simplify \(\frac{\sqrt{6}}{\sqrt{2}}\)
- Simplify the square roots: \(\sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \times \sqrt{3}\)
- Then simplify the fraction: \(\frac{\sqrt{6}}{\sqrt{2}} = \frac{\sqrt{2} \times \sqrt{3}}{\sqrt{2}} = \sqrt{3}\)
Therefore, \(\frac{\sqrt{6}}{\sqrt{2}}\) simplifies to \(\sqrt{3}\).
Practice Problems
Here are some additional examples for practice:
- Simplify \(\frac{3\sqrt{5}}{5}\)
- Simplify \(\frac{4}{\sqrt{18}}\)
Remember, the key steps are to simplify the square root and then rationalize the denominator. With practice, simplifying fractions with square roots will become easier.
Step-by-Step Examples
In this section, we will go through several examples to illustrate the process of simplifying fractions that involve square roots.
Example 1: Simplifying the Square Root of a Fraction
Consider the fraction \(\frac{18}{50}\).
- First, reduce the fraction to its simplest form: \[ \frac{18}{50} = \frac{9}{25} \]
- Next, take the square root of the numerator and the denominator separately: \[ \sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5} \]
Example 2: Simplifying a Mixed Number
Consider the mixed number \(1 \frac{13}{36}\).
- Convert the mixed number to an improper fraction: \[ 1 \frac{13}{36} = \frac{49}{36} \]
- Take the square root of the numerator and the denominator: \[ \sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} \]
- Convert back to a mixed number if necessary: \[ \frac{7}{6} = 1 \frac{1}{6} \]
Example 3: Rationalizing the Denominator
Consider the fraction \(\frac{2}{3 + \sqrt{3}}\).
- Multiply the numerator and the denominator by the conjugate of the denominator: \[ \frac{2}{3 + \sqrt{3}} \times \frac{3 - \sqrt{3}}{3 - \sqrt{3}} = \frac{2(3 - \sqrt{3})}{(3 + \sqrt{3})(3 - \sqrt{3})} \]
- Use the difference of squares to simplify the denominator: \[ (3 + \sqrt{3})(3 - \sqrt{3}) = 3^2 - (\sqrt{3})^2 = 9 - 3 = 6 \]
- Simplify the fraction: \[ \frac{2(3 - \sqrt{3})}{6} = \frac{6 - 2\sqrt{3}}{6} = 1 - \frac{\sqrt{3}}{3} \]
Example 4: Simplifying a More Complex Fraction
Consider the fraction \(\frac{\sqrt{6} - \sqrt{2}}{\sqrt{6} + \sqrt{2}}\).
- Multiply the numerator and the denominator by the conjugate of the denominator: \[ \frac{\sqrt{6} - \sqrt{2}}{\sqrt{6} + \sqrt{2}} \times \frac{\sqrt{6} - \sqrt{2}}{\sqrt{6} - \sqrt{2}} = \frac{(\sqrt{6} - \sqrt{2})^2}{(\sqrt{6})^2 - (\sqrt{2})^2} \]
- Simplify the numerator and the denominator: \[ (\sqrt{6} - \sqrt{2})^2 = 6 - 2\sqrt{12} + 2 = 8 - 4\sqrt{3} \] \[ (\sqrt{6})^2 - (\sqrt{2})^2 = 6 - 2 = 4 \]
- Simplify the fraction: \[ \frac{8 - 4\sqrt{3}}{4} = 2 - \sqrt{3} \]

Special Cases
When simplifying fractions that involve square roots, there are several special cases to consider. Here, we’ll explore these special cases and provide detailed steps to simplify them.
Case 1: Numerator or Denominator is a Square Root
When the numerator or the denominator is a square root, the fraction can often be simplified by rationalizing the denominator. Here are the steps:
- Multiply the numerator and the denominator by the conjugate of the denominator if it is a binomial involving a square root.
- Simplify the resulting expression.
Example:
Simplify
- Multiply by the conjugate of the denominator:
- Result:
Case 2: Both Numerator and Denominator are Square Roots
When both the numerator and the denominator are square roots, simplify each separately and then simplify the fraction if possible.
Example:
Simplify
- Simplify the square roots:
- Simplify the fraction:
Case 3: Fraction Involves a Mixed Radical
For fractions involving mixed radicals, rationalize the denominator by multiplying by the conjugate.
Example:
Simplify
- Multiply by the conjugate of the denominator:
- Simplify the expression:
Case 4: Square Root of a Fraction
To simplify the square root of a fraction, apply the square root separately to the numerator and the denominator.
Example:
Simplify
- Separate the square root:
- Simplify the fraction:
These special cases highlight different scenarios where simplifying fractions involving square roots can be challenging but manageable with the right approach. Practice these methods to become more proficient in handling such problems.
Square Roots of Improper Fractions
To simplify the square root of an improper fraction, we follow a systematic approach to make the process clear and manageable. Let's break down the steps involved:
- Convert to an Improper Fraction:
If you have a mixed number, convert it to an improper fraction first. For example:
- \(1\frac{7}{9}\) converts to \(\frac{16}{9}\).
- Apply the Square Root to the Fraction:
Apply the square root to both the numerator and the denominator separately. This utilizes the property:
\[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]
- Example: \(\sqrt{\frac{16}{9}} = \frac{\sqrt{16}}{\sqrt{9}} = \frac{4}{3}\).
- Simplify the Result:
After applying the square root, simplify the fraction if possible. In the example above, \(\frac{4}{3}\) is already in its simplest form.
Example Problems
Let's go through a few more examples to reinforce the concept:
- Example 1: Simplify \(\sqrt{\frac{25}{49}}\)
- Step 1: Apply the square root to both numerator and denominator: \(\sqrt{\frac{25}{49}} = \frac{\sqrt{25}}{\sqrt{49}}\)
- Step 2: Simplify the square roots: \(\frac{\sqrt{25}}{\sqrt{49}} = \frac{5}{7}\)
- Example 2: Simplify \(\sqrt{2\frac{9}{16}}\)
- Step 1: Convert to an improper fraction: \(2\frac{9}{16} = \frac{41}{16}\)
- Step 2: Apply the square root: \(\sqrt{\frac{41}{16}} = \frac{\sqrt{41}}{\sqrt{16}}\)
- Step 3: Simplify the denominator: \(\frac{\sqrt{41}}{4}\)
Special Case: Non-Perfect Squares
When dealing with fractions where the numerator or denominator is not a perfect square, the result may remain in radical form:
- Example: Simplify \(\sqrt{\frac{7}{2}}\)
- Step 1: Apply the square root to both parts: \(\sqrt{\frac{7}{2}} = \frac{\sqrt{7}}{\sqrt{2}}\)
- Step 2: Rationalize the denominator (if required): \(\frac{\sqrt{7}}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{14}}{2}\)
Practice Problems
Try simplifying these on your own:
- \(\sqrt{\frac{45}{81}}\)
- \(\sqrt{3\frac{4}{9}}\)
- \(\sqrt{\frac{50}{72}}\)
Remember, the key steps are to apply the square root to both the numerator and denominator and then simplify the resulting fraction!
Mixed Numbers and Square Roots
When working with mixed numbers and square roots, the process typically involves converting the mixed number into an improper fraction, simplifying the square root, and then simplifying the fraction if necessary. Here’s a detailed step-by-step guide:
Step-by-Step Guide
-
Convert Mixed Number to Improper Fraction
Convert the mixed number to an improper fraction. For example, to convert \(1\frac{3}{4}\):
- Multiply the whole number by the denominator: \(1 \times 4 = 4\)
- Add the numerator to this product: \(4 + 3 = 7\)
- The improper fraction is \(\frac{7}{4}\)
-
Apply the Square Root
Apply the square root to both the numerator and the denominator of the improper fraction. Using our example:
\[
\sqrt{\frac{7}{4}} = \frac{\sqrt{7}}{\sqrt{4}} = \frac{\sqrt{7}}{2}
\] -
Simplify the Result
Simplify the resulting fraction if possible. In this case, \(\frac{\sqrt{7}}{2}\) is already in its simplest form.
Examples
-
Convert \(2\frac{1}{9}\) to an improper fraction:
\[
2 \frac{1}{9} = \frac{19}{9}
\]Apply the square root:
\[
\sqrt{\frac{19}{9}} = \frac{\sqrt{19}}{3}
\] -
Convert \(1\frac{13}{36}\) to an improper fraction:
\[
1 \frac{13}{36} = \frac{49}{36}
\]Apply the square root:
\[
\sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6}
\]
Practice Problems
- Simplify \(\sqrt{1\frac{3}{16}}\)
- Simplify \(\sqrt{3\frac{4}{25}}\)
By following these steps, you can effectively simplify square roots of mixed numbers, making complex fractions more manageable.
Quotient Rule for Square Roots
The Quotient Rule for Square Roots states that for any non-negative real numbers \(a\) and \(b\), where \(b \ne 0\), the square root of a fraction is the fraction of the square roots:
\[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]
This property allows us to simplify complex square roots involving fractions by breaking them down into simpler components.
Steps to Simplify Using the Quotient Rule
- Rewrite the expression: Start by expressing the square root of the fraction as the fraction of the square roots.
- Simplify the numerator and denominator separately: Simplify the square roots in the numerator and the denominator individually.
- Combine the results: After simplifying, combine the results back into a single fraction.
Example 1
Simplify \(\sqrt{\frac{21}{64}}\).
- Rewrite using the quotient rule: \(\sqrt{\frac{21}{64}} = \frac{\sqrt{21}}{\sqrt{64}}\).
- Simplify the denominator: \(\sqrt{64} = 8\).
- Combine the results: \(\frac{\sqrt{21}}{8}\).
Example 2
Simplify \(\sqrt{\frac{27m^3}{196}}\).
- Rewrite using the quotient rule: \(\sqrt{\frac{27m^3}{196}} = \frac{\sqrt{27m^3}}{\sqrt{196}}\).
- Simplify the numerator and the denominator: \(\sqrt{27m^3} = 3m^{3/2} \sqrt{3}\) and \(\sqrt{196} = 14\).
- Combine the results: \(\frac{3m^{3/2} \sqrt{3}}{14}\).
Example 3
Simplify \(\frac{\sqrt{48}}{\sqrt{108}}\).
- Rewrite using the quotient rule: \(\frac{\sqrt{48}}{\sqrt{108}} = \sqrt{\frac{48}{108}}\).
- Simplify the fraction inside the radical: \(\frac{48}{108} = \frac{4}{9}\).
- Find the square roots: \(\sqrt{\frac{4}{9}} = \frac{2}{3}\).
Important Notes
- The Quotient Rule can be used in reverse to combine square roots into a single fraction if needed.
- Always simplify the fraction inside the square root first if possible to make further simplification easier.

Common Mistakes to Avoid
Simplifying square roots in fractions can be tricky, and there are several common mistakes that students often make. By being aware of these pitfalls, you can enhance your accuracy and avoid errors.
- Not Simplifying the Square Root Completely: Ensure you simplify the square root as much as possible. This involves breaking down the number into its prime factors and extracting any perfect squares.
- Rationalizing the Denominator Incorrectly: When rationalizing the denominator, remember to multiply both the numerator and the denominator by the necessary square root to eliminate the square root from the denominator completely.
- Forgetting to Simplify the Fraction: After simplifying the square root and rationalizing the denominator, always check if the fraction itself can be reduced to its lowest terms.
- Mixing Up Operations: Keep the order of operations in mind. Simplify square roots before performing operations with fractions, and be careful with multiplication and division steps.
- Overlooking Negative Signs: Pay attention to negative signs, especially when dealing with square roots. A negative square root can significantly change the problem's outcome.
By being mindful of these common mistakes and applying the correct simplification techniques, you can confidently simplify square roots in fractions and tackle more complex mathematical problems.
Practice Problems
Practice simplifying square roots with fractions by working through the following problems. These exercises will help you master the techniques of simplifying, rationalizing, and combining square roots with fractions.
-
Simplify the following square root fractions:
- \(\sqrt{\frac{49}{64}}\)
- \(\sqrt{\frac{18}{50}}\)
- \(\sqrt{\frac{72}{98}}\)
Solutions:
- \(\sqrt{\frac{49}{64}} = \frac{\sqrt{49}}{\sqrt{64}} = \frac{7}{8}\)
- \(\sqrt{\frac{18}{50}} = \sqrt{\frac{9 \cdot 2}{25 \cdot 2}} = \sqrt{\frac{9}{25}} = \frac{3}{5}\)
- \(\sqrt{\frac{72}{98}} = \sqrt{\frac{36 \cdot 2}{49 \cdot 2}} = \sqrt{\frac{36}{49}} = \frac{6}{7}\)
-
Rationalize the denominator of the following expressions:
- \(\frac{5}{\sqrt{3}}\)
- \(\frac{2}{\sqrt{12}}\)
- \(\frac{7}{\sqrt{2}}\)
Solutions:
- \(\frac{5}{\sqrt{3}} = \frac{5 \cdot \sqrt{3}}{\sqrt{3} \cdot \sqrt{3}} = \frac{5\sqrt{3}}{3}\)
- \(\frac{2}{\sqrt{12}} = \frac{2 \cdot \sqrt{12}}{\sqrt{12} \cdot \sqrt{12}} = \frac{2\sqrt{12}}{12} = \frac{\sqrt{3}}{3}\)
- \(\frac{7}{\sqrt{2}} = \frac{7 \cdot \sqrt{2}}{\sqrt{2} \cdot \sqrt{2}} = \frac{7\sqrt{2}}{2}\)
-
Simplify the following mixed numbers with square roots:
- \(\sqrt{1\frac{13}{36}}\)
- \(\sqrt{2\frac{25}{49}}\)
- \(\sqrt{3\frac{9}{16}}\)
Solutions:
- \(\sqrt{1\frac{13}{36}} = \sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} = 1\frac{1}{6}\)
- \(\sqrt{2\frac{25}{49}} = \sqrt{\frac{123}{49}} = \frac{\sqrt{123}}{\sqrt{49}} = \frac{\sqrt{123}}{7}\)
- \(\sqrt{3\frac{9}{16}} = \sqrt{\frac{57}{16}} = \frac{\sqrt{57}}{\sqrt{16}} = \frac{\sqrt{57}}{4}\)
These practice problems are designed to build your confidence and skill in simplifying square roots with fractions. Keep practicing to improve your proficiency!
Advanced Techniques
In this section, we will explore some advanced techniques for simplifying square roots with fractions. These methods can help streamline complex calculations and improve your mathematical accuracy.
Using the Product Rule
The product rule for square roots states that for any non-negative numbers \(a\) and \(b\), the square root of their product is the product of their square roots:
\[\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\]
This rule can be particularly useful when dealing with square roots of fractions where both the numerator and the denominator can be factored into simpler terms.
Using the Quotient Rule
The quotient rule for square roots allows us to separate the square root of a fraction into the square roots of the numerator and the denominator:
\[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]
Applying this rule can simplify the process of rationalizing the denominator and making further simplifications.
Simplifying Complex Fractions
When simplifying complex fractions involving square roots, the following steps can be helpful:
- Apply the quotient rule to separate the square root of the fraction.
- Simplify the square roots of the numerator and the denominator individually.
- If the denominator still contains a square root, rationalize it by multiplying both the numerator and the denominator by the square root present in the denominator.
- Simplify the resulting fraction by combining like terms and reducing it to its lowest terms.
Example: Simplifying \(\sqrt{\frac{72}{18}}\)
- Apply the quotient rule: \[\sqrt{\frac{72}{18}} = \frac{\sqrt{72}}{\sqrt{18}}\]
- Simplify each square root: \[\sqrt{72} = \sqrt{36 \cdot 2} = 6\sqrt{2}\] and \[\sqrt{18} = \sqrt{9 \cdot 2} = 3\sqrt{2}\]
- Combine the results: \[\frac{6\sqrt{2}}{3\sqrt{2}} = \frac{6}{3} = 2\]
- Thus, \(\sqrt{\frac{72}{18}} = 2\).
Rationalizing the Denominator
Rationalizing the denominator involves removing any square roots from the denominator of a fraction. This can be done by multiplying both the numerator and the denominator by the appropriate form of 1 to eliminate the square root.
For example, to rationalize \(\frac{1}{\sqrt{2}}\):
- Multiply both the numerator and the denominator by \(\sqrt{2}\): \[\frac{1}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}\]
Handling Higher-Order Roots
For higher-order roots, such as cube roots or fourth roots, similar rules apply. For instance, the product and quotient rules for cube roots are:
\[\sqrt[3]{a \cdot b} = \sqrt[3]{a} \cdot \sqrt[3]{b}\] and \[\sqrt[3]{\frac{a}{b}} = \frac{\sqrt[3]{a}}{\sqrt[3]{b}}\]
These techniques can be extended to roots of any order, making them versatile tools in simplifying complex expressions.
Practice Problems
- Simplify \(\sqrt{\frac{50}{2}}\).
- Simplify \(\frac{3}{\sqrt{5}}\) by rationalizing the denominator.
- Apply the quotient rule to simplify \(\sqrt{\frac{80}{16}}\).
By mastering these advanced techniques, you can tackle more challenging problems with confidence and ease.
Applications in Real Life
Simplifying square roots with fractions is not just a mathematical exercise; it has numerous practical applications in various fields. Here are some examples:
-
Architecture and Construction:
Architects and construction workers frequently use square roots and fractions to determine accurate measurements. For example, the Pythagorean theorem, which involves square roots, is used to calculate the lengths of sides in right-angled triangles, essential in designing buildings and other structures.
-
Engineering:
Electrical engineers use radical expressions to calculate power and resistance in electrical circuits. For instance, the formula \(V = \sqrt{PR}\) (where \(V\) is voltage, \(P\) is power, and \(R\) is resistance) involves square roots and fractions to ensure precise measurements and functionality of electrical systems.
-
Finance:
In finance, radical expressions and fractions are used to calculate compound interest, depreciation, and inflation rates. For example, the formula for compound interest \(A = P(1+\frac{r}{n})^{nt}\) requires understanding and simplifying fractions and roots to determine the amount of interest earned over time.
-
Biology:
Biologists use square roots and fractions to compare different animal sizes and surface areas. This is crucial in studies involving metabolic rates, where the surface area-to-volume ratio plays a significant role.
-
Cooking and Recipes:
Recipes often require measurements involving fractions, such as \(\frac{1}{2}\) cup or \(\frac{3}{4}\) teaspoon. Understanding how to simplify these fractions, especially when scaling recipes up or down, is essential for accurate cooking.
-
Fitness and Health:
In fitness, square roots and fractions are used to calculate body mass index (BMI) and other health-related metrics. For example, the BMI formula \(BMI = \frac{weight}{height^2}\) involves squaring the height (a square root operation when inverted) and dividing, showcasing the practical application of these mathematical concepts.
These examples highlight the importance of understanding and simplifying square roots with fractions, as they are integral to various real-life applications across different fields.
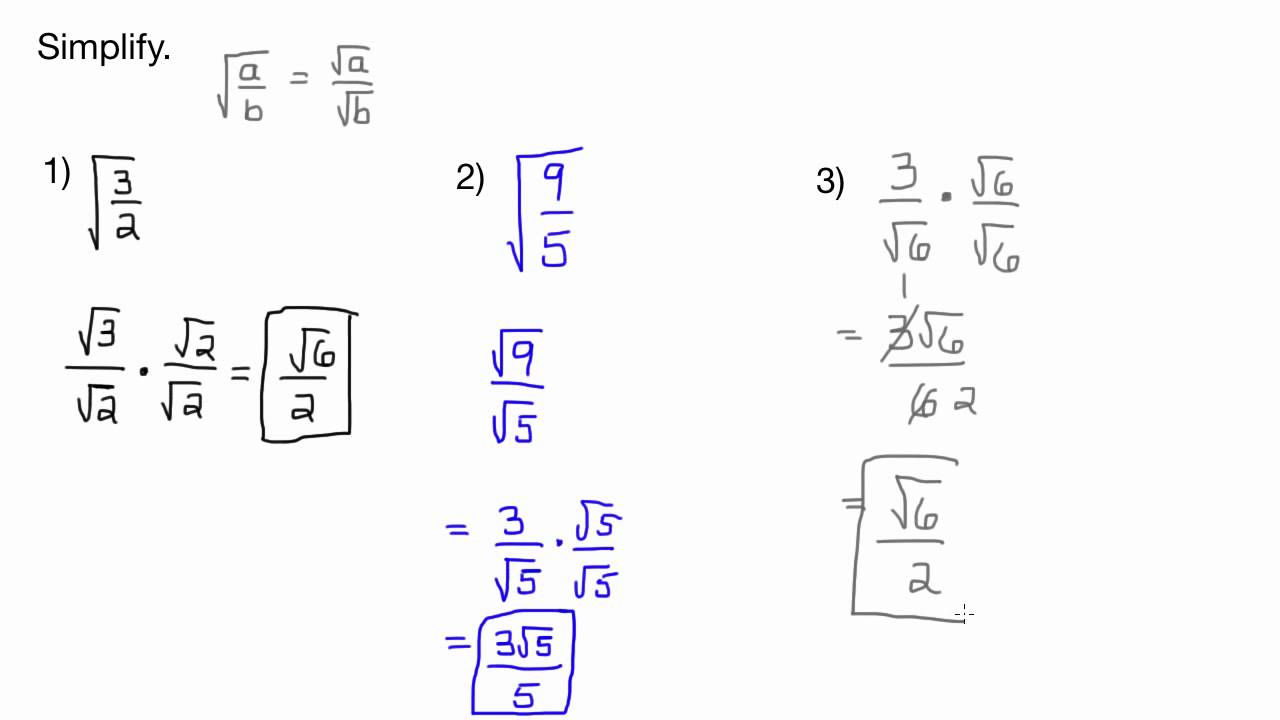
Frequently Asked Questions
Here are some commonly asked questions about simplifying square roots with fractions:
- Q: How do I simplify a fraction with a square root in the numerator?
A: To simplify a fraction with a square root in the numerator, first simplify the square root if possible. For example, to simplify \( \frac{\sqrt{18}}{3} \), we start by simplifying the square root:
\( \sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2} \)
Then, divide by the denominator:
\( \frac{3\sqrt{2}}{3} = \sqrt{2} \)
- Q: What does it mean to rationalize the denominator?
A: Rationalizing the denominator involves eliminating the square root from the denominator of a fraction. To do this, multiply both the numerator and the denominator by the conjugate of the denominator. For example, to rationalize \( \frac{5}{\sqrt{3}} \), multiply by \( \frac{\sqrt{3}}{\sqrt{3}} \):
\( \frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3} \)
- Q: How do I simplify a fraction with square roots in both the numerator and the denominator?
A: Simplify each square root separately and then divide. For example, to simplify \( \frac{\sqrt{18}}{\sqrt{2}} \), we start by simplifying the square roots:
\( \sqrt{18} = 3\sqrt{2} \) and \( \sqrt{2} \) remains as it is.
Then, divide:
\( \frac{3\sqrt{2}}{\sqrt{2}} = 3 \)
- Q: What if the fraction involves a mixed number?
A: Convert the mixed number to an improper fraction before simplifying. For example, to simplify \( \sqrt{1\frac{13}{36}} \):
First, convert the mixed number:
\( 1\frac{13}{36} = \frac{49}{36} \)
Then find the square root of the improper fraction:
\( \sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} = 1\frac{1}{6} \)
- Q: Are there any special rules for simplifying square roots with fractions?
A: Yes, always look for perfect square factors in the numerator and the denominator to simplify the square roots. Use the quotient rule, which states that \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \). This can often make the simplification process easier.
Conclusion
Simplifying square roots that involve fractions can initially seem challenging, but with a systematic approach, it becomes a manageable task. Here are the key steps and takeaways to remember:
- Understand the Basics: Ensure a solid grasp of both square roots and fractions independently before combining them.
- Factor and Simplify: Factor both the numerator and the denominator to their prime factors. Simplify the fraction as much as possible before taking the square root.
- Apply the Quotient Rule: Utilize the quotient rule for square roots, which states that \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\). This allows you to separate the square root of the fraction into two simpler square roots.
- Rationalize the Denominator: If the denominator contains a square root, multiply both the numerator and the denominator by a value that will eliminate the square root from the denominator. For example, for \(\frac{1}{\sqrt{2}}\), multiply by \(\frac{\sqrt{2}}{\sqrt{2}}\) to get \(\frac{\sqrt{2}}{2}\).
- Special Cases: Pay attention to mixed numbers and improper fractions. Convert mixed numbers to improper fractions before simplifying. For improper fractions, simplify them to their lowest terms before applying the square root.
Let's look at a comprehensive example:
Example: Simplify \(\sqrt{\frac{18}{50}}\)
- Step 1: Simplify the fraction: \(\frac{18}{50} = \frac{9}{25}\)
- Step 2: Apply the quotient rule: \(\sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5}\)
Through these steps, you can simplify even the most complex fractions involving square roots. Remember, practice is essential to master these techniques, so continually work on problems to build confidence.
Simplifying square roots with fractions is not just an academic exercise but a valuable skill in various real-life applications, such as engineering, physics, and computer science. Understanding and mastering these principles will serve you well in both academic and professional settings.
Xem video này để học cách đơn giản hóa phân số dưới dấu căn bậc hai một cách dễ dàng. Hãy cùng khám phá phương pháp đơn giản hóa phân số với căn bậc hai.
Đơn giản hóa một phân số dưới dấu căn bậc hai - "Gian lận hợp pháp cho toán học"
READ MORE:
Khám phá cách đơn giản hóa căn bậc hai của phân số trong video này. Học cách giải quyết sqrt(a/b) và a/sqrt(b) một cách dễ dàng.
Đơn giản hóa căn bậc hai của phân số: sqrt(a/b), a/sqrt(b)
















