Topic simplify square root 50: Simplifying the square root of 50 may seem challenging, but with the right approach, it becomes a straightforward task. This guide will walk you through the process step-by-step, ensuring you understand how to simplify √50 to its simplest form, 5√2. Let's dive into the fascinating world of square roots!
Table of Content
- Simplifying the Square Root of 50
- Introduction to Simplifying Square Roots
- Understanding Prime Factorization
- Step-by-Step Guide to Simplifying √50
- Breaking Down the Number 50
- Identifying Perfect Squares
- Applying the Square Root Property
- Simplifying √50 to 5√2
- Visual Representation of the Simplification Process
- Common Mistakes to Avoid
- Practice Problems and Solutions
- Conclusion and Summary
- Additional Resources and References
- YOUTUBE:
Simplifying the Square Root of 50
The process of simplifying the square root of 50 involves breaking it down into its prime factors and simplifying the expression by identifying perfect squares.
Step-by-Step Solution
- Find the prime factorization of 50.
- Identify pairs of prime factors.
- Rewrite the square root using the identified pairs.
- Simplify the expression by taking the square root of the perfect square.
Detailed Breakdown
- Prime factorization of 50: \( 50 = 2 \times 25 \)
- Prime factorization of 25: \( 25 = 5 \times 5 \)
- Thus, \( 50 = 2 \times 5^2 \)
Using the property of square roots \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \):
\( \sqrt{50} = \sqrt{2 \times 5^2} = \sqrt{2} \times \sqrt{5^2} = \sqrt{2} \times 5 \)
Therefore, the simplified form of \( \sqrt{50} \) is \( 5\sqrt{2} \).
Conclusion
By breaking down the number 50 into its prime factors and simplifying the square root, we find that the simplified form of \( \sqrt{50} \) is \( 5\sqrt{2} \).
| Original Expression | Simplified Form |
| \( \sqrt{50} \) | \( 5\sqrt{2} \) |

READ MORE:
Introduction to Simplifying Square Roots
Simplifying square roots is an essential skill in mathematics that helps in reducing complex expressions to their simplest form. By understanding the process of breaking down numbers into their prime factors, you can easily simplify square roots. In this guide, we will focus on simplifying the square root of 50 step-by-step.
The basic idea is to factor the number under the square root into its prime factors and then apply the square root to each factor. Let's start with the key steps:
- Identify the prime factors of the number.
- Group the prime factors into pairs.
- Take the square root of each pair.
- Multiply the results to get the simplified form.
Let's apply these steps to simplify the square root of 50:
- Step 1: Prime factorization of 50: \( 50 = 2 \times 25 \)
- Step 2: Prime factorization of 25: \( 25 = 5 \times 5 \)
- Thus, \( 50 = 2 \times 5^2 \)
Using the property of square roots, \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \):
\( \sqrt{50} = \sqrt{2 \times 5^2} = \sqrt{2} \times \sqrt{5^2} = \sqrt{2} \times 5 \)
Therefore, the simplified form of \( \sqrt{50} \) is \( 5\sqrt{2} \).
| Original Expression | Simplified Form |
| \( \sqrt{50} \) | \( 5\sqrt{2} \) |
Understanding Prime Factorization
Prime factorization is the process of breaking down a composite number into its prime factors, which are prime numbers that multiply together to give the original number. This method is crucial for simplifying square roots as it allows us to identify perfect squares within the number.
Let's go through the steps of prime factorization:
- Identify the smallest prime number: Start with the smallest prime number, which is 2.
- Divide the number: If the number is divisible by 2, divide it by 2 and continue dividing by 2 until it is no longer divisible. Then, move to the next smallest prime number, which is 3, and repeat the process.
- Continue the process: Continue this process with the next smallest primes (5, 7, 11, etc.) until the result is a prime number.
Let’s apply this to the number 50:
- 50 is an even number, so it is divisible by 2.
- 50 ÷ 2 = 25
- 25 is not divisible by 2, so we move to the next prime number, which is 3. 25 is not divisible by 3, so we move to the next prime number, which is 5.
- 25 ÷ 5 = 5
- 5 is a prime number, so we stop here.
Thus, the prime factorization of 50 is:
\( 50 = 2 \times 5 \times 5 = 2 \times 5^2 \)
| Number | Prime Factors |
| 50 | 2, 5, 5 |
| Prime Factorization | \( 2 \times 5^2 \) |
By understanding prime factorization, you can simplify square roots more efficiently. For instance, in the case of \(\sqrt{50}\), recognizing the prime factors helps us see that \( 50 = 2 \times 5^2 \), leading to the simplified form \( 5\sqrt{2} \).
Step-by-Step Guide to Simplifying √50
Simplifying the square root of 50 involves breaking down the number into its prime factors and using properties of square roots to simplify the expression. Here is a detailed, step-by-step guide to simplify \( \sqrt{50} \).
- Find the prime factorization of 50:
- Start by dividing 50 by the smallest prime number, which is 2.
- 50 ÷ 2 = 25
- Next, divide 25 by the smallest prime number greater than 2, which is 3. Since 25 is not divisible by 3, we try the next prime number, which is 5.
- 25 ÷ 5 = 5
- Now, 5 is a prime number, so we stop here.
Thus, the prime factors of 50 are 2 and 5.
- Express 50 as a product of its prime factors:
\( 50 = 2 \times 5 \times 5 = 2 \times 5^2 \)
- Apply the square root to each factor:
Using the property of square roots, \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \), we can rewrite the square root of 50 as:
\( \sqrt{50} = \sqrt{2 \times 5^2} \)
- Simplify the square root expression:
Since 5 is a perfect square, we can simplify \( \sqrt{5^2} \) to 5. Therefore:
\( \sqrt{50} = \sqrt{2} \times \sqrt{5^2} = \sqrt{2} \times 5 \)
Simplifying further, we get:
\( \sqrt{50} = 5\sqrt{2} \)
Thus, the simplified form of \( \sqrt{50} \) is \( 5\sqrt{2} \).
| Original Expression | Simplified Form |
| \( \sqrt{50} \) | \( 5\sqrt{2} \) |
Breaking Down the Number 50
To simplify the square root of 50, we first need to break down the number into its prime factors. This involves finding the prime numbers that multiply together to give 50. Here’s a detailed step-by-step breakdown of the number 50:
- Identify the smallest prime number:
The smallest prime number is 2. We start by checking if 50 is divisible by 2.
- Divide 50 by 2:
50 is an even number, so it is divisible by 2. We divide 50 by 2:
\( 50 \div 2 = 25 \)
- Check the result (25) for further prime factors:
Now, we need to factorize 25. The next smallest prime number is 3, but 25 is not divisible by 3. We move to the next prime number, which is 5.
25 is divisible by 5. We divide 25 by 5:
\( 25 \div 5 = 5 \)
- Identify remaining prime factor:
The result is 5, which is a prime number. So, we stop here.
Therefore, the prime factorization of 50 is:
\( 50 = 2 \times 5 \times 5 \) or \( 50 = 2 \times 5^2 \)
Breaking down the number 50 into its prime factors helps us simplify the square root. We use these prime factors in the next steps to find the simplified form of \( \sqrt{50} \).
| Number | Prime Factors |
| 50 | 2, 5, 5 |
| Prime Factorization | \( 2 \times 5^2 \) |
By understanding the prime factorization of 50, we can more easily simplify the square root of 50 to its simplest form, \( 5\sqrt{2} \).

Identifying Perfect Squares
To simplify the square root of a number, it is essential to identify and factor out perfect squares. A perfect square is a number that is the square of an integer. Recognizing these within a number can simplify the square root process significantly. Here is a step-by-step method to identify perfect squares in the number 50:
- List the prime factorization of the number:
From our previous breakdown, we know the prime factors of 50 are:
\( 50 = 2 \times 5^2 \)
- Identify any repeated factors:
In the factorization \( 2 \times 5^2 \), the number 5 is repeated twice. This repetition indicates a perfect square, as \( 5^2 = 25 \) is a perfect square.
- Extract the perfect square from the factorization:
We can rewrite the expression to highlight the perfect square:
\( 50 = 2 \times (5^2) \)
- Apply the square root property:
Using the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \), we simplify the square root of the expression:
\( \sqrt{50} = \sqrt{2 \times 5^2} = \sqrt{2} \times \sqrt{5^2} \)
Since \( \sqrt{5^2} = 5 \), we further simplify to:
\( \sqrt{50} = \sqrt{2} \times 5 \)
Thus, we get:
\( \sqrt{50} = 5\sqrt{2} \)
Identifying and extracting perfect squares from a number allows for the simplification of square roots. In this case, recognizing that 25 is a perfect square within 50 enables us to simplify \( \sqrt{50} \) to \( 5\sqrt{2} \).
| Original Expression | Perfect Square Identified | Simplified Form |
| \( \sqrt{50} \) | \( 25 = 5^2 \) | \( 5\sqrt{2} \) |
Applying the Square Root Property
To simplify the square root of a number, we use the square root property. This property states that the square root of a product is equal to the product of the square roots of the factors. This can be expressed as \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \). Let’s apply this property step-by-step to simplify \( \sqrt{50} \).
- Write the prime factorization:
From our previous breakdowns, we know:
\( 50 = 2 \times 5^2 \)
- Apply the square root property:
Using the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \), we can rewrite the square root of 50:
\( \sqrt{50} = \sqrt{2 \times 5^2} \)
- Separate the factors inside the square root:
We can break it down further:
\( \sqrt{50} = \sqrt{2} \times \sqrt{5^2} \)
- Simplify the perfect square:
Since \( \sqrt{5^2} = 5 \), we can simplify the expression:
\( \sqrt{50} = \sqrt{2} \times 5 \)
Thus, we get:
\( \sqrt{50} = 5\sqrt{2} \)
By applying the square root property, we can efficiently simplify complex square root expressions. For instance, recognizing that \( 50 = 2 \times 5^2 \) allows us to transform \( \sqrt{50} \) into the simpler form \( 5\sqrt{2} \).
| Original Expression | Square Root Property Applied | Simplified Form |
| \( \sqrt{50} \) | \( \sqrt{2 \times 5^2} \) | \( 5\sqrt{2} \) |
Simplifying √50 to 5√2
Simplifying the square root of 50 involves breaking down the number into its prime factors and identifying perfect squares. Follow these detailed steps to simplify √50 to 5√2:
- Prime Factorization:
Start by finding the prime factors of 50. The prime factors are:
- 50 = 2 × 25
- 25 = 5 × 5
- So, 50 = 2 × 5 × 5
- Identify Perfect Squares:
Look for pairs of prime factors. In this case, we have one pair of 5's:
- \(5 × 5 = 25\), which is a perfect square.
- Apply the Square Root Property:
Use the property that √(a × b) = √a × √b to separate the perfect square from the other factors:
- √50 = √(2 × 5 × 5)
- √50 = √(5² × 2)
- Simplify the Expression:
Since the square root of a perfect square is a whole number, simplify the expression:
- √(5² × 2) = 5 × √2
- Therefore, √50 = 5√2
So, the simplified form of √50 is 5√2.
Visual Representation of the Simplification Process
To better understand the simplification of √50 to 5√2, let's visualize each step of the process. We'll break down the steps and provide a visual guide to illustrate how we arrive at the simplified form.
- Prime Factorization:
First, find the prime factors of 50:
50 = 2 × 25 25 = 5 × 5 So, 50 = 2 × 5 × 5 This can be visualized as:
\( \sqrt{50} = \sqrt{2 \times 5 \times 5} \)
- Group the Factors:
Next, group the factors to identify perfect squares:
\( \sqrt{2 \times 5 \times 5} = \sqrt{(5 \times 5) \times 2} \)
- Separate the Perfect Square:
Extract the perfect square (5 × 5 = 25) from the square root:
\( \sqrt{(5 \times 5) \times 2} = \sqrt{5^2 \times 2} \)
This can be separated as:
\( \sqrt{5^2} \times \sqrt{2} \)
- Simplify the Expression:
Since the square root of 5² is 5, we simplify the expression to:
\( \sqrt{5^2} \times \sqrt{2} = 5 \times \sqrt{2} \)
So, \( \sqrt{50} = 5\sqrt{2} \)
This visual representation helps to clearly see the steps involved in simplifying √50 to 5√2.

Common Mistakes to Avoid
When simplifying the square root of 50, there are several common mistakes that students often make. Here are the most frequent errors and how to avoid them:
- Incorrect Prime Factorization:
One of the first steps is to correctly factor the number into its prime components. An error here can lead to incorrect simplification.
- For example, incorrectly writing the factorization as 50 = 5 × 10 instead of the correct 50 = 2 × 25 or 50 = 2 × 5 × 5.
Correct Prime Factorization: \( 50 = 2 \times 5 \times 5 \)
- Misidentifying Perfect Squares:
Ensure that you correctly identify and separate perfect squares within the prime factors.
- Incorrect: \( \sqrt{50} = \sqrt{2 \times 25} \)
- Correct: \( \sqrt{50} = \sqrt{2 \times 5^2} \)
- Forgetting to Simplify Completely:
After identifying the perfect square, make sure to fully simplify the square root.
- Incorrect: \( \sqrt{2 \times 5^2} = \sqrt{2} \times \sqrt{5^2} = \sqrt{2} \times 5 \)
- Correct: \( \sqrt{2 \times 5^2} = \sqrt{2} \times \sqrt{5^2} = 5\sqrt{2} \)
- Not Using the Square Root Property Properly:
Make sure to apply the square root property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \) correctly.
- Incorrect: \( \sqrt{50} = \sqrt{25 + 25} = \sqrt{25} + \sqrt{25} \)
- Correct: \( \sqrt{50} = \sqrt{2 \times 5^2} = \sqrt{2} \times \sqrt{5^2} = 5\sqrt{2} \)
- Misinterpreting the Final Answer:
Ensure that the final simplified form is presented correctly.
- Incorrect: \( \sqrt{50} = 5\sqrt{2} \) with misplaced radicals or coefficients
- Correct: \( \sqrt{50} = 5\sqrt{2} \) with the coefficient outside the radical
By being aware of these common mistakes, you can ensure accurate simplification of square roots and avoid errors in your calculations.
Practice Problems and Solutions
To reinforce your understanding of simplifying square roots, here are some practice problems along with detailed solutions. Follow the steps to simplify each square root:
- Problem 1: Simplify \( \sqrt{72} \)
Solution:
- Prime Factorization: \( 72 = 2 \times 36 \)
- Factor further: \( 36 = 6 \times 6 = 2 \times 3 \times 2 \times 3 \)
- Combine factors: \( 72 = 2^3 \times 3^2 \)
- Identify perfect squares: \( \sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} \)
- Simplify: \( \sqrt{72} = 6 \sqrt{2} \)
Answer: \( \sqrt{72} = 6 \sqrt{2} \)
- Problem 2: Simplify \( \sqrt{18} \)
Solution:
- Prime Factorization: \( 18 = 2 \times 9 \)
- Factor further: \( 9 = 3 \times 3 \)
- Combine factors: \( 18 = 2 \times 3^2 \)
- Identify perfect squares: \( \sqrt{18} = \sqrt{(3^2) \times 2} \)
- Simplify: \( \sqrt{18} = 3 \sqrt{2} \)
Answer: \( \sqrt{18} = 3 \sqrt{2} \)
- Problem 3: Simplify \( \sqrt{200} \)
Solution:
- Prime Factorization: \( 200 = 2 \times 100 \)
- Factor further: \( 100 = 10 \times 10 = 2^2 \times 5^2 \)
- Combine factors: \( 200 = 2^3 \times 5^2 \)
- Identify perfect squares: \( \sqrt{200} = \sqrt{(2^2 \times 5^2) \times 2} \)
- Simplify: \( \sqrt{200} = 10 \sqrt{2} \)
Answer: \( \sqrt{200} = 10 \sqrt{2} \)
- Problem 4: Simplify \( \sqrt{98} \)
Solution:
- Prime Factorization: \( 98 = 2 \times 49 \)
- Factor further: \( 49 = 7 \times 7 \)
- Combine factors: \( 98 = 2 \times 7^2 \)
- Identify perfect squares: \( \sqrt{98} = \sqrt{(7^2) \times 2} \)
- Simplify: \( \sqrt{98} = 7 \sqrt{2} \)
Answer: \( \sqrt{98} = 7 \sqrt{2} \)
These practice problems help solidify the process of simplifying square roots. Practice regularly to enhance your skills and avoid common mistakes.
Conclusion and Summary
Simplifying the square root of 50 is a straightforward process when following a systematic approach. By understanding the steps and practicing regularly, you can simplify any square root with ease. Here is a summary of the key points:
- Prime Factorization:
Break down the number into its prime factors. For 50, this is:
\( 50 = 2 \times 5 \times 5 \)
- Identify Perfect Squares:
Group the factors to find any perfect squares. In this case, \( 5 \times 5 \) forms the perfect square 25:
\( \sqrt{50} = \sqrt{2 \times 5^2} \)
- Apply the Square Root Property:
Use the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \) to separate the perfect square:
\( \sqrt{2 \times 5^2} = \sqrt{2} \times \sqrt{5^2} \)
- Simplify the Expression:
The square root of \( 5^2 \) is 5, so the expression simplifies to:
\( \sqrt{50} = 5 \sqrt{2} \)
In conclusion, simplifying √50 to 5√2 involves breaking down the number into its prime factors, identifying and extracting perfect squares, and applying the square root property to simplify the expression. Regular practice with similar problems can help reinforce these concepts and ensure accuracy in your calculations.
Additional Resources and References
To further enhance your understanding of simplifying square roots, here are some additional resources and references that provide detailed explanations, practice problems, and interactive tools:
- Online Tutorials:
- : Comprehensive video tutorials and practice problems.
- : Step-by-step lessons on simplifying square roots and other radicals.
- Interactive Tools:
- : An interactive tool to visualize and simplify square roots.
- : An online calculator that provides step-by-step solutions for simplifying square roots.
- Practice Worksheets:
- : Printable worksheets with various problems and solutions.
- : A collection of worksheets for additional practice.
- Reference Books:
- Algebra for Dummies by Mary Jane Sterling: A beginner-friendly book with detailed explanations and examples.
- Schaum's Outline of Elementary Algebra by Barnett Rich: A comprehensive guide with numerous practice problems.
These resources will provide you with a solid foundation in simplifying square roots and help you practice and master the concepts. Make use of these tools to improve your skills and confidence in handling square roots.
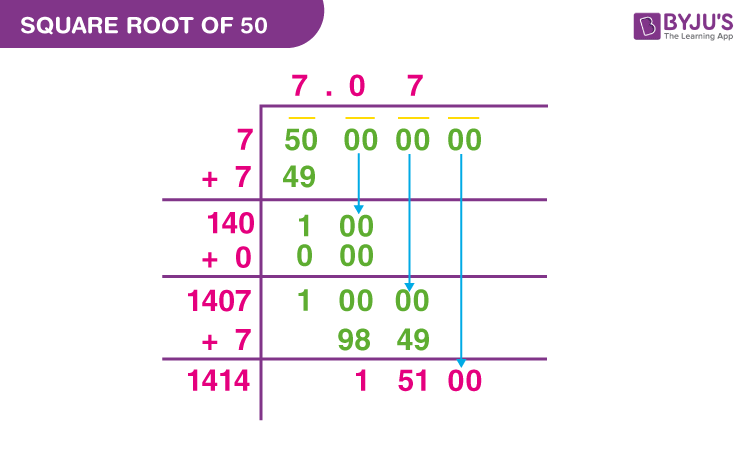
Cách Đơn Giản Hóa Căn Bậc Hai Của 50: Sqrt(50)
READ MORE:
Đơn Giản Hóa Căn Bậc Hai Của Một Số | Đơn Giản Hóa Toán Học, Căn(50)