Topic simplify square root of 6: Learn how to simplify the square root of 6 with our easy step-by-step guide. Discover the prime factorization method and avoid common mistakes to master this fundamental mathematical skill. Perfect for students and math enthusiasts looking to deepen their understanding of square roots and their applications.
Table of Content
- How to Simplify the Square Root of 6
- Introduction to Simplifying Square Roots
- Understanding the Basics of Square Roots
- Step-by-Step Guide to Simplify Square Root of 6
- Prime Factorization Method
- Common Mistakes to Avoid
- Mathematical Representation and Examples
- Approximation of Square Root of 6
- Applications of Simplified Square Roots
- Comparison with Other Square Roots
- Practice Problems and Solutions
- Additional Resources and Tools
- Conclusion
- YOUTUBE: Xem video này để học cách đơn giản hóa căn bậc hai của số 6. Hướng dẫn chi tiết từ cơ bản đến nâng cao.
How to Simplify the Square Root of 6
To simplify the square root of a number, we look for factors that are perfect squares. Unfortunately, 6 does not have any perfect square factors. However, we can express it in its simplest radical form.
Step-by-Step Simplification
- Identify the prime factors of 6.
- 6 = 2 × 3
- Since there are no pairs of prime factors, the square root of 6 cannot be simplified further.
Mathematical Representation
The simplified form of \(\sqrt{6}\) remains as \(\sqrt{6}\).
Alternative Approach
If you need to approximate the value of \(\sqrt{6}\), you can use a calculator:
\(\sqrt{6} \approx 2.449\)
Examples of Using Square Root of 6
Here are some examples of how \(\sqrt{6}\) can be used in mathematical expressions:
- Addition:
\(\sqrt{6} + \sqrt{3} = \sqrt{9} = 3\) - Multiplication:
\(\sqrt{6} \times \sqrt{2} = \sqrt{12} = 2\sqrt{3}\) - Division:
\(\frac{\sqrt{6}}{\sqrt{2}} = \sqrt{3}\)
Table of Square Roots for Comparison
| Number | Square Root | Simplified Form |
|---|---|---|
| 4 | \(\sqrt{4}\) | 2 |
| 5 | \(\sqrt{5}\) | \(\sqrt{5}\) |
| 6 | \(\sqrt{6}\) | \(\sqrt{6}\) |
| 7 | \(\sqrt{7}\) | \(\sqrt{7}\) |

READ MORE:
Introduction to Simplifying Square Roots
Simplifying square roots involves reducing the number inside the radical to its smallest possible whole number. This process is fundamental in mathematics as it helps in solving equations more efficiently and understanding the properties of numbers. To simplify a square root, you need to factorize the number into its prime factors and then apply the square root to each factor.
For example, to simplify \(\sqrt{12}\), follow these steps:
- Factorize 12 into its prime factors: \(12 = 2 \times 2 \times 3\)
- Group the factors into pairs: \(\sqrt{12} = \sqrt{(2 \times 2) \times 3}\)
- Simplify the pairs: \(\sqrt{(2 \times 2) \times 3} = 2\sqrt{3}\)
This method can be applied to any square root to simplify it as much as possible.
Here’s another example with \(\sqrt{45}\):
- Factorize 45 into its prime factors: \(45 = 3 \times 3 \times 5\)
- Group the factors into pairs: \(\sqrt{45} = \sqrt{(3 \times 3) \times 5}\)
- Simplify the pairs: \(\sqrt{(3 \times 3) \times 5} = 3\sqrt{5}\)
The same principles apply when working with fractions and more complex expressions:
- For a fraction like \(\sqrt{\frac{30}{10}}\), you can simplify inside the radical first: \(\sqrt{\frac{30}{10}} = \sqrt{3}\)
- For a product like \(\sqrt{6} \times \sqrt{15}\), combine and factorize: \(\sqrt{6 \times 15} = \sqrt{90} = \sqrt{(3 \times 3) \times 10} = 3\sqrt{10}\)
By mastering these techniques, you'll find it easier to handle various mathematical problems that involve square roots.
Understanding the Basics of Square Roots
Square roots are a fundamental concept in mathematics, often encountered in algebra and geometry. Simplifying square roots involves expressing the square root in its simplest form. This process is essential for solving various mathematical problems efficiently.
To understand square roots, it's important to know that the square root of a number \( x \) is a value that, when multiplied by itself, gives \( x \). For instance, the square root of 9 is 3 because \( 3 \times 3 = 9 \).
Here are some steps to simplify square roots:
- Identify if the number inside the square root is a perfect square (e.g., 4, 9, 16).
- If it is not a perfect square, factor the number into its prime factors.
- Group the prime factors into pairs.
- Take one factor from each pair outside the square root.
For example, to simplify \( \sqrt{18} \):
- Factor 18 into its prime factors: \( 18 = 2 \times 3 \times 3 \).
- Group the prime factors: \( 18 = (3 \times 3) \times 2 \).
- Simplify by taking the square root of the grouped factors: \( \sqrt{18} = \sqrt{3^2 \times 2} = 3\sqrt{2} \).
In the case of the square root of 6, since 6 cannot be factored into perfect squares, it remains \( \sqrt{6} \), which is already in its simplest form.
Understanding these basics helps in solving more complex mathematical problems and lays the foundation for higher-level math concepts.
Step-by-Step Guide to Simplify Square Root of 6
Simplifying the square root of 6 involves breaking it down into its prime factors and checking if any perfect squares can be extracted. Here’s a detailed, step-by-step guide to simplify √6:
- Identify the prime factors of 6:
- 6 can be factored into 2 and 3.
- Thus, 6 = 2 × 3.
- Express the square root of 6 using its prime factors:
- √6 = √(2 × 3).
- Check for any perfect square factors:
- In this case, 2 and 3 are both prime numbers and not perfect squares.
- Simplify the expression (if possible):
- Since there are no perfect square factors in 2 and 3, √6 is already in its simplest form.
- Verify the result using a calculator:
- Calculating √6 will give an approximate value of 2.449.
Therefore, the square root of 6 is simplified to √6, which is an irrational number and cannot be reduced further.
Prime Factorization Method
The prime factorization method is a useful technique for simplifying square roots. It involves breaking down a number into its prime factors, which can then be used to simplify the square root. Here is a step-by-step guide to simplifying the square root of 6 using the prime factorization method.
-
Find the prime factors of the number 6.
- The prime factors of 6 are 2 and 3, because \(6 = 2 \times 3\).
-
Write the square root of 6 in terms of its prime factors.
\[\sqrt{6} = \sqrt{2 \times 3}\]
-
Simplify the expression.
Since there are no repeated prime factors, the square root cannot be simplified further. Thus, the simplified form of \(\sqrt{6}\) is just \(\sqrt{6}\).
-
Express the result.
The final simplified form of the square root of 6 is \(\sqrt{6}\).
The prime factorization method is straightforward and effective for simplifying square roots, especially when dealing with numbers that have repeated prime factors.
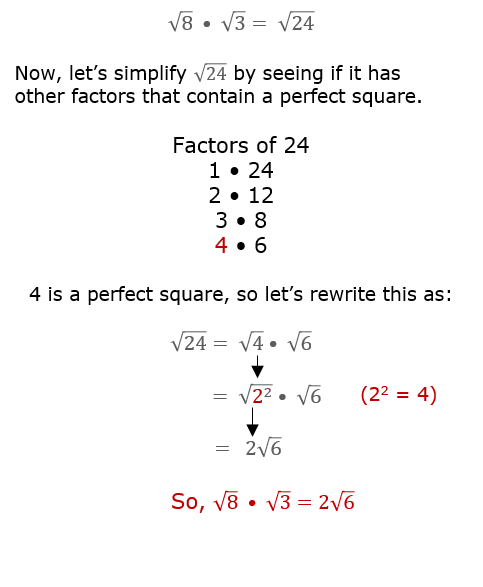
Common Mistakes to Avoid
When simplifying the square root of 6, it's important to be aware of common pitfalls that can lead to errors. Here are some key mistakes to avoid and tips to ensure accuracy:
- Overlooking Perfect Squares: Ensure that you identify and extract any perfect squares within the radicand. This step is crucial for simplifying square roots effectively.
- Rushing Through Calculations: Take your time with each step of the simplification process. Rushing can lead to mistakes, especially in more complex problems.
- Incorrect Prime Factorization: Be thorough when performing prime factorization. Missing a factor can lead to incorrect simplification.
- Assuming Linearity: Remember that the square root function is not linear. For example, \(\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\).
- Forgetting Negative Solutions: While simplifying, remember that both positive and negative roots should be considered if they are within the scope of the problem.
By being mindful of these common mistakes and taking a careful, step-by-step approach, you can simplify square roots accurately and efficiently.
Mathematical Representation and Examples
The square root of 6 can be represented mathematically using the radical symbol as follows:
√6
To break this down further, we use the prime factorization method:
- Prime factorization of 6: 6 = 2 × 3
- Therefore, √6 can be expressed as √(2 × 3).
- This can be split into the product of square roots: √6 = √2 × √3.
However, since neither 2 nor 3 are perfect squares, √6 cannot be simplified further. This makes √6 an irrational number.
### Examples ###
Here are some practical examples of using the square root of 6:
- Example 1:
Find the length of a side of a square with an area of 6 square units.
Solution:
The area A of a square is given by A = side². So, side = √A = √6 ≈ 2.449 units. - Example 2:
Simplify the expression √6 × √6.
Solution:
√6 × √6 = (√6)² = 6. - Example 3:
Approximate the value of √6 to three decimal places.
Solution:
Using a calculator, √6 ≈ 2.449. - Example 4:
If the surface area of a sphere is 24π square units, find the radius.
Solution:
Surface area of a sphere = 4πr² = 24π
Solving for r, we get r² = 6, so r = √6 ≈ 2.449 units.
### Conclusion ###
The square root of 6, represented as √6, is an irrational number that cannot be simplified further. It has practical applications in various mathematical problems and real-world scenarios.
Approximation of Square Root of 6
Approximating the square root of a non-perfect square like 6 can be done using various methods, including estimation, calculator usage, and iterative algorithms. Here, we'll explore a detailed step-by-step approach to approximate the square root of 6.
Estimation Method
-
Identify the perfect squares closest to 6. These are 4 (2²) and 9 (3²).
-
Recognize that √6 is between √4 and √9, thus:
\[ 2 < \sqrt{6} < 3 \]
-
Choose a number between 2 and 3. Let's start with 2.5 and square it:
\[ 2.5 \times 2.5 = 6.25 \]
Since 6.25 is greater than 6, choose a smaller number. Let's try 2.4:
\[ 2.4 \times 2.4 = 5.76 \]
Since 5.76 is less than 6, we can refine further. Try 2.45:
\[ 2.45 \times 2.45 = 6.0025 \]
This value is very close to 6, so we have a good approximation.
Calculator Method
Using a calculator provides a more precise approximation:
To find \(\sqrt{6}\) using a calculator:
\[ \sqrt{6} \approx 2.44948974278 \]
For practical purposes, this value can be rounded to two decimal places:
\[ \sqrt{6} \approx 2.45 \]
Iterative Method (Newton's Method)
Newton's Method provides a systematic approach to approximate square roots:
-
Start with an initial guess. Let \( x_0 = 2 \).
-
Apply the formula:
\[ x_{n+1} = \frac{1}{2} \left( x_n + \frac{6}{x_n} \right) \]
-
Iterate until the values stabilize:
First iteration: \[ x_1 = \frac{1}{2} \left( 2 + \frac{6}{2} \right) = 2.5 \]
Second iteration: \[ x_2 = \frac{1}{2} \left( 2.5 + \frac{6}{2.5} \right) \approx 2.45 \]
Continue iterating for more precision.
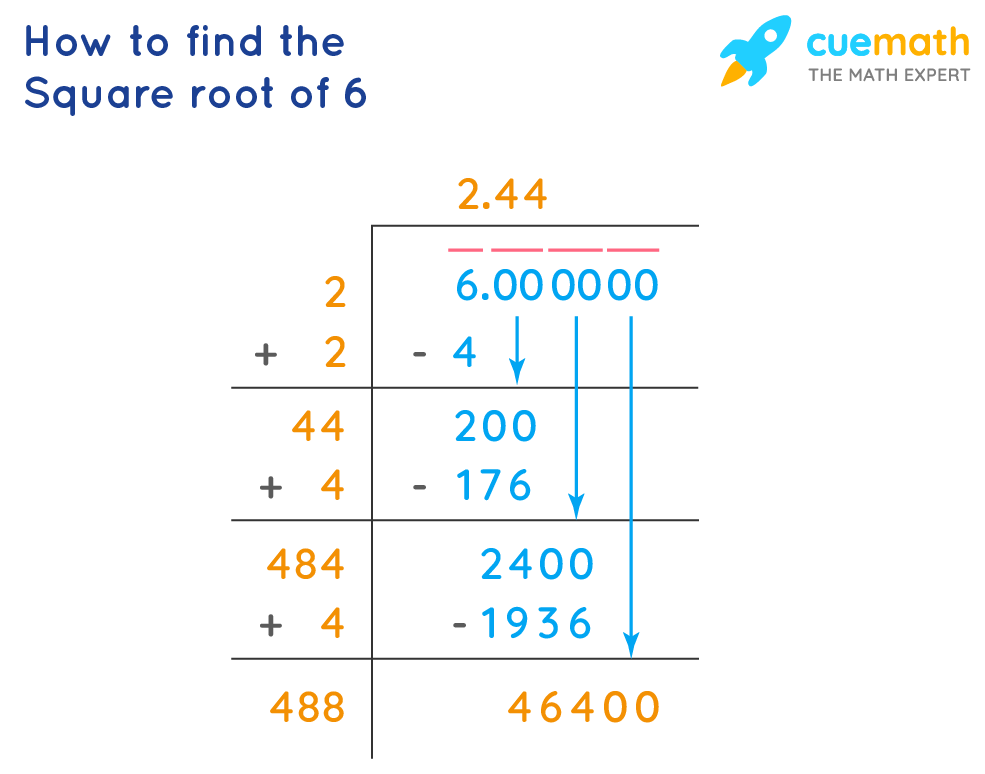
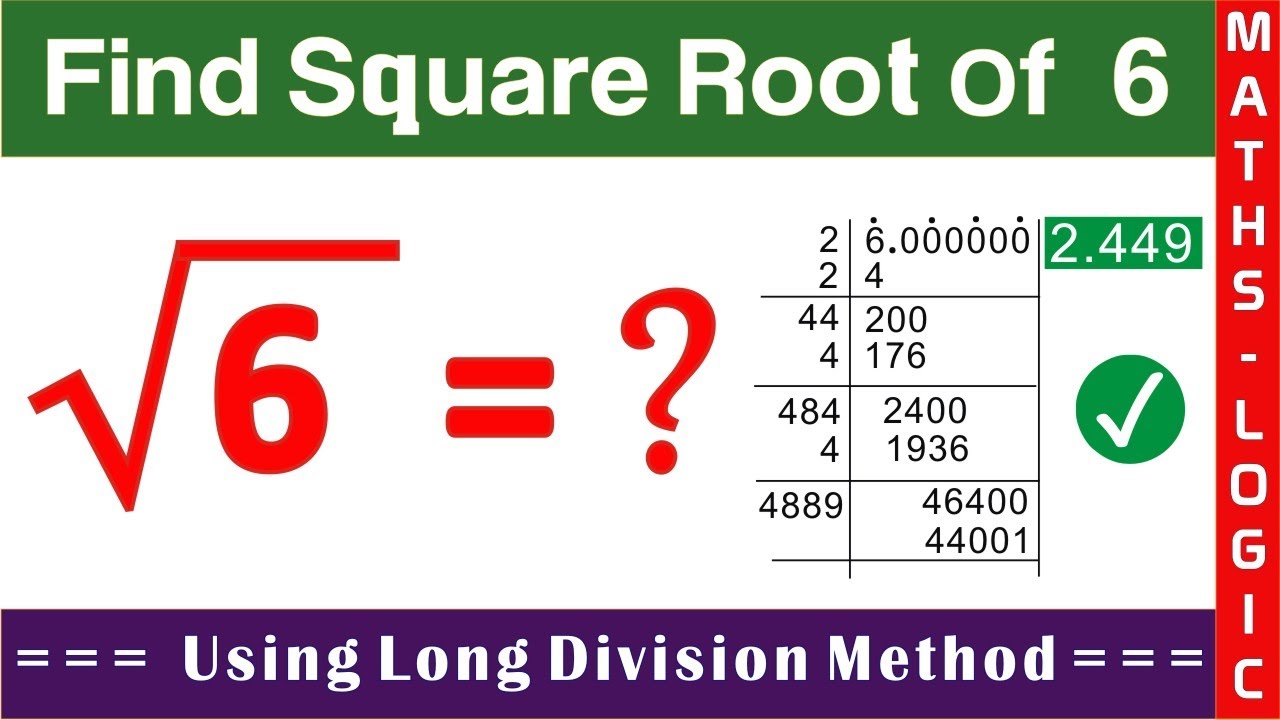
Long Division Method
The long division method provides another precise way to approximate square roots:
-
Start with an initial guess (e.g., 2).
-
Square the guess and subtract from 6, then continue with pairs of zeros.
-
Adjust the guess and refine the quotient until the desired precision is achieved.
This method yields a result close to:
\[ \sqrt{6} \approx 2.449 \]
Conclusion
Various methods can approximate the square root of 6, each with different levels of precision and complexity. Whether using simple estimation, calculators, iterative methods, or long division, the approximate value remains around 2.45, providing sufficient accuracy for most practical applications.
Applications of Simplified Square Roots
Simplified square roots have various applications in different fields. Here are some key areas where they are particularly useful:
- Physics: In physics, square roots are often used to calculate quantities such as velocities, energies, and distances. For example, the time \( t \) it takes for an object to fall from a height \( h \) is given by the equation: \[ t = \sqrt{\frac{2h}{g}} \] where \( g \) is the acceleration due to gravity. Simplifying square roots in such equations can make the calculations more manageable.
- Engineering: Engineers use square roots in various calculations, including those related to material strength, electrical circuits, and structural integrity. For instance, the resonant frequency \( f \) of an LC circuit is given by: \[ f = \frac{1}{2\pi\sqrt{LC}} \] where \( L \) is the inductance and \( C \) is the capacitance. Simplified square roots help in determining accurate results for designing efficient systems.
- Architecture: In architecture, simplified square roots are used to determine proportions and dimensions of structures to ensure stability and aesthetic appeal. For example, calculating the length of the diagonal of a square floor plan: \[ d = \sqrt{2a} \] where \( a \) is the side length of the square. Simplifying the square root of 2 can aid in quick approximations during the design process.
- Finance: Financial models often incorporate square roots to calculate risks, returns, and various statistical measures. For instance, the standard deviation \( \sigma \) of a set of returns, which is a measure of volatility, is calculated as: \[ \sigma = \sqrt{\frac{1}{N} \sum_{i=1}^N (R_i - \overline{R})^2} \] where \( N \) is the number of observations, \( R_i \) are the individual returns, and \( \overline{R} \) is the mean return. Simplified square roots make it easier to interpret these statistical measures.
- Medicine: In medical research, square roots are used in various formulas to assess data, such as the standard error of the mean (SEM): \[ \text{SEM} = \frac{\sigma}{\sqrt{n}} \] where \( \sigma \) is the standard deviation and \( n \) is the sample size. Simplifying square roots in such formulas helps in obtaining accurate medical statistics.
Overall, the ability to simplify square roots is crucial in many scientific, engineering, and practical applications, enabling more straightforward and efficient calculations.
Comparison with Other Square Roots
The square root of 6 is often compared to other square roots to understand its relative value and properties. Here, we will compare \(\sqrt{6}\) with several other common square roots.
- \(\sqrt{4} = 2\)
- \(\sqrt{5} \approx 2.236\)
- \(\sqrt{6} \approx 2.449\)
- \(\sqrt{7} \approx 2.646\)
- \(\sqrt{8} \approx 2.828\)
From this comparison, it's clear that \(\sqrt{6}\) falls between \(\sqrt{5}\) and \(\sqrt{7}\). Here is a more detailed look at each:
| Square Root | Value |
|---|---|
| \(\sqrt{4}\) | 2 |
| \(\sqrt{5}\) | Approximately 2.236 |
| \(\sqrt{6}\) | Approximately 2.449 |
| \(\sqrt{7}\) | Approximately 2.646 |
| \(\sqrt{8}\) | Approximately 2.828 |
These values demonstrate the gradual increase in square roots as the numbers increase. Additionally, understanding these values helps in various mathematical contexts such as comparing lengths, solving equations, and understanding the properties of numbers.
To put this in a real-world context, consider comparing the areas of squares with these side lengths:
- A square with a side length of \(\sqrt{4}\) has an area of \(4\).
- A square with a side length of \(\sqrt{5}\) has an area of \(5\).
- A square with a side length of \(\sqrt{6}\) has an area of \(6\).
- A square with a side length of \(\sqrt{7}\) has an area of \(7\).
- A square with a side length of \(\sqrt{8}\) has an area of \(8\).
This practical application illustrates the incremental changes in the square roots and their corresponding areas.
Practice Problems and Solutions
Practicing the simplification of square roots can help reinforce understanding and accuracy. Below are several practice problems along with their step-by-step solutions.
-
Problem 1: Simplify \(\sqrt{72}\).
- Prime factorize 72: \(72 = 2 \times 2 \times 2 \times 3 \times 3\).
- Group the prime factors into pairs: \((2 \times 2) \times 2 \times (3 \times 3)\).
- Take the square root of each pair: \(2 \times 3 = 6\).
- Therefore, \(\sqrt{72} = 6\sqrt{2}\).
-
Problem 2: Simplify \(\sqrt{98}\).
- Prime factorize 98: \(98 = 2 \times 7 \times 7\).
- Group the prime factors: \(2 \times (7 \times 7)\).
- Take the square root of the pair: \(7\).
- Therefore, \(\sqrt{98} = 7\sqrt{2}\).
-
Problem 3: Simplify \(\sqrt{200}\).
- Prime factorize 200: \(200 = 2 \times 2 \times 2 \times 5 \times 5\).
- Group the prime factors into pairs: \((2 \times 2) \times 2 \times (5 \times 5)\).
- Take the square root of each pair: \(2 \times 5 = 10\).
- Therefore, \(\sqrt{200} = 10\sqrt{2}\).
-
Problem 4: Simplify \(\sqrt{45}\).
- Prime factorize 45: \(45 = 3 \times 3 \times 5\).
- Group the prime factors: \((3 \times 3) \times 5\).
- Take the square root of the pair: \(3\).
- Therefore, \(\sqrt{45} = 3\sqrt{5}\).
These problems demonstrate how to simplify square roots by factorizing the number into its prime factors and then grouping pairs to simplify the expression under the radical.
Additional Resources and Tools
For further exploration into simplifying the square root of 6 and related mathematical concepts, here are some helpful resources and tools:
- : Provides a detailed explanation and examples on simplifying the square root of 6 using different methods.
- : Offers step-by-step guidance on simplifying square roots, including the square root of 6.
- : Allows you to enter the square root expression and shows the simplified form, helpful for verifying your calculations.
- : Provides computational insights and various approaches to simplifying square roots, including the square root of 6.
These resources offer different perspectives and tools to enhance your understanding and proficiency in simplifying the square root of 6, catering to learners at various levels of mathematical expertise.
Conclusion
Mastering the simplification of the square root of 6 opens the door to understanding broader mathematical principles. Through this guide, you've learned effective techniques such as prime factorization and approximation. Remember, practice makes perfect—continue to explore applications and practice problems to solidify your skills.
Xem video này để học cách đơn giản hóa căn bậc hai của số 6. Hướng dẫn chi tiết từ cơ bản đến nâng cao.
Video: Căn bậc hai của 6 - Hướng dẫn chi tiết
READ MORE:
Xem video này để học cách đơn giản hóa các căn bậc hai. Hướng dẫn từ cơ bản đến nâng cao về phép tính căn bậc hai.
Video: Cách đơn giản hóa căn bậc hai