Topic is the square root of 2 rational: The question "Is the square root of 2 rational?" has intrigued mathematicians for centuries. This article delves into the fascinating proof that reveals the true nature of \( \sqrt{2} \), exploring its implications and significance in mathematics. Join us as we uncover why \( \sqrt{2} \) is a cornerstone in the study of irrational numbers.
Table of Content
- Is the Square Root of 2 Rational?
- Introduction to Rational and Irrational Numbers
- Historical Background of the Square Root of 2
- Definition and Properties of Rational Numbers
- Definition and Properties of Irrational Numbers
- Mathematical Proofs of the Irrationality of Square Root of 2
- Geometric Proof of Irrationality
- Impact on Mathematics and Number Theory
- Applications of Irrational Numbers
- Frequently Asked Questions
- YOUTUBE: Xem video để hiểu tại sao căn bậc hai của 2 là số vô tỉ. Bài giảng Đại Số I từ Khan Academy giải thích chi tiết và dễ hiểu.
Is the Square Root of 2 Rational?
The question of whether the square root of 2 (\(\sqrt{2}\)) is a rational number has been a topic of mathematical inquiry for centuries. Let's explore this concept in detail.
Definition of Rational and Irrational Numbers
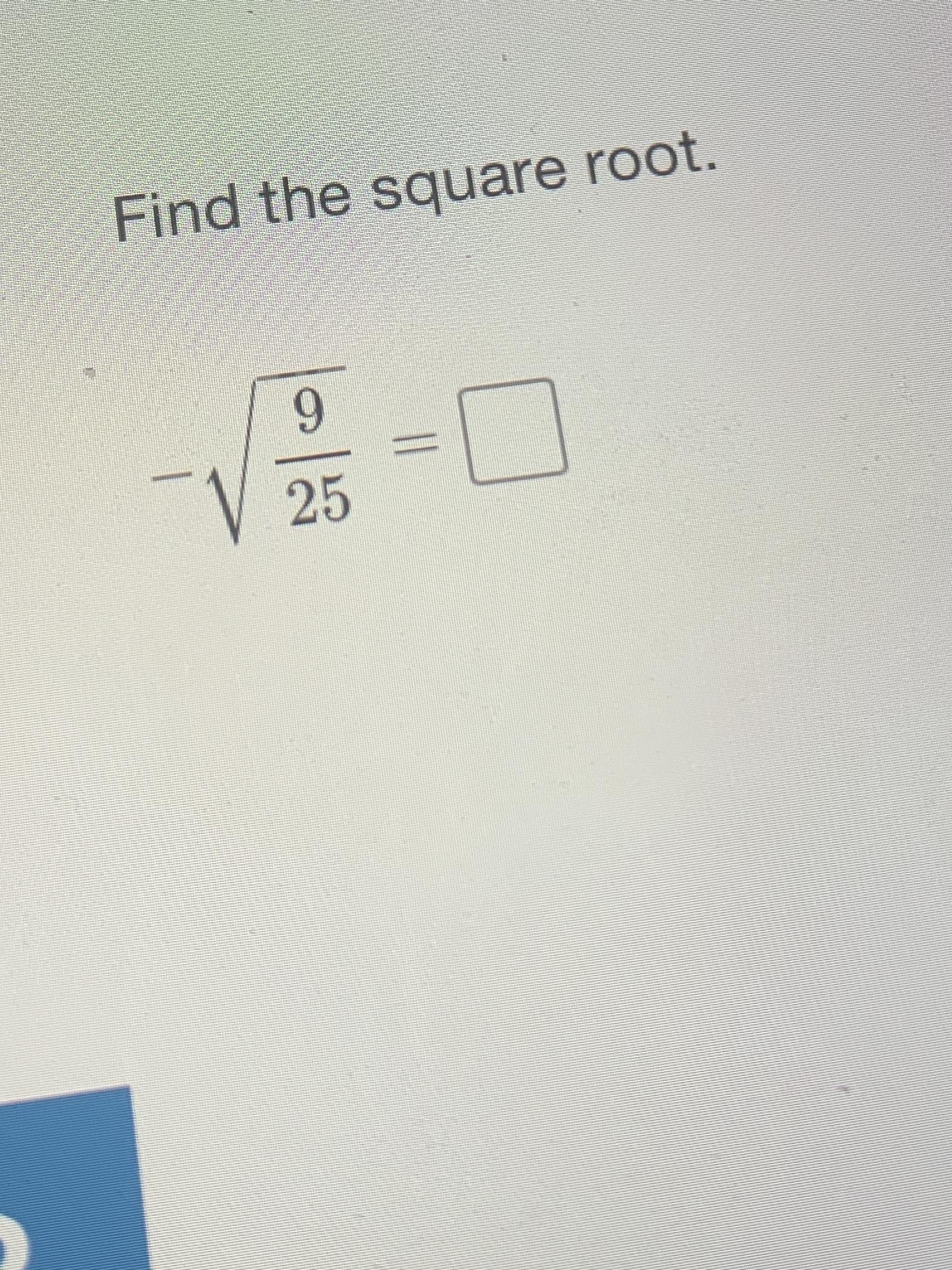
- Rational Numbers: A number is rational if it can be expressed as the quotient of two integers, \( \frac{a}{b} \), where \(a\) and \(b\) are integers and \(b \neq 0\).
- Irrational Numbers: A number is irrational if it cannot be expressed as a simple fraction; its decimal form is non-repeating and non-terminating.
Proof by Contradiction
The most common method to demonstrate that \( \sqrt{2} \) is irrational is by using a proof by contradiction. Here's how it works:
- Assume that \( \sqrt{2} \) is rational. This means there exist two integers \(a\) and \(b\) such that \( \sqrt{2} = \frac{a}{b} \), where \(a\) and \(b\) have no common factors other than 1 (i.e., the fraction is in its simplest form).
- Square both sides of the equation: \( 2 = \frac{a^2}{b^2} \), which implies \( 2b^2 = a^2 \).
- This means \(a^2\) is even (since it is equal to \(2b^2\), which is a multiple of 2). Therefore, \(a\) must also be even (because the square of an odd number is odd).
- Since \(a\) is even, we can write \(a = 2k\) for some integer \(k\). Substituting this into the equation, we get \(2b^2 = (2k)^2\), which simplifies to \(2b^2 = 4k^2\), or \(b^2 = 2k^2\).
- This implies \(b^2\) is also even, and therefore, \(b\) must be even as well.
- If both \(a\) and \(b\) are even, then they have a common factor of 2. This contradicts our initial assumption that \(a\) and \(b\) have no common factors other than 1.
Since our assumption that \( \sqrt{2} \) is rational leads to a contradiction, we conclude that \( \sqrt{2} \) must be irrational.
Conclusion
Through a proof by contradiction, we have shown that \( \sqrt{2} \) cannot be expressed as a ratio of two integers. Therefore, \( \sqrt{2} \) is an irrational number. This conclusion is a fundamental result in mathematics, emphasizing the rich structure and diversity of numbers.

READ MORE:
Introduction to Rational and Irrational Numbers
Understanding the concepts of rational and irrational numbers is fundamental in mathematics. These classifications help in identifying different properties and behaviors of numbers.
Rational Numbers: A rational number is any number that can be expressed as the quotient or fraction \(\frac{a}{b}\) of two integers, where \(a\) (the numerator) and \(b\) (the denominator) are integers, and \(b \neq 0\). Examples include:
- \(\frac{1}{2}\)
- \(\frac{-3}{4}\)
- \(5\) (which can be written as \(\frac{5}{1}\))
Rational numbers can be either positive or negative and include fractions, whole numbers, and terminating or repeating decimals.
Irrational Numbers: An irrational number cannot be expressed as a simple fraction. Its decimal expansion is non-terminating and non-repeating. Examples include:
- \(\sqrt{2}\)
- \(\pi\)
- \(e\) (Euler's number)
Unlike rational numbers, irrational numbers cannot be written as a precise fraction of two integers. Their decimal representations go on forever without repeating.
To summarize:
| Rational Numbers | Irrational Numbers |
|---|---|
| Can be expressed as \(\frac{a}{b}\) | Cannot be expressed as \(\frac{a}{b}\) |
| Decimals are terminating or repeating | Decimals are non-terminating and non-repeating |
| Examples: \(\frac{3}{4}, 0.5, -2\) | Examples: \(\sqrt{2}, \pi, e\) |
Understanding the distinction between rational and irrational numbers is crucial for deeper exploration in mathematics, particularly in number theory and real analysis. This foundational knowledge helps us comprehend the properties and behaviors of different types of numbers.
Historical Background of the Square Root of 2
The concept of the square root of 2, denoted as \( \sqrt{2} \), has a rich history that dates back to ancient civilizations. It is not only a fundamental mathematical constant but also a symbol of the discovery of irrational numbers.
Here is a detailed historical background of the square root of 2:
- Babylonian Mathematics:
The earliest known approximations of \( \sqrt{2} \) come from the Babylonians around 1800 BCE. They used a method similar to the modern iterative processes to estimate \( \sqrt{2} \) accurately up to several decimal places.
- Ancient Greece:
In ancient Greece, the Pythagoreans discovered the concept of irrational numbers. The legend says that Hippasus, a member of the Pythagorean school, demonstrated that \( \sqrt{2} \) cannot be expressed as a ratio of two integers. This discovery was shocking and revolutionary at the time, leading to the realization that not all numbers are rational.
- Geometric Interpretation:
The Greeks also provided a geometric interpretation of \( \sqrt{2} \) through the diagonal of a square. If a square has a side length of 1 unit, then the length of the diagonal is \( \sqrt{2} \) units, according to the Pythagorean theorem.
- Medieval and Renaissance Europe:
During the medieval period, mathematicians continued to study the properties of \( \sqrt{2} \). In the Renaissance, the development of algebra further clarified the nature of irrational numbers, solidifying the understanding that \( \sqrt{2} \) cannot be simplified to a fraction.
- Modern Mathematics:
In contemporary mathematics, \( \sqrt{2} \) is well-established as an irrational number. Its proof through contradiction is a standard topic in mathematical education, emphasizing the importance of irrational numbers in number theory and real analysis.
The historical journey of \( \sqrt{2} \) reflects significant milestones in the evolution of mathematical thought. From ancient approximations to modern proofs, the study of \( \sqrt{2} \) has been pivotal in understanding the broader landscape of numbers.
Definition and Properties of Rational Numbers
Rational numbers play a crucial role in mathematics, representing quantities that can be precisely expressed as the ratio of two integers.
Definition of Rational Numbers:
A rational number is any number that can be expressed in the form of \( \frac{a}{b} \), where \( a \) and \( b \) are integers, and \( b \neq 0 \). This means that rational numbers include both fractions and integers, as integers can be written with a denominator of 1.
Properties of Rational Numbers:
- Closure Property:
The set of rational numbers is closed under addition, subtraction, multiplication, and division (except by zero). This means that performing these operations on rational numbers will always yield another rational number.
- Commutative Property:
Rational numbers are commutative for addition and multiplication. For any rational numbers \( \frac{a}{b} \) and \( \frac{c}{d} \):
- \( \frac{a}{b} + \frac{c}{d} = \frac{c}{d} + \frac{a}{b} \)
- \( \frac{a}{b} \times \frac{c}{d} = \frac{c}{d} \times \frac{a}{b} \)
- Associative Property:
Rational numbers are associative for addition and multiplication. For any rational numbers \( \frac{a}{b} \), \( \frac{c}{d} \), and \( \frac{e}{f} \):
- \( \left( \frac{a}{b} + \frac{c}{d} \right) + \frac{e}{f} = \frac{a}{b} + \left( \frac{c}{d} + \frac{e}{f} \right) \)
- \( \left( \frac{a}{b} \times \frac{c}{d} \right) \times \frac{e}{f} = \frac{a}{b} \times \left( \frac{c}{d} \times \frac{e}{f} \right) \)
- Distributive Property:
Rational numbers follow the distributive property of multiplication over addition. For any rational numbers \( \frac{a}{b} \), \( \frac{c}{d} \), and \( \frac{e}{f} \):
- \( \frac{a}{b} \times \left( \frac{c}{d} + \frac{e}{f} \right) = \left( \frac{a}{b} \times \frac{c}{d} \right) + \left( \frac{a}{b} \times \frac{e}{f} \right) \)
- Decimal Representation:
Rational numbers can be represented as either terminating or repeating decimals. For example:
- \( \frac{1}{4} = 0.25 \) (terminating)
- \( \frac{1}{3} = 0.333\ldots \) (repeating)
- Density Property:
Between any two rational numbers, there exists another rational number. This property illustrates that rational numbers are densely packed on the number line.
Understanding the definition and properties of rational numbers is essential for exploring more complex mathematical concepts, as rational numbers serve as the building blocks for various operations and theories in mathematics.
Definition and Properties of Irrational Numbers
Irrational numbers are a fascinating and fundamental concept in mathematics. Unlike rational numbers, irrational numbers cannot be expressed as a simple fraction. Let's explore the definition and properties of irrational numbers in detail.
Definition of Irrational Numbers:
An irrational number is a real number that cannot be written as the quotient of two integers. In other words, it cannot be expressed in the form \( \frac{a}{b} \), where \( a \) and \( b \) are integers, and \( b \neq 0 \). The decimal representation of an irrational number is non-terminating and non-repeating.
Properties of Irrational Numbers:
- Non-terminating and Non-repeating Decimals:
Irrational numbers have decimal expansions that go on forever without repeating. For example, the decimal expansion of \( \pi \) (pi) is approximately 3.14159..., and it does not repeat.
- Cannot be Expressed as Fractions:
Irrational numbers cannot be written as a ratio of two integers. This differentiates them fundamentally from rational numbers, which can be expressed as fractions.
- Examples of Irrational Numbers:
- \(\sqrt{2}\): The square root of 2 is approximately 1.414213..., and its decimal expansion is non-terminating and non-repeating.
- \(\pi\): Pi is a well-known irrational number used in geometry and trigonometry, representing the ratio of a circle's circumference to its diameter.
- \(e\): Euler's number \(e\) is another important irrational number, approximately equal to 2.71828..., and is the base of the natural logarithm.
- Density on the Number Line:
Just like rational numbers, irrational numbers are densely packed on the number line. Between any two real numbers, there is always at least one irrational number.
- Closure Property:
Irrational numbers are not closed under addition, subtraction, multiplication, or division. For example, the sum or product of two irrational numbers can be rational. However, specific operations on irrational numbers may still result in an irrational number. For instance:
- \(\sqrt{2} + \sqrt{2} = 2\sqrt{2}\), which is irrational.
- \(\pi \times \frac{1}{\pi} = 1\), which is rational.
The discovery and study of irrational numbers have significantly impacted mathematics, providing a deeper understanding of the number system and its complexities. Recognizing the properties of irrational numbers is crucial for exploring advanced mathematical concepts and their applications.
Mathematical Proofs of the Irrationality of Square Root of 2
The irrationality of the square root of 2, denoted as \( \sqrt{2} \), is a well-established fact in mathematics. There are several proofs demonstrating this property. Here, we will explore the most famous proof by contradiction and a geometric proof.
Proof by Contradiction:
- Assume that \( \sqrt{2} \) is rational. This means it can be expressed as a fraction \( \frac{a}{b} \) where \( a \) and \( b \) are coprime integers (i.e., they have no common factors other than 1), and \( b \neq 0 \).
- According to the assumption, \( \sqrt{2} = \frac{a}{b} \). Squaring both sides gives \( 2 = \frac{a^2}{b^2} \), which simplifies to \( a^2 = 2b^2 \).
- This equation implies that \( a^2 \) is even because it is equal to \( 2b^2 \), and thus divisible by 2. Consequently, \( a \) must also be even (since the square of an odd number is odd).
- If \( a \) is even, we can write \( a = 2k \) for some integer \( k \). Substituting \( a = 2k \) into the equation \( a^2 = 2b^2 \) gives \( (2k)^2 = 2b^2 \), which simplifies to \( 4k^2 = 2b^2 \) and further to \( 2k^2 = b^2 \).
- This equation implies that \( b^2 \) is also even, and thus \( b \) must be even as well.
- Since both \( a \) and \( b \) are even, they have a common factor of 2, which contradicts the initial assumption that \( a \) and \( b \) are coprime.
- Therefore, the assumption that \( \sqrt{2} \) is rational must be false. Hence, \( \sqrt{2} \) is irrational.
Geometric Proof:
- Consider a right-angled isosceles triangle with legs of length 1. By the Pythagorean theorem, the length of the hypotenuse is \( \sqrt{2} \).
- Assume that \( \sqrt{2} \) is rational and can be expressed as \( \frac{a}{b} \) in simplest form.
- Construct a smaller right-angled isosceles triangle within the original triangle by drawing a perpendicular from the hypotenuse to the opposite vertex. This smaller triangle will also have legs of integer lengths, and its hypotenuse will again be \( \sqrt{2} \).
- Continue this process indefinitely to create smaller and smaller triangles, each with hypotenuses \( \sqrt{2} \) in the form of \( \frac{a}{b} \) in simplest form.
- This leads to an infinite descent, implying the existence of infinitely many pairs of coprime integers \( (a, b) \) with the property \( a^2 = 2b^2 \), which is impossible.
- Hence, the assumption that \( \sqrt{2} \) is rational must be false, proving that \( \sqrt{2} \) is irrational.
These proofs illustrate the irrationality of \( \sqrt{2} \), highlighting its importance in the study of number theory and the understanding of irrational numbers.
Geometric Proof of Irrationality
The geometric proof of the irrationality of the square root of 2 involves demonstrating that it is impossible to construct a square with a diagonal and side length both represented by rational numbers.
Consider a square with side length \(1\). The length of the diagonal \(d\) of this square can be calculated using the Pythagorean theorem:
\[ d = \sqrt{1^2 + 1^2} = \sqrt{2} \]
Suppose, for the sake of contradiction, that \(\sqrt{2}\) is a rational number. Then, we can express it as a fraction \(\frac{a}{b}\) in its lowest terms, where \(a\) and \(b\) are coprime integers (i.e., they have no common factors other than 1). Hence, we have:
\[ \sqrt{2} = \frac{a}{b} \]
Squaring both sides of the equation, we get:
\[ 2 = \frac{a^2}{b^2} \]
Multiplying both sides by \(b^2\) gives:
\[ 2b^2 = a^2 \]
This implies that \(a^2\) is an even number (since it is 2 times \(b^2\)). Therefore, \(a\) must also be even (as the square of an odd number is odd). Let \(a = 2k\) for some integer \(k\).
Substituting \(a = 2k\) into the equation \(2b^2 = a^2\), we get:
\[ 2b^2 = (2k)^2 \]
\[ 2b^2 = 4k^2 \]
Dividing both sides by 2, we obtain:
\[ b^2 = 2k^2 \]
This implies that \(b^2\) is also even, which means \(b\) must be even. Hence, both \(a\) and \(b\) are even, contradicting our assumption that \(\frac{a}{b}\) is in its lowest terms (since both have a common factor of 2).
Therefore, our initial assumption that \(\sqrt{2}\) is rational must be false. Thus, \(\sqrt{2}\) is irrational.
This geometric proof, derived from the properties of a square and the Pythagorean theorem, elegantly shows the irrationality of the square root of 2.
Impact on Mathematics and Number Theory
The discovery of the irrationality of the square root of 2 had a profound impact on mathematics and number theory. This revelation marked a significant milestone in the understanding of numbers and their properties, challenging the previously held belief that all numbers could be expressed as ratios of integers.
Here are some key impacts:
- Foundation of Irrational Numbers: The realization that \(\sqrt{2}\) is irrational was one of the first known examples of numbers that could not be expressed as fractions. This discovery laid the groundwork for the formal definition and study of irrational numbers.
- Advancement of Greek Mathematics: The ancient Greeks, particularly the Pythagoreans, were initially shocked by the existence of irrational numbers. This challenged their belief in the perfection of numbers and led to deeper investigations into the nature of numbers and magnitudes.
- Development of Proof Techniques: The proof of the irrationality of \(\sqrt{2}\) is a classic example of a proof by contradiction. This method has become a fundamental technique in mathematical proofs, demonstrating the power of logical reasoning in establishing mathematical truths.
- Influence on Geometry: The discovery influenced geometric constructions and the understanding of geometric relationships. The fact that the diagonal of a square is irrational emphasized the need for a more comprehensive understanding of lengths and areas in geometry.
- Expansion of Number Theory: The study of irrational numbers has significantly expanded number theory, leading to the exploration of other classes of numbers such as transcendental numbers and complex numbers. The irrationality of \(\sqrt{2}\) has inspired mathematicians to investigate the properties and relationships of numbers more deeply.
- Mathematical Philosophy: The existence of irrational numbers challenged the philosophical views on the nature of numbers and reality. This has influenced mathematical thought and the philosophy of mathematics, prompting questions about the foundations of mathematical knowledge.
Overall, the discovery that \(\sqrt{2}\) is irrational has had a lasting impact on mathematics, prompting significant advancements in various fields and shaping the way mathematicians understand and work with numbers.
Applications of Irrational Numbers
Irrational numbers, such as \( \sqrt{2} \), \( \pi \), and \( e \), have numerous applications across various fields. Here are some key examples:
- Geometry and Trigonometry:
Irrational numbers frequently appear in geometric contexts. For instance:
- The diagonal of a square with side length 1 is \( \sqrt{2} \), illustrating the Pythagorean theorem.
- \( \pi \) (pi) is essential in calculating the circumference and area of circles, given by \( C = 2\pi r \) and \( A = \pi r^2 \) respectively.
- Calculus:
Irrational numbers are foundational in calculus. Euler's number \( e \approx 2.718 \) is the base of natural logarithms and is critical in the study of exponential growth and decay, such as in the formula for continuous compound interest:
\[
A = P e^{rt}
\]
where \( A \) is the amount of money accumulated after time \( t \), \( P \) is the principal amount, \( r \) is the interest rate, and \( e \) is the base of the natural logarithm. - Physics:
In physics, irrational numbers help describe natural phenomena. For example, the period of a simple pendulum of length \( l \) in a gravitational field \( g \) is:
\[
T = 2\pi \sqrt{\frac{l}{g}}
\]
where \( \pi \) is an irrational number. - Engineering:
Irrational numbers are used in engineering to solve complex problems. For instance, in signal processing, the Fourier transform involves \( \pi \) to transform signals between time and frequency domains.
- Architecture and Design:
In architecture, irrational numbers contribute to aesthetic proportions. The golden ratio \( \phi \approx 1.618 \) is often used to create visually pleasing designs. It appears in the proportions of various structures and artworks.
These examples illustrate the significance of irrational numbers in mathematical theory and their practical applications in real-world scenarios.

Frequently Asked Questions
-
What is a rational number?
A rational number is a number that can be expressed as the quotient or fraction p/q of two integers, where p and q are integers and q is not zero. Examples include 1/2, -3/4, and 5.
-
What is an irrational number?
An irrational number cannot be expressed as a simple fraction. Irrational numbers have non-terminating and non-repeating decimal expansions. Examples include π and the square root of 2.
-
Why is the square root of 2 considered irrational?
The square root of 2 is considered irrational because it cannot be written as a fraction p/q. If we assume that √2 can be expressed as a fraction, we derive a contradiction. This is shown using a proof by contradiction.
-
How does the proof by contradiction for the irrationality of the square root of 2 work?
To prove √2 is irrational, we start by assuming it is rational, so it can be written as p/q in its simplest form. By squaring both sides, we get p2 = 2q2. This implies that p2 is even, so p must be even. If p is even, it can be written as 2k. Substituting back, we find that q must also be even. This contradicts our assumption that p/q is in simplest form, proving that √2 is irrational.
-
What are some practical applications of irrational numbers?
Irrational numbers are used in various fields, such as engineering, physics, and computer science. For example, the square root of 2 is critical in geometry, particularly in calculating the diagonal of a square. Irrational numbers also appear in trigonometry and calculus.
-
How did the discovery of irrational numbers impact mathematics?
The discovery of irrational numbers, such as the square root of 2, challenged the ancient Greek belief that all numbers are rational. This led to a deeper understanding of the number line and the development of real number theory, which is fundamental to modern mathematics.
Xem video để hiểu tại sao căn bậc hai của 2 là số vô tỉ. Bài giảng Đại Số I từ Khan Academy giải thích chi tiết và dễ hiểu.
Chứng Minh Căn Bậc Hai của 2 là Số Vô Tỉ | Đại Số I | Khan Academy
READ MORE:
Video chứng minh căn bậc hai của 2 là số vô tỉ. Bài giảng chi tiết giúp bạn hiểu rõ hơn về số vô tỉ và số hữu tỉ.
Chứng Minh Căn Bậc Hai của Hai là Số Vô Tỉ