Topic how to simplify square roots with exponents: Learn how to simplify square roots with exponents effortlessly with this comprehensive guide. Whether you're dealing with basic or complex expressions, understanding the principles of rational exponents and their relationship to square roots will make the process easier. Dive into practical examples, step-by-step instructions, and common mistakes to avoid to master this essential math skill.
Table of Content
- How to Simplify Square Roots with Exponents
- Introduction to Square Roots and Exponents
- Basic Concepts of Simplifying Square Roots
- Rational Exponents and Their Relationship to Radicals
- Converting Between Radicals and Rational Exponents
- Properties of Exponents and Radicals
- Simplifying Square Roots with Variables
- Using the Quotient Property for Simplification
- Working with Higher-Order Roots
- Approximating Non-Perfect Squares
- Examples of Simplifying Complex Expressions
- Common Mistakes and How to Avoid Them
- Practice Problems and Solutions
- YOUTUBE:
How to Simplify Square Roots with Exponents
Simplifying square roots with exponents can seem challenging at first, but with a systematic approach, it becomes much easier. This guide will walk you through the process using various mathematical properties and rules.
Understanding Rational Exponents
Rational exponents are another way to express roots. For example:
- The square root of a number
\(\sqrt{x}\) can be written as\(x^{1/2}\) . - The cube root of a number
\(\sqrt[3]{x}\) can be written as\(x^{1/3}\) .
Using rational exponents can simplify the manipulation of expressions, especially when dealing with higher-order roots and powers.
Quotient Property of Square Roots
The quotient property of square roots states:
where \(a\) and \(b\) are non-negative real numbers and \(b \ne 0\). This property is useful for simplifying square roots involving fractions.
Step-by-Step Simplification Process
- Simplify the fraction inside the radical if possible. Remove any common factors in the numerator and the denominator.
- Apply the quotient property. Rewrite the square root of the fraction as the quotient of the square roots of the numerator and the denominator.
- Simplify the radicals in the numerator and the denominator. If either the numerator or the denominator is a perfect square, simplify it.
Examples
Example 1
Simplify
- Simplify the fraction:
\(\frac{72z^{12}}{2z^{10}} = 36z^2\) - Apply the square root:
\(\sqrt{36z^2} = 6z\)
Example 2
Simplify
- Simplify the fraction (if possible): In this case, it cannot be simplified further.
- Apply the quotient property:
\(\sqrt{\frac{27m^3}{196}} = \frac{\sqrt{27m^3}}{\sqrt{196}}\) - Simplify the radicals:
\(\frac{\sqrt{27m^3}}{\sqrt{196}} = \frac{3m\sqrt{3m}}{14}\)
Using Calculators for Approximations
For non-perfect squares, approximations are necessary. For example, to approximate
This approximation can be found quickly using the square root function on most calculators.

READ MORE:
Introduction to Square Roots and Exponents
Square roots and exponents are fundamental concepts in mathematics, often appearing in various forms and applications. Understanding these concepts is essential for simplifying complex expressions and solving equations efficiently.
A square root of a number is a value that, when multiplied by itself, gives the original number. The symbol for the square root is √, and the number under the radical symbol is called the radicand. For instance, √16 = 4 because 4 × 4 = 16.
Exponents, on the other hand, denote repeated multiplication of a base number. For example, 32 means 3 multiplied by itself, resulting in 9. When dealing with fractional exponents, they represent both roots and powers, such as 41/2 = √4 = 2.
Combining square roots with exponents can simplify the representation of roots. For example, √a can be written as a1/2, and the cube root of a (³√a) can be expressed as a1/3. This notation is particularly useful in algebraic manipulations and solving equations.
To simplify square roots with exponents, follow these steps:
- Convert the radical expression to an exponent form. For example, √a becomes a1/2.
- Apply the laws of exponents to simplify the expression. For instance, (am)n = am*n.
- If necessary, convert the expression back to radical form. For example, a3/2 becomes √(a3).
By mastering these steps, you can efficiently work with complex expressions involving square roots and exponents, making mathematical problem-solving more manageable.
Basic Concepts of Simplifying Square Roots
Simplifying square roots involves reducing the expression under the square root to its simplest form. This can often make the expression easier to work with in further calculations. Here are the basic steps to simplify square roots:
-
Prime Factorization: Break down the number under the square root into its prime factors.
Example: \(\sqrt{72}\)
- Find the prime factors of 72: \(72 = 2 \times 2 \times 2 \times 3 \times 3\)
- Rewrite the square root: \(\sqrt{72} = \sqrt{2^3 \times 3^2}\)
-
Pairing the Factors: Group the prime factors into pairs.
- For each pair of factors, move one factor out of the square root.
- \(\sqrt{2^3 \times 3^2} = \sqrt{(2^2 \times 2) \times (3^2)} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\)
-
Result: The simplified form of the square root is \(6\sqrt{2}\).
Examples
| Expression | Simplified Form |
|---|---|
| \(\sqrt{50}\) | \(5\sqrt{2}\) |
| \(\sqrt{98}\) | \(7\sqrt{2}\) |
| \(\sqrt{200}\) | \(10\sqrt{2}\) |
Rational Exponents
Square roots can also be expressed using rational exponents. The square root of a number is equivalent to raising that number to the power of \(\frac{1}{2}\).
- \(\sqrt{x} = x^{\frac{1}{2}}\)
- Example: \(\sqrt{16} = 16^{\frac{1}{2}} = 4\)
Understanding how to convert between radical notation and rational exponents can help in simplifying complex expressions and solving equations involving exponents.
Practice Problems
- Simplify \(\sqrt{45}\).
- Express \(\sqrt{81}\) using rational exponents.
- Simplify \(\sqrt{128}\) and express the result in simplest form.
Rational Exponents and Their Relationship to Radicals
Rational exponents offer a different way to represent roots. A rational exponent is an exponent that is a fraction, where the numerator is the power and the denominator is the root. For example, the square root of a number can be written as a rational exponent:
\(\sqrt{a} = a^{\frac{1}{2}}\) \(\sqrt[3]{a} = a^{\frac{1}{3}}\) \(\sqrt[n]{a} = a^{\frac{1}{n}}\)
Using rational exponents can simplify the process of working with roots, especially when dealing with more complex expressions. Below is a table that shows the relationship between radical forms and their equivalent rational exponent forms:
| Radical Form | Exponent Form | Principal Root |
|---|---|---|
| 4 | ||
| 5 | ||
| 2 |
Examples
Let’s look at some examples to understand how to convert between radical and exponent forms:
- Convert
\(\sqrt[3]{x}\) to exponent form: - Convert
\(16^{\frac{1}{4}}\) to radical form:
Understanding the relationship between radicals and rational exponents is essential for simplifying expressions and solving equations involving roots. Rational exponents allow for easier manipulation of terms, especially when applying exponent rules.
Converting Between Radicals and Rational Exponents
Understanding the relationship between radicals and rational exponents is crucial for simplifying expressions efficiently. This section will explain how to convert between these two forms using Mathjax to illustrate the concepts.
Definition and Basic Conversion
A radical can be expressed as a rational exponent. The general form is:
\[ \sqrt[n]{a} = a^{\frac{1}{n}} \]
For example, the square root of \(a\) can be written as:
\[ \sqrt{a} = a^{\frac{1}{2}} \]
Similarly, the cube root of \(a\) is:
\[ \sqrt[3]{a} = a^{\frac{1}{3}} \]
Examples
- \[ \sqrt{16} = 16^{\frac{1}{2}} = 4 \]
- \[ \sqrt[3]{8} = 8^{\frac{1}{3}} = 2 \]
- \[ \sqrt[4]{81} = 81^{\frac{1}{4}} = 3 \]
Properties of Rational Exponents
Rational exponents follow the same properties as integer exponents. Here are some key properties:
- Product of Powers: \[ a^{m} \cdot a^{n} = a^{m+n} \]
- Quotient of Powers: \[ \frac{a^{m}}{a^{n}} = a^{m-n} \]
- Power of a Power: \[ (a^{m})^{n} = a^{m \cdot n} \]
- Power of a Product: \[ (ab)^{m} = a^{m}b^{m} \]
- Power of a Quotient: \[ \left(\frac{a}{b}\right)^{m} = \frac{a^{m}}{b^{m}} \]
Converting from Rational Exponents to Radicals
To convert a rational exponent back to a radical, the process is reversed:
- \[ a^{\frac{m}{n}} = \sqrt[n]{a^m} \]
For example:
- \[ 27^{\frac{2}{3}} = \sqrt[3]{27^2} = \sqrt[3]{729} = 9 \]
- \[ 16^{\frac{3}{4}} = \sqrt[4]{16^3} = \sqrt[4]{4096} = 8 \]
Practice Problems
Convert the following expressions between radical form and rational exponents:
- \[ \sqrt[5]{32} \rightarrow \] \(32^{\frac{1}{5}}\)
- \[ 81^{\frac{3}{4}} \rightarrow \] \(\sqrt[4]{81^3}\)
- \[ \sqrt[6]{64} \rightarrow \] \(64^{\frac{1}{6}}\)
- \[ 125^{\frac{2}{3}} \rightarrow \] \(\sqrt[3]{125^2}\)
By mastering these conversions, you can simplify complex expressions and solve equations more effectively.

Properties of Exponents and Radicals
Understanding the properties of exponents and radicals is essential for simplifying expressions and solving equations that involve these mathematical elements. Here are the key properties:
Properties of Exponents
- Product of Powers Property: \(a^m \cdot a^n = a^{m+n}\)
- Quotient of Powers Property: \(\frac{a^m}{a^n} = a^{m-n}\) (for \(a \neq 0\))
- Power of a Power Property: \((a^m)^n = a^{m \cdot n}\)
- Power of a Product Property: \((ab)^n = a^n \cdot b^n\)
- Power of a Quotient Property: \(\left(\frac{a}{b}\right)^n = \frac{a^n}{b^n}\) (for \(b \neq 0\))
- Zero Exponent Property: \(a^0 = 1\) (for \(a \neq 0\))
- Negative Exponent Property: \(a^{-n} = \frac{1}{a^n}\) (for \(a \neq 0\))
Properties of Radicals
- Product Property: \(\sqrt{a} \cdot \sqrt{b} = \sqrt{ab}\)
- Quotient Property: \(\frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}}\) (for \(b \neq 0\))
- Power of a Radical Property: \((\sqrt[n]{a})^n = a\)
- Radical of a Power Property: \(\sqrt[n]{a^m} = a^{m/n}\)
Combining Exponents and Radicals
When working with expressions that involve both exponents and radicals, it's often useful to convert between radical and exponential form:
- Converting Radicals to Exponents: \(\sqrt[n]{a} = a^{1/n}\)
- Converting Exponents to Radicals: \(a^{m/n} = \sqrt[n]{a^m}\)
These properties are crucial for simplifying complex expressions and solving algebraic equations. Mastering them will enhance your mathematical problem-solving skills.
Simplifying Square Roots with Variables
When simplifying square roots that contain variables, the goal is to find factors under the radical that are perfect squares, allowing us to take their square root. Here are the steps to simplify such expressions:
- Factor the Expression: Identify and separate the expression into its prime factors or components.
- Pair the Factors: Group the variables into pairs of identical factors to form perfect squares.
- Rewrite as Squares: Express the paired factors as squares.
- Apply the Square Root: Take the square root of each pair, remembering to use absolute values if the original variables could be negative.
- Simplify: Multiply the simplified components to get the final result.
Let's look at some examples:
Example 1
Simplify \( \sqrt{9x^6} \)
- Factor: \( \sqrt{9 \cdot x^6} = \sqrt{3^2 \cdot (x^3)^2} \)
- Rewrite: \( \sqrt{3^2} \cdot \sqrt{(x^3)^2} \)
- Apply the square root: \( 3 \cdot |x^3| \)
- Result: \( 3|x^3| \)
Example 2
Simplify \( \sqrt{100x^2y^4} \)
- Factor: \( \sqrt{100 \cdot x^2 \cdot y^4} = \sqrt{10^2 \cdot (x)^2 \cdot (y^2)^2} \)
- Rewrite: \( \sqrt{10^2} \cdot \sqrt{x^2} \cdot \sqrt{(y^2)^2} \)
- Apply the square root: \( 10 \cdot |x| \cdot y^2 \)
- Result: \( 10|x|y^2 \)
Example 3
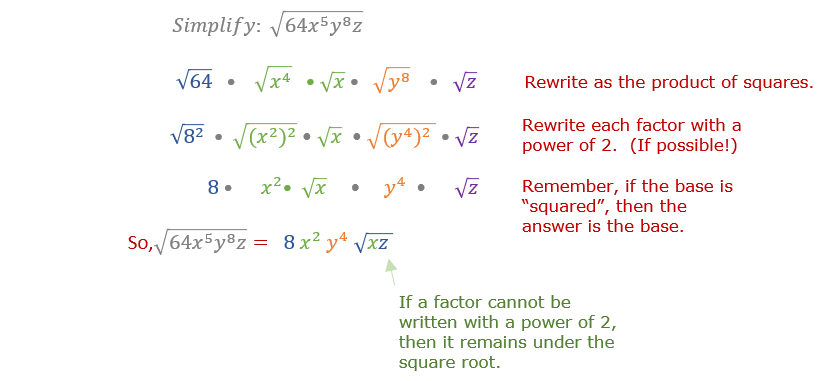
Simplify \( \sqrt{49x^{10}y^8} \)
- Factor: \( \sqrt{49 \cdot x^{10} \cdot y^8} = \sqrt{7^2 \cdot (x^5)^2 \cdot (y^4)^2} \)
- Rewrite: \( \sqrt{7^2} \cdot \sqrt{(x^5)^2} \cdot \sqrt{(y^4)^2} \)
- Apply the square root: \( 7 \cdot |x^5| \cdot y^4 \)
- Result: \( 7|x^5|y^4 \)
These steps can be applied to any square root containing variables to simplify the expression effectively. Always remember to account for the absolute values when variables might be negative to ensure the simplified expression remains valid.
Using the Quotient Property for Simplification
The Quotient Property of Square Roots states that the square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator. Mathematically, this property is expressed as:
, where b ≠ 0.
Here are the steps to simplify square roots using the Quotient Property:
- Simplify the fraction inside the square root, if possible.
For example, simplify :
- Use the Quotient Property to split the square root into two separate radicals.
For example, simplify :
- Simplify the square roots in the numerator and the denominator separately.
For example, simplify :
Let's look at some examples:
- Example 1: Simplify
- Example 2: Simplify
The Quotient Property is a powerful tool for simplifying square roots, especially when dealing with variables and more complex expressions.
Working with Higher-Order Roots
Higher-order roots extend beyond the familiar square roots and include cube roots, fourth roots, fifth roots, and so on. These roots are represented with a radical symbol and an index indicating the degree of the root, or as a rational exponent.
Understanding Higher-Order Roots
The n-th root of a number a is written as or with an index of n. For example, the cube root of 8 is , which equals 2, because = 8.
Properties of Higher-Order Roots
- Product Property: =
- Quotient Property: =
Simplifying Higher-Order Roots
To simplify higher-order roots, you can often use the properties of exponents:
- Rewrite the expression using rational exponents. For example, can be written as .
- Apply the rules for exponents to simplify. For instance, simplifies to .
- Convert back to radical form if needed.
Example Problems
Let's consider a few examples to illustrate these principles:
| Expression | Rational Exponent Form | Simplified Form |
|---|---|---|
| 2 | ||
| 2 |
Using a Calculator for Approximations
For higher-order roots that are not perfect powers, calculators can be used for approximation. For example, to find , you would input 30^(1/3) into a scientific calculator to get approximately 3.107.
Practice Problems
- Simplify .
- Approximate using a calculator.
Approximating Non-Perfect Squares
Approximating square roots of non-perfect squares involves several methods, which help us find values close to the actual square root. Here, we explore a few effective techniques:
Using Nearby Perfect Squares
One common method to approximate the square root of a non-perfect square is to use the nearest perfect squares. Follow these steps:
- Identify the perfect squares closest to the given number. For example, for \(\sqrt{90}\), the closest perfect squares are 81 and 100.
- Determine the square roots of these perfect squares: \(\sqrt{81} = 9\) and \(\sqrt{100} = 10\).
- Estimate that \(\sqrt{90}\) lies between 9 and 10. Since 90 is closer to 81 than 100, a good initial estimate might be around 9.5.
To improve this estimate, we can use the following refined method:
- Find the differences: \(100 - 81 = 19\) and \(90 - 81 = 9\).
- Form a fraction with these differences: \(\frac{9}{19}\).
- Add this fraction to the smaller square root: \(9 + \frac{9}{19} \approx 9.47\).
- Square the result to check accuracy: \((9.47)^2 \approx 89.5\), which is close to 90.
Using the Long Division Method
The long division method provides a more precise approach:
- Pair the digits of the number starting from the decimal point.
- Find the largest number whose square is less than or equal to the first pair or digit.
- Subtract the square from the first pair and bring down the next pair of digits.
- Double the current quotient and find the next digit, which is appended to the divisor and quotient. Repeat the process.
For example, using this method, we find \(\sqrt{3} \approx 1.732\) up to three decimal places.
Using a Calculator
For practical purposes, calculators provide quick approximations:
- Use the square root function on your calculator.
- For example, \(\sqrt{5} \approx 2.236\), and rounding to two decimal places gives \(\sqrt{5} \approx 2.24\).
These methods provide a framework for estimating square roots of non-perfect squares accurately.
Examples of Simplifying Complex Expressions
When simplifying complex expressions involving square roots and exponents, it is crucial to follow systematic steps to ensure accuracy. Here are some detailed examples to guide you:
Example 1: Simplifying Expressions with Radicals
Simplify: \( \sqrt{\frac{72z^{12}}{2z^{10}}} \)
First, simplify the fraction inside the radical:
\[ \sqrt{\frac{72z^{12}}{2z^{10}}} = \sqrt{36z^2} \]
Next, separate the square root of the product into the product of square roots:
\[ \sqrt{36z^2} = \sqrt{36} \cdot \sqrt{z^2} \]
Simplify each square root:
\[ \sqrt{36} = 6 \quad \text{and} \quad \sqrt{z^2} = z \]
Combine the results:
\[ 6z \]
Example 2: Simplifying Radical Expressions Using the Quotient Property
Simplify: \( \sqrt{\frac{45x^5}{y^4}} \)
Rewrite using the quotient property:
\[ \sqrt{\frac{45x^5}{y^4}} = \frac{\sqrt{45x^5}}{\sqrt{y^4}} \]
Simplify the square roots separately:
\[ \sqrt{45x^5} = \sqrt{9x^4 \cdot 5x} = 3x^2\sqrt{5x} \]
\[ \sqrt{y^4} = y^2 \]
Combine the results:
\[ \frac{3x^2\sqrt{5x}}{y^2} \]
Example 3: Simplifying Expressions with Rational Exponents
Simplify: \( \left( \frac{27m^3}{196} \right)^{1/2} \)
Apply the exponent to the numerator and the denominator separately:
\[ \left( \frac{27m^3}{196} \right)^{1/2} = \frac{27^{1/2} \cdot m^{3/2}}{196^{1/2}} \]
Simplify each component:
\[ 27^{1/2} = \sqrt{27} = 3\sqrt{3} \]
\[ 196^{1/2} = \sqrt{196} = 14 \]
Combine the results:
\[ \frac{3m^{3/2}\sqrt{3}}{14} \]
Example 4: Using Approximation for Non-Perfect Squares
Approximate \( \sqrt{17} \):
Identify the nearest perfect squares around 17:
\[ 4^2 = 16 \quad \text{and} \quad 5^2 = 25 \]
So, \( \sqrt{17} \) is between 4 and 5.
Use trial and error to get a closer approximation:
\[ 4.1^2 = 16.81 \quad \text{and} \quad 4.2^2 = 17.64 \]
Thus, \( \sqrt{17} \approx 4.1 \).
For more precision, use a calculator:
\[ \sqrt{17} \approx 4.123 \]
Common Mistakes and How to Avoid Them
Simplifying square roots with exponents can be tricky, and it's easy to make mistakes. Here are some common errors and tips on how to avoid them:
1. Misapplying the Product Rule
When simplifying expressions involving the product of square roots, many students mistakenly multiply the exponents directly. Remember:
\(\sqrt{a} \cdot \sqrt{b} = \sqrt{ab}\)
Correct Example:
\(\sqrt{3} \cdot \sqrt{12} = \sqrt{36} = 6\)
2. Incorrect Use of the Quotient Rule
Another common mistake is dividing the exponents instead of using the quotient rule properly. Always remember:
\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
Correct Example:
\(\sqrt{\frac{16}{4}} = \frac{\sqrt{16}}{\sqrt{4}} = \frac{4}{2} = 2\)
3. Misunderstanding Negative Exponents
Negative exponents often confuse students. A negative exponent indicates a reciprocal:
\(a^{-n} = \frac{1}{a^n}\)
Correct Example:
\(\sqrt{x^{-2}} = \frac{1}{\sqrt{x^2}} = \frac{1}{x}\)
4. Forgetting to Simplify Completely
Always simplify the expression as much as possible. For instance:
\(\sqrt{50}\) should be simplified further:
\(\sqrt{50} = \sqrt{25 \cdot 2} = 5\sqrt{2}\)
5. Ignoring Parentheses
When dealing with exponents and radicals, the correct use of parentheses is crucial. For example, \( (a^b)^c \) is different from \( a^{bc} \).
Correct Example:
\((\sqrt{a})^2 = a\) versus \(\sqrt{a^2} = |a|\)
6. Incorrectly Applying Fractional Exponents
Fractional exponents represent roots, and misapplying them is a common mistake. Recall that:
\(a^{\frac{m}{n}} = \sqrt[n]{a^m}\)
Correct Example:
\(27^{\frac{2}{3}} = \sqrt[3]{27^2} = \sqrt[3]{729} = 9\)
7. Misinterpreting the Order of Operations
Always follow the correct order of operations (PEMDAS/BODMAS). For example:
Incorrect: \(\sqrt{3 \cdot 4} \neq \sqrt{3} \cdot \sqrt{4}\)
Correct: \(\sqrt{3 \cdot 4} = \sqrt{12} = 2\sqrt{3}\)
Tips to Avoid These Mistakes
- Always double-check your application of rules.
- Practice problems of increasing difficulty to gain confidence.
- Use parentheses to clarify operations and avoid ambiguity.
- Review the properties of exponents and radicals regularly.
- Break down complex expressions into simpler parts.
By being mindful of these common mistakes and consistently practicing, you can improve your skills in simplifying square roots with exponents.
Practice Problems and Solutions
Here are some practice problems to help you master the skill of simplifying square roots with exponents. Each problem includes a detailed solution to guide you through the process.
-
Problem: Simplify \( \sqrt{50} \)
Solution:
- Factor the radicand into a product of perfect squares: \( \sqrt{50} = \sqrt{25 \times 2} \).
- Use the product rule of square roots: \( \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} \).
- Simplify the square root of the perfect square: \( \sqrt{25} = 5 \).
- Combine the simplified terms: \( 5\sqrt{2} \).
Answer: \( 5\sqrt{2} \)
-
Problem: Simplify \( \sqrt{72} \)
Solution:
- Factor the radicand into a product of perfect squares: \( \sqrt{72} = \sqrt{36 \times 2} \).
- Use the product rule of square roots: \( \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} \).
- Simplify the square root of the perfect square: \( \sqrt{36} = 6 \).
- Combine the simplified terms: \( 6\sqrt{2} \).
Answer: \( 6\sqrt{2} \)
-
Problem: Simplify \( \sqrt{200} \)
Solution:
- Factor the radicand into a product of perfect squares: \( \sqrt{200} = \sqrt{100 \times 2} \).
- Use the product rule of square roots: \( \sqrt{100 \times 2} = \sqrt{100} \times \sqrt{2} \).
- Simplify the square root of the perfect square: \( \sqrt{100} = 10 \).
- Combine the simplified terms: \( 10\sqrt{2} \).
Answer: \( 10\sqrt{2} \)
-
Problem: Simplify \( \sqrt{8x^4} \)
Solution:
- Factor the radicand into a product of perfect squares: \( \sqrt{8x^4} = \sqrt{4 \times 2 \times x^4} \).
- Use the product rule of square roots: \( \sqrt{4 \times 2 \times x^4} = \sqrt{4} \times \sqrt{2} \times \sqrt{x^4} \).
- Simplify the square root of the perfect squares: \( \sqrt{4} = 2 \) and \( \sqrt{x^4} = x^2 \).
- Combine the simplified terms: \( 2x^2\sqrt{2} \).
Answer: \( 2x^2\sqrt{2} \)
-
Problem: Simplify \( \sqrt{\frac{18y^3}{2}} \)
Solution:
- Simplify the fraction inside the square root: \( \sqrt{\frac{18y^3}{2}} = \sqrt{9y^3} \).
- Factor the radicand into a product of perfect squares: \( \sqrt{9y^3} = \sqrt{9 \times y^2 \times y} \).
- Use the product rule of square roots: \( \sqrt{9 \times y^2 \times y} = \sqrt{9} \times \sqrt{y^2} \times \sqrt{y} \).
- Simplify the square root of the perfect squares: \( \sqrt{9} = 3 \) and \( \sqrt{y^2} = y \).
- Combine the simplified terms: \( 3y\sqrt{y} \).
Answer: \( 3y\sqrt{y} \)

Đơn Giản Hóa Căn Bậc Với Biến Số, Số Mũ, Phân Số, Căn Bậc Ba - Đại Số
READ MORE:
Đơn Giản Hóa Căn Bậc Hai của Số Chính Phương và Số Mũ










