Topic how to simplify negative square roots: Understanding how to simplify negative square roots is essential for mastering complex numbers. This guide will walk you through the steps, making the process simple and clear. Discover the role of imaginary units and learn how to handle negative radicands with confidence, ensuring you have the tools to tackle any math challenge.
Table of Content
- How to Simplify Negative Square Roots
- Introduction to Simplifying Negative Square Roots
- Understanding Negative Numbers and Imaginary Units
- Step-by-Step Guide to Simplifying Negative Square Roots
- Examples of Simplifying Negative Square Roots
- Common Mistakes and How to Avoid Them
- Practice Problems and Solutions
- Advanced Topics: Complex Numbers and Their Applications
- YOUTUBE: Hướng dẫn cách tìm căn bậc hai của một số âm để giúp người học toán hiểu rõ hơn về số ảo và cách đơn giản hóa căn bậc hai âm.
How to Simplify Negative Square Roots
Simplifying the square root of a negative number involves understanding the concept of imaginary numbers. The key idea is to factor out the negative part of the radicand as 'i', where .
Steps to Simplify Negative Square Roots
-
Identify the negative radicand:
For example,
-
Express the negative radicand as a product of -1 and a positive number:
-
Separate the square root of -1 and the square root of the positive number:
-
Simplify the square root of the positive number if possible:
For example,
Examples
-
Step-by-Step:
General Formula
The general formula for simplifying the square root of a negative number is:
Practice Problems
-
Simplify
Solution:
-
Simplify
Solution:
Remember, always express the negative square root in terms of 'i', and simplify the remaining positive square root if possible.

READ MORE:
Introduction to Simplifying Negative Square Roots
Simplifying negative square roots can initially seem challenging, but it becomes manageable with a systematic approach. The process involves converting the negative square root into an imaginary number, represented by the symbol 'i'. This section will guide you step-by-step through the fundamentals of simplifying negative square roots.
Here is the general approach to simplifying the square root of a negative number:
- Identify the negative square root to be simplified, such as
√(-a). - Express the negative inside the square root as a product of -1 and a positive number:
√(-a) = √(-1 * a). - Use the property of square roots that allows the separation of the product:
√(-1 * a) = √(-1) * √(a). - Recognize that
√(-1)is defined as the imaginary unit 'i':√(-1) = i. - Combine the results to obtain the simplified form:
√(-a) = i√(a).
Let's look at some examples:
- Example 1: Simplify
√(-9) - Step 1:
√(-9) = √(-1 * 9) - Step 2:
√(-1 * 9) = √(-1) * √(9) - Step 3:
√(-1) = i - Step 4:
√(9) = 3 - Step 5: Combine the results:
√(-9) = i * 3 = 3i - Example 2: Simplify
√(-16) - Step 1:
√(-16) = √(-1 * 16) - Step 2:
√(-1 * 16) = √(-1) * √(16) - Step 3:
√(-1) = i - Step 4:
√(16) = 4 - Step 5: Combine the results:
√(-16) = i * 4 = 4i
By following these steps, you can simplify any negative square root and express it in terms of imaginary numbers. This method helps in dealing with complex numbers and provides a clearer understanding of their properties and operations.
Understanding Negative Numbers and Imaginary Units
Negative numbers and imaginary units are fundamental concepts in understanding complex numbers and simplifying negative square roots. Let's explore these concepts in detail.
Negative Numbers
A negative number is any number less than zero. It is often represented with a minus sign (-). Negative numbers are used in various real-world contexts, such as temperatures below freezing, debts, and declines in stock prices.
Imaginary Units
Imaginary units are introduced to handle the square roots of negative numbers, which do not have real solutions. The imaginary unit is denoted by i and is defined as:
\( i = \sqrt{-1} \)
Using this definition, we can simplify the square root of any negative number. For instance, to simplify \( \sqrt{-4} \):
- Express the negative number as the product of -1 and a positive number: \( \sqrt{-4} = \sqrt{-1 \times 4} \)
- Separate the square root of the product: \( \sqrt{-1 \times 4} = \sqrt{-1} \times \sqrt{4} \)
- Substitute \( \sqrt{-1} \) with \( i \): \( \sqrt{-1} \times \sqrt{4} = i \times 2 \)
- Simplify the expression: \( i \times 2 = 2i \)
Therefore, \( \sqrt{-4} = 2i \).
General Formula
The general formula to simplify the square root of any negative number is:
\( \sqrt{-a} = i\sqrt{a} \)
where \( a \) is a positive real number. This formula helps in simplifying complex expressions and solving equations involving negative square roots.
Examples
Here are some examples to illustrate the concept:
- \( \sqrt{-9} = i\sqrt{9} = 3i \)
- \( \sqrt{-16} = i\sqrt{16} = 4i \)
- \( \sqrt{-25} = i\sqrt{25} = 5i \)
By understanding and using imaginary units, we can simplify expressions involving negative square roots and solve more complex mathematical problems efficiently.
Step-by-Step Guide to Simplifying Negative Square Roots
Simplifying negative square roots involves a few straightforward steps. Here's a detailed, step-by-step guide to help you understand the process:
- Understand the Imaginary Unit \(i\):
The imaginary unit \(i\) is defined as \(i = \sqrt{-1}\). This is the foundation for simplifying square roots of negative numbers.
- Rewrite the Negative Square Root Using \(i\):
To simplify \(\sqrt{-a}\), rewrite it as \(\sqrt{-1 \cdot a}\). Using the property of square roots, this can be further broken down into \(\sqrt{-1} \cdot \sqrt{a}\).
Since \(\sqrt{-1} = i\), the expression simplifies to \(i \cdot \sqrt{a}\).
- Examples:
-
Example 1: Simplify \(\sqrt{-4}\)
Step 1: Rewrite as \(\sqrt{-1 \cdot 4}\)
Step 2: Separate into \(\sqrt{-1} \cdot \sqrt{4}\)
Step 3: Simplify \(\sqrt{-1}\) to \(i\) and \(\sqrt{4}\) to \(2\)
Final Answer: \(2i\)
-
Example 2: Simplify \(\sqrt{-9}\)
Step 1: Rewrite as \(\sqrt{-1 \cdot 9}\)
Step 2: Separate into \(\sqrt{-1} \cdot \sqrt{9}\)
Step 3: Simplify \(\sqrt{-1}\) to \(i\) and \(\sqrt{9}\) to \(3\)
Final Answer: \(3i\)
-
- General Formula:
For any negative number \(-a\), the square root can be simplified using the formula:
\(\sqrt{-a} = i \cdot \sqrt{a}\)
- Practice Problems:
- Simplify \(\sqrt{-16}\)
- Simplify \(\sqrt{-25}\)
- Simplify \(\sqrt{-49}\)
Use the steps outlined above to solve these practice problems.
Examples of Simplifying Negative Square Roots
To simplify negative square roots, we need to understand the concept of imaginary numbers. The imaginary unit is represented as \( i \), where \( i = \sqrt{-1} \). When simplifying negative square roots, we separate the negative part and express it using \( i \). Here are some examples:
-
Example 1: Simplify \( \sqrt{-4} \)
- Separate the negative part: \( \sqrt{-4} = \sqrt{4} \cdot \sqrt{-1} \)
- Simplify \( \sqrt{4} \) to get 2.
- Replace \( \sqrt{-1} \) with \( i \).
- Combine the results: \( 2i \).
Thus, \( \sqrt{-4} = 2i \).
-
Example 2: Simplify \( \sqrt{-9} \)
- Separate the negative part: \( \sqrt{-9} = \sqrt{9} \cdot \sqrt{-1} \)
- Simplify \( \sqrt{9} \) to get 3.
- Replace \( \sqrt{-1} \) with \( i \).
- Combine the results: \( 3i \).
Thus, \( \sqrt{-9} = 3i \).
-
Example 3: Simplify \( \sqrt{-25} \)
- Separate the negative part: \( \sqrt{-25} = \sqrt{25} \cdot \sqrt{-1} \)
- Simplify \( \sqrt{25} \) to get 5.
- Replace \( \sqrt{-1} \) with \( i \).
- Combine the results: \( 5i \).
Thus, \( \sqrt{-25} = 5i \).
These steps can be applied to any negative square root to simplify it using the imaginary unit \( i \).
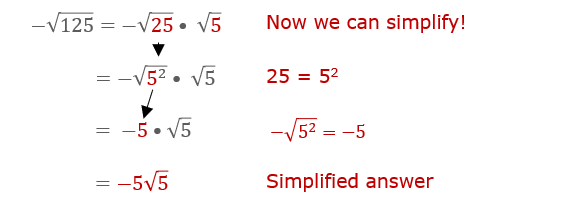
Common Mistakes and How to Avoid Them
Simplifying negative square roots can be tricky. Here are some common mistakes and tips to avoid them:
- Mistake 1: Forgetting the imaginary unit. Remember, the square root of a negative number involves the imaginary unit i where \(i = \sqrt{-1}\). For example, \(\sqrt{-4}\) is not -2, but \(2i\).
- Mistake 2: Incorrectly simplifying the negative sign. Always separate the negative sign and use the imaginary unit. For instance, \(\sqrt{-25} = \sqrt{25} \cdot i = 5i\).
- Mistake 3: Overcomplicating the process. Simplify the positive part first, then add the imaginary unit. For example, \(\sqrt{-36} = \sqrt{36} \cdot i = 6i\).
- Misconception: Thinking imaginary numbers aren't useful. Imaginary numbers are crucial in fields like engineering and physics for solving real-world problems.
By understanding and avoiding these common errors, you can simplify negative square roots more effectively.
Practice Problems and Solutions
Practicing problems is essential for mastering the simplification of negative square roots. Below are several practice problems along with their detailed solutions to help reinforce your understanding:
-
Problem: Simplify \( \sqrt{-64} \)
Solution:
- Identify the negative square root: \( \sqrt{-64} \)
- Express the negative under the square root using the imaginary unit \( i \):
- \( \sqrt{-64} = \sqrt{64} \cdot \sqrt{-1} \)
- Calculate the square root of the positive part:
- \( \sqrt{64} = 8 \)
- Combine the results with the imaginary unit:
- \( \sqrt{-64} = 8i \)
-
Problem: Simplify \( \sqrt{-36} \)
Solution:
- Identify the negative square root: \( \sqrt{-36} \)
- Express the negative under the square root using the imaginary unit \( i \):
- \( \sqrt{-36} = \sqrt{36} \cdot \sqrt{-1} \)
- Calculate the square root of the positive part:
- \( \sqrt{36} = 6 \)
- Combine the results with the imaginary unit:
- \( \sqrt{-36} = 6i \)
-
Problem: Simplify \( \sqrt{-81} \)
Solution:
- Identify the negative square root: \( \sqrt{-81} \)
- Express the negative under the square root using the imaginary unit \( i \):
- \( \sqrt{-81} = \sqrt{81} \cdot \sqrt{-1} \)
- Calculate the square root of the positive part:
- \( \sqrt{81} = 9 \)
- Combine the results with the imaginary unit:
- \( \sqrt{-81} = 9i \)
-
Problem: Simplify \( \sqrt{-121} \)
Solution:
- Identify the negative square root: \( \sqrt{-121} \)
- Express the negative under the square root using the imaginary unit \( i \):
- \( \sqrt{-121} = \sqrt{121} \cdot \sqrt{-1} \)
- Calculate the square root of the positive part:
- \( \sqrt{121} = 11 \)
- Combine the results with the imaginary unit:
- \( \sqrt{-121} = 11i \)
-
Problem: Simplify \( \sqrt{-1} \)
Solution:
- Identify the negative square root: \( \sqrt{-1} \)
- Express the negative under the square root using the imaginary unit \( i \):
- \( \sqrt{-1} = \sqrt{1} \cdot \sqrt{-1} \)
- Calculate the square root of the positive part:
- \( \sqrt{1} = 1 \)
- Combine the results with the imaginary unit:
- \( \sqrt{-1} = i \)
By practicing these problems, you will enhance your skills in simplifying negative square roots and gain a deeper understanding of working with imaginary numbers.
Advanced Topics: Complex Numbers and Their Applications
Complex numbers extend the concept of one-dimensional real numbers to the two-dimensional complex plane by introducing an imaginary unit \( i \), where \( i = \sqrt{-1} \). This allows for the representation and manipulation of numbers that include a real part and an imaginary part.
Definition and Properties
- A complex number is expressed in the form \( a + bi \), where \( a \) is the real part and \( b \) is the imaginary part.
- The imaginary unit \( i \) satisfies the equation \( i^2 = -1 \).
- Examples of complex numbers include \( 3 + 4i \) and \( -2 + 7i \).
Arithmetic Operations
Complex numbers can be added, subtracted, multiplied, and divided using the following rules:
- Addition: \( (a + bi) + (c + di) = (a + c) + (b + d)i \)
- Subtraction: \( (a + bi) - (c + di) = (a - c) + (b - d)i \)
- Multiplication: \( (a + bi)(c + di) = (ac - bd) + (ad + bc)i \)
- Division: \( \frac{a + bi}{c + di} = \frac{(a + bi)(c - di)}{c^2 + d^2} = \frac{(ac + bd) + (bc - ad)i}{c^2 + d^2} \)
Applications of Complex Numbers
Complex numbers are used in various fields such as engineering, physics, and applied mathematics. Here are some applications:
| Field | Application |
|---|---|
| Electrical Engineering | Analyzing AC circuits where the imaginary unit represents the phase difference between voltage and current. |
| Quantum Mechanics | Describing the quantum states of particles and solving the Schrödinger equation. |
| Control Theory | Designing and analyzing control systems using methods like root locus and Bode plots. |
| Signal Processing | Performing Fourier transforms to decompose signals into their frequency components. |
Complex Plane
Complex numbers can be represented on a complex plane where the x-axis represents the real part and the y-axis represents the imaginary part. For example, the complex number \( -2 + 3i \) is plotted as the point (-2, 3).
Plotting complex numbers helps in visualizing and understanding their properties and operations better.
Example
Consider the complex number \( \sqrt{-25} \).
- Express the square root of -25 as \( \sqrt{25} \cdot \sqrt{-1} \).
- Since \( \sqrt{25} = 5 \) and \( \sqrt{-1} = i \), we get \( 5i \).
- Thus, \( \sqrt{-25} = 5i \).
Practice Problems
- Simplify \( \sqrt{-64} \) (Answer: \( 8i \))
- Simplify \( \sqrt{-36} \) (Answer: \( 6i \))
- Simplify \( \sqrt{-81} \) (Answer: \( 9i \))
- Simplify \( \sqrt{-121} \) (Answer: \( 11i \))
Hướng dẫn cách tìm căn bậc hai của một số âm để giúp người học toán hiểu rõ hơn về số ảo và cách đơn giản hóa căn bậc hai âm.
Làm Thế Nào Để Tìm Căn Bậc Hai Của Một Số Âm