Topic 3 square root of 125 simplified: Discover how to simplify the expression 3 square root of 125 with our easy-to-follow guide. This article breaks down the process into simple steps, ensuring you understand each part of the simplification. Perfect for students and math enthusiasts, learn how to master this concept and apply it with confidence.
Table of Content
- Simplification of 3√125
- Introduction
- Understanding Square Roots
- Prime Factorization Method
- Step-by-Step Simplification of 3√125
- Breaking Down 125 into Prime Factors
- Simplifying the Square Root of 125
- Multiplying by the Coefficient
- Examples of Similar Simplifications
- Applications in Algebra
- Common Mistakes to Avoid
- Practice Problems
- Conclusion
- Frequently Asked Questions
- YOUTUBE: Hướng dẫn cách rút gọn căn bậc 2 của 125 thành dạng đơn giản nhất. Xem video để hiểu rõ hơn về quy trình và các bước cụ thể.
Simplification of 3√125
To simplify the expression \(3 \sqrt{125}\), we need to follow these steps:
Step-by-Step Simplification
- Identify the prime factors of the number inside the square root. The number 125 can be factored as follows:
\[
125 = 5 \times 5 \times 5 = 5^3
\] - Apply the square root to the factorized form:
\[
\sqrt{125} = \sqrt{5^3}
\] - Simplify the square root of the prime factors:
\[
\sqrt{5^3} = \sqrt{5^2 \times 5} = 5 \sqrt{5}
\] - Multiply the simplified square root by the coefficient outside the square root:
\[
3 \sqrt{125} = 3 \times 5 \sqrt{5} = 15 \sqrt{5}
\]
Final Simplified Form
The simplified form of \(3 \sqrt{125}\) is:
\[
15 \sqrt{5}
\]

READ MORE:
Introduction
Simplifying the expression \(3 \sqrt{125}\) might seem challenging at first, but it can be made easy by breaking it down into simple steps. This guide will walk you through the process of simplifying \(3 \sqrt{125}\) using fundamental principles of algebra and arithmetic. By understanding each part of the simplification process, you will be able to tackle similar problems with confidence.
Here is an overview of the steps involved:
- Prime Factorization: Breaking down the number 125 into its prime factors.
- Applying the Square Root: Simplifying the square root of the factored form.
- Combining Results: Multiplying the simplified square root by the coefficient.
By the end of this guide, you will have a clear understanding of how to simplify expressions like \(3 \sqrt{125}\) and apply these techniques to other mathematical problems.
Understanding Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, gives the original number. The square root of a number \(x\) is denoted as \(\sqrt{x}\). For example, \(\sqrt{9} = 3\) because \(3 \times 3 = 9\).
Here are some key points to understand about square roots:
- Positive and Negative Roots: Every positive number has two square roots: one positive and one negative. For instance, \(\sqrt{16} = 4\) and \(-\sqrt{16} = -4\).
- Perfect Squares: Numbers like 1, 4, 9, 16, and 25 are perfect squares because their square roots are integers.
- Non-Perfect Squares: For numbers that are not perfect squares, their square roots are irrational numbers. For example, \(\sqrt{2}\) and \(\sqrt{3}\) are irrational.
When simplifying square roots, we often look for perfect square factors. For example, to simplify \(\sqrt{50}\), we can write 50 as \(25 \times 2\), and since 25 is a perfect square, we get:
\[
\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5 \sqrt{2}
\]
Understanding these basics is crucial for simplifying more complex expressions, such as \(3 \sqrt{125}\). By identifying and extracting the perfect square factors, we can simplify square roots effectively.
Prime Factorization Method
The prime factorization method is a useful technique for simplifying square roots, particularly when dealing with larger numbers. By breaking down a number into its prime factors, we can more easily identify and extract perfect squares. Here’s a step-by-step guide to using the prime factorization method to simplify \(3 \sqrt{125}\):
- Find the Prime Factors of 125:
Start by breaking down 125 into its prime factors. Since 125 ends in a 5, we know it is divisible by 5:
\[
125 \div 5 = 25
\]
Now, break down 25 into its prime factors:
\[
25 \div 5 = 5
\]
Therefore, the prime factorization of 125 is:
\[
125 = 5 \times 5 \times 5 = 5^3
\] - Apply the Square Root to the Prime Factors:
Rewrite the square root of 125 using its prime factors:
\[
\sqrt{125} = \sqrt{5^3}
\]
Split the exponent to separate the perfect square:
\[
\sqrt{5^3} = \sqrt{5^2 \times 5} = \sqrt{5^2} \times \sqrt{5}
\]
Simplify the square root of the perfect square:
\[
\sqrt{5^2} = 5
\]
Therefore:
\[
\sqrt{5^3} = 5 \sqrt{5}
\] - Multiply by the Coefficient:
Now, multiply the simplified square root by the coefficient 3:
\[
3 \sqrt{125} = 3 \times 5 \sqrt{5} = 15 \sqrt{5}
\]
Using the prime factorization method, we have successfully simplified \(3 \sqrt{125}\) to \(15 \sqrt{5}\). This method is highly effective for simplifying square roots, especially when dealing with larger numbers or complex expressions.
Step-by-Step Simplification of 3√125
Simplifying the expression involves several steps. Here, we will break down each step in detail.
-
Understanding the Cube Root:
The cube root of a number n, written as , is the number that, when multiplied by itself three times, gives the original number n.
-
Breaking Down 125 into Prime Factors:
To simplify , we first express 125 as a product of prime factors:
- 125 ÷ 5 = 25
- 25 ÷ 5 = 5
- 5 ÷ 5 = 1
So, 125 can be written as \(5 \times 5 \times 5\) or \(5^3\).
-
Simplifying the Cube Root of 125:
Next, we use the property of cube roots: = a.
Applying this property to \(5^3\), we get:
= = 5. -
Multiplying by the Coefficient:
Now we multiply the simplified cube root by the coefficient 3:
3 × 5 = 15.
-
Final Simplified Form:
Therefore, the simplified form of is 15.

Breaking Down 125 into Prime Factors
To simplify the expression involving the cube root of 125, we first need to break down 125 into its prime factors. This step is crucial as it allows us to simplify the cube root more easily. Let's go through the process step-by-step:
-
Identifying Prime Factors:
Prime factors are the prime numbers that multiply together to give the original number. In this case, we need to find the prime factors of 125.
-
Dividing by the Smallest Prime Number:
We start by dividing 125 by the smallest prime number, which is 2. However, 125 is not divisible by 2, so we move to the next smallest prime number, which is 3. Similarly, 125 is not divisible by 3. The next prime number is 5.
Since 125 is divisible by 5, we divide:
125 ÷ 5 = 25
-
Continuing the Division:
We continue dividing the result (25) by 5:
25 ÷ 5 = 5
Finally, we divide the last result (5) by 5:
5 ÷ 5 = 1
-
Listing All Prime Factors:
Now that we have divided 125 completely, we can list all the prime factors:
125 = 5 × 5 × 5
Or, expressed using exponents:
125 = 53
-
Conclusion:
The prime factorization of 125 is 53. This form will help us simplify the cube root of 125 in the subsequent steps.
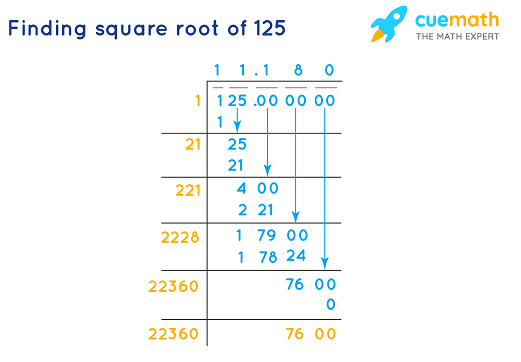
Simplifying the Square Root of 125
To simplify the square root of 125, we need to express it in its simplest radical form. Let's go through the process step-by-step:
-
Prime Factorization:
First, we find the prime factorization of 125. From our previous section, we know:
125 = 53
-
Separate the Perfect Squares:
To simplify √125, we look for any perfect square factors of 125. Here, 25 is a perfect square (since 25 = 52), and it is a factor of 125:
125 = 25 × 5
-
Apply the Square Root to Each Factor:
We use the property of square roots that √(a × b) = √a × √b:
√125 = √(25 × 5) = √25 × √5
Since 25 is a perfect square:
√25 = 5
-
Simplify the Expression:
Now, we can simplify the expression:
√125 = 5 × √5
-
Conclusion:
The simplified form of the square root of 125 is:
√125 = 5√5
Multiplying by the Coefficient
Once we have simplified the square root of 125, the next step is to multiply the result by the coefficient, which in this case is 3. Let's break down the steps:
Recall the simplified form of \( \sqrt{125} \) which we found to be \( 5\sqrt{5} \).
We need to multiply this result by the coefficient 3:
- \[ 3 \times 5\sqrt{5} \]
Perform the multiplication of the coefficient and the simplified radical expression:
- \[ 3 \times 5\sqrt{5} = 15\sqrt{5} \]
So, the final result after multiplying by the coefficient is:
- \[ 3\sqrt{125} = 15\sqrt{5} \]
This process involves multiplying the external coefficient directly with the numerical part of the simplified square root, resulting in the final simplified form.
Examples of Similar Simplifications
Understanding how to simplify square roots with coefficients can be further reinforced by looking at similar examples. Below are step-by-step simplifications for various expressions involving square roots:
Example 1: Simplifying \( 2\sqrt{50} \)
- Factor the number inside the square root:
- \( 50 = 2 \times 25 \)
- Rewrite the square root using its factors:
- \( \sqrt{50} = \sqrt{2 \times 25} \)
- Simplify the square root by taking the square root of 25:
- \( \sqrt{50} = \sqrt{2} \times \sqrt{25} = \sqrt{2} \times 5 = 5\sqrt{2} \)
- Multiply by the coefficient outside the square root:
- \( 2\sqrt{50} = 2 \times 5\sqrt{2} = 10\sqrt{2} \)
Example 2: Simplifying \( 3\sqrt{72} \)
- Factor the number inside the square root:
- \( 72 = 36 \times 2 \)
- Rewrite the square root using its factors:
- \( \sqrt{72} = \sqrt{36 \times 2} \)
- Simplify the square root by taking the square root of 36:
- \( \sqrt{72} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \)
- Multiply by the coefficient outside the square root:
- \( 3\sqrt{72} = 3 \times 6\sqrt{2} = 18\sqrt{2} \)
Example 3: Simplifying \( 4\sqrt{18} \)
- Factor the number inside the square root:
- \( 18 = 9 \times 2 \)
- Rewrite the square root using its factors:
- \( \sqrt{18} = \sqrt{9 \times 2} \)
- Simplify the square root by taking the square root of 9:
- \( \sqrt{18} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2} \)
- Multiply by the coefficient outside the square root:
- \( 4\sqrt{18} = 4 \times 3\sqrt{2} = 12\sqrt{2} \)
Example 4: Simplifying \( 5\sqrt{32} \)
- Factor the number inside the square root:
- \( 32 = 16 \times 2 \)
- Rewrite the square root using its factors:
- \( \sqrt{32} = \sqrt{16 \times 2} \)
- Simplify the square root by taking the square root of 16:
- \( \sqrt{32} = \sqrt{16} \times \sqrt{2} = 4\sqrt{2} \)
- Multiply by the coefficient outside the square root:
- \( 5\sqrt{32} = 5 \times 4\sqrt{2} = 20\sqrt{2} \)
These examples illustrate the process of simplifying square roots with coefficients, reinforcing the principles used in simplifying \( 3\sqrt{125} \).
Applications in Algebra
The simplified form of the expression \( 3\sqrt{125} \), which is \( 15\sqrt{5} \), has various applications in algebra. Understanding these applications can help in solving more complex algebraic problems and equations. Here are a few common scenarios:
1. Solving Radical Equations
Radical equations often require simplifying expressions involving square roots. For instance, consider the equation:
\[
3\sqrt{125x} = 45
\]
First, simplify the square root term:
\[
3 \cdot 5\sqrt{5x} = 45 \implies 15\sqrt{5x} = 45 \implies \sqrt{5x} = 3
\]
Square both sides:
\[
5x = 9 \implies x = \frac{9}{5}
\]
2. Simplifying Expressions
In algebra, you often encounter expressions that need to be simplified for easier manipulation:
\[
3\sqrt{125} + 2\sqrt{80}
\]
Simplify each term:
\[
3 \cdot 5\sqrt{5} + 2 \cdot 4\sqrt{5} = 15\sqrt{5} + 8\sqrt{5} = 23\sqrt{5}
\]
3. Polynomial and Radical Operations
Combining polynomials and radicals is a common task. For example, when adding or subtracting radical terms:
\[
(3\sqrt{125} + x) - (2\sqrt{80} - y)
\]
Simplify each term:
\[
(15\sqrt{5} + x) - (8\sqrt{5} - y) = 15\sqrt{5} + x - 8\sqrt{5} + y = 7\sqrt{5} + x + y
\]
4. Quadratic Equations
Radicals often appear in the solutions of quadratic equations. For example, solving the equation:
\[
x^2 - 15\sqrt{5}x + 56 = 0
\]
using the quadratic formula:
\[
x = \frac{15\sqrt{5} \pm \sqrt{(15\sqrt{5})^2 - 4 \cdot 1 \cdot 56}}{2 \cdot 1}
\]
5. Real-World Applications
Real-world problems, such as those in physics or engineering, often require the manipulation of square roots. For example, calculating the magnitude of a vector or solving problems involving geometric distances can involve expressions like \( 15\sqrt{5} \).
By mastering the simplification of radicals and understanding their applications, students can enhance their problem-solving skills and tackle a wide range of algebraic challenges.
Common Mistakes to Avoid
When simplifying expressions involving radicals, such as \(3\sqrt{125}\), there are several common mistakes to watch out for. Being aware of these pitfalls can help you ensure accuracy and efficiency in your calculations.
- Confusing Square Roots and Cube Roots:
Square roots (√) and cube roots (∛) are distinct operations. Misinterpreting these can lead to incorrect results. For instance, mistaking \(3\sqrt{125}\) for \(3\sqrt[3]{125}\) would yield a different outcome.
- Ignoring the Properties of Radicals:
It’s crucial to apply properties like the product rule for radicals correctly. For example, recognizing that \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\) can simplify the process.
- Overlooking Prime Factorization:
Prime factorization is essential for simplifying radicals. Skipping this step can prevent you from fully simplifying the expression. For \( \sqrt{125} \), prime factorization reveals \(125 = 5^3\), which simplifies the process.
- Mistaking the Radical for a Factor:
Ensure clarity on whether a number inside the radical is part of a larger expression or a standalone factor. Misunderstanding this can lead to errors in simplification.
- Forgetting to Simplify Completely:
After an initial simplification, further reduction might be possible. Always recheck your work to see if additional simplification can be done. For example, \(3\sqrt{125}\) simplifies to \(15\sqrt{5}\) after factoring and reducing.
- Not Recognizing Perfect Squares or Cubes:
Identifying perfect squares or cubes can significantly ease the simplification. For instance, recognizing \(125\) as \(5^3\) helps in breaking down and simplifying the radical expression.
By avoiding these common mistakes, you can streamline the process of simplifying radicals and ensure your mathematical work is both accurate and efficient.
Practice Problems
Here are some practice problems to help you master simplifying square roots and applying coefficients:
-
Simplify \( 3\sqrt{72} \):
- Factor 72 into prime factors: \( 72 = 2^3 \times 3^2 \)
- Simplify the square root: \( \sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{2^2 \times 2 \times 3^2} = 3\sqrt{8} \)
- Combine with the coefficient: \( 3 \times 3\sqrt{8} = 9\sqrt{8} \)
-
Simplify \( 2\sqrt{50} \):
- Factor 50 into prime factors: \( 50 = 2 \times 5^2 \)
- Simplify the square root: \( \sqrt{50} = \sqrt{2 \times 5^2} = 5\sqrt{2} \)
- Combine with the coefficient: \( 2 \times 5\sqrt{2} = 10\sqrt{2} \)
-
Simplify \( 4\sqrt{45} \):
- Factor 45 into prime factors: \( 45 = 3^2 \times 5 \)
- Simplify the square root: \( \sqrt{45} = \sqrt{3^2 \times 5} = 3\sqrt{5} \)
- Combine with the coefficient: \( 4 \times 3\sqrt{5} = 12\sqrt{5} \)
-
Simplify \( 5\sqrt{32} \):
- Factor 32 into prime factors: \( 32 = 2^5 \)
- Simplify the square root: \( \sqrt{32} = \sqrt{2^4 \times 2} = 4\sqrt{2} \)
- Combine with the coefficient: \( 5 \times 4\sqrt{2} = 20\sqrt{2} \)
-
Simplify \( 7\sqrt{18} \):
- Factor 18 into prime factors: \( 18 = 2 \times 3^2 \)
- Simplify the square root: \( \sqrt{18} = \sqrt{2 \times 3^2} = 3\sqrt{2} \)
- Combine with the coefficient: \( 7 \times 3\sqrt{2} = 21\sqrt{2} \)
These problems should provide a good foundation for simplifying expressions involving square roots and coefficients. Remember to always break down the radicand into its prime factors and simplify before multiplying by the coefficient.
Conclusion
In this guide, we have explored the simplification of \(3\sqrt{125}\) step-by-step. By breaking down 125 into its prime factors, simplifying the square root, and then multiplying by the coefficient, we arrived at the final simplified form of \(15\sqrt{5}\).
This process demonstrates the importance of understanding prime factorization and the properties of square roots in algebra. Such skills are crucial for solving more complex algebraic problems and for ensuring accuracy in mathematical computations.
Practicing these steps with different numbers will help reinforce the method and improve your confidence in handling similar problems. Remember to always check your work for common mistakes, such as misidentifying the root or incorrectly multiplying the coefficients.
By mastering these techniques, you can apply them in various algebraic contexts, enhancing your overall mathematical proficiency. Keep practicing, and you'll find that simplifying radicals becomes second nature.
We hope this guide has been helpful in your journey to understand and simplify radical expressions. Happy calculating!

Frequently Asked Questions
- What is the square root of 125 simplified?
- How do you simplify the square root of 125?
- Why is the simplified form of the square root of 125 \(5\sqrt{5}\)?
- Can the square root of 125 be simplified further?
- What are some common mistakes to avoid when simplifying the square root of 125?
- Mistaking the prime factorization of 125.
- Forgetting to simplify the square root after finding the prime factors.
- Trying to simplify further when 125 cannot be expressed as the product of larger perfect squares.
The square root of 125 simplified is \(5\sqrt{5}\).
To simplify the square root of 125, you first break it down into its prime factors. Then, you take out any pairs of the same factors from inside the square root. The remaining factors stay inside, and the square root simplifies to the product of the factor pairs outside the root.
The simplified form of the square root of 125 is \(5\sqrt{5}\) because 125 can be expressed as the product of the perfect square 25 and the prime factor 5. When taking the square root, the perfect square comes out as 5, and the prime factor remains inside the root.
No, the square root of 125 cannot be simplified further because 125 is not divisible by any other perfect square larger than 25.
Hướng dẫn cách rút gọn căn bậc 2 của 125 thành dạng đơn giản nhất. Xem video để hiểu rõ hơn về quy trình và các bước cụ thể.
Cách Rút Gọn Căn Bậc 2 của 125: √125
READ MORE:
Xem video này để học cách rút gọn các biểu thức chứa các căn bậc 3 và cách thực hiện phép tính trong các trường hợp phức tạp hơn.
Trung Cấp 9 4 / Rút gọn biểu thức chứa các căn bậc cao hơn (căn bậc 3 của 125)