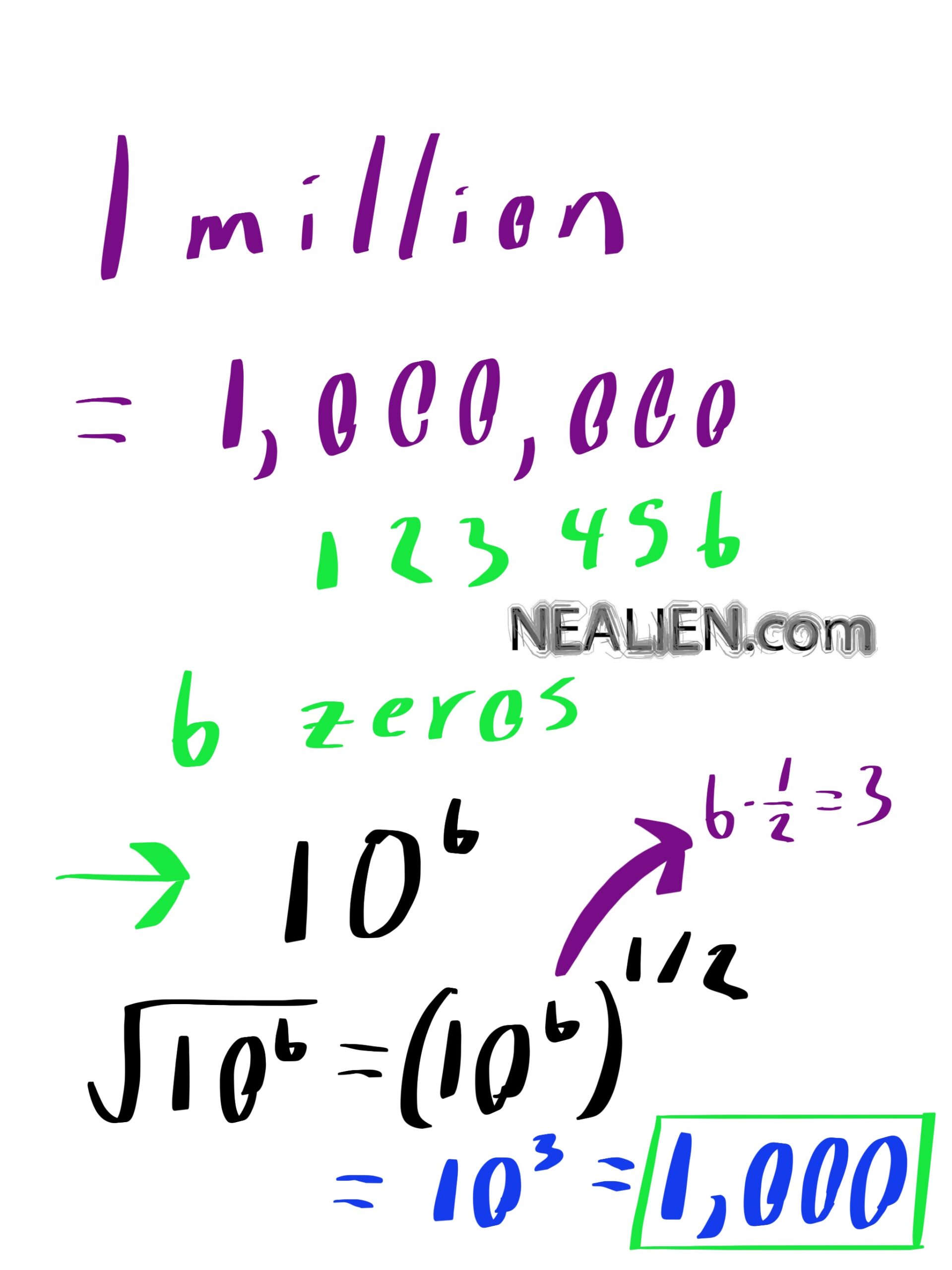Topic square root of 1 to 10: Discover the fascinating world of square roots with our comprehensive guide to the square roots of numbers 1 to 10. Whether you're a student or a math enthusiast, this article will help you understand and calculate these essential mathematical values, along with practical applications and real-life examples. Dive in and enhance your mathematical knowledge!
Table of Content
- Square Root of Numbers from 1 to 10
- Introduction to Square Roots
- Understanding Square Roots
- The Importance of Square Roots in Mathematics
- Calculating Square Roots
- Square Root of 1
- Square Root of 2
- Square Root of 3
- Square Root of 4
- Square Root of 5
- Square Root of 6
- Square Root of 7
- Square Root of 8
- Square Root of 9
- Square Root of 10
- Applications of Square Roots
- Real-Life Examples of Square Roots
- Tools and Techniques for Finding Square Roots
- Conclusion
- YOUTUBE: Khám phá các hình vuông hoàn hảo và căn bậc hai từ 1 đến 10 với Thầy J. Video này sẽ giúp bạn nắm vững các khái niệm toán học cơ bản về hình vuông và căn bậc hai một cách dễ hiểu.
Square Root of Numbers from 1 to 10
The square root of a number \( x \) is a value that, when multiplied by itself, gives \( x \). This is mathematically represented as:
\( y = \sqrt{x} \quad \text{such that} \quad y^2 = x \)
Values of Square Roots from 1 to 10
- \(\sqrt{1} = 1\)
- \(\sqrt{2} \approx 1.414\)
- \(\sqrt{3} \approx 1.732\)
- \(\sqrt{4} = 2\)
- \(\sqrt{5} \approx 2.236\)
- \(\sqrt{6} \approx 2.449\)
- \(\sqrt{7} \approx 2.646\)
- \(\sqrt{8} \approx 2.828\)
- \(\sqrt{9} = 3\)
- \(\sqrt{10} \approx 3.162\)
Methods to Calculate Square Roots
There are several methods to find the square roots of numbers:
-
Prime Factorization Method
This method involves expressing the number as a product of prime factors and then pairing them to find the square root. For example:
\( \sqrt{9} = \sqrt{3 \times 3} = 3 \)
-
Long Division Method
This method is used for non-perfect squares. It involves dividing the number and finding the average of the quotients repeatedly until a satisfactory approximation is achieved.
-
Repeated Subtraction Method
This method involves subtracting successive odd numbers from the original number until the result is zero. The number of steps taken is the square root. For example:
\( 9 - 1 = 8 \)
\( 8 - 3 = 5 \)
\( 5 - 5 = 0 \)
(3 steps, so \( \sqrt{9} = 3 \))
Applications and Examples
Here are some practical examples of how to use square roots:
-
Find the side length of a square metal sheet with an area of 10 square inches:
\( a^2 = 10 \quad \Rightarrow \quad a = \sqrt{10} \approx 3.162 \) inches
-
Find the radius of a circular tabletop with an area of \( 10\pi \) square inches:
\( \pi r^2 = 10\pi \quad \Rightarrow \quad r = \sqrt{10} \approx 3.162 \) inches
-
Calculate \( 17\sqrt{4} + 12\sqrt{9} \):
\( 17 \times 2 + 12 \times 3 = 34 + 36 = 70 \)
Frequently Asked Questions (FAQs)
- What is the square root of a number? It is the value that, when multiplied by itself, gives the original number.
- How many numbers between 1 and 10 have irrational square roots? The numbers 2, 3, 5, 6, 7, 8, and 10.
- Which numbers between 1 and 10 are perfect squares? The numbers 1, 4, and 9.
| Number | Square Root |
|---|---|
| 1 | 1 |
| 2 | 1.414 |
| 3 | 1.732 |
| 4 | 2 |
| 5 | 2.236 |
| 6 | 2.449 |
| 7 | 2.646 |
| 8 | 2.828 |
| 9 | 3 |
| 10 | 3.162 |

READ MORE:
Introduction to Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. It is a fundamental concept in mathematics, frequently used in various fields such as algebra, geometry, and physics. Understanding square roots is essential for solving quadratic equations, calculating areas, and analyzing scientific data.
Mathematically, the square root of a number \( x \) is denoted as \( \sqrt{x} \) or \( x^{1/2} \). For example, \( \sqrt{9} = 3 \) because \( 3 \times 3 = 9 \).
- The square root of 1 is \( \sqrt{1} = 1 \).
- The square root of 2 is approximately \( \sqrt{2} \approx 1.414 \).
- The square root of 3 is approximately \( \sqrt{3} \approx 1.732 \).
- The square root of 4 is \( \sqrt{4} = 2 \).
- The square root of 5 is approximately \( \sqrt{5} \approx 2.236 \).
- The square root of 6 is approximately \( \sqrt{6} \approx 2.449 \).
- The square root of 7 is approximately \( \sqrt{7} \approx 2.646 \).
- The square root of 8 is approximately \( \sqrt{8} \approx 2.828 \).
- The square root of 9 is \( \sqrt{9} = 3 \).
- The square root of 10 is approximately \( \sqrt{10} \approx 3.162 \).
Square roots are not only limited to perfect squares like 1, 4, and 9. They also apply to non-perfect squares, resulting in irrational numbers. Understanding and calculating square roots can be achieved through various methods, including prime factorization, long division, and using a calculator for precision.
The concept of square roots extends beyond simple arithmetic. It is foundational in advanced mathematical concepts, including the Pythagorean theorem, where the square root is used to determine the length of the hypotenuse in a right triangle. Additionally, square roots play a crucial role in statistics, particularly in calculating standard deviation and variance.
By mastering square roots, you can enhance your problem-solving skills and deepen your comprehension of mathematical principles. This guide will provide detailed explanations, examples, and practical applications to help you confidently navigate the world of square roots from 1 to 10.
Understanding Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, yields the original number. The square root symbol is denoted as \( \sqrt{} \), and it can be applied to both perfect squares and non-perfect squares.
Here are the steps to understand and calculate square roots:
- Basic Definition: The square root of a number \( x \) is a value \( y \) such that \( y \times y = x \). In mathematical terms, this is written as \( y = \sqrt{x} \).
- Perfect Squares: These are numbers whose square roots are integers. For example:
- \( \sqrt{1} = 1 \)
- \( \sqrt{4} = 2 \)
- \( \sqrt{9} = 3 \)
- Non-Perfect Squares: These numbers do not have integer square roots and result in irrational numbers. For example:
- \( \sqrt{2} \approx 1.414 \)
- \( \sqrt{3} \approx 1.732 \)
- \( \sqrt{5} \approx 2.236 \)
- Prime Factorization Method: This method involves breaking down a number into its prime factors to find its square root. For example:
- To find \( \sqrt{36} \):
- Factorize 36: \( 36 = 2 \times 2 \times 3 \times 3 \)
- Pair the prime factors: \( (2 \times 3) \times (2 \times 3) \)
- Calculate the product of one set of prime factors: \( 2 \times 3 = 6 \)
- So, \( \sqrt{36} = 6 \)
- To find \( \sqrt{36} \):
- Long Division Method: This method is useful for finding the square root of non-perfect squares:
- Example: To find \( \sqrt{10} \):
- Start with a pair of digits, and find the largest number whose square is less than or equal to the first pair. Here, the first digit is 1, and the largest square less than or equal to 1 is 1.
- Double the result (1) and use it as the divisor. Bring down the next pair of digits (00), and find a number that, when added to the doubled result and multiplied by itself, is less than or equal to the dividend.
- Repeat the process to get a more accurate result. \( \sqrt{10} \approx 3.162 \).
- Example: To find \( \sqrt{10} \):
- Using a Calculator: For quick and precise calculations, especially for non-perfect squares, using a calculator is the most efficient method.
Understanding square roots involves recognizing their applications in various mathematical contexts. From solving quadratic equations to determining distances in geometry, square roots are a versatile and indispensable tool in mathematics.
The Importance of Square Roots in Mathematics
Square roots are fundamental in mathematics due to their numerous applications and intrinsic properties. Understanding square roots is crucial for several reasons:
- Foundational Concept: The square root is a basic arithmetic operation, just as essential as addition, subtraction, multiplication, and division. It helps in simplifying expressions and solving equations.
- Geometry and Trigonometry: Square roots are vital in geometry, particularly in calculating distances, areas, and volumes. They are used in the Pythagorean theorem to determine the length of sides in right triangles. For instance, the distance between two points in a plane or space can be found using the distance formula, which involves square roots.
- Algebra: Square roots play a key role in solving quadratic equations through the quadratic formula. The solutions of these equations often require taking the square root of a number, which provides insight into the nature of the solutions (real or complex).
- Physics and Engineering: In physics, square roots are used to describe relationships in formulas, such as calculating the speed of an object when given its kinetic energy. Engineering applications often involve square roots in determining dimensions and tolerances.
- Probability and Statistics: The concept of standard deviation, a measure of the amount of variation or dispersion in a set of values, involves the square root. This is crucial for understanding data distributions and making predictions based on data analysis.
- Computer Science: Algorithms for computing various functions, such as square roots, are fundamental in computer science. Efficient methods to approximate square roots are necessary for computer graphics, simulations, and other computational tasks.
- Real-Life Applications: Square roots appear in various real-life contexts, such as calculating areas and perimeters, determining the strength of materials, and in financial models to assess risks and returns.
Overall, the importance of square roots in mathematics cannot be overstated. They are a cornerstone of mathematical education and practice, bridging arithmetic, algebra, geometry, and beyond. Mastery of square roots enables deeper comprehension and application of mathematical principles in both academic and real-world scenarios.
Calculating Square Roots
Calculating square roots can be approached in several ways, ranging from simple methods suitable for perfect squares to more complex techniques for non-perfect squares. Here are some of the common methods used:
1. Using a Calculator
The simplest way to find the square root of a number is to use a calculator. Most calculators have a square root button (√) that makes this process straightforward.
2. Prime Factorization Method
This method involves expressing the number as a product of prime factors and then applying the square root to these factors. This is particularly useful for perfect squares.
- Example: Find the square root of 36.
- Prime factorize 36: \(36 = 2^2 \times 3^2\)
- Apply the square root: \(\sqrt{36} = \sqrt{2^2 \times 3^2} = 2 \times 3 = 6\)
3. Long Division Method
The long division method is used for finding the square roots of non-perfect squares and works by repeatedly dividing and averaging to get a more accurate result.
- Start by grouping the digits in pairs, starting from the decimal point.
- Find the largest number whose square is less than or equal to the first pair. Write this number as the divisor and quotient.
- Subtract the square of the quotient from the first pair and bring down the next pair of digits.
- Double the quotient and determine the next digit of the quotient such that the new number formed by the divisor and the new digit, when multiplied by the new digit, is less than or equal to the current dividend.
- Repeat the steps until you achieve the desired precision.
Example: Find the square root of 10.
| Step | Calculation |
| 1 | Guess: 3 (since 32 = 9) |
| 2 | 3.12 = 9.61 |
| 3 | 3.162 = 9.9856 |
| 4 | Continue to desired accuracy |
4. Newton’s Method (Heron's Method)
This iterative method improves an initial guess by averaging it with the quotient of the original number and the guess.
- Start with an initial guess \(x_0\).
- Apply the formula \(x_{n+1} = \frac{x_n + \frac{S}{x_n}}{2}\), where \(S\) is the number you are finding the square root of.
- Repeat the process until the difference between \(x_n\) and \(x_{n+1}\) is sufficiently small.
Example: Find the square root of 10.
- Initial guess: \(x_0 = 3\)
- First iteration: \(x_1 = \frac{3 + \frac{10}{3}}{2} = 3.1667\)
- Second iteration: \(x_2 = \frac{3.1667 + \frac{10}{3.1667}}{2} = 3.1623\)
5. Estimation Method
This method involves estimating the square root by identifying the two nearest perfect squares and interpolating between them.
- Example: Estimate \(\sqrt{5}\).
- Identify nearest perfect squares: \(2^2 = 4\) and \(3^2 = 9\)
- Estimate: \(\sqrt{5} \approx 2.2\)
6. Repeated Subtraction Method
This is a manual method where you repeatedly subtract consecutive odd numbers from the target number until you reach zero. The number of subtractions gives the square root.
- Example: Find \(\sqrt{9}\).
- 9 - 1 = 8
- 8 - 3 = 5
- 5 - 5 = 0
- Since we reached zero in 3 steps, \(\sqrt{9} = 3\).
Using these methods, one can calculate the square roots of numbers with varying levels of precision and complexity, suitable for different applications in mathematics.

Square Root of 1
The square root of 1 is a fundamental concept in mathematics, representing a value which, when multiplied by itself, results in 1. This is expressed mathematically as:
Thus, the square root of 1 is:
This is because:
Therefore, the square root of 1 has two solutions:
- 1
- -1
However, by convention, we often refer to the principal (positive) square root, which is simply 1.
Derivation and Explanation
To derive the square root of 1, consider the polynomial equation:
Solving this equation involves finding the values of x that satisfy the equation. Since the square of both 1 and -1 equals 1, the solutions are:
The value of the square root of 1 is straightforward because it is a perfect square. This simplicity helps in understanding more complex square root calculations.
Examples and Practice
Consider these examples to solidify your understanding:
-
Example 1: Verify that the square root of 1 is indeed 1.
-
Example 2: Verify that the square root of 1 is also -1.
Understanding the square root of 1 lays the foundation for more complex mathematical concepts and operations involving square roots.
Square Root of 2
The square root of 2, represented as √2, is an irrational number that cannot be expressed as a simple fraction. It is approximately equal to 1.414213562373095. This value is used extensively in various mathematical calculations and real-world applications.
Here are some key points about the square root of 2:
- It is an irrational number, meaning its decimal representation is non-terminating and non-repeating.
- The square root of 2 can be approximated using different methods, such as the long division method and the estimation method.
Calculating the Square Root of 2
There are several methods to calculate the square root of 2. Two commonly used methods are:
Long Division Method
This method involves a step-by-step approach to find the value of √2 to a desired level of accuracy:
- Start by finding the largest number whose square is less than or equal to 2. This number is 1.
- Place a decimal point in the quotient and bring down pairs of zeros. The new dividend is now 100.
- Double the current divisor (1) to get 2, and determine the largest digit (x) such that 2x * x is less than or equal to 100. Here, x is 4, making the new divisor 24. Calculate the remainder and repeat the process to get more decimal places.
Estimation and Approximation Method
This iterative method uses the formula:
\[
y_{n+1} = \frac{\left(\frac{x}{y_n} + y_n\right)}{2}
\]
For x = 2 and starting with y₁ = 1:
- Iteration 1: \[ y_1 = \frac{2 + 1}{2} = 1.5 \]
- Iteration 2: \[ y_2 = \frac{\left(\frac{2}{1.5} + 1.5\right)}{2} = 1.4166 \]
- Iteration 3: \[ y_3 = \frac{\left(\frac{2}{1.4166} + 1.4166\right)}{2} \approx 1.414215 \]
This process can be repeated to achieve the desired precision.
Applications of the Square Root of 2
The square root of 2 is significant in various fields:
- Geometry: The diagonal of a square with side length 1 is √2.
- Paper Sizes: The aspect ratio of A-series paper sizes is √2.
- Trigonometry: It appears in calculations involving 45-degree angles.
Example Problems
Here are some example problems involving the square root of 2:
- Find the diagonal of a square with side length 2 units. Using the Pythagorean theorem, the diagonal is \(2\sqrt{2} \approx 2.828\).
- Calculate the length of the diagonal of an A4 paper. The ratio of the sides is √2, maintaining the same proportion across different sizes of A-series paper.
Square Root of 3
The square root of 3, represented as \( \sqrt{3} \), is an important mathematical constant with various applications. The value of \( \sqrt{3} \) is approximately 1.732050807568877293527446341505872366, but it is commonly rounded to 1.732 for simplicity.
Properties of the Square Root of 3
- \( \sqrt{3} \) is an irrational number, meaning it cannot be expressed as a simple fraction and has an infinite number of non-repeating decimals.
- \( \sqrt{3} \) often appears in geometry, especially in relation to equilateral triangles and hexagons.
Geometric Significance
In geometry, \( \sqrt{3} \) is significant for several reasons:
- The height of an equilateral triangle with side length 2 is \( \sqrt{3} \).
- In a 30-60-90 triangle, the longer leg is \( \sqrt{3} \) times the shorter leg.
- The space diagonal of a unit cube is \( \sqrt{3} \).
Calculating the Square Root of 3
Since \( \sqrt{3} \) is not a perfect square, we use methods such as the long division method or iterative approaches to find its value.
Long Division Method
The long division method provides a systematic way to find the square root of a number:
- Pair the digits of the number from the decimal point. For 3, it becomes 3.00, 00, 00, ...
- Find the largest number whose square is less than or equal to the leftmost pair. For 3, it is 1 (since \(1^2 = 1\)).
- Subtract the square of this number from the pair, and bring down the next pair of digits. In this case, 3 - 1 = 2, and bring down 00 to make it 200.
- Double the number already found (1) and use it as the starting digit of the next divisor (20_). Find a digit \( d \) such that \( (20 + d) \times d \leq 200 \). Here, it is 7 (since \(207 \times 7 = 1449 \leq 200\)).
- Repeat the process for more pairs of digits to get a more precise value.
Applications of \( \sqrt{3} \)
- In power engineering, the voltage between two phases in a three-phase system is \( \sqrt{3} \) times the line-to-neutral voltage.
- It appears in various trigonometric functions and calculations involving regular polygons and solids.
Understanding the properties and calculation methods of \( \sqrt{3} \) is essential for various mathematical and engineering applications.
Square Root of 4
The square root of 4 is one of the simpler square roots to calculate, as 4 is a perfect square. A perfect square is an integer that is the square of another integer. In this case, the number 4 is the square of 2.
Mathematically, the square root of 4 is expressed as:
\[
\sqrt{4} = 2
\]
However, every positive number has two square roots: one positive and one negative. Thus, the square roots of 4 are:
\[
\sqrt{4} = \pm 2
\]
This means that both 2 and -2 are square roots of 4, because:
\[
2^2 = 4 \quad \text{and} \quad (-2)^2 = 4
\]
Here is a detailed step-by-step approach to finding the square root of 4:
- Identify the perfect square: Since 4 is a perfect square, we know there exists an integer whose square is 4.
- Prime Factorization: Break down 4 into its prime factors:
- \[ 4 = 2 \times 2 \]
- Pair the prime factors: Group the factors into pairs:
- \[ (2 \times 2) = 2^2 \]
- Take the square root: For every pair of identical factors, take one factor out of the square root:
- \[ \sqrt{2^2} = 2 \]
This confirms that the square root of 4 is 2.
Table of Perfect Squares Close to 4
| Number | Square |
|---|---|
| 1 | 1 |
| 2 | 4 |
| 3 | 9 |
| 4 | 16 |
| 5 | 25 |
These examples show other perfect squares and their corresponding square roots. Notice that only the perfect squares yield whole number roots.
In summary, the square root of 4 is a straightforward example due to its nature as a perfect square. This principle is fundamental in mathematics, simplifying the process of finding roots for other numbers.

Square Root of 5
The square root of 5, denoted as \( \sqrt{5} \), is an irrational number, which means it cannot be expressed as a simple fraction. The approximate value of \( \sqrt{5} \) is 2.236, which can be found using the long division method.
Long Division Method to Find \( \sqrt{5} \)
To find the square root of 5 using the long division method, follow these steps:
- Start with the number 5, written as 5.00000000 to include decimal places.
- Find a number whose square is less than 5. The number 2 fits this criterion since \( 2^2 = 4 \).
- Place 2 as the initial quotient and subtract \( 2^2 = 4 \) from 5, leaving a remainder of 1.
- Bring down two zeros, making the remainder 100. Place a decimal point in the quotient.
- Double the initial quotient (2) to get 4. Determine a number to append to 4 (forming 42) such that 42 times that number is less than or equal to 100. The number 2 works, as \( 42 \times 2 = 84 \).
- Subtract 84 from 100 to get a remainder of 16. Bring down the next pair of zeros, making it 1600.
- Repeat the process, doubling the current quotient (2.2), and find the next digit. This process continues to provide more precise digits of \( \sqrt{5} \).
Following these steps, we find:
\[
\sqrt{5} \approx 2.2360 \ldots
\]
Properties of \( \sqrt{5} \)
- \( \sqrt{5} \) is irrational, so its decimal representation is non-terminating and non-repeating.
- Geometrically, \( \sqrt{5} \) can be seen as the length of the diagonal of a rectangle with sides 1 and 2, according to the Pythagorean theorem.
- \( \sqrt{5} \) is related to the golden ratio \( \phi \) and the Lucas numbers. Specifically, the ratio of the \( n \)-th Lucas number to the \( n \)-th Fibonacci number approaches \( \sqrt{5} \) as \( n \) increases.
Applications of \( \sqrt{5} \)
The square root of 5 appears in various mathematical contexts, including algebra, geometry, and number theory. It is essential in solving quadratic equations and in the study of irrational numbers. Additionally, it appears in the construction of geometric shapes like the golden rectangle and in the analysis of continued fractions.
Square Root of 6
The square root of 6, represented as \( \sqrt{6} \), is a mathematical value which, when multiplied by itself, gives the number 6. This value is an irrational number, meaning it cannot be expressed as a simple fraction and has an infinite, non-repeating decimal expansion.
The approximate value of \( \sqrt{6} \) to several decimal places is:
- Rounded to the nearest tenth: 2.4
- Rounded to the nearest hundredth: 2.45
- Rounded to the nearest thousandth: 2.449
Here is the process of calculating \( \sqrt{6} \) using the long division method:
- Set up 6 in pairs of digits from right to left: 6.00.
- The largest perfect square less than or equal to 6 is 4 (since \( 2^2 = 4 \)).
- Subtract 4 from 6, giving 2, and bring down 00 to make 200.
- Double the quotient (2), getting 4, and use this to find the next digit in the quotient by finding the largest digit X such that \( 4X \times X \leq 200 \). This results in 4.
- Repeat the process to further refine the value.
The decimal representation of \( \sqrt{6} \) using a calculator or computer is approximately:
- Using a calculator: \( \sqrt{6} \approx 2.4495 \)
- Using Excel, Numbers, or Google Sheets:
=SQRT(6)gives \( \approx 2.449489742783 \)
The square root of 6 in exponential form is expressed as:
\( \sqrt{6} = 6^{1/2} \)
Since 6 is not a perfect square, \( \sqrt{6} \) is in its simplest radical form. It cannot be simplified further.
The value of \( \sqrt{6} \) cannot be precisely expressed as a fraction, but it can be approximated:
- \( \sqrt{6} \approx \frac{245}{100} \)
Square Root of 7
The square root of 7, denoted as \( \sqrt{7} \), is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation goes on forever without repeating. The approximate value of \( \sqrt{7} \) is 2.6457513110645906.
Methods to Calculate \( \sqrt{7} \)
- Long Division Method: This traditional method involves dividing the number into pairs of digits, estimating the largest number whose square is less than or equal to the number, and iteratively finding more precise digits of the square root.
- Prime Factorization: While effective for perfect squares, it is less useful for non-perfect squares like 7. It involves breaking down the number into its prime factors and pairing them to find the square root.
- Newton's Method: An iterative numerical method that starts with an initial guess and repeatedly improves the approximation by averaging the guess and the quotient of the original number divided by the guess.
Step-by-Step Long Division Method for \( \sqrt{7} \)
- Start by placing a bar over 7. As there are no pairs of digits, consider it as 7.0000 for more precision.
- Find the largest number whose square is less than or equal to 7. In this case, it is 2 (since \(2^2 = 4\)). Place 2 as the first digit of the square root.
- Subtract \(2^2\) (which is 4) from 7, leaving a remainder of 3. Bring down the next pair of zeros, making it 300.
- Double the quotient obtained so far (2), making it 4. Now, find a digit X such that \(40X \times X \leq 300\). The suitable digit is 6 (since \(46 \times 6 = 276\)). Place 6 as the next digit in the square root.
- Continue this process to obtain more digits for greater precision. Each step improves the approximation of \( \sqrt{7} \).
Applications of \( \sqrt{7} \)
Square roots are fundamental in various fields of science and engineering. For example:
- Geometry: The length of the diagonal of a rectangle with sides of 1 and \( \sqrt{6} \) can be calculated using the Pythagorean theorem, resulting in \( \sqrt{7} \).
- Physics: Square roots are used in formulas involving areas and volumes, such as the formula for the period of a pendulum where the length is proportional to the square root of its period.
Example Problem
Suppose you have a square garden with an area of 7 square meters. To find the side length of the garden:
| Area (A) | = | 7 m2 |
| Side length (s) | = | \(\sqrt{7}\) m ≈ 2.646 m |
Thus, the side length of the garden is approximately 2.646 meters.
Square Root of 8
The square root of 8 is an important mathematical concept that finds applications in various fields. The square root of a number \( x \) is a number \( y \) such that \( y^2 = x \). For the number 8, the square root is denoted as \( \sqrt{8} \).
Using the approximation method, we find:
\[ \sqrt{8} \approx 2.828 \]
Methods to Calculate Square Root of 8
- Prime Factorization Method:
- Find the prime factors of 8: \( 8 = 2 \times 2 \times 2 \)
- Pair the prime factors: \( 8 = (2^2) \times 2 \)
- Take the square root of the paired factor and multiply: \( \sqrt{8} = \sqrt{4 \times 2} = \sqrt{4} \times \sqrt{2} = 2 \times \sqrt{2} \approx 2.828 \)
- Long Division Method:
- Start with 8 and pair the digits from the right.
- Find the largest number whose square is less than or equal to 8, which is 2 (since \( 2^2 = 4 \)).
- Use the long division process to find more decimal places.
- The detailed steps yield \( \sqrt{8} \approx 2.828 \).
Applications of the Square Root of 8
Understanding the square root of 8 is crucial in various scientific and engineering calculations. Here are some practical applications:
- Geometry: Calculating the diagonal of a square with side length 4 units. The diagonal \( d \) can be found using the formula \( d = \sqrt{2a^2} \), where \( a \) is the side length. For \( a = 4 \):
\[ d = \sqrt{2 \times 4^2} = \sqrt{2 \times 16} = \sqrt{32} = 4\sqrt{2} = 4 \times 2.828 = 11.312 \text{ units} \]
- Physics: In mechanics, understanding the square root of 8 helps in solving problems related to acceleration and kinetic energy where such calculations are necessary.
- Statistics: It helps in calculating standard deviation and variance, which are fundamental in data analysis and interpretation.
Conclusion
The square root of 8, approximately 2.828, is an irrational number that plays a significant role in various mathematical and practical applications. Mastery of calculating square roots, including non-perfect squares like 8, enhances problem-solving skills and mathematical understanding.

Square Root of 9
The square root of 9 is a fundamental concept in mathematics. The value is:
\[
\sqrt{9} = 3
\]
This is because 3 multiplied by itself equals 9:
\[
3 \times 3 = 9 \quad \text{or} \quad 3^2 = 9
\]
Below are some methods to understand and calculate the square root of 9:
- Prime Factorization Method:
- Long Division Method:
Prime factorization of 9 is:
\[
9 = 3 \times 3
\]
Pairing the prime factors, we get:
\[
\sqrt{9} = 3
\]
For larger numbers, the long division method can be used, though for perfect squares like 9, the result is straightforward. The method involves dividing the number and averaging the divisors iteratively.
Applications of Square Root of 9
Knowing the square root of 9 is useful in various mathematical applications such as solving quadratic equations, geometry, and algebra. For example, in geometry, understanding square roots is crucial for calculating the side lengths of squares and right triangles.
Real-Life Examples
- If you have a square-shaped garden with an area of 9 square meters, each side of the garden would be 3 meters because the side length is the square root of the area.
- In physics, square roots are used to solve equations involving area and volume, where 9 might be the resultant value needing a square root calculation.
Interactive Learning
Using tools like a calculator or mathematical software can help in verifying the square root of numbers, including non-perfect squares.
For further practice, consider solving problems where the area or side lengths involve square roots, and use the prime factorization or long division methods to solidify your understanding.
Square Root of 10
The square root of 10 is an irrational number, meaning it cannot be exactly expressed as a simple fraction. The approximate value of the square root of 10 is:
\[
\sqrt{10} \approx 3.162
\]
This value is derived through methods such as the long division method, which is useful for finding the square roots of non-perfect squares. Below, we explain the steps involved in calculating the square root of 10 using the long division method.
Steps to Calculate Square Root of 10
- Place a bar over every pair of digits of the number starting from the decimal point (right side). For 10, it would look like |10.
- Find the largest number whose square is less than or equal to the leftmost pair. In this case, 32 = 9, which is less than 10.
- Subtract 9 from 10, giving a remainder of 1. Bring down a pair of zeros to the right of this remainder, making it 100.
- Double the quotient (3) to get 6. Now find a number (x) such that 6x * x is less than or equal to 100. The number is 1, since 61 * 1 = 61, which is less than 100.
- Subtract 61 from 100, giving a remainder of 39. Bring down another pair of zeros, making it 3900.
- Double the quotient (31) to get 62. Find a number (x) such that 62x * x is less than or equal to 3900. The number is 6, since 626 * 6 = 3756, which is less than 3900.
- Repeat this process to get a more accurate value.
After following the steps, you will find that:
\[
\sqrt{10} \approx 3.162
\]
Properties of the Square Root of 10
- The square root of 10 is an irrational number.
- It is not a perfect square, hence its square root is a non-terminating, non-repeating decimal.
- The value lies between the square roots of 9 and 16 (i.e., between 3 and 4).
Applications of Square Root of 10
The square root of 10 is used in various mathematical and scientific calculations. For example:
- Geometry: To find the side length of a square with an area of 10 square units.
- Physics: In calculations involving the diagonal of a rectangle with sides in a ratio that leads to an area of 10 square units.
- Engineering: In various design and structural calculations where precise measurements are required.
Example Problem
Let's solve a problem using the square root of 10:
Suppose you have a circular tabletop with an area of \(10\pi\) square inches. To find the radius of the tabletop:
\[
\text{Area} = \pi r^2 \implies 10\pi = \pi r^2 \implies r^2 = 10 \implies r = \sqrt{10} \approx 3.162 \text{ inches}
\]
Thus, the radius of the tabletop is approximately 3.162 inches.
Applications of Square Roots
Square roots are a fundamental mathematical concept with a wide range of practical applications across various fields. Here are some key applications:
-
Construction and Architecture
Square roots are used to calculate the lengths of diagonal braces and other angled components in construction to ensure structural integrity. For example, the Pythagorean Theorem, which involves square roots, helps determine the length of a diagonal support in a right triangle.
Formula: \( \sqrt{a^2 + b^2} = c \)
-
Engineering
In engineering, square roots help calculate the natural frequency of structures such as bridges and buildings, allowing engineers to predict how these structures will respond to various loads, like wind or traffic.
-
Finance
Square roots are used to calculate stock market volatility. By taking the square root of the variance of a stock's returns, investors can assess the risk associated with a particular investment.
-
Science
Square roots are essential in various scientific calculations, such as determining the velocity of a moving object, the intensity of sound waves, and the amount of radiation absorbed by a material.
-
Statistics
In statistics, square roots are used to calculate the standard deviation, which is a measure of the amount of variation or dispersion in a set of values. The standard deviation is the square root of the variance.
Formula: \( \sigma = \sqrt{\frac{\sum (x_i - \mu)^2}{N}} \)
-
Computer Science and Cryptography
Square roots are used in algorithms for encryption and in computer graphics to calculate distances between points. They are also essential in developing secure communication systems.
-
Navigation
Square roots are used in navigation to compute distances between points on a map or globe. The distance formula, derived from the Pythagorean Theorem, helps pilots and sailors determine their course.
Formula in 2D: \( D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \)
-
Physics
In physics, square roots are used in formulas to determine physical quantities such as the kinetic energy of an object. For example, the velocity \(v\) of an object can be determined using the equation:
Formula: \( v = \sqrt{\frac{2KE}{m}} \)
These examples highlight the diverse and crucial applications of square roots in everyday life, making them an indispensable tool in various domains.
Real-Life Examples of Square Roots
Square roots have numerous practical applications in various fields. Here are some detailed real-life examples:
-
Construction:
In construction, square roots are used to determine the length of diagonal braces and other angled components necessary to maintain the structural integrity of a building. For example, using the Pythagorean theorem, the length of the hypotenuse (the diagonal) can be calculated by taking the square root of the sum of the squares of the other two sides.
-
Navigation:
Square roots are crucial in navigation for computing distances between two points on a map or globe. The distance formula in two dimensions is derived from the Pythagorean theorem: . In three dimensions, it includes the z-coordinates as well.
-
Finance:
In finance, square roots are used to calculate stock market volatility. The standard deviation, a measure of how much a stock's price fluctuates, is the square root of the variance of the stock's returns.
-
Physics:
Square roots are frequently used in physics to calculate various properties such as the velocity of moving objects, the intensity of sound waves, and the amount of radiation absorbed by a material.
-
Computer Graphics:
In computer graphics, square roots are used to compute distances between points, lengths of vectors, and other geometric calculations essential for rendering images and animations.
-
Architecture:
Architects use square roots to calculate areas and ensure accurate measurements for designing spaces. For example, to determine the number of tiles needed for a floor, an architect calculates the area by squaring the length and width of the space.
These examples highlight the importance and versatility of square roots in various real-life applications, demonstrating how fundamental mathematical concepts are applied in practical scenarios.

Tools and Techniques for Finding Square Roots
Finding square roots is a fundamental mathematical operation with numerous applications in various fields such as science, engineering, and finance. There are several tools and techniques available for calculating square roots, ranging from manual methods to advanced computational algorithms. Below, we will explore these methods in detail.
1. Manual Methods
Prime Factorization
This method is effective for perfect squares. To find the square root of a number using prime factorization, follow these steps:
- Factorize the number into its prime factors.
- Pair the prime factors.
- Multiply one number from each pair to get the square root.
For example, to find the square root of 81:
- Prime factors of 81: \( 3 \times 3 \times 3 \times 3 \)
- Pair the factors: \( (3 \times 3) \times (3 \times 3) \)
- Square root: \( 3 \times 3 = 9 \)
Long Division Method
This method is useful for both perfect and imperfect squares. Here's how it works:
- Group the digits in pairs, starting from the decimal point.
- Find the largest number whose square is less than or equal to the first group.
- Subtract the square of this number from the first group and bring down the next pair of digits.
- Double the number in the quotient and use it as a new divisor, finding the largest digit to complete the divisor such that its product with the digit gives a number less than or equal to the current remainder.
- Repeat the process until you reach the desired precision.
For example, finding the square root of 5 using the long division method involves:
- Writing 5 as 5.000000
- Finding the largest number whose square is less than or equal to 5 (which is 2)
- Using 2 as the first digit and proceeding with the division and subtraction steps.
2. Computational Methods
Newton's Method (Heron's Method)
Newton's method is an iterative approach to find increasingly accurate approximations of square roots:
- Start with an initial guess \( x_0 \).
- Use the formula \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{S}{x_n} \right) \) where \( S \) is the number for which the square root is being calculated.
- Repeat the process until the difference between successive approximations is within a desired tolerance.
Using Libraries and Packages
Programming languages offer built-in functions to simplify square root calculations. For example:
- Python:
math.sqrt() - Java:
Math.sqrt() - C++:
sqrt()from thecmathlibrary
These functions use optimized algorithms to return accurate results efficiently.
Software Tools
Various software tools and calculators can quickly find square roots. These include scientific calculators, online square root calculators, and mathematical software like MATLAB and Wolfram Alpha.
3. Applications in Real-World Problems
Understanding and applying square roots is essential in fields such as:
- Geometry: Calculating areas and dimensions.
- Physics: Solving equations involving acceleration and force.
- Finance: Computing compound interest and standard deviation in statistics.
Mastering the various methods and tools for finding square roots equips you with the skills to tackle a wide range of mathematical challenges effectively.
Conclusion
The study of square roots, particularly those of numbers between 1 and 10, is fundamental in understanding various mathematical principles. Square roots simplify complex calculations, help in solving quadratic equations, and are essential in geometry and algebra.
Memorizing the square roots of numbers 1 to 10 can significantly enhance one's mathematical proficiency. For instance:
- √1 = 1
- √2 ≈ 1.414
- √3 ≈ 1.732
- √4 = 2
- √5 ≈ 2.236
- √6 ≈ 2.449
- √7 ≈ 2.646
- √8 ≈ 2.828
- √9 = 3
- √10 ≈ 3.162
Understanding these values is crucial for faster problem-solving and deeper comprehension of mathematical relationships. Moreover, the applications of square roots extend beyond theoretical mathematics into real-world problems, such as calculating distances, areas, and in various fields like physics, engineering, and computer science.
Overall, mastering the concept of square roots opens up a vast array of possibilities and tools that are indispensable in both academic and practical scenarios. Continuous practice and utilization of techniques such as prime factorization and the long division method will ensure proficiency in finding square roots efficiently.
Khám phá các hình vuông hoàn hảo và căn bậc hai từ 1 đến 10 với Thầy J. Video này sẽ giúp bạn nắm vững các khái niệm toán học cơ bản về hình vuông và căn bậc hai một cách dễ hiểu.
Hình Vuông Hoàn Hảo và Căn Bậc Hai (1-10) | 10 Hình Vuông Hoàn Hảo Đầu Tiên & Căn Bậc Hai | Toán Học với Thầy J
READ MORE:
Video này cung cấp danh sách các số bình phương từ 1 đến 10 để giúp học sinh học toán hiệu quả hơn.
Danh sách các số bình phương từ 1 đến 10 | Toán học