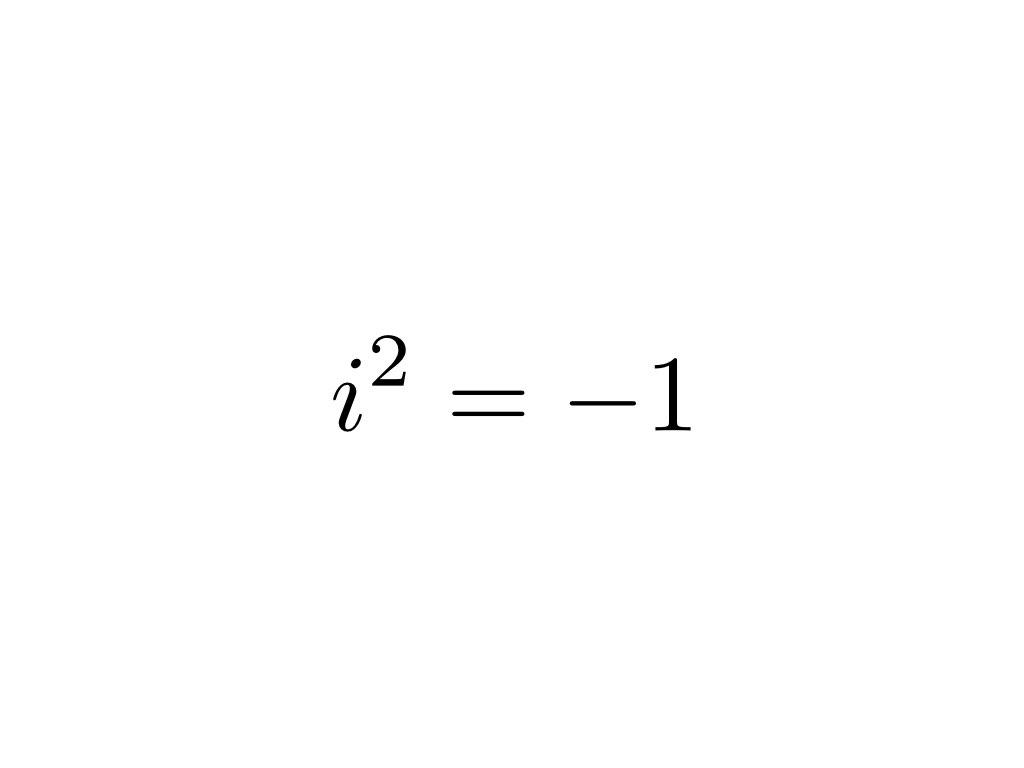Topic 1/2 pi r squared: Understanding the formula 1/2 πr² is essential for mastering circle area calculations. This article explores the derivation, practical applications, and fascinating history behind this fundamental mathematical concept. Whether you're a student or a math enthusiast, dive in to enhance your knowledge and appreciation of geometry.
Table of Content
- Understanding the Formula for the Area of a Circle
- Introduction
- Definition of Circle and Disk
- Formula for the Area of a Circle
- Historical Background
- Derivation of the Area Formula
- Comparisons with Other Geometric Shapes
- Real-World Applications
- Common Misconceptions and Mistakes
- Practical Examples and Problems
- Conclusion
- YOUTUBE: Xem video về cách tính diện tích hình tròn bằng công thức \( A = \frac{1}{2} \pi r^2 \). Hãy xem và tìm hiểu cách áp dụng công thức này vào các ví dụ thực tế.
Understanding the Formula for the Area of a Circle
The formula for the area of a circle is an essential concept in geometry, represented as:
Key Concepts
- Radius (r): The distance from the center of the circle to any point on its circumference.
- Diameter (d): Twice the radius, spanning the circle through its center.
- Pi (π): A constant approximately equal to 3.14159, representing the ratio of a circle's circumference to its diameter.
Deriving the Area Formula
The area of a circle can be derived through integration or by understanding the relationship between the radius and the circle's circumference. The area covered by the circle is proportional to the square of its radius:
Calculating the Area
- Identify the radius (r) of the circle.
- Square the radius (multiply it by itself).
- Multiply the squared radius by π.
For example, if the radius of a circle is 5 cm, the area is calculated as:
Applications of the Formula
The area formula is widely used in various fields, including architecture, engineering, and everyday life scenarios like determining the size of a pizza or the amount of material needed for a circular tablecloth.
| Given | Formula | Calculation |
|---|---|---|
| Radius (r) | ||
| Diameter (d) |
By understanding and applying the formula for the area of a circle, we can solve a variety of practical problems involving circular shapes and spaces.
READ MORE:
Introduction
The formula \( \frac{1}{2} \pi r^2 \) is commonly encountered when discussing the area of geometric shapes, particularly circles. This formula is a fundamental concept in geometry, essential for calculating the area enclosed by a circular boundary. Understanding its derivation and applications provides a deeper insight into various mathematical principles.
The area of a circle, represented by \( \pi r^2 \), where \( r \) is the radius, can be visualized and derived through several methods, including integral calculus and geometric proofs by ancient mathematicians like Archimedes. The formula is crucial in both theoretical and practical scenarios, from academic studies to real-world applications.
This section explores the derivation, significance, and practical examples of the area formula \( \frac{1}{2} \pi r^2 \), ensuring a comprehensive understanding of this key mathematical concept.
- Understanding the Formula: \( \pi r^2 \)
- Geometric Derivation Methods
- Applications in Real Life
- Examples and Practice Problems
By delving into these topics, readers will gain a robust comprehension of the circle's area formula and its diverse applications, reinforcing foundational geometry knowledge.
Definition of Circle and Disk
A circle is a simple geometric shape consisting of all points in a plane that are at a given distance (the radius) from a given point (the center). The disk, on the other hand, is the region of the plane enclosed by the circle.
- Circle: The boundary line of the shape.
- Disk: The interior region enclosed by the circle.
In mathematical terms, if the center of the circle is at the point \( (h, k) \) and the radius is \( r \), then the set of all points \( (x, y) \) that form the circle is defined by the equation:
The area of a disk is given by the formula:
For example, if the radius \( r \) of the disk is 5 units, the area \( A \) is:
The concept of the circle and disk are foundational in geometry, playing a critical role in various mathematical applications and real-world scenarios.
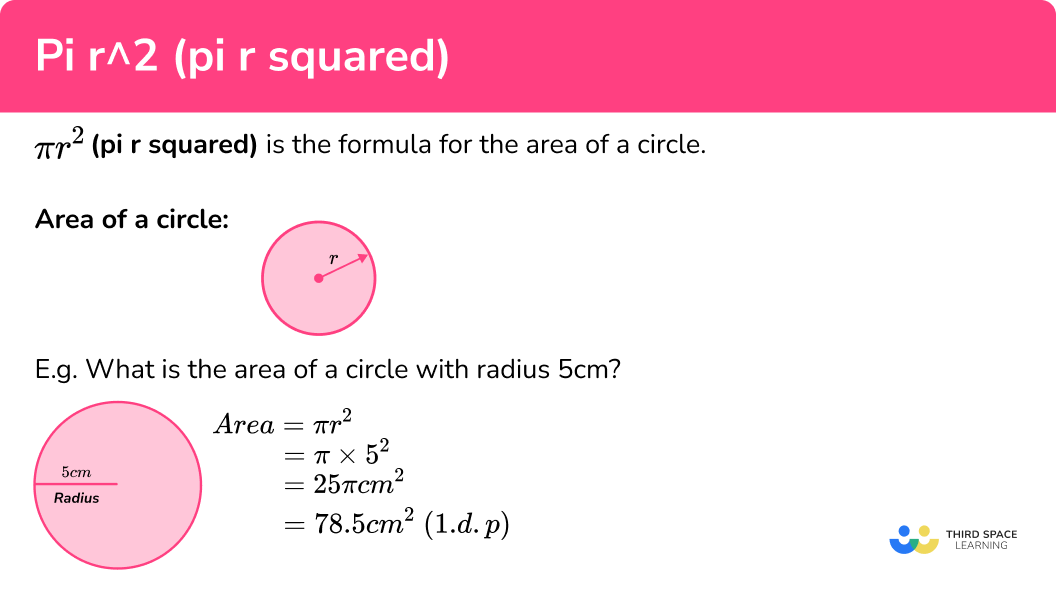
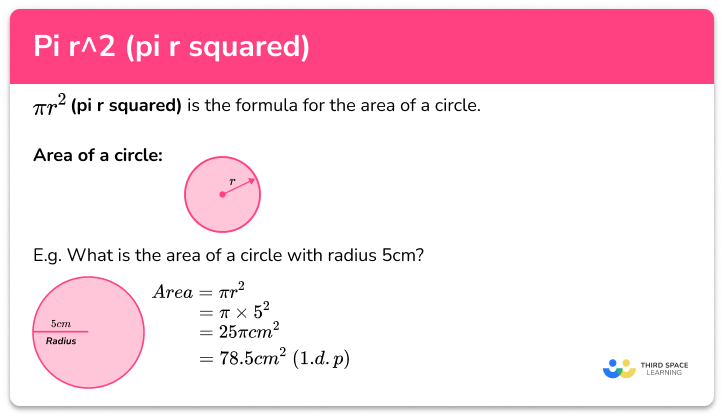
Formula for the Area of a Circle
The formula for the area of a circle is a fundamental concept in geometry, which is derived using the constant π (Pi) and the radius of the circle. The area is given by:
\[ A = \pi r^2 \]
Where:
- \( A \) is the area of the circle.
- \( \pi \) (Pi) is a mathematical constant approximately equal to 3.14159.
- \( r \) is the radius of the circle.
Here is a step-by-step process to understand and calculate the area of a circle:
- Identify the radius (r) of the circle. The radius is the distance from the center of the circle to any point on its circumference.
- Square the radius: Calculate \( r^2 \) by multiplying the radius by itself.
- Multiply the squared radius by π (Pi): \( A = \pi \times r^2 \). This gives you the area of the circle.
For example, if the radius of a circle is 5 units, the area calculation would be:
\[ A = \pi \times 5^2 = \pi \times 25 \approx 78.54 \text{ square units} \]
This formula is useful in various practical applications such as determining the area of circular plots, fields, or any round objects.
| Radius (r) | Area (A) |
|---|---|
| 1 | \( \pi \) |
| 2 | \( 4\pi \) |
| 3 | \( 9\pi \) |
| 4 | \( 16\pi \) |
| 5 | \( 25\pi \) |
In summary, the formula for the area of a circle, \( \pi r^2 \), is an essential tool in mathematics and geometry, providing a straightforward method to calculate the space within a circular boundary.
Historical Background
The concept of calculating the area of a circle has deep historical roots, originating in ancient civilizations. Early mathematicians in Greece, such as Archimedes, made significant contributions to understanding the properties of circles.
Archimedes developed a method for approximating the area of a circle by inscribing polygons within it and calculating their areas. This method led to the realization that the area of a circle is proportional to the square of its radius. Over time, this understanding evolved into the formula we use today, A = \(\pi r^2\), where \(A\) is the area and \(r\) is the radius.
Further advancements were made during the Middle Ages and the Renaissance, where mathematicians in the Islamic world and Europe expanded upon these early works. They refined the calculation methods and solidified the use of π (pi) as a mathematical constant. The historical journey of discovering the area of a circle highlights the collaborative nature of mathematical development across cultures and eras.

Derivation of the Area Formula
The area of a circle can be derived through several methods, each illustrating the relationship between the circle's radius and its area. Here, we will explore two common derivations: using the concept of a rectangle and using the concept of a triangle.
- Using the Area of a Rectangle:
- Divide the circle into a large number of equal sectors.
- Arrange these sectors alternately to form a shape resembling a parallelogram.
- As the number of sectors increases, this shape approaches a rectangle.
- The base of this rectangle is half the circumference of the circle, \( \pi r \).
- The height of this rectangle is the radius of the circle, \( r \).
- Thus, the area of the rectangle is \( \pi r \times r = \pi r^2 \).
- Using the Area of a Triangle:
- Consider the circle as being composed of concentric circles, resembling a spiral if cut and laid flat.
- When laid flat, this spiral forms a triangle where the base is the circumference, \( 2\pi r \), and the height is the radius, \( r \).
- The area of the triangle is given by \( \frac{1}{2} \times \text{base} \times \text{height} \).
- Substitute the values to get \( \frac{1}{2} \times 2\pi r \times r = \pi r^2 \).
These derivations help illustrate why the formula for the area of a circle is \( A = \pi r^2 \), linking geometric shapes to circular measurements.
Comparisons with Other Geometric Shapes
When comparing the area of a circle, calculated using the formula \( \pi r^2 \), to other geometric shapes, several interesting observations can be made:
-
Square:
- The area of a square with side length \( s \) is \( s^2 \). For a square to have the same area as a circle, \( s \) must equal the diameter of the circle divided by \( \sqrt{\pi} \).
-
Rectangle:
- The area of a rectangle is given by \( l \times w \). A rectangle with a length of \( 2r \) (the diameter of the circle) and a width of \( \frac{\pi r}{2} \) will have the same area as the circle.
-
Triangle:
- The area of an equilateral triangle with side length \( s \) is \( \frac{\sqrt{3}}{4}s^2 \). To have the same area as a circle, \( s \) must be approximately \( 2 \sqrt{\frac{\pi}{\sqrt{3}}}r \).
-
Ellipse:
- The area of an ellipse is \( \pi a b \), where \( a \) and \( b \) are the semi-major and semi-minor axes. If \( a = b = r \), the ellipse is a circle.
These comparisons highlight the unique properties of circles and provide insights into the relationships between different geometric shapes and their areas.
Real-World Applications
The formula for the area of a circle, \( A = \frac{1}{2} \pi r^2 \), finds numerous applications across various fields:
- Engineering: Used in calculating the surface area of circular components like discs and cylinders in mechanical and civil engineering.
- Physics: Essential for determining areas related to circular motion, such as the cross-sectional area of circular conductors or the area swept by a rotating object.
- Architecture: Applies to the design of circular structures, from domes to circular windows, optimizing space and structural integrity.
- Mathematics Education: Frequently used to introduce students to geometric formulas and their applications in real-world scenarios.
- Economics: Relevant in pricing circular items based on their surface area, influencing material costs and pricing strategies.
Common Misconceptions and Mistakes
There are several common misconceptions and mistakes associated with the formula \( A = \frac{1}{2} \pi r^2 \):
- Confusion with Diameter: Some mistakenly use the diameter instead of the radius in the formula, leading to incorrect area calculations.
- Application in Three Dimensions: Incorrectly applying the formula to calculate volumes or other three-dimensional properties, when it specifically relates to two-dimensional area.
- Decimal and Fractional Misinterpretations: Misunderstanding the use of π and the fraction \( \frac{1}{2} \), leading to errors in converting between decimal and fractional representations.
- Contextual Misuse: Using the formula in contexts where it does not apply, such as in non-circular geometries or situations where surface area differs from true circular area.
- Historical and Cultural Misconceptions: Failing to recognize the historical development of the formula and its cultural significance, potentially leading to misinterpretations of its applications.
Practical Examples and Problems
Understanding the formula \( A = \frac{1}{2} \pi r^2 \) through practical examples and problems:
- Example 1: Calculate the area of a circular swimming pool with a radius of 10 meters using the formula.
- Example 2: Determine the surface area of a circular pizza with a diameter of 16 inches, converting units appropriately.
- Problem 1: Given the area of a circular garden is 100 square meters, find the radius using the formula.
- Problem 2: Explore how changes in the radius affect the area, considering practical scenarios like resizing circular gardens or adjusting circular paintings.
- Application: Apply the formula in real-life scenarios, such as estimating the material required for circular carpets or planning circular gardens for optimal space utilization.
Conclusion
In conclusion, the formula \( A = \frac{1}{2} \pi r^2 \) for the area of a circle is a fundamental tool with broad applications:
- It provides a precise method to calculate the area of circular shapes, essential in various fields from engineering to mathematics education.
- The formula's simplicity allows for easy application in both theoretical problems and real-world scenarios involving circular geometries.
- Understanding this formula enhances appreciation for the elegance of mathematics and its practical utility in everyday life.
- Despite misconceptions, mastering the correct use of \( \pi \) and the radius ensures accurate calculations, promoting mathematical literacy and problem-solving skills.
- Continued exploration and application of this formula contribute to ongoing advancements in science, technology, and design.
Xem video về cách tính diện tích hình tròn bằng công thức \( A = \frac{1}{2} \pi r^2 \). Hãy xem và tìm hiểu cách áp dụng công thức này vào các ví dụ thực tế.
Diện tích hình tròn, công thức được giải thích | Video hấp dẫn
READ MORE:
Xem video về các khái niệm về hình tròn, chu vi và diện tích để hiểu rõ hơn về các công thức \( C = 2 \pi r \) và \( A = \pi r^2 \). Hãy khám phá và nâng cao kiến thức toán học của bạn với Math Antics.
Toán Học Sự Kiện - Hình Tròn, Chu vi và Diện tích