Topic what is the perimeter of wxy: Understanding the perimeter of triangle WXY is essential for solving various geometric problems. This article delves into different methods to calculate the perimeter, providing practical examples and step-by-step instructions. Whether you're a student or a geometry enthusiast, this guide will help you master the concept of perimeters in triangles.
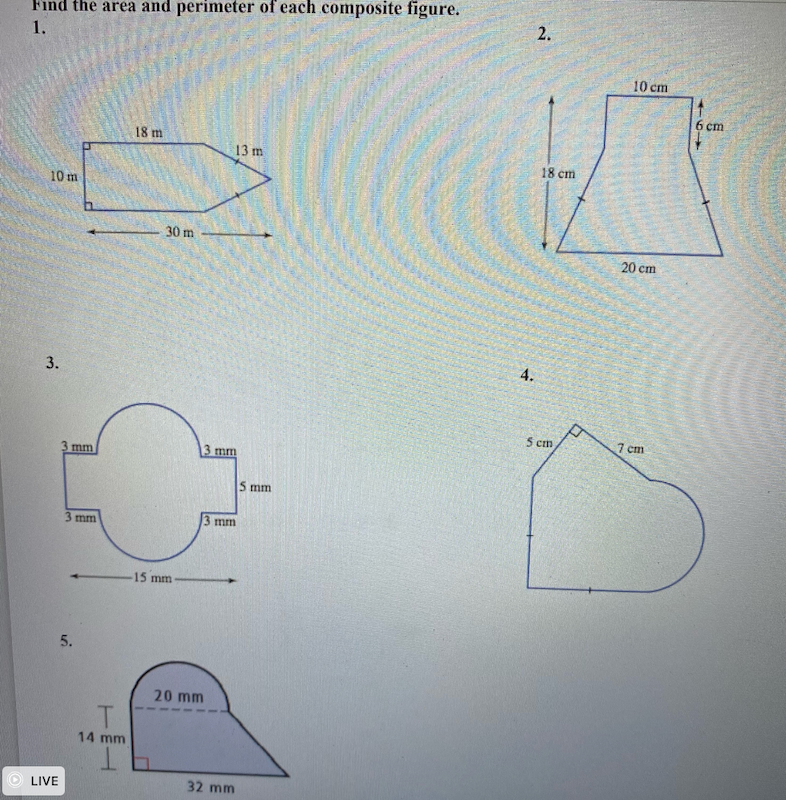
Table of Content
- Understanding the Perimeter of Triangle WXY
- Introduction
- Understanding Triangle Perimeters
- Midsegments in Triangles
- Calculating the Perimeter of Triangle WXY
- Examples and Practice Problems
- Special Cases and Considerations
- Applications in Real World Geometry
- YOUTUBE: Xem video về cách tính diện tích và chu vi của tam giác trên mặt phẳng tọa độ. Hiểu cách tính chu vi của tam giác WXY trong bài viết của chúng tôi?
Understanding the Perimeter of Triangle WXY
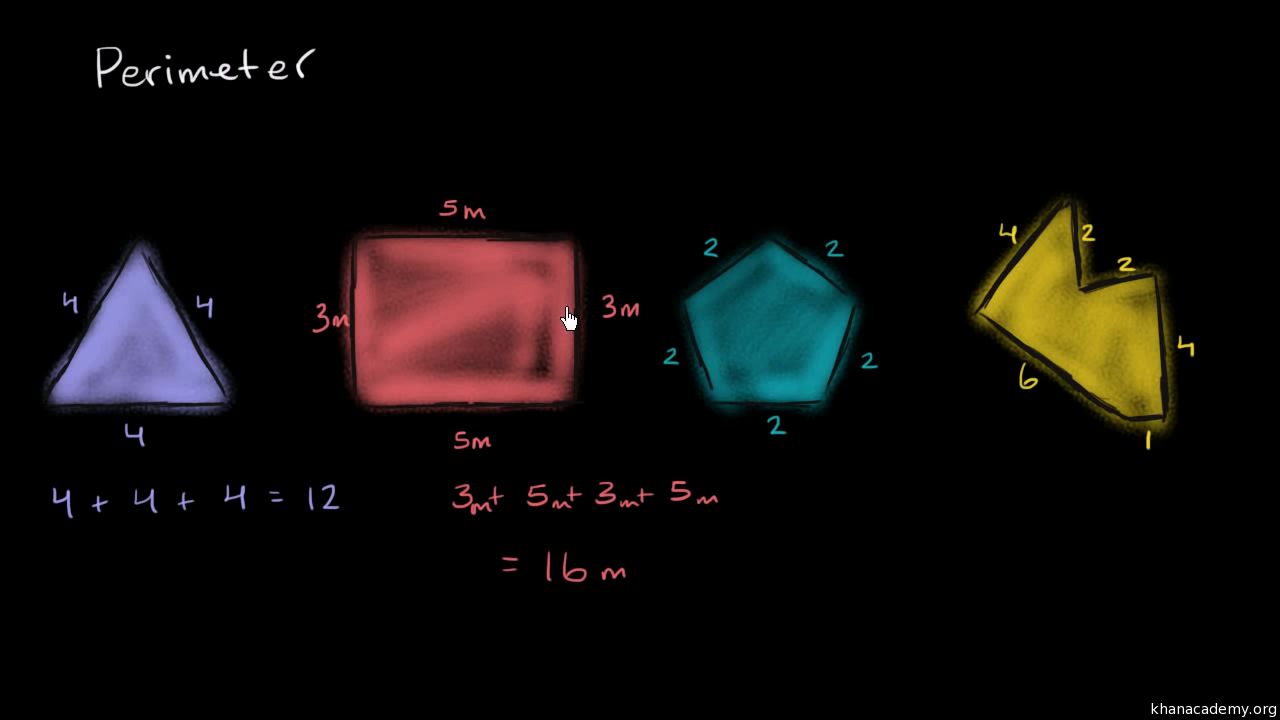
The perimeter of a triangle is the sum of the lengths of its three sides. To calculate the perimeter of triangle WXY, we need the measurements of all its sides. Here are some examples and methods to find the perimeter:
Example Calculation
Given that triangle WXY has sides WX, XY, and YW with lengths:
- WX = 5 cm
- XY = 7 cm
- YW = 10 cm
The perimeter \( P \) of triangle WXY is calculated as:
\[
P = WX + XY + YW = 5 \, \text{cm} + 7 \, \text{cm} + 10 \, \text{cm} = 22 \, \text{cm}
\]
Using Midsegments
If QR, RS, and SQ are midsegments of triangle WXY, then each midsegment is half the length of the opposite side:
- If QR = 4.56 cm, then WX = 2 \times 4.56 = 9.12 cm
- If RS = 4.08 cm, then XY = 2 \times 4.08 = 8.16 cm
- If SQ = 5.58 cm, then YW = 2 \times 5.58 = 11.16 cm
The perimeter \( P \) is:
\[
P = WX + XY + YW = 9.12 \, \text{cm} + 8.16 \, \text{cm} + 11.16 \, \text{cm} = 28.44 \, \text{cm}
\]
Similar Triangles
If triangle WXY is similar to another triangle with a known perimeter and a scale factor, the perimeter can be found by applying the scale factor. For example, if triangle CDE is similar to WXY with a scale factor of 4:3 and the perimeter of CDE is 64 cm, then the perimeter of WXY is:
\[
P_{WXY} = P_{CDE} \times \frac{3}{4} = 64 \, \text{cm} \times \frac{3}{4} = 48 \, \text{cm}
\]
Using a Right Triangle
For a right triangle WXY with legs WX and WY, and hypotenuse XY, if the lengths are:
- WX = 3 cm
- WY = 4 cm
- XY = 5 cm (using Pythagorean theorem)
The perimeter \( P \) is:
\[
P = WX + WY + XY = 3 \, \text{cm} + 4 \, \text{cm} + 5 \, \text{cm} = 12 \, \text{cm}
\]
Special Cases
In certain design and architectural contexts, the term "perimeter" might refer to the boundary length of a specific area or structure. For example, the New York Aquarium project involved creating an engaging perimeter for visitors, showcasing the diverse applications of perimeter calculations in practical scenarios.
Understanding how to calculate the perimeter of a triangle like WXY helps in various fields such as mathematics, architecture, and engineering.

READ MORE:
Introduction
The perimeter of triangle WXY is a fundamental concept in geometry, representing the total distance around the triangle. To find the perimeter, you need the lengths of all three sides: WX, XY, and YW. The formula to calculate the perimeter \( P \) is:
\[
P = WX + XY + YW
\]
Understanding how to determine these lengths can involve various methods, including using the Pythagorean theorem for right triangles, properties of midsegments, or applying similarity ratios. This section will guide you through the detailed steps and examples to accurately calculate the perimeter of triangle WXY.
Understanding Triangle Perimeters
The perimeter of a triangle, such as WXY, is the sum of the lengths of its sides. To understand how to find the perimeter, we need to follow a series of steps:
- Identify the lengths of all three sides of the triangle. In this case, let's denote the sides as WX, XY, and YW.
- Use the formula for the perimeter of a triangle, which is the sum of the lengths of the sides:
\[
P = WX + XY + YW
\] - Measure each side length accurately. This can be done using various methods depending on the given information:
- If you know the coordinates of the vertices, use the distance formula to calculate the side lengths.
- If the triangle is right-angled, apply the Pythagorean theorem to find the lengths of the sides.
- If the triangle is similar to another triangle, use the scale factor to determine the side lengths.
- Add the lengths of the three sides to find the perimeter.
For example, if the lengths of WX, XY, and YW are 5 units, 7 units, and 9 units respectively, the perimeter would be:
\[
P = 5 + 7 + 9 = 21 \text{ units}
\]
Understanding these steps allows you to determine the perimeter of any triangle, including WXY, effectively and accurately.
Midsegments in Triangles
A midsegment in a triangle is a line segment that connects the midpoints of two sides of the triangle. This segment has special properties and is an essential concept in triangle geometry.
The key properties of a midsegment in a triangle are:
- The midsegment is parallel to the third side of the triangle.
- The length of the midsegment is half the length of the third side.
To understand these properties, let's consider a triangle ABC where D, E, and F are the midpoints of sides AB, BC, and CA respectively. The midsegment DE will be parallel to side AC and its length will be half of AC.
Here are the steps to find a midsegment:
- Identify the midpoints of two sides of the triangle.
- Draw a line segment connecting these midpoints.
Let's look at an example:
Consider triangle ABC with vertices A(0, 0), B(4, 0), and C(2, 6). The midpoints of sides AB and AC can be calculated as follows:
| Midpoint of AB: | D = ((0+4)/2, (0+0)/2) = (2, 0) |
| Midpoint of AC: | E = ((0+2)/2, (0+6)/2) = (1, 3) |
The segment DE is the midsegment, parallel to side BC, and its length is half of BC. If the length of BC is calculated using the distance formula:
\[
BC = \sqrt{(4-2)^2 + (0-6)^2} = \sqrt{4 + 36} = \sqrt{40} = 2\sqrt{10}
\]
The length of DE would be:
\[
DE = \frac{1}{2} \times BC = \frac{1}{2} \times 2\sqrt{10} = \sqrt{10}
\]
Understanding midsegments helps in solving various geometric problems and proves useful in triangle similarity and congruence theorems.
Calculating the Perimeter of Triangle WXY
To calculate the perimeter of triangle WXY, we need to find the sum of the lengths of all three sides: WX, WY, and XY. The perimeter (P) of a triangle is given by the formula:
Depending on the information available about the triangle, there are several methods to determine its perimeter:
- Using Side Lengths: If the lengths of all three sides, WX, WY, and XY, are known, simply add them together to find the perimeter.
- Using Coordinates: If the coordinates of the vertices W, X, and Y are known, you can use the distance formula to find the lengths of the sides and then add them up to find the perimeter.
- Using Special Properties: If the triangle is a special type, such as equilateral, isosceles, or right-angled, you can use the properties of these triangles to calculate the perimeter more efficiently.
Let's explore each of these methods in more detail:
| Method | Formula | Notes |
| Using Side Lengths | \( P = WX + WY + XY \) | Requires knowledge of all three side lengths. |
| Using Coordinates | \( P = \sqrt{(x2-x1)^2 + (y2-y1)^2} + \sqrt{(x3-x2)^2 + (y3-y2)^2} + \sqrt{(x3-x1)^2 + (y3-y1)^2} \) | Requires knowledge of the coordinates of the vertices. |
| Using Special Properties | Varies depending on the type of triangle (e.g., equilateral, isosceles, right-angled). | Can simplify calculations based on known properties. |
Once you have determined the method that best suits the information available about triangle WXY, you can proceed to calculate its perimeter accordingly.

Examples and Practice Problems
Let's work through some examples and practice problems to reinforce our understanding of calculating the perimeter of triangle WXY.
- Example 1: Given that the lengths of the sides WX, WY, and XY are 5 cm, 8 cm, and 7 cm respectively, calculate the perimeter of triangle WXY.
- Example 2: The coordinates of the vertices W, X, and Y are (2, 3), (5, 7), and (8, 4) respectively. Calculate the perimeter of triangle WXY.
- Practice Problem: For the triangle WXY with side lengths WX = 6 cm, WY = 10 cm, and XY = 8 cm, calculate its perimeter.
Using the formula for the perimeter of a triangle, \( P = WX + WY + XY \), we substitute the given values:
\[ P = 5\,cm + 8\,cm + 7\,cm = 20\,cm \]So, the perimeter of triangle WXY is 20 cm.
Using the distance formula to find the lengths of the sides:
\[ WX = \sqrt{(5-2)^2 + (7-3)^2} = \sqrt{9 + 16} = \sqrt{25} = 5\,units \] \[ WY = \sqrt{(8-5)^2 + (4-7)^2} = \sqrt{9 + 9} = \sqrt{18} \approx 4.24\,units \] \[ XY = \sqrt{(8-2)^2 + (4-3)^2} = \sqrt{36 + 1} = \sqrt{37} \approx 6.08\,units \]Adding these side lengths together:
\[ P = 5\,units + 4.24\,units + 6.08\,units \approx 15.32\,units \]So, the perimeter of triangle WXY is approximately 15.32 units.
Use the formula \( P = WX + WY + XY \) and substitute the given values to find the perimeter.
Calculate \( P = 6\,cm + 10\,cm + 8\,cm \) to find the perimeter of the triangle.
Special Cases and Considerations
When dealing with the calculation of the perimeter of triangle WXY, there are several special cases and considerations to keep in mind:
- Equilateral Triangle: If triangle WXY is equilateral, meaning all three sides are of equal length, then calculating the perimeter becomes straightforward. In this case, the perimeter is simply three times the length of one side, i.e., \( P = 3 \times \text{length of side} \).
- Isosceles Triangle: An isosceles triangle has two sides of equal length. If WXY is an isosceles triangle, the perimeter can be calculated by adding the lengths of the two equal sides and the length of the third side, i.e., \( P = 2 \times \text{length of equal side} + \text{length of third side} \).
- Right-angled Triangle: In a right-angled triangle, one angle is a right angle (90 degrees). If triangle WXY is right-angled, we can use the Pythagorean theorem to find the length of the third side if the lengths of the other two sides are known. Once all side lengths are known, the perimeter can be calculated as usual.
- Triangle Inequality Theorem: According to the triangle inequality theorem, the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. When calculating the perimeter, ensure that this condition is met for triangle WXY.
These special cases and considerations provide valuable insights into the calculation of the perimeter of triangle WXY and help ensure accurate results in various scenarios.
Applications in Real World Geometry
Understanding how to calculate the perimeter of triangle WXY has various applications in real-world geometry. Here are some examples:
- Construction: In construction, knowing the perimeter of a triangular area helps determine the amount of material needed for fencing or landscaping. It also aids in estimating the cost of the project.
- Architecture: Architects use perimeter calculations to design buildings with triangular shapes or incorporate triangular elements into their designs. Perimeter calculations ensure structural stability and efficient use of space.
- Navigation: In navigation and cartography, understanding triangle perimeters is essential for calculating distances between points on a map. This knowledge helps in planning routes and estimating travel times.
- Engineering: Engineers utilize perimeter calculations in various fields such as civil engineering, mechanical engineering, and aerospace engineering. For example, in civil engineering, perimeters are crucial for designing roadways, bridges, and pipelines.
- Geometry in Nature: Natural structures often exhibit triangular shapes, such as mountain peaks and pyramids. Understanding triangle perimeters enables scientists and researchers to analyze these formations and study natural phenomena.
These applications highlight the practical significance of knowing how to calculate the perimeter of triangle WXY and its relevance in diverse real-world scenarios.
READ MORE:
Xem video về cách tính diện tích và chu vi của tam giác trên mặt phẳng tọa độ. Hiểu cách tính chu vi của tam giác WXY trong bài viết của chúng tôi?
Diện tích và Chu vi của Tam giác trên Mặt phẳng Tọa độ