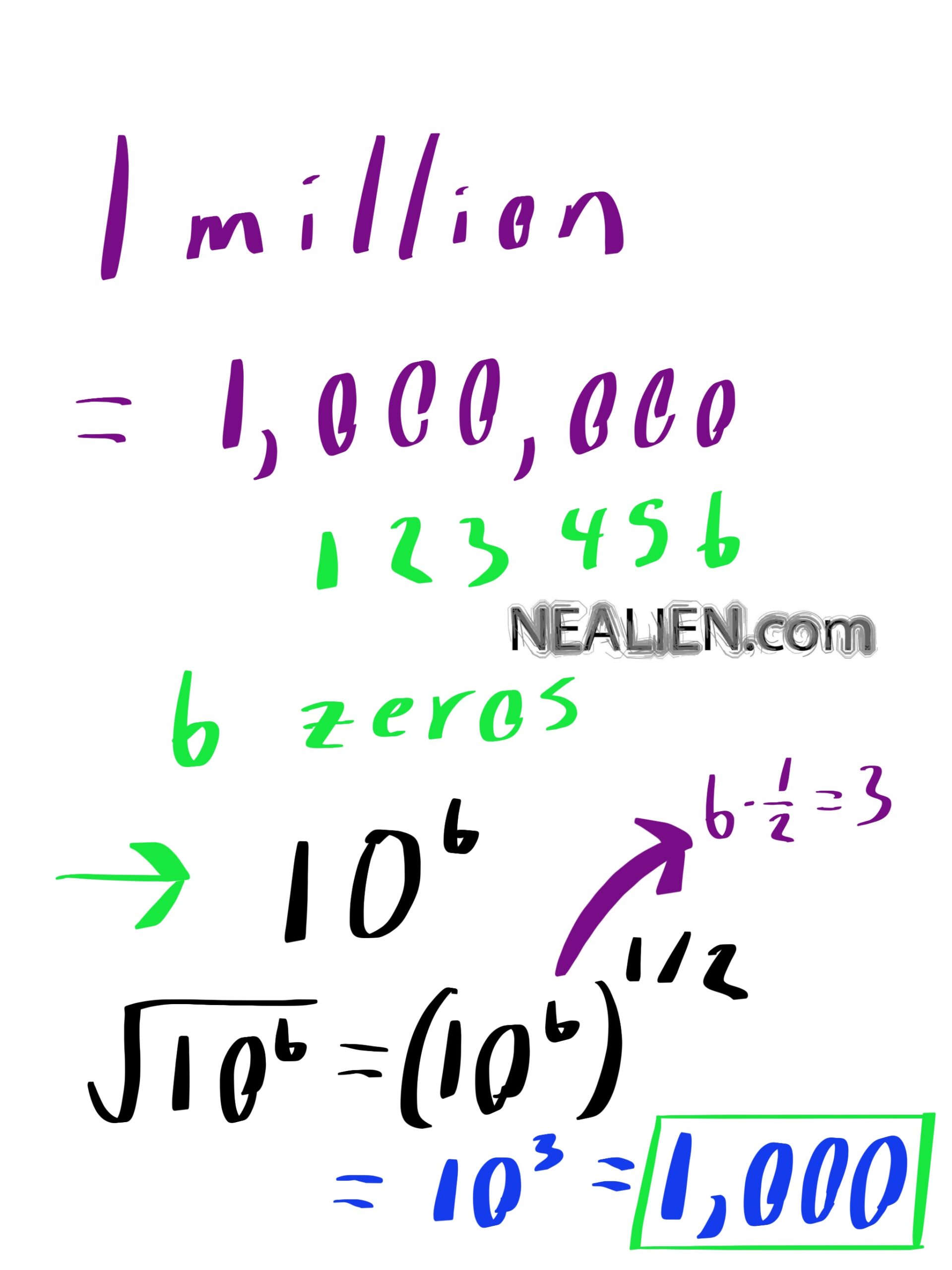Topic square root 1-1000: The concept of square roots is fundamental in mathematics, representing the value that, when multiplied by itself, gives the original number. In this article, we explore the square roots of numbers from 1 to 1000, providing a comprehensive guide to understanding and calculating these values. Whether you're a student or a math enthusiast, this guide will enhance your knowledge and problem-solving skills.
Table of Content
Square Root Table for Numbers 1 to 1000
The square root of a number is a value that, when multiplied by itself, gives the original number. This table provides the square roots of numbers from 1 to 1000, including both perfect squares and non-integer results.
Table of Square Roots from 1 to 1000
| Number | Square Root |
|---|---|
| 1 | \(\sqrt{1} = 1\) |
| 2 | \(\sqrt{2} \approx 1.414\) |
| 3 | \(\sqrt{3} \approx 1.732\) |
| 4 | \(\sqrt{4} = 2\) |
| 5 | \(\sqrt{5} \approx 2.236\) |
Understanding Square Roots
Square roots are essential in various mathematical applications, including algebra, geometry, and calculus. The principal square root of a non-negative number \(a\) is denoted by \(\sqrt{a}\), and it represents the non-negative root of \(a\). For example, \(\sqrt{25} = 5\), because \(5^2 = 25\).
Perfect Squares
A perfect square is an integer that is the square of another integer. For example, 1, 4, 9, 16, and 25 are perfect squares because they are \(1^2\), \(2^2\), \(3^2\), \(4^2\), and \(5^2\) respectively. Below is a list of the first few perfect squares:
- \(1^2 = 1\)
- \(2^2 = 4\)
- \(3^2 = 9\)
- \(4^2 = 16\)
Non-Perfect Squares
Non-perfect squares are numbers whose square roots are not integers. For example, the square root of 2 is approximately 1.414, which is an irrational number. These roots are often represented as decimal values for practical use. For example:
Using the Square Root Table
This table can be useful for quick reference in solving mathematical problems, performing calculations, or verifying results. Whether for educational purposes or practical applications, knowing the square roots of numbers can simplify many tasks.
Conclusion
Square roots are a fundamental concept in mathematics, and understanding them is crucial for various mathematical disciplines. This table serves as a comprehensive guide for the square roots of numbers from 1 to 1000.

READ MORE:
Introduction to Square Roots
A square root of a number is a value that, when multiplied by itself, yields the original number. It is an essential concept in mathematics with various applications in algebra, geometry, and real-world problem-solving. The symbol for square root is '√', also known as the radical sign, and the number under the radical sign is called the radicand. For example, in √25, 25 is the radicand, and the square root of 25 is 5 because 5 * 5 = 25.
Square roots can be determined through various methods such as factorization and long division. If a number is a perfect square, its square root is an integer. For non-perfect squares, the result is an irrational number. Understanding square roots involves recognizing perfect squares and applying methods to approximate roots for non-perfect squares.
- Definition: The square root of a number is a value that, when squared, returns the original number. Mathematically, if x is the square root of y, then x = √y or x² = y.
- Symbol: The square root symbol is '√', and the value under the symbol is the radicand.
- Properties: Square roots have properties that make them useful in simplifying expressions and solving equations. For instance, √(a * b) = √a * √b and √(a/b) = √a / √b.
- Perfect Squares: Numbers like 1, 4, 9, 16, and 25 are perfect squares because their square roots are integers (1, 2, 3, 4, and 5, respectively).
- Calculation Methods:
- Prime Factorization: Breaking down a number into its prime factors and pairing them to find the square root.
- Long Division Method: A manual method for finding square roots of larger numbers or decimals.
- Estimation Method: Approximating the square root by finding the nearest perfect squares.
Understanding square roots is fundamental for advancing in mathematics, particularly in topics such as quadratic equations, geometry, and higher-level algebra. Mastering the concept of square roots helps in solving various mathematical problems more efficiently and accurately.
Definition and Symbol of Square Roots
A square root of a number is a value that, when multiplied by itself, gives the original number. It is denoted by the symbol √. For example, the square root of 9 is 3, because 3 multiplied by itself equals 9.
Methods to Calculate Square Roots
There are several methods to calculate square roots:
- Prime Factorization Method: This method involves breaking down the number into its prime factors and then taking the square root of each factor.
- Long Division Method: In this method, the square root is calculated through a series of long division steps.
- Estimation Method: This method involves making an educated guess and refining it iteratively to get closer to the actual square root.
- Using a Calculator: Modern calculators have the capability to compute square roots directly, providing quick and accurate results.
Properties of Square Roots
The properties of square roots include:
- Non-Negativity: The square root of a non-negative real number is always a non-negative real number.
- Multiplication Property: The square root of a product is equal to the product of the square roots of the individual factors.
- Division Property: The square root of a quotient is equal to the quotient of the square roots of the numerator and denominator.
- Exponent Property: The square root of a number raised to an even power is equal to the number raised to half the exponent.

Perfect Squares and Their Roots
Perfect squares are numbers that are the result of squaring an integer. Here are some perfect squares and their square roots:
| Perfect Square | Square Root |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
Square Root Tables
Here are tables showing square roots from 1 to 1000:
| Number | Square Root |
| 1 | 1 |
| 2 | 1.414 |
Applications of Square Roots
Square roots have numerous applications in various fields, ranging from geometry and algebra to real-life problems. Understanding these applications can enhance our comprehension and problem-solving skills.
In Geometry
-
Calculating Distances: In coordinate geometry, the distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) is found using the distance formula:
\[
d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}
\] -
Area of a Circle: The radius of a circle can be derived if the area is known:
\[
r = \sqrt{\frac{A}{\pi}}
\] -
Pythagorean Theorem: For a right-angled triangle, the length of the hypotenuse can be calculated if the lengths of the other two sides are known:
\[
c = \sqrt{a^2 + b^2}
\]
In Algebra
-
Solving Quadratic Equations: The roots of a quadratic equation \(ax^2 + bx + c = 0\) are given by the quadratic formula:
\[
x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}
\] -
Simplifying Expressions: Square roots are used to simplify algebraic expressions and radical equations.
In Real-Life Problems
-
Construction and Architecture: Square roots are used in various calculations, such as determining the length of the diagonal of a square or rectangle.
-
Finance: Square roots are used in financial models to calculate standard deviation and to perform risk assessments.
-
Science and Engineering: Square roots are used in formulas for calculating phenomena such as sound intensity, gravitational force, and electrical resistance.
-
Medicine: Square roots are used in medical imaging techniques like MRI and CT scans to calculate areas and volumes of organs and tumors.
Interactive Examples
Engaging with interactive examples can help deepen understanding of square roots and their applications. Below are step-by-step examples that illustrate how to calculate and apply square roots in various contexts.
Example 1: Finding the Square Root of a Number
-
Problem: Find the square root of 144.
Solution:
- Step 1: Recognize that 144 is a perfect square.
- Step 2: Identify the number whose square is 144: \( 12^2 = 144 \).
- Step 3: Conclude that the square root of 144 is: \[ \sqrt{144} = 12 \]
Example 2: Using the Pythagorean Theorem
-
Problem: A right-angled triangle has legs of length 3 and 4 units. Find the length of the hypotenuse.
Solution:
- Step 1: Use the Pythagorean theorem formula: \[ c = \sqrt{a^2 + b^2} \]
- Step 2: Substitute the given values: \[ c = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} \]
- Step 3: Calculate the square root of 25: \[ \sqrt{25} = 5 \]
- Step 4: Conclude that the length of the hypotenuse is 5 units.
Example 3: Solving a Quadratic Equation
-
Problem: Solve the quadratic equation \(x^2 - 6x + 9 = 0\).
Solution:
- Step 1: Recognize that the equation can be factored: \[ (x - 3)^2 = 0 \]
- Step 2: Take the square root of both sides: \[ x - 3 = 0 \]
- Step 3: Solve for \(x\): \[ x = 3 \]
- Step 4: Conclude that the solution to the equation is \(x = 3\).
Example 4: Real-Life Application in Finance
-
Problem: Calculate the standard deviation of a set of data points: {2, 4, 4, 4, 5, 5, 7, 9}.
Solution:
- Step 1: Find the mean of the data set: \[ \text{Mean} = \frac{2 + 4 + 4 + 4 + 5 + 5 + 7 + 9}{8} = 5 \]
- Step 2: Calculate each data point's deviation from the mean, square it, and find the average of these squares: \[ \text{Variance} = \frac{(2-5)^2 + (4-5)^2 + (4-5)^2 + (4-5)^2 + (5-5)^2 + (5-5)^2 + (7-5)^2 + (9-5)^2}{8} = 4 \]
- Step 3: Take the square root of the variance to find the standard deviation: \[ \text{Standard Deviation} = \sqrt{4} = 2 \]
- Step 4: Conclude that the standard deviation of the data set is 2.

FAQs on Square Roots
-
Q: What is a square root?
A: A square root of a number \(x\) is a value \(y\) such that \(y^2 = x\). In other words, it is a number that, when multiplied by itself, gives the original number. For example, the square root of 25 is 5 because \(5 \times 5 = 25\).
-
Q: How do you calculate the square root of a number?
A: Square roots can be calculated using various methods:
- Prime Factorization: Factorize the number into its prime factors and pair them.
- Long Division: A manual method to find the square root digit by digit.
- Estimation: Guess and check the approximate value of the square root.
- Calculator: Use a scientific calculator to find the square root quickly.
Q: What is the square root of a negative number?
A: The square root of a negative number is not a real number. Instead, it is an imaginary number. The square root of \(-1\) is represented as \(i\), so the square root of any negative number \(-x\) can be written as \(i\sqrt{x}\).
-
Q: What are perfect squares?
A: Perfect squares are numbers that are the square of an integer. For example, 1, 4, 9, 16, and 25 are perfect squares because they can be written as \(1^2, 2^2, 3^2, 4^2,\) and \(5^2\) respectively.
-
Q: Why are square roots important?
A: Square roots are essential in various mathematical and real-world applications, such as solving quadratic equations, finding distances in geometry, calculating areas and volumes, and in many scientific and engineering formulas.
-
Q: Can all numbers have a square root?
A: All non-negative numbers have a real square root. Positive numbers have two real square roots (one positive and one negative), zero has one square root (zero), and negative numbers have no real square root, only imaginary ones.
-
Q: How do you simplify a square root?
A: To simplify a square root:
- Factor the number into its prime factors.
- Pair the prime factors.
- Bring each pair out of the square root.
- Multiply the factors outside the square root.
For example, to simplify \(\sqrt{72}\):
- Prime factorize 72: \(72 = 2^3 \times 3^2\).
- Pair the prime factors: \((2^2) \times (3^2) \times 2\).
- Bring the pairs out: \(2 \times 3 \times \sqrt{2}\).
- Simplify: \(6\sqrt{2}\).
Căn Bậc Hai của Số Chính Phương từ 1-1000
READ MORE:
Chetan Sharma - Căn Bậc Hai từ 1-1000