Topic how to simplify radical expressions step by step: Master the art of simplifying radical expressions with our detailed step-by-step guide. Learn essential techniques, avoid common mistakes, and enhance your understanding of radicals and radicands. Dive into practical examples, practice problems, and advanced methods to simplify even the most complex radicals effortlessly.
Table of Content
- How to Simplify Radical Expressions Step by Step
- Introduction to Radical Expressions
- Understanding Radicals and Radicands
- Basic Rules for Simplifying Radicals
- Identifying Perfect Square Factors
- Simplifying Radicals: Step by Step Process
- Simplifying Radicals with Coefficients
- Special Cases in Simplifying Radicals
- Simplifying Radicals with Variables
- Combining Like Radicals
- Rationalizing the Denominator
- Common Mistakes to Avoid
- Examples and Practice Problems
- Advanced Techniques for Complex Radicals
- FAQs on Simplifying Radical Expressions
- Conclusion and Summary
- YOUTUBE:
How to Simplify Radical Expressions Step by Step
Simplifying radical expressions involves breaking down the radicand (the number inside the radical) into its prime factors and then applying various mathematical rules to simplify the expression. Below are the steps to simplify different types of radical expressions.
Steps to Simplify Basic Radical Expressions
- Identify the largest perfect square factor of the radicand.
- Rewrite the radical expression as the product of the square root of this factor and another factor.
- Simplify the square root of the perfect square factor.
- Combine the simplified square root with the remaining radical.
For example:
\(\sqrt{45}\)
The largest perfect square factor of 45 is 9.
Rewrite the expression: \(\sqrt{45} = \sqrt{9 \times 5} = \sqrt{9} \times \sqrt{5}\)
Simplify: \(\sqrt{9} = 3\)
The simplified form: \(3\sqrt{5}\)
Steps to Simplify Radical Expressions with Variables
- For each variable, determine if the exponent is even or odd.
- If the exponent is even, take half of the exponent.
- If the exponent is odd, subtract one from the exponent to make it even, then proceed as above.
For example:
\(\sqrt{x^{10}}\)
Since 10 is even, take half of 10.
The simplified form: \(x^5\)
Example: Simplifying Radicals with Variables and Coefficients
- Identify the largest perfect square factor of the numerical part.
- Rewrite the expression, separating the numerical and variable parts.
- Simplify the numerical and variable parts separately.
- Combine the simplified parts.
For example:
\(\sqrt{50x^6y^3}\)
Rewrite as: \(\sqrt{25 \times 2 \times x^6 \times y^3} = \sqrt{25} \times \sqrt{2} \times \sqrt{x^6} \times \sqrt{y^3}\)
Simplify: \(5 \times \sqrt{2} \times x^3 \times y^{1.5} = 5x^3y\sqrt{2y}\)
Rules for Simplifying Radical Expressions
- \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}, \; b \neq 0\)
- \(\sqrt{a} + \sqrt{b} \neq \sqrt{a + b}\)
- \(\sqrt{a} - \sqrt{b} \neq \sqrt{a - b}\)
These rules help in simplifying complex radical expressions by breaking them into smaller, more manageable parts.
Tips and Tricks
- When dealing with radicals in the denominator, multiply by the conjugate to simplify.
- Always express the square root of a number as non-negative by using absolute values.
By following these steps and rules, you can simplify various radical expressions effectively.

READ MORE:
Introduction to Radical Expressions
Radical expressions, which involve roots such as square roots or cube roots, are fundamental in various mathematical contexts. Simplifying these expressions is a crucial skill that helps in solving equations more efficiently. In this section, we will explore the basics of radical expressions, their components, and the general approach to simplifying them.
A radical expression consists of a radicand (the number under the radical sign) and an index (the degree of the root). For example, in the expression , 16 is the radicand, and the square root symbol (√) implies an index of 2.
The process of simplifying radical expressions generally involves the following steps:
- Identify Perfect Square Factors: Find the largest perfect square factor of the radicand. For instance, in , the largest perfect square factor is 36.
- Rewrite the Radicand: Express the radicand as a product of its perfect square factor and another factor. For example, .
- Simplify the Expression: Simplify the radical by taking the square root of the perfect square factor. In our example, .
Using these steps, any radical expression can be simplified systematically. Mastering this process enhances problem-solving skills and mathematical fluency.
Understanding Radicals and Radicands
Radical expressions are expressions that contain a square root, cube root, or any higher-order root. The radical symbol (√) is used to indicate these roots, and the number or expression inside the radical symbol is called the radicand. Understanding radicals and radicands is essential for simplifying radical expressions effectively.
- Radical: The symbol √ used to denote roots.
- Radicand: The number or expression inside the radical symbol.
For example, in the expression √25, 25 is the radicand, and the radical symbol indicates the square root.
When simplifying radical expressions, it's crucial to identify perfect square factors of the radicand. This process involves breaking down the radicand into its prime factors and grouping them into pairs.
- Identify the prime factors: Break down the radicand into its prime factors. For instance, 72 can be broken down into 2 x 2 x 2 x 3 x 3.
- Group the prime factors: Group the prime factors into pairs. In this case, (2 x 2) and (3 x 3) can be paired, leaving one 2 unpaired.
- Simplify the pairs: Take the square root of each pair and multiply them together. For the example of √72, we get 6√2 because (2 x 2) gives 2, and (3 x 3) gives 3, and 2 x 3 is 6.
This step-by-step process simplifies the radical expression to its simplest form, making it easier to work with in further calculations.
In summary, understanding the roles of radicals and radicands and learning to identify perfect square factors are the foundation of simplifying radical expressions.
Basic Rules for Simplifying Radicals
When simplifying radicals, certain rules and steps should be followed to ensure the expression is in its simplest form. Here are the basic rules for simplifying radicals:
- Find the largest perfect square factor of the radicand.
- Rewrite the radical as a product of the square root of the perfect square and the square root of the remaining factor.
- Simplify the square roots.
Let's explore each step in detail:
Step 1: Find the largest perfect square factor
The largest perfect square factor is the highest perfect square that can divide the radicand without leaving a remainder. For example, to simplify \( \sqrt{72} \), identify 36 as the largest perfect square factor because \( 72 = 36 \times 2 \).
Step 2: Rewrite the radical
Express the original radical as the product of the square root of the perfect square and the square root of the remaining factor. For \( \sqrt{72} \), rewrite it as:
$$ \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} $$
Step 3: Simplify the square roots
Simplify the square root of the perfect square and multiply it by the square root of the remaining factor. For \( \sqrt{72} \), simplify it as:
$$ \sqrt{36} \times \sqrt{2} = 6 \sqrt{2} $$
Here are a few more examples:
- Simplify \( \sqrt{50} \):
$$ \sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5 \sqrt{2} $$ - Simplify \( \sqrt{200} \):
$$ \sqrt{200} = \sqrt{100 \times 2} = \sqrt{100} \times \sqrt{2} = 10 \sqrt{2} $$ - Simplify \( \sqrt{32} \):
$$ \sqrt{32} = \sqrt{16 \times 2} = \sqrt{16} \times \sqrt{2} = 4 \sqrt{2} $$
Following these basic rules will help you simplify radicals efficiently and accurately.
Identifying Perfect Square Factors
Identifying perfect square factors is a crucial step in simplifying radical expressions. Perfect square factors are numbers that can be expressed as the square of an integer. For instance, \( 1, 4, 9, 16, 25, \) and \( 36 \) are all perfect squares. When simplifying a radical expression, you need to find the largest perfect square factor of the radicand.
Here’s a step-by-step process to identify perfect square factors:
- List all the factors of the number inside the radical (the radicand).
- Identify the factors that are perfect squares.
- Choose the largest perfect square factor from the list.
Let's apply this process to a few examples:
| Example | Steps | Result |
|---|---|---|
| \(\sqrt{50}\) |
|
\(\sqrt{50} = 5\sqrt{2}\) |
| \(\sqrt{72}\) |
|
\(\sqrt{72} = 6\sqrt{2}\) |
By following these steps, you can simplify any radical expression by identifying and using perfect square factors.
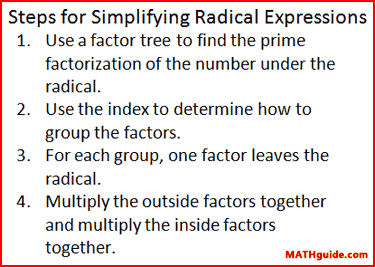
Simplifying Radicals: Step by Step Process
Simplifying radicals involves a systematic approach to ensure the expression is in its simplest form. Here is a detailed step-by-step process:
-
Identify Perfect Square Factors:
Determine the largest perfect square factor of the number under the radical. For instance, to simplify \(\sqrt{72}\), notice that 36 (a perfect square) is a factor of 72.
- 72 = 36 × 2
-
Rewrite the Radical:
Express the radicand (number under the radical) as a product of its perfect square factor and another number.
- \(\sqrt{72} = \sqrt{36 \cdot 2}\)
-
Apply the Product Rule:
Use the product rule of square roots: \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\).
- \(\sqrt{36 \cdot 2} = \sqrt{36} \cdot \sqrt{2}\)
-
Simplify the Radical:
Find the square root of the perfect square factor.
- \(\sqrt{36} = 6\)
-
Combine and Simplify:
Multiply the simplified radical by any remaining radicals.
- \(6 \cdot \sqrt{2} = 6\sqrt{2}\)
Thus, \(\sqrt{72}\) simplifies to \(6\sqrt{2}\). This process can be applied to any radical expression to simplify it effectively.
Simplifying Radicals with Coefficients
When simplifying radicals that include coefficients, the process involves simplifying the radical part first and then dealing with the coefficient. Here's a detailed, step-by-step guide on how to do this:
-
Identify the largest perfect square factor:
Find the largest perfect square that is a factor of the radicand (the number inside the radical). This makes the simplification process easier.
Example: Simplify \(3\sqrt{72}\)
The largest perfect square factor of 72 is 36 (since \(36 \times 2 = 72\)).
-
Rewrite the radical as a product of square roots:
Express the radicand as a product of the largest perfect square factor and its co-factor.
Example: \(3\sqrt{72} = 3\sqrt{36 \times 2}\)
-
Simplify the radical:
Simplify the square root of the perfect square factor.
Example: \(3\sqrt{36 \times 2} = 3 \times \sqrt{36} \times \sqrt{2} = 3 \times 6 \times \sqrt{2}\)
-
Multiply the coefficient by the simplified radical:
Combine the coefficient with the simplified radical.
Example: \(3 \times 6 \times \sqrt{2} = 18\sqrt{2}\)
Thus, \(3\sqrt{72}\) simplifies to \(18\sqrt{2}\).
Here are a few more examples to illustrate the process:
-
Example 1: Simplify \(5\sqrt{50}\)
Step 1: Identify the largest perfect square factor of 50, which is 25 (since \(25 \times 2 = 50\)).
Step 2: Rewrite the radical: \(5\sqrt{50} = 5\sqrt{25 \times 2}\)
Step 3: Simplify the radical: \(5\sqrt{25 \times 2} = 5 \times \sqrt{25} \times \sqrt{2} = 5 \times 5 \times \sqrt{2}\)
Step 4: Multiply the coefficient: \(5 \times 5 \times \sqrt{2} = 25\sqrt{2}\)
Final answer: \(25\sqrt{2}\)
-
Example 2: Simplify \(2\sqrt{128}\)
Step 1: Identify the largest perfect square factor of 128, which is 64 (since \(64 \times 2 = 128\)).
Step 2: Rewrite the radical: \(2\sqrt{128} = 2\sqrt{64 \times 2}\)
Step 3: Simplify the radical: \(2\sqrt{64 \times 2} = 2 \times \sqrt{64} \times \sqrt{2} = 2 \times 8 \times \sqrt{2}\)
Step 4: Multiply the coefficient: \(2 \times 8 \times \sqrt{2} = 16\sqrt{2}\)
Final answer: \(16\sqrt{2}\)
-
Example 3: Simplify \(7\sqrt{18}\)
Step 1: Identify the largest perfect square factor of 18, which is 9 (since \(9 \times 2 = 18\)).
Step 2: Rewrite the radical: \(7\sqrt{18} = 7\sqrt{9 \times 2}\)
Step 3: Simplify the radical: \(7\sqrt{9 \times 2} = 7 \times \sqrt{9} \times \sqrt{2} = 7 \times 3 \times \sqrt{2}\)
Step 4: Multiply the coefficient: \(7 \times 3 \times \sqrt{2} = 21\sqrt{2}\)
Final answer: \(21\sqrt{2}\)
By following these steps, you can simplify any radical expression with coefficients efficiently.
Special Cases in Simplifying Radicals
In this section, we will discuss some special cases encountered while simplifying radicals. These cases often involve scenarios that differ from the standard simplification process. Understanding these will help in handling a variety of radical expressions effectively.
1. Radicals with Fractions
When simplifying radicals that involve fractions, it's essential to address both the numerator and the denominator separately. Here are the steps:
- Simplify the numerator and the denominator separately.
- Combine the simplified results.
- Rationalize the denominator if necessary.
For example, consider the expression \( \sqrt{\frac{4}{9}} \):
\[
\sqrt{\frac{4}{9}} = \frac{\sqrt{4}}{\sqrt{9}} = \frac{2}{3}
\]
2. Radicals with Negative Numbers
When dealing with negative radicands, the concept of imaginary numbers comes into play. The square root of a negative number is expressed using the imaginary unit \( i \), where \( i = \sqrt{-1} \).
For example:
\[
\sqrt{-16} = 4i
\]
3. Radicals Involving Variables with Exponents
To simplify radicals that involve variables with exponents, the process depends on whether the exponent is even or odd:
- If the exponent is even, take half of the exponent.
- If the exponent is odd, reduce the exponent by one (making it even), and factor out the variable.
Examples:
\[
\sqrt{x^6} = x^3
\]
\[
\sqrt{y^5} = \sqrt{y^4 \cdot y} = y^2 \sqrt{y}
\]
4. Radicals with Higher-Order Roots
Sometimes, you might need to simplify radicals involving cube roots or other higher-order roots. The process is similar but involves factoring the radicand into cubes or other appropriate powers.
For example:
\[
\sqrt[3]{54} = \sqrt[3]{27 \cdot 2} = \sqrt[3]{27} \cdot \sqrt[3]{2} = 3 \sqrt[3]{2}
\]
5. Combining Radicals
When combining like radicals, only radicals with the same radicand can be combined. This is similar to combining like terms in algebra.
For example:
\[
2\sqrt{3} + 5\sqrt{3} = 7\sqrt{3}
\]
But \( 2\sqrt{3} + 4\sqrt{5} \) cannot be combined because the radicands are different.
6. Rationalizing the Denominator
To rationalize a denominator containing a radical, multiply both the numerator and the denominator by a radical that will eliminate the radical in the denominator.
For example:
\[
\frac{3}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{3\sqrt{2}}{2}
\]
By understanding these special cases, you can handle a broader range of radical expressions with confidence and precision.
Simplifying Radicals with Variables
When simplifying radicals that include variables, the process is similar to simplifying numerical radicals, but you must also consider the exponents of the variables. Here is a step-by-step guide:
-
Identify Perfect Square Factors:
Start by identifying perfect square factors in the radicand, both for the numerical part and the variables.
Example: \(\sqrt{50x^4y^3}\)
-
Rewrite the Radicand:
Rewrite the radicand as the product of its perfect square factors and remaining factors.
\(\sqrt{50x^4y^3} = \sqrt{25 \cdot 2 \cdot x^4 \cdot y^2 \cdot y}\)
-
Simplify the Radicals:
Simplify the square roots of the perfect square factors.
\(\sqrt{25} = 5\)
\(\sqrt{x^4} = x^2\)
\(\sqrt{y^2} = y\)
The expression now becomes: \(5x^2y\sqrt{2y}\)
-
Combine and Simplify:
Combine the simplified parts outside the radical and leave the remaining factors inside.
Final answer: \(5x^2y\sqrt{2y}\)
Here are more examples to illustrate the process:
-
Example 1: Simplify \(\sqrt{72x^5}\)
- Identify perfect square factors: \(\sqrt{36 \cdot 2 \cdot x^4 \cdot x}\)
- Simplify the square roots: \(\sqrt{36} = 6\), \(\sqrt{x^4} = x^2\)
- Combine: \(6x^2\sqrt{2x}\)
Final answer: \(6x^2\sqrt{2x}\)
-
Example 2: Simplify \(\sqrt{18x^2y^3}\)
- Identify perfect square factors: \(\sqrt{9 \cdot 2 \cdot x^2 \cdot y^2 \cdot y}\)
- Simplify the square roots: \(\sqrt{9} = 3\), \(\sqrt{x^2} = x\), \(\sqrt{y^2} = y\)
- Combine: \(3xy\sqrt{2y}\)
Final answer: \(3xy\sqrt{2y}\)

Combining Like Radicals
Combining like radicals is similar to combining like terms in algebra. The key is that the radicals must have the same radicand (the expression inside the radical). Here are the steps to combine like radicals:
- Identify Like Radicals:
Like radicals have the same index and the same radicand. For example, \( \sqrt{3} \) and \( 2\sqrt{3} \) are like radicals, but \( \sqrt{3} \) and \( \sqrt{5} \) are not.
- Simplify Each Radical:
If possible, simplify the radicals before combining them. For example, \( \sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2} \).
- Combine Coefficients of Like Radicals:
Add or subtract the coefficients of the like radicals just as you would with like terms in algebra. For example:
- \(3\sqrt{2} + 4\sqrt{2} = (3 + 4)\sqrt{2} = 7\sqrt{2}\)
- \(5\sqrt{3} - 2\sqrt{3} = (5 - 2)\sqrt{3} = 3\sqrt{3}\)
Here are some examples to illustrate the process:
| Expression | Steps | Result |
|---|---|---|
| \(2\sqrt{5} + 3\sqrt{5}\) | \((2 + 3)\sqrt{5}\) | \(5\sqrt{5}\) |
| \(7\sqrt{6} - 4\sqrt{6}\) | \((7 - 4)\sqrt{6}\) | \(3\sqrt{6}\) |
| \(2\sqrt{3} + 5\sqrt{3} - \sqrt{3}\) | \((2 + 5 - 1)\sqrt{3}\) | \(6\sqrt{3}\) |
If the radicals are not like terms, they cannot be combined. For instance, \( \sqrt{2} \) and \( \sqrt{3} \) remain separate in any expression because their radicands are different.
Combining like radicals is an essential skill for simplifying radical expressions, and it helps in solving more complex algebraic problems involving radicals.
Rationalizing the Denominator
Rationalizing the denominator involves eliminating any radicals present in the denominator of a fraction. This process often requires multiplying both the numerator and the denominator by a suitable radical that will cancel out the radical in the denominator. Here are the steps to rationalize the denominator:
Step-by-Step Process
-
Identify the radical in the denominator:
Given a fraction like \(\frac{a}{\sqrt{b}}\), where \(\sqrt{b}\) is the radical in the denominator.
-
Multiply by a form of 1:
To eliminate the radical, multiply the fraction by \(\frac{\sqrt{b}}{\sqrt{b}}\), which is equivalent to 1 and does not change the value of the fraction:
\[
\frac{a}{\sqrt{b}} \times \frac{\sqrt{b}}{\sqrt{b}} = \frac{a \cdot \sqrt{b}}{b}
\] -
Simplify the fraction:
The denominator is now rationalized, and the fraction becomes \(\frac{a \cdot \sqrt{b}}{b}\).
Examples
-
Example 1:
Simplify \(\frac{5}{\sqrt{2}}\).
- Identify the radical in the denominator: \(\sqrt{2}\).
- Multiply by \(\frac{\sqrt{2}}{\sqrt{2}}\): \[ \frac{5}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{5\sqrt{2}}{2} \]
- Simplify the fraction: \(\frac{5\sqrt{2}}{2}\).
-
Example 2:
Simplify \(\frac{3}{\sqrt{5}}\).
- Identify the radical in the denominator: \(\sqrt{5}\).
- Multiply by \(\frac{\sqrt{5}}{\sqrt{5}}\): \[ \frac{3}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} = \frac{3\sqrt{5}}{5} \]
- Simplify the fraction: \(\frac{3\sqrt{5}}{5}\).
Rationalizing Binomial Denominators
When the denominator is a binomial involving radicals, such as \(\frac{1}{a + \sqrt{b}}\), rationalizing involves using the conjugate of the denominator.
-
Multiply by the conjugate:
The conjugate of \(a + \sqrt{b}\) is \(a - \sqrt{b}\). Multiply both the numerator and the denominator by this conjugate:
\[
\frac{1}{a + \sqrt{b}} \times \frac{a - \sqrt{b}}{a - \sqrt{b}} = \frac{a - \sqrt{b}}{a^2 - b}
\] -
Simplify the result:
The product of the binomials in the denominator results in the difference of squares:
\[
\frac{a - \sqrt{b}}{a^2 - b}
\]
Examples
-
Example 1:
Simplify \(\frac{2}{3 + \sqrt{7}}\).
- Identify the conjugate: \(3 - \sqrt{7}\).
- Multiply by \(\frac{3 - \sqrt{7}}{3 - \sqrt{7}}\): \[ \frac{2}{3 + \sqrt{7}} \times \frac{3 - \sqrt{7}}{3 - \sqrt{7}} = \frac{2(3 - \sqrt{7})}{(3)^2 - (\sqrt{7})^2} \]
- Simplify the fraction: \[ \frac{6 - 2\sqrt{7}}{9 - 7} = \frac{6 - 2\sqrt{7}}{2} = 3 - \sqrt{7} \]
By following these steps, you can effectively rationalize denominators, making your expressions simpler and easier to work with.
Common Mistakes to Avoid
Simplifying radical expressions can be tricky, and certain common mistakes often occur. Here are some key errors to avoid to ensure accuracy in your calculations:
- Forgetting to Simplify Completely: Ensure all radicals are simplified as much as possible. For example,
\(\sqrt{50}\) should be simplified to \(5\sqrt{2}\), not left as \(\sqrt{50}\). - Incorrectly Combining Radicals: Only like radicals can be combined. For instance, \(2\sqrt{3}\) and \(3\sqrt{3}\) can be combined to \(5\sqrt{3}\), but \(2\sqrt{2}\) and \(3\sqrt{3}\) cannot be combined.
- Ignoring the Product Rule: The product rule states that
\(\sqrt{a} \times \sqrt{b} = \sqrt{ab}\) . Forgetting this can lead to incorrect simplification. Always check for this rule when simplifying expressions. - Misapplying the Quotient Rule: Similar to the product rule, the quotient rule states that
\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\) . Ensure to apply this rule correctly to avoid mistakes. - Not Rationalizing the Denominator: Leaving a radical in the denominator is generally considered improper form. Always rationalize the denominator, transforming
\(\frac{1}{\sqrt{2}}\) into\(\frac{\sqrt{2}}{2}\) . - Overlooking Variable Simplifications: When dealing with variables, simplify them correctly. For example,
\(\sqrt{x^4} = x^2\) . Ensure all variable exponents are simplified. - Incorrect Distribution: When distributing radicals over addition or subtraction, remember
\(\sqrt{a+b} \neq \sqrt{a} + \sqrt{b}\) . This is a common misconception. Always handle distribution with care.
By being mindful of these common mistakes and double-checking your work, you can simplify radical expressions accurately and efficiently.
Examples and Practice Problems
Here are some examples and practice problems to help you master the process of simplifying radical expressions:
Example 1: Simplifying \( \sqrt{72} \)
- Identify the largest perfect square factor of 72, which is 36.
- Rewrite the radical as \( \sqrt{36 \times 2} \).
- Apply the product rule of square roots: \( \sqrt{36} \times \sqrt{2} \).
- Simplify: \( 6\sqrt{2} \).
Example 2: Simplifying \( \sqrt{48} \)
- Identify the largest perfect square factor of 48, which is 16.
- Rewrite the radical as \( \sqrt{16 \times 3} \).
- Apply the product rule of square roots: \( \sqrt{16} \times \sqrt{3} \).
- Simplify: \( 4\sqrt{3} \).
Example 3: Simplifying \( \sqrt{200} \)
- Identify the largest perfect square factor of 200, which is 100.
- Rewrite the radical as \( \sqrt{100 \times 2} \).
- Apply the product rule of square roots: \( \sqrt{100} \times \sqrt{2} \).
- Simplify: \( 10\sqrt{2} \).
Example 4: Simplifying \( \sqrt{180} \)
- Identify the largest perfect square factor of 180, which is 36.
- Rewrite the radical as \( \sqrt{36 \times 5} \).
- Apply the product rule of square roots: \( \sqrt{36} \times \sqrt{5} \).
- Simplify: \( 6\sqrt{5} \).
Practice Problems
- Simplify \( \sqrt{50} \).
- Simplify \( \sqrt{98} \).
- Simplify \( \sqrt{32} \).
- Simplify \( \sqrt{125} \).
- Simplify \( \sqrt{75} \).
To solve these practice problems, follow the steps outlined in the examples above. Identify the largest perfect square factor, rewrite the radical expression, apply the product rule, and simplify.
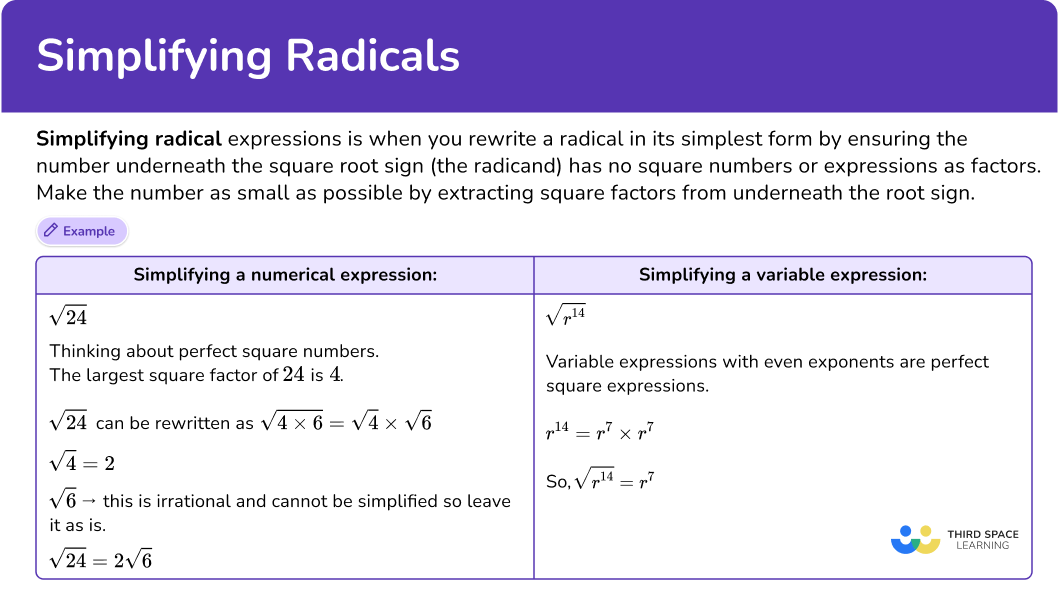
Advanced Techniques for Complex Radicals
When simplifying complex radicals, there are several advanced techniques that can be employed to make the process more efficient and accurate. Below are some of these techniques explained in detail:
- Prime Factorization: Break down the radicand into its prime factors to simplify the expression. This is particularly useful for large numbers or when dealing with variables.
For example, simplify \( \sqrt{180} \):
- Find the prime factors: \( 180 = 2 \times 2 \times 3 \times 3 \times 5 \)
- Group the prime factors into pairs: \( \sqrt{180} = \sqrt{(2 \times 2) \times (3 \times 3) \times 5} \)
- Simplify each pair: \( \sqrt{(2^2) \times (3^2) \times 5} = 2 \times 3 \times \sqrt{5} = 6\sqrt{5} \)
- Rationalizing the Denominator: Ensure that no radicals remain in the denominator of a fraction by multiplying both the numerator and the denominator by a suitable radical.
For example, rationalize \( \frac{1}{\sqrt{3}} \):
- Multiply the numerator and the denominator by \( \sqrt{3} \): \( \frac{1}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{3}}{3} \)
- Using Conjugates: For expressions with binomial denominators, multiply by the conjugate to eliminate radicals.
For example, simplify \( \frac{1}{\sqrt{3} + \sqrt{2}} \):
- Multiply the numerator and the denominator by the conjugate: \( \frac{1}{\sqrt{3} + \sqrt{2}} \times \frac{\sqrt{3} - \sqrt{2}}{\sqrt{3} - \sqrt{2}} = \frac{\sqrt{3} - \sqrt{2}}{(\sqrt{3})^2 - (\sqrt{2})^2} = \frac{\sqrt{3} - \sqrt{2}}{3 - 2} = \sqrt{3} - \sqrt{2} \)
- Exponent Rules: Apply rules of exponents to simplify radicals involving higher powers.
For example, simplify \( \sqrt[3]{x^9} \):
- Use the property \( \sqrt[n]{a^n} = a \): \( \sqrt[3]{x^9} = (x^9)^{1/3} = x^{9 \cdot 1/3} = x^3 \)
- Combining Techniques: Often, simplifying complex radicals involves a combination of the above techniques.
For example, simplify \( \sqrt{50x^4y^6} \):
- Prime factorization: \( 50 = 2 \times 5^2 \)
- Rewrite the radicand: \( \sqrt{50x^4y^6} = \sqrt{2 \times 5^2 \times x^4 \times y^6} \)
- Simplify each part: \( \sqrt{2} \times \sqrt{5^2} \times \sqrt{x^4} \times \sqrt{y^6} = \sqrt{2} \times 5 \times x^2 \times y^3 = 5x^2y^3\sqrt{2} \)
FAQs on Simplifying Radical Expressions
-
What are radical expressions?
Radical expressions are expressions that contain a square root, cube root, or any higher-order root.
-
How do you simplify a basic radical expression?
To simplify a basic radical expression, find the largest perfect square factor of the radicand (the number inside the radical). Then, rewrite the radicand as the product of this factor and another number. Finally, take the square root of the perfect square factor and move it outside the radical.
-
What are the steps to simplify radicals with coefficients?
- Identify the largest perfect square factor of the radicand.
- Rewrite the radicand as the product of this factor and another number.
- Simplify the radical by taking the square root of the perfect square factor.
- Multiply the coefficient by any number that comes out of the square root.
-
What does it mean to rationalize the denominator?
Rationalizing the denominator means to eliminate any radicals in the denominator of a fraction. This is done by multiplying both the numerator and the denominator by a suitable radical that will make the denominator a rational number.
-
Can you combine like radicals? If so, how?
Yes, you can combine like radicals. Like radicals are radicals that have the same radicand. To combine them, add or subtract the coefficients while keeping the radicand the same.
-
How do you simplify radicals with variables?
- Factor the variables inside the radical as much as possible.
- Apply the same principles used for simplifying numerical radicals: look for pairs of identical factors.
- Move pairs of variables outside the radical as a single instance of the variable.
- Keep any remaining variables inside the radical.
-
What are some common mistakes to avoid when simplifying radicals?
- Not fully factoring the radicand.
- Forgetting to simplify both the numerical and variable parts of the radicand.
- Incorrectly combining or simplifying unlike radicals.
- Failing to rationalize the denominator properly.
-
How can you simplify complex radical expressions?
Simplify complex radical expressions by breaking them down into smaller, more manageable parts. Use factorization, apply the rules of exponents and radicals, and combine like terms where possible.
Conclusion and Summary
Simplifying radical expressions is a fundamental skill in algebra that requires understanding the properties of radicals and applying systematic steps. This guide has provided a comprehensive approach to mastering this skill.
To summarize:
- Understanding Radicals: Radicals, also known as roots, are expressions that represent the root of a number or variable. The radicand is the number or expression inside the radical sign.
- Basic Simplification Rules:
- \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
- \(\sqrt{a} + \sqrt{b} \neq \sqrt{a + b}\)
- Identifying Perfect Squares: Break down the radicand into its prime factors and identify pairs of factors, which can be simplified out of the radical.
- Simplifying Step-by-Step: Write the radicand as a product of perfect squares and other factors, then simplify each part separately before combining them.
- Handling Coefficients: When coefficients are present, simplify the radical part first, then multiply by the coefficient.
- Special Cases: Handle special cases such as radicals with variables and rationalizing denominators using specific algebraic techniques.
- Common Mistakes: Avoid errors such as incorrectly combining radicals and misapplying the rules of radicals.
By following these guidelines, students can confidently approach and simplify any radical expression. Practice is essential, and working through various examples will help solidify these concepts.
Continue practicing with more complex radicals and explore advanced techniques to further enhance your skills. With persistence and careful application of the steps outlined in this guide, simplifying radical expressions will become a straightforward and manageable task.
We hope this comprehensive guide has been helpful in simplifying radical expressions. Remember, mathematics is a skill that improves with practice, so keep working on problems and challenging yourself with new exercises.
Thank you for following along, and happy simplifying!
Phương Pháp Đơn Giản Hóa Biểu Thức Căn Bậc
READ MORE:
Đơn Giản Hóa Biểu Thức Căn Bậc Với Biến Số