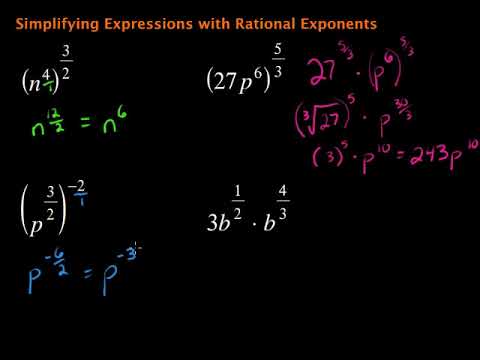Topic how do you simplify the radical expression: Understanding how to simplify radical expressions is essential for mastering algebra. This guide will take you through the basics, properties, and advanced techniques needed to simplify any radical expression effectively. Whether you are a student or just looking to brush up on your math skills, this article will provide clear and practical steps for simplification.
Table of Content
- Simplifying Radical Expressions
- Introduction to Radical Expressions
- Basic Concepts of Simplifying Radicals
- Properties and Rules of Radical Expressions
- Prime Factorization Method
- Simplifying Radicals with Coefficients
- Simplifying Radicals with Variables
- Using the Product Property to Simplify Radicals
- Using the Quotient Property to Simplify Radicals
- Combining Like Radicals
- Rationalizing the Denominator
- Special Cases and Common Mistakes
- Practice Problems and Solutions
- Advanced Techniques for Complex Expressions
- Applications of Simplified Radicals in Real-World Problems
- Conclusion and Key Takeaways
- YOUTUBE:
Simplifying Radical Expressions
Simplifying radical expressions involves breaking down the expression into its simplest form. Below are detailed steps and rules to help you simplify various types of radical expressions.
Steps to Simplify Radical Expressions
-
Prime Factorization: Write the number under the radical as a product of its prime factors.
For example, \(\sqrt{72} = \sqrt{2 \times 2 \times 2 \times 3 \times 3}\).
-
Group Factors: Combine the factors in pairs of identical factors.
Continuing the example, \(\sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2} = \sqrt{4 \times 9 \times 2}\).
-
Simplify: Take the square root of the paired factors and multiply them outside the radical.
\(\sqrt{72} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\).
Rules for Simplifying Radical Expressions
- \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}, \, b \ne 0\)
- \(\sqrt{a} + \sqrt{b} \ne \sqrt{a + b}\)
- \(\sqrt{a} - \sqrt{b} \ne \sqrt{a - b}\)
Examples of Simplifying Radical Expressions
Example 1: Simplifying \(\sqrt{45}\)
Find the largest perfect square factor of 45. \(45 = 9 \times 5\).
\(\sqrt{45} = \sqrt{9 \times 5} = \sqrt{9} \times \sqrt{5} = 3\sqrt{5}\).
Example 2: Simplifying \(\sqrt{80}\)
Find the largest perfect square factor of 80. \(80 = 16 \times 5\).
\(\sqrt{80} = \sqrt{16 \times 5} = \sqrt{16} \times \sqrt{5} = 4\sqrt{5}\).
Example 3: Simplifying \(\sqrt{x^{10}}\)
Since the exponent is even, divide the exponent by 2.
\(\sqrt{x^{10}} = x^{5}\).
Example 4: Simplifying \(\sqrt{a^2 b^4}\)
Find the largest perfect square factor of each term.
\(\sqrt{a^2 b^4} = a b^2\).
Additional Tips and Tricks
- For radicals in the denominator, multiply the numerator and the denominator by the conjugate of the denominator to simplify.
- Remember that the square root of a negative number is not a real number, so always ensure the radicand (the number under the radical) is non-negative.
Example Problems
- Simplify \(\frac{\sqrt{12}}{\sqrt{5}}\)
- Simplify \(\frac{10b^2c^2}{c\sqrt{4b^3}}\)
Solution: \(\frac{\sqrt{12}}{\sqrt{5}} = \frac{\sqrt{12 \times 5}}{\sqrt{5 \times 5}} = \frac{\sqrt{60}}{5} = \frac{2\sqrt{15}}{5} = \frac{2}{5}\sqrt{15}\).
Solution: \(\frac{10b^2c^2}{c\sqrt{4b^3}} = \frac{10b^2c^2}{2bc\sqrt{b}} = \frac{5bc^2}{\sqrt{b}} = \frac{5bc\sqrt{b}}{b} = 5c\sqrt{b}\).

READ MORE:
Introduction to Radical Expressions
Radical expressions, often involving roots such as square roots or cube roots, are a fundamental concept in algebra. Understanding how to simplify these expressions is crucial for solving various mathematical problems efficiently. Let's delve into the basics of radical expressions and how to approach their simplification.
Radical expressions include a radicand (the number or expression inside the radical symbol) and an index (which denotes the degree of the root, such as 2 for square roots). The general form is √a, where a is the radicand.
Why Simplify Radical Expressions?
- To make calculations easier and more manageable.
- To compare and combine radical expressions more effectively.
- To prepare expressions for further algebraic operations.
Steps to Simplify Radical Expressions
Here is a step-by-step guide to simplifying a basic radical expression:
- Identify and extract perfect square factors from the radicand. For example, in √72, the perfect square factor is 36.
- Rewrite the radicand as a product of its factors. Using the example √72: √(36 * 2).
- Apply the product rule of square roots: √(a * b) = √a * √b. So, √(36 * 2) = √36 * √2.
- Simplify the expression by taking the square root of the perfect square. In this case, √36 = 6, so √72 = 6√2.
Key Properties of Radicals
Understanding these properties helps in simplifying more complex expressions:
- Product Property: √(ab) = √a * √b
- Quotient Property: √(a/b) = √a / √b, provided b ≠ 0
- Sum/Difference Property: √a + √b ≠ √(a + b) and √a - √b ≠ √(a - b)
Examples
Let's go through a few examples to illustrate these steps:
- Simplify √50: Since 50 = 25 * 2, we can write √50 = √(25 * 2) = √25 * √2 = 5√2.
- Simplify √18: Since 18 = 9 * 2, we can write √18 = √(9 * 2) = √9 * √2 = 3√2.
Practice Problems
Try simplifying the following radical expressions:
- √45
- √80
- √98
With practice, simplifying radical expressions becomes a straightforward process, making algebraic manipulations and solving equations much more manageable.
Basic Concepts of Simplifying Radicals
Simplifying radicals involves expressing a radical expression in its simplest form. This means ensuring there are no perfect square factors (other than 1) inside the square root. The process relies on the properties of square roots and prime factorization. Let's break down the steps:
Steps to Simplify Radical Expressions
-
Identify Perfect Square Factors: Look for perfect square factors of the radicand (the number under the radical). A perfect square is a number that has a whole number as its square root, such as 4, 9, 16, 25, etc.
-
Rewrite the Radicand: Express the radicand as a product of its perfect square factors and other factors. For example:
- For \(\sqrt{72}\), rewrite 72 as \(36 \times 2\) because 36 is a perfect square.
So, \(\sqrt{72} = \sqrt{36 \times 2}\).
-
Apply the Product Property: Use the product property of square roots, which states \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\). This allows you to separate the factors under the square root:
- \(\sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2}\).
-
Simplify the Square Roots: Simplify the square roots of the perfect squares. For instance:
- \(\sqrt{36} = 6\).
Thus, \(\sqrt{36} \times \sqrt{2} = 6\sqrt{2}\).
-
Combine and Simplify: Combine the simplified terms to get the final simplified form of the radical:
- \(\sqrt{72} = 6\sqrt{2}\).
Example Problems
Let's look at a few more examples to solidify the concept:
- Example 1: Simplify \(\sqrt{50}\).
- Find perfect square factors of 50: \(25 \times 2\).
- Apply the product property: \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2}\).
- Simplify: \(\sqrt{25} = 5\), so \(\sqrt{50} = 5\sqrt{2}\).
- Example 2: Simplify \(\sqrt{18}\).
- Find perfect square factors of 18: \(9 \times 2\).
- Apply the product property: \(\sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2}\).
- Simplify: \(\sqrt{9} = 3\), so \(\sqrt{18} = 3\sqrt{2}\).
Prime Factorization Method
Another method to simplify radicals is prime factorization:
-
Factor the Radicand: Write the radicand as a product of prime factors. For example, for \(\sqrt{48}\), prime factors are \(2 \times 2 \times 2 \times 2 \times 3\).
-
Group the Factors: Pair the prime factors:
- \(\sqrt{2 \times 2 \times 2 \times 2 \times 3} = \sqrt{(2 \times 2) \times (2 \times 2) \times 3}\).
-
Simplify: Bring the pairs out of the square root:
- \(\sqrt{(2 \times 2) \times (2 \times 2) \times 3} = 2 \times 2 \sqrt{3} = 4\sqrt{3}\).
Rules for Simplifying Radical Expressions
- \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\), \(b \neq 0\)
- \(\sqrt{a} + \sqrt{b} \neq \sqrt{a + b}\)
- \(\sqrt{a} - \sqrt{b} \neq \sqrt{a - b}\)
Properties and Rules of Radical Expressions
Understanding the properties and rules of radical expressions is crucial for simplifying them effectively. Below are some fundamental properties and rules that are commonly used:
1. Product Property of Radicals
The product property states that the square root of a product is equal to the product of the square roots of the factors.
\(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\)
This property allows us to break down complex radicals into simpler components.
2. Quotient Property of Radicals
The quotient property states that the square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator.
\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
This property helps in simplifying fractions that involve radicals.
3. Simplifying Radicals
A radical expression is simplified if there are no perfect square factors other than 1 in the radicand. To simplify a radical expression, we look for factors that are perfect squares and then apply the product property.
For example, to simplify \(\sqrt{72}\):
- Find the largest perfect square factor of 72, which is 36.
- Rewrite the radicand as a product: \(\sqrt{72} = \sqrt{36 \cdot 2}\).
- Apply the product property: \(\sqrt{36 \cdot 2} = \sqrt{36} \cdot \sqrt{2} = 6\sqrt{2}\).
4. Rationalizing the Denominator
When a radical expression appears in the denominator, we often rationalize it by multiplying the numerator and denominator by a suitable radical that will eliminate the radical in the denominator.
For example, to rationalize \(\frac{1}{\sqrt{2}}\):
- Multiply both the numerator and the denominator by \(\sqrt{2}\): \(\frac{1}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}\).
5. Combining Like Radicals
Like radicals have the same radicand and index. We can combine them by adding or subtracting their coefficients.
For example, \(\sqrt{2} + 3\sqrt{2} = 4\sqrt{2}\).
6. Dealing with Variables
When variables are involved in the radicand, we apply the same principles. For example, to simplify \(\sqrt{12x^2y^4}\):
- Factor the radicand into perfect squares: \(12x^2y^4 = 4 \cdot 3 \cdot x^2 \cdot y^4\).
- Apply the product property: \(\sqrt{4 \cdot 3 \cdot x^2 \cdot y^4} = \sqrt{4} \cdot \sqrt{3} \cdot \sqrt{x^2} \cdot \sqrt{y^4}\).
- Simplify: \(2 \cdot \sqrt{3} \cdot x \cdot y^2 = 2xy^2\sqrt{3}\).
Prime Factorization Method
The prime factorization method is a fundamental approach to simplifying radical expressions. This method involves breaking down the number inside the radical into its prime factors. Here’s a step-by-step guide to using this method:
-
Identify the Prime Factors: Find the prime factorization of the number inside the radical (the radicand). For example, to simplify \(\sqrt{72}\), first find the prime factors of 72.
- 72 = 2 × 2 × 2 × 3 × 3
-
Group the Prime Factors: Group the prime factors into pairs. Each pair of prime factors represents a perfect square that can be taken out of the radical.
- \(72 = (2 \times 2) \times (3 \times 3) \times 2\)
-
Simplify the Radical: For each pair of prime factors, take one factor out of the radical. Multiply these factors together to get the simplified form of the radical.
- \(\sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\)
Here are a few more examples to illustrate the process:
| Radical Expression | Prime Factorization | Grouped Factors | Simplified Form |
|---|---|---|---|
| \(\sqrt{50}\) | 50 = 2 × 5 × 5 | (5 × 5) × 2 | 5\(\sqrt{2}\) |
| \(\sqrt{180}\) | 180 = 2 × 2 × 3 × 3 × 5 | (2 × 2) × (3 × 3) × 5 | 6\(\sqrt{5}\) |
Using the prime factorization method, you can simplify radical expressions by identifying and extracting perfect square factors, leaving a simplified radical in its most reduced form.

Simplifying Radicals with Coefficients
When simplifying radicals with coefficients, the process involves breaking down the radicand (the number inside the radical) into its prime factors, simplifying the radical, and then multiplying the coefficient by any numbers that are simplified out of the radical. Here is a step-by-step guide:
-
Identify the largest perfect square factor of the radicand.
For example, simplify \( 3\sqrt{72} \).
-
Rewrite the radicand as a product of the perfect square and its matching factor.
\( 3\sqrt{72} = 3\sqrt{36 \times 2} \)
-
Apply the product rule for radicals \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \).
\( 3\sqrt{36 \times 2} = 3(\sqrt{36} \times \sqrt{2}) \)
-
Simplify the perfect square radical.
\( 3(\sqrt{36} \times \sqrt{2}) = 3(6 \times \sqrt{2}) \)
-
Multiply the coefficient by the simplified radical.
\( 3 \times 6 \sqrt{2} = 18\sqrt{2} \)
So, \( 3\sqrt{72} = 18\sqrt{2} \).
Let’s consider another example:
-
Example: Simplify \( 5\sqrt{50} \).
-
Identify the largest perfect square factor of 50, which is 25.
\( 5\sqrt{50} = 5\sqrt{25 \times 2} \)
-
Rewrite the radical.
\( 5\sqrt{25 \times 2} = 5(\sqrt{25} \times \sqrt{2}) \)
-
Simplify the perfect square radical.
\( 5(\sqrt{25} \times \sqrt{2}) = 5(5 \times \sqrt{2}) \)
-
Multiply the coefficient by the simplified radical.
\( 5 \times 5\sqrt{2} = 25\sqrt{2} \)
So, \( 5\sqrt{50} = 25\sqrt{2} \).
By following these steps, you can simplify any radical expression with coefficients. Practice these steps with different radicands and coefficients to become more familiar with the process.
| Expression | Step-by-Step Simplification | Final Result |
|---|---|---|
| \( 2\sqrt{18} \) | \( 2\sqrt{9 \times 2} = 2(\sqrt{9} \times \sqrt{2}) = 2(3 \times \sqrt{2}) \) | \( 6\sqrt{2} \) |
| \( 4\sqrt{32} \) | \( 4\sqrt{16 \times 2} = 4(\sqrt{16} \times \sqrt{2}) = 4(4 \times \sqrt{2}) \) | \( 16\sqrt{2} \) |
| \( 7\sqrt{45} \) | \( 7\sqrt{9 \times 5} = 7(\sqrt{9} \times \sqrt{5}) = 7(3 \times \sqrt{5}) \) | \( 21\sqrt{5} \) |
Simplifying Radicals with Variables
Simplifying radicals with variables follows similar rules to simplifying radicals with numerical coefficients. The main goal is to factor the expression under the radical and then apply the properties of radicals to simplify. Here are the steps to simplify radicals with variables:
-
Factor the expression under the radical: Write the expression under the radical as a product of its prime factors, including the variables. For example, to simplify \( \sqrt{50x^4y^6} \), first factor the expression inside the radical:
\[
\sqrt{50x^4y^6} = \sqrt{2 \cdot 5^2 \cdot x^4 \cdot y^6}
\] -
Group the factors into pairs of identical factors: Group the factors in pairs (for square roots) or in triples (for cube roots), etc. Here, we group the pairs:
\[
\sqrt{2 \cdot 5^2 \cdot x^4 \cdot y^6} = \sqrt{2 \cdot (5^2) \cdot (x^2)^2 \cdot (y^3)^2}
\] -
Bring out the pairs as single factors: For every pair (or appropriate group size), bring out one factor from under the radical:
\[
\sqrt{2 \cdot (5^2) \cdot (x^2)^2 \cdot (y^3)^2} = 5 \cdot x^2 \cdot y^3 \cdot \sqrt{2}
\] -
Combine the simplified factors: Multiply the factors that have been brought out of the radical:
\[
5 \cdot x^2 \cdot y^3 \cdot \sqrt{2}
\]So, the simplified form of \( \sqrt{50x^4y^6} \) is \( 5x^2y^3\sqrt{2} \).
Here are some more examples to illustrate this process:
-
Example 1: Simplify \( \sqrt{18x^3y^4} \)
\[
\sqrt{18x^3y^4} = \sqrt{2 \cdot 3^2 \cdot x^2 \cdot x \cdot (y^2)^2} = 3xy^2\sqrt{2x}
\] -
Example 2: Simplify \( \sqrt[3]{40m^5} \)
\[
\sqrt[3]{40m^5} = \sqrt[3]{2^3 \cdot 5 \cdot m^3 \cdot m^2} = 2m\sqrt[3]{5m^2}
\]
Remember, when simplifying radicals with variables, always look for perfect square (or cube, etc.) factors to simplify. This helps in reducing the expression under the radical and makes the final expression simpler and more manageable.
Using the Product Property to Simplify Radicals
The product property of radicals states that the radical of a product is the product of the radicals. This property can be used to simplify radicals, especially when dealing with non-perfect square numbers. The property is defined as follows:
\[\sqrt[n]{a \cdot b} = \sqrt[n]{a} \cdot \sqrt[n]{b}\]
Here’s a step-by-step guide to using the product property to simplify radicals:
- Identify the largest perfect square factor of the number under the radical (the radicand).
- Rewrite the radicand as a product of this perfect square factor and another factor.
- Apply the product property to split the radical into two separate radicals.
- Simplify the radical of the perfect square factor.
Let's look at some examples to illustrate this process:
Example 1: Simplify \(\sqrt{98}\)
- Find the largest perfect square factor of 98. Here, it’s 49.
- Rewrite 98 as \(49 \cdot 2\).
- Apply the product property: \(\sqrt{98} = \sqrt{49 \cdot 2} = \sqrt{49} \cdot \sqrt{2}\).
- Simplify: \(\sqrt{49} = 7\), so \(\sqrt{98} = 7\sqrt{2}\).
Example 2: Simplify \(\sqrt[3]{16}\)
- Find the largest perfect cube factor of 16. Here, it’s 8.
- Rewrite 16 as \(8 \cdot 2\).
- Apply the product property: \(\sqrt[3]{16} = \sqrt[3]{8 \cdot 2} = \sqrt[3]{8} \cdot \sqrt[3]{2}\).
- Simplify: \(\sqrt[3]{8} = 2\), so \(\sqrt[3]{16} = 2\sqrt[3]{2}\).
By using the product property, complex radicals can be broken down into simpler parts, making them easier to work with.
Using the Quotient Property to Simplify Radicals
The Quotient Property of radicals states that for any non-negative real numbers \(a\) and \(b\) (with \(b \neq 0\)), the square root of a quotient is the quotient of the square roots:
\[
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\]
Here is a step-by-step guide to using the Quotient Property to simplify radical expressions:
- Simplify the fraction under the radical if possible: Factor the numerator and the denominator to see if there are any common factors that can be cancelled out.
- Apply the Quotient Property: Rewrite the square root of the fraction as the quotient of the square roots of the numerator and the denominator.
- Simplify the radicals: Simplify the square roots in the numerator and the denominator separately.
Let's look at some examples:
Example 1
Simplify \(\sqrt{\frac{45}{80}}\)
- First, simplify inside the radical by factoring out the greatest common factor: \[ \sqrt{\frac{45}{80}} = \sqrt{\frac{5 \cdot 9}{5 \cdot 16}} = \sqrt{\frac{9}{16}} \]
- Apply the Quotient Property: \[ \sqrt{\frac{9}{16}} = \frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4} \]
Example 2
Simplify \(\sqrt[3]{\frac{16}{54}}\)
- Simplify inside the radical: \[ \sqrt[3]{\frac{16}{54}} = \sqrt[3]{\frac{2 \cdot 8}{2 \cdot 27}} = \sqrt[3]{\frac{8}{27}} \]
- Apply the Quotient Property: \[ \sqrt[3]{\frac{8}{27}} = \frac{\sqrt[3]{8}}{\sqrt[3]{27}} = \frac{2}{3} \]
Example 3
Simplify \(\sqrt{\frac{75x^5}{3x}}\)
- Simplify inside the radical: \[ \sqrt{\frac{75x^5}{3x}} = \sqrt{\frac{25x^4}{1}} = \sqrt{25x^4} \]
- Simplify the radical: \[ \sqrt{25x^4} = 5x^2 \]
These examples illustrate how to apply the Quotient Property of radicals to simplify radical expressions step-by-step. Always start by simplifying the fraction inside the radical, apply the Quotient Property, and then simplify the radicals in the numerator and denominator separately.

Combining Like Radicals
Combining like radicals is similar to combining like terms in algebra. The radicals must have the same radicand (the number or expression inside the radical) and the same index (the degree of the root). Here is a step-by-step guide on how to combine like radicals:
- Identify the radicals with the same radicand.
- Simplify each radical if possible. This may involve factoring out perfect squares.
- Combine the coefficients of the like radicals by addition or subtraction.
Let's look at a few examples:
Example 1: Combine \(2\sqrt{3} + 5\sqrt{3}\)
- The radicands are the same (\(\sqrt{3}\)), so we can combine the coefficients.
- \(2\sqrt{3} + 5\sqrt{3} = (2 + 5)\sqrt{3} = 7\sqrt{3}\)
Example 2: Combine \(4\sqrt{5} - 3\sqrt{5}\)
- The radicands are the same (\(\sqrt{5}\)), so we can combine the coefficients.
- \(4\sqrt{5} - 3\sqrt{5} = (4 - 3)\sqrt{5} = 1\sqrt{5} = \sqrt{5}\)
Example 3: Combine \(3\sqrt{2} + 2\sqrt{3}\)
- The radicands are different (\(\sqrt{2}\) and \(\sqrt{3}\)), so we cannot combine these terms.
- The expression remains as \(3\sqrt{2} + 2\sqrt{3}\).
Sometimes, you may need to simplify the radicals before combining them:
Example 4: Combine \(2\sqrt{50} + 3\sqrt{2}\)
- Simplify \(\sqrt{50}\): \( \sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\)
- Now the expression is \(2(5\sqrt{2}) + 3\sqrt{2} = 10\sqrt{2} + 3\sqrt{2}\)
- Combine the coefficients: \(10\sqrt{2} + 3\sqrt{2} = 13\sqrt{2}\)
Here is another more complex example involving variables:
Example 5: Combine \(3\sqrt{12x^2} + 2\sqrt{3x^2}\)
- Simplify \(\sqrt{12x^2}\): \( \sqrt{12x^2} = \sqrt{4 \times 3x^2} = 2x\sqrt{3}\)
- Simplify \(\sqrt{3x^2}\): \( \sqrt{3x^2} = x\sqrt{3}\)
- Now the expression is \(3(2x\sqrt{3}) + 2(x\sqrt{3}) = 6x\sqrt{3} + 2x\sqrt{3}\)
- Combine the coefficients: \(6x\sqrt{3} + 2x\sqrt{3} = 8x\sqrt{3}\)
By following these steps, you can effectively combine like radicals and simplify your expressions.
Rationalizing the Denominator
Rationalizing the denominator is a technique used to eliminate radicals from the denominator of a fraction. This process simplifies the expression, making it easier to work with. Below are the steps to rationalize the denominator for different types of expressions:
Single-Term Denominators
When the denominator is a single-term radical, multiply both the numerator and the denominator by the same radical.
- Identify the radical in the denominator. For example, consider the fraction \( \frac{4\sqrt{3}}{\sqrt{7}} \).
- Multiply both the numerator and the denominator by the radical in the denominator: \( \frac{4\sqrt{3} \cdot \sqrt{7}}{\sqrt{7} \cdot \sqrt{7}} \).
- Simplify the expression: \( \frac{4\sqrt{21}}{7} \). Now the denominator is a rational number.
Binomial Denominators
When the denominator is a binomial with a radical, use the conjugate to rationalize.
- Identify the conjugate of the denominator. For example, for the fraction \( \frac{3}{\sqrt{2} + 3} \), the conjugate is \( \sqrt{2} - 3 \).
- Multiply both the numerator and the denominator by this conjugate: \( \frac{3(\sqrt{2} - 3)}{(\sqrt{2} + 3)(\sqrt{2} - 3)} \).
- Simplify using the difference of squares: \( \frac{3\sqrt{2} - 9}{2 - 9} = \frac{3\sqrt{2} - 9}{-7} \).
Examples
Here are some examples to illustrate the process:
- Example 1: Rationalize \( \frac{5}{\sqrt{3}} \):
- Multiply by \( \frac{\sqrt{3}}{\sqrt{3}} \): \( \frac{5\sqrt{3}}{3} \).
- Example 2: Rationalize \( \frac{2}{3 + \sqrt{5}} \):
- Multiply by \( \frac{3 - \sqrt{5}}{3 - \sqrt{5}} \): \( \frac{2(3 - \sqrt{5})}{(3 + \sqrt{5})(3 - \sqrt{5})} = \frac{6 - 2\sqrt{5}}{9 - 5} = \frac{6 - 2\sqrt{5}}{4} \).
By following these steps, you can rationalize any denominator, ensuring your final expression is simpler and easier to manage. Practicing these techniques will help you become more proficient in handling radical expressions.
Special Cases and Common Mistakes
When simplifying radical expressions, there are several special cases and common mistakes to be aware of. Understanding these can help ensure accuracy and avoid errors. Below, we will discuss some of these cases and mistakes, along with tips to address them effectively.
Special Cases
- Radicals with Perfect Squares: If the number under the radical (the radicand) is a perfect square, it can be simplified directly. For example, \( \sqrt{36} = 6 \) because \( 36 = 6^2 \).
- Radicals with Variables: When simplifying radicals with variables, consider the exponent of the variable:
- If the exponent is even, the variable can be simplified. For example, \( \sqrt{x^6} = x^3 \) because \( x^6 = (x^3)^2 \).
- If the exponent is odd, separate the variable into a product of an even and an odd exponent. For example, \( \sqrt{y^5} = y^2 \sqrt{y} \) because \( y^5 = y^4 \cdot y \) and \( \sqrt{y^4} = y^2 \).
- Combining Radicals: Radicals can only be combined if they have the same radicand. For example, \( 2\sqrt{3} + 3\sqrt{3} = 5\sqrt{3} \), but \( 2\sqrt{2} + 3\sqrt{3} \) cannot be simplified further.
Common Mistakes
- Adding and Subtracting Radicals: A common mistake is to add or subtract the radicands directly. Remember, \( \sqrt{a} + \sqrt{b} \neq \sqrt{a+b} \). For example, \( \sqrt{4} + \sqrt{9} = 2 + 3 = 5 \), not \( \sqrt{13} \).
- Incorrectly Simplifying Radicals: Ensure that you factor the radicand completely. For example, \( \sqrt{50} = \sqrt{25 \cdot 2} = 5\sqrt{2} \), not \( 7\sqrt{2} \).
- Forgetting Absolute Values: When simplifying variables under even roots, always use absolute values to ensure non-negative results. For example, \( \sqrt{x^2} = |x| \).
Practice Problems
Below are some practice problems to help solidify these concepts:
- Simplify \( \sqrt{72} \).
- Simplify \( \sqrt{x^8} \).
- Simplify \( \sqrt{50} + \sqrt{18} \).
- Simplify \( \sqrt{y^7} \).
Solutions:
- \( \sqrt{72} = \sqrt{36 \cdot 2} = 6\sqrt{2} \)
- \( \sqrt{x^8} = x^4 \)
- \( \sqrt{50} + \sqrt{18} = 5\sqrt{2} + 3\sqrt{2} = 8\sqrt{2} \)
- \( \sqrt{y^7} = y^3 \sqrt{y} \)
By understanding these special cases and being mindful of common mistakes, you can effectively simplify radical expressions with greater confidence and accuracy.
Practice Problems and Solutions
Here are some practice problems and their step-by-step solutions to help you master simplifying radical expressions:
Problem 1: Simplify \( \sqrt{50} \)
- Factorize the number inside the radical: \( \sqrt{50} = \sqrt{2 \times 25} \).
- Separate the factors: \( \sqrt{2 \times 25} = \sqrt{2} \times \sqrt{25} \).
- Simplify the perfect square: \( \sqrt{25} = 5 \).
- Combine the results: \( \sqrt{50} = 5\sqrt{2} \).
Problem 2: Simplify \( \sqrt[3]{54x^3} \)
- Factorize the expression inside the radical: \( \sqrt[3]{54x^3} = \sqrt[3]{2 \times 3^3 \times x^3} \).
- Separate the factors: \( \sqrt[3]{2 \times 3^3 \times x^3} = \sqrt[3]{2} \times \sqrt[3]{3^3} \times \sqrt[3]{x^3} \).
- Simplify the perfect cubes: \( \sqrt[3]{3^3} = 3 \) and \( \sqrt[3]{x^3} = x \).
- Combine the results: \( \sqrt[3]{54x^3} = 3x \sqrt[3]{2} \).
Problem 3: Simplify \( \frac{\sqrt{12}}{\sqrt{3}} \)
- Combine the radicals: \( \frac{\sqrt{12}}{\sqrt{3}} = \sqrt{\frac{12}{3}} \).
- Simplify the fraction inside the radical: \( \sqrt{\frac{12}{3}} = \sqrt{4} \).
- Simplify the perfect square: \( \sqrt{4} = 2 \).
- Result: \( \frac{\sqrt{12}}{\sqrt{3}} = 2 \).
Problem 4: Simplify \( \sqrt{18x^4y^2} \)
- Factorize the expression inside the radical: \( \sqrt{18x^4y^2} = \sqrt{2 \times 3^2 \times x^4 \times y^2} \).
- Separate the factors: \( \sqrt{2 \times 3^2 \times x^4 \times y^2} = \sqrt{2} \times \sqrt{3^2} \times \sqrt{x^4} \times \sqrt{y^2} \).
- Simplify the perfect squares: \( \sqrt{3^2} = 3 \), \( \sqrt{x^4} = x^2 \), and \( \sqrt{y^2} = y \).
- Combine the results: \( \sqrt{18x^4y^2} = 3x^2y\sqrt{2} \).
Problem 5: Rationalize and Simplify \( \frac{\sqrt{7}}{\sqrt{5}} \)
- Multiply the numerator and the denominator by \( \sqrt{5} \): \( \frac{\sqrt{7}}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} = \frac{\sqrt{35}}{5} \).
- Result: \( \frac{\sqrt{7}}{\sqrt{5}} = \frac{\sqrt{35}}{5} \).
Problem 6: Simplify \( \sqrt[4]{16y^4} \)
- Factorize the expression inside the radical: \( \sqrt[4]{16y^4} = \sqrt[4]{2^4 \times y^4} \).
- Separate the factors: \( \sqrt[4]{2^4 \times y^4} = \sqrt[4]{2^4} \times \sqrt[4]{y^4} \).
- Simplify the perfect fourth powers: \( \sqrt[4]{2^4} = 2 \) and \( \sqrt[4]{y^4} = y \).
- Combine the results: \( \sqrt[4]{16y^4} = 2y \).

Advanced Techniques for Complex Expressions
When dealing with complex radical expressions, advanced techniques can simplify them effectively. Here are some methods:
- Combining Like Terms:
Identify and combine like terms under the same radical sign. For example:
\(\sqrt{50} + 2\sqrt{2}\)
First, simplify each term:
\(\sqrt{50} = \sqrt{25 \cdot 2} = 5\sqrt{2}\)
Then combine like terms:
\(5\sqrt{2} + 2\sqrt{2} = 7\sqrt{2}\)
- Rationalizing the Denominator:
Remove radicals from the denominator by multiplying by a conjugate or appropriate term. For example:
\(\frac{3}{\sqrt{2}}\)
Multiply numerator and denominator by \(\sqrt{2}\):
\(\frac{3 \cdot \sqrt{2}}{\sqrt{2} \cdot \sqrt{2}} = \frac{3\sqrt{2}}{2}\)
- Using the Product Property:
Simplify expressions using the property \(\sqrt[n]{a \cdot b} = \sqrt[n]{a} \cdot \sqrt[n]{b}\). For example:
\(\sqrt{12} \cdot \sqrt{3}\)
Combine the radicals:
\(\sqrt{12 \cdot 3} = \sqrt{36} = 6\)
- Using the Quotient Property:
Simplify expressions using the property \(\sqrt[n]{\frac{a}{b}} = \frac{\sqrt[n]{a}}{\sqrt[n]{b}}\). For example:
\(\sqrt{\frac{18}{2}}\)
Simplify under the radical:
\(\sqrt{9} = 3\)
- Working with Higher Order Roots:
Apply the same techniques to higher order roots, like cube roots. For example:
\(\sqrt[3]{40m^5}\)
Factor into cubes and simplify:
\(\sqrt[3]{2^3 \cdot 5 \cdot m^3 \cdot m^2} = 2m\sqrt[3]{5m^2}\)
- Simplifying Expressions with Multiple Radicals:
For complex expressions with multiple radicals, simplify each radical individually before combining. For example:
\(2\sqrt[4]{16x^9} \cdot \sqrt[4]{y^3} \cdot \sqrt[4]{81x^3y}\)
Simplify each term and combine:
\(2 \cdot 2x^2 \cdot \sqrt[4]{x} \cdot \sqrt[4]{y^3} \cdot 3 \cdot \sqrt[4]{x^3y} = 12x^2 \cdot x \cdot y = 12x^3y\)
By applying these advanced techniques, you can effectively simplify complex radical expressions, making them easier to work with in algebraic equations and real-world applications.
Applications of Simplified Radicals in Real-World Problems
Understanding how to simplify radical expressions is not only important for mathematical proficiency but also for solving a variety of real-world problems. Simplified radicals can appear in fields such as physics, engineering, architecture, and even finance. Below are some applications demonstrating how simplified radicals are used in real-world scenarios:
1. Engineering and Architecture
In engineering and architecture, simplified radicals are often used to calculate dimensions, areas, and volumes. For instance, when dealing with the geometry of a structure, the Pythagorean theorem may be employed, which involves radicals. Simplifying these radicals can help engineers and architects make precise calculations efficiently.
Example:
Consider the need to find the length of a diagonal in a rectangular building plot where the length is 30 meters and the width is 40 meters. Using the Pythagorean theorem:
\[ d = \sqrt{30^2 + 40^2} \]
\[ = \sqrt{900 + 1600} \]
\[ = \sqrt{2500} \]
\[ = 50 \text{ meters} \]
2. Physics
In physics, radicals often appear in equations involving motion, energy, and waves. Simplifying these expressions can lead to easier interpretations and solutions of physical problems. For example, the equations for kinetic energy and potential energy might involve square roots.
Example:
When calculating the period \( T \) of a simple pendulum, the formula involves a square root:
\[ T = 2\pi \sqrt{\frac{L}{g}} \]
Where \( L \) is the length of the pendulum and \( g \) is the acceleration due to gravity. Simplifying the radical expression provides a clearer understanding of how changes in length \( L \) affect the period \( T \).
3. Finance
In finance, radicals can be used in calculations involving interest rates and investment growth, especially when dealing with compound interest or continuous compounding.
Example:
For continuous compounding, the formula for the amount \( A \) after time \( t \) is:
\[ A = P e^{rt} \]
where \( P \) is the principal amount, \( r \) is the interest rate, and \( t \) is the time. Simplifying the expression involving \( e \) can help in understanding the growth rate of investments.
4. Medicine
In medicine, simplified radicals can be used in calculations for dosages and concentrations, where precise measurements are crucial for patient safety and treatment efficacy.
Example:
When determining the correct dosage of medication based on body surface area (BSA), the formula:
\[ \text{BSA} = \sqrt{\frac{height \times weight}{3600}} \]
uses a square root. Simplifying this expression helps medical professionals quickly and accurately calculate dosages.
5. Surveying and Navigation
Surveyors and navigators use simplified radicals to determine distances and angles accurately, which is crucial for mapping and navigation.
Example:
When calculating the shortest distance between two points on a map using coordinate geometry, the distance formula involves radicals:
\[ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
Simplifying this expression helps surveyors provide accurate measurements.
Conclusion
Simplified radicals are not just academic exercises; they are practical tools that facilitate accurate and efficient problem-solving in various real-world applications. By mastering the simplification of radical expressions, one can tackle complex problems in multiple disciplines with confidence.
Conclusion and Key Takeaways
Simplifying radical expressions is a crucial skill in algebra and mathematics as a whole. By understanding and applying the rules and properties of radicals, one can simplify complex expressions into more manageable forms. Here are the key takeaways from this guide:
- Prime Factorization: Breaking down a number into its prime factors is the foundation for simplifying radicals. This method helps in identifying pairs of factors that can be taken out of the radical sign.
- Product and Quotient Rules: These rules allow the simplification of radicals by multiplying or dividing the radicands first and then taking the root.
- Coefficients and Variables: When simplifying radicals with coefficients and variables, treat each part separately. Simplify the numeric part and the variable part by using their respective properties.
- Combining Like Radicals: Similar to combining like terms in algebra, like radicals can be combined to simplify expressions further. Ensure the radicands are identical before combining.
- Rationalizing the Denominator: This process eliminates radicals from the denominator of a fraction. Multiply the numerator and the denominator by the conjugate of the denominator when necessary.
- Special Cases: Be mindful of special cases such as simplifying radicals with even and odd exponents, and always consider the absolute value for even roots to ensure non-negative results.
By mastering these concepts, you can simplify radical expressions confidently and accurately, enhancing your problem-solving skills in mathematics. Practice regularly with different types of expressions to become proficient in applying these techniques.
Phương Pháp Đơn Giản Hóa Biểu Thức Căn Bậc Hai Dễ Dàng
READ MORE:
Đơn Giản Hóa Biểu Thức Căn Bậc Hai Với Biến Số