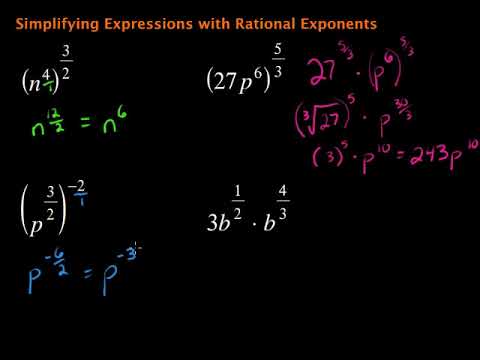How Do You Simplify a Radical Expression: A Comprehensive Guide

Simplifying a radical expression involves expressing it in its simplest form. Here are the steps and rules to simplify radical expressions:

Steps to Simplify a Radical Expression

1. Find the Prime Factorization: Write the number under the radical as a product of its prime factors.

   Example: \( \sqrt{72} = \sqrt{2 \times 2 \times 2 \times 3 \times 3} \)

2. Pair the Factors: Combine the factors in pairs of identical factors.

   Example: \( \sqrt{2 \times 2 \times 2 \times 3 \times 3} = \sqrt{(2^2) \times 2 \times (3^2)} \)

3. Simplify the Radical: Bring out the factors with pairs out of the radical sign.

   Example: \( \sqrt{(2^2) \times 2 \times (3^2)} = 2 \times 3 \sqrt{2} = 6\sqrt{2} \)

Properties of Radicals

• Product Property: \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \)

• Quotient Property: \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \), provided \( b \neq 0 \)

Examples

Example 1: Simplify \( \sqrt{50} \)

Step 1: Prime factorization: \( \sqrt{50} = \sqrt{2 \times 5 \times 5} \)

Step 2: Pair the factors: \( \sqrt{(5^2) \times 2} \)

Step 3: Simplify: \( 5\sqrt{2} \)

Example 2: Simplify \( \sqrt{48} \)

Step 1: Prime factorization: \( \sqrt{48} = \sqrt{2 \times 2 \times 2 \times 2 \times 3} \)

Step 2: Pair the factors: \( \sqrt{(2^2) \times (2^2) \times 3} \)

Step 3: Simplify: \( 2 \times 2\sqrt{3} = 4\sqrt{3} \)

Tips for Simplifying Radical Expressions

• Always look for the largest perfect square factor to simplify the process.

• If there is a radical in the denominator, rationalize it by multiplying the numerator and the denominator by the conjugate of the denominator.

• Remember that the square root of a number will always be non-negative, hence use the absolute value when necessary.

Radical expressions are mathematical expressions that contain a radical symbol (√) with an expression underneath it. The expression under the radical sign is called the radicand, and it can be a number, a variable, or a combination of both. Understanding how to simplify radical expressions is a fundamental skill in algebra and higher mathematics.

A radical expression is in its simplest form when the radicand has no perfect square factors other than 1, and no fractions appear inside the radical. Let's explore the basic properties and steps for simplifying radical expressions.

Basic Properties of Radicals

• Product Property: \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\)
• Quotient Property: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\), where \(b \ne 0\)
• Sum and Difference: \(\sqrt{a} + \sqrt{b} \ne \sqrt{a + b}\) and \(\sqrt{a} - \sqrt{b} \ne \sqrt{a - b}\)

Using these properties, we can break down and simplify radical expressions step-by-step.

Steps to Simplify Radical Expressions

1. Factor the Radicand: Begin by factoring the number or expression inside the radical to find its prime factors or factor pairs.
2. Pair the Factors: Group the factors into pairs. Each pair of identical factors can be taken out of the radical as a single factor.
3. Simplify the Expression: Multiply the factors outside the radical and reduce the expression inside the radical if possible.

Here is a practical example to illustrate the process:

Example: Simplifying \(\sqrt{72}\)

Step 1: Factor the radicand \(72\) into its prime factors:

\[
72 = 2 \times 2 \times 2 \times 3 \times 3
\]

Step 2: Pair the factors:

\[
72 = (2 \times 2) \times 2 \times (3 \times 3)
\]

Step 3: Simplify by taking out pairs of factors:

\[
\sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}
\]

Thus, \(\sqrt{72}\) simplifies to \(6\sqrt{2}\).

By following these steps and using the properties of radicals, you can simplify any radical expression effectively.

Radicals are mathematical symbols used to represent the root of a number. The most common radical is the square root, denoted as \(\sqrt{}\), but radicals can also represent cube roots, fourth roots, and so on. The number or expression inside the radical symbol is called the radicand.

In general, a radical expression is written as \(\sqrt[n]{a}\), where \(n\) is the index of the radical and \(a\) is the radicand. When the index \(n\) is 2, it is typically omitted, making \(\sqrt{a}\) imply the square root of \(a\).

Basic Properties of Radicals

• The square root of a number \(a\) is a value \(x\) such that \(x^2 = a\).
• For any non-negative number \(a\), the principal square root is always non-negative.
• If \(a \geq 0\) and \(b \geq 0\), then \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\) (Product Property).
• If \(a \geq 0\) and \(b > 0\), then \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\) (Quotient Property).

Examples

Consider the radical expression \(\sqrt{50}\). To simplify this, we find the largest perfect square factor of 50, which is 25. Thus, we can rewrite:

\(\sqrt{50} = \sqrt{25 \cdot 2} = \sqrt{25} \cdot \sqrt{2} = 5\sqrt{2}\)

For a cube root example, consider \(\sqrt[3]{27x^3}\). We apply the product property and simplify:

\(\sqrt[3]{27x^3} = \sqrt[3]{3^3 \cdot x^3} = \sqrt[3]{3^3} \cdot \sqrt[3]{x^3} = 3x\)

Prime Factorization Method

Simplifying radicals often involves prime factorization. To simplify \(\sqrt{72}\), we first factor 72 into prime factors:

72 = 2^3 \cdot 3^2

Using the properties of radicals, we can simplify:

\(\sqrt{72} = \sqrt{2^3 \cdot 3^2} = \sqrt{2^2 \cdot 2 \cdot 3^2} = \sqrt{2^2} \cdot \sqrt{3^2} \cdot \sqrt{2} = 2 \cdot 3 \cdot \sqrt{2} = 6\sqrt{2}\)

Rationalizing the Denominator

Rationalizing involves eliminating radicals from the denominator of a fraction. For example, to rationalize \(\frac{5}{\sqrt{3}}\), multiply the numerator and the denominator by \(\sqrt{3}\):

\(\frac{5}{\sqrt{3}} \cdot \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)

Understanding radicals and radicands is essential for simplifying radical expressions and performing various operations involving radicals.

Understanding the basic properties of radicals is crucial for simplifying radical expressions. These properties help us manipulate and simplify radicals in a systematic way. Here are some fundamental properties of radicals:

• Product Property of Radicals: This property states that the radical of a product is the product of the radicals. Mathematically, it can be expressed as: \[ \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \] For example, \(\sqrt{36} = \sqrt{9 \cdot 4} = \sqrt{9} \cdot \sqrt{4} = 3 \cdot 2 = 6\).
• Quotient Property of Radicals: This property indicates that the radical of a quotient is the quotient of the radicals. It can be written as: \[ \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \] For instance, \(\sqrt{\frac{25}{4}} = \frac{\sqrt{25}}{\sqrt{4}} = \frac{5}{2}\).
• Power Property of Radicals: This property relates to exponents and radicals, stating that: \[ (\sqrt[n]{a})^m = a^{\frac{m}{n}} \] For example, \((\sqrt[3]{8})^2 = 8^{\frac{2}{3}} = (2^3)^{\frac{2}{3}} = 2^2 = 4\).

Let's explore these properties further with examples:

Example 1: Simplifying Using the Product Property

Consider the radical expression \(\sqrt{72}\). To simplify it using the product property:

1. Factor the radicand into a product of prime factors: \(72 = 2^3 \cdot 3^2\).
2. Group the factors into pairs of squares: \(72 = (2^2 \cdot 3^2) \cdot 2\).
3. Apply the product property: \(\sqrt{72} = \sqrt{(2^2 \cdot 3^2) \cdot 2} = \sqrt{2^2} \cdot \sqrt{3^2} \cdot \sqrt{2} = 2 \cdot 3 \cdot \sqrt{2} = 6\sqrt{2}\).

Example 2: Simplifying Using the Quotient Property

Consider the radical expression \(\sqrt{\frac{50}{2}}\). To simplify it using the quotient property:

1. Divide the radicand: \(\frac{50}{2} = 25\).
2. Apply the quotient property: \(\sqrt{\frac{50}{2}} = \sqrt{25} = 5\).

Example 3: Simplifying Radicals with Variables

Consider the radical expression \(\sqrt{x^4}\). To simplify it:

1. Recognize that the exponent is even: \(x^4 = (x^2)^2\).
2. Apply the power property: \(\sqrt{x^4} = (x^2)^2 = x^2\).

By understanding and applying these basic properties of radicals, you can simplify various radical expressions effectively. Practice these steps with different examples to become proficient in handling radicals.

The product property of radicals is a fundamental concept used to simplify radical expressions. This property states that the square root of a product is equal to the product of the square roots of the individual factors. Mathematically, this can be expressed as:

\(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\)

Here are the steps to apply the product property of radicals:

1. Identify the Factors: Start by identifying the factors of the radicand (the number under the radical sign).
2. Separate the Factors: Use the product property to separate the factors into individual square roots.
3. Simplify Each Factor: Simplify each square root if possible.
4. Multiply the Results: Multiply the simplified square roots together.

Let's go through an example:

Example: Simplify \(\sqrt{50}\).

Solution:

1. Identify the Factors: \(50 = 25 \times 2\)
2. Separate the Factors: \(\sqrt{50} = \sqrt{25 \times 2}\)
3. Apply the Product Property: \(\sqrt{25 \times 2} = \sqrt{25} \cdot \sqrt{2}\)
4. Simplify Each Factor: \(\sqrt{25} = 5\) and \(\sqrt{2}\) remains as is.
5. Multiply the Results: \(5 \cdot \sqrt{2} = 5\sqrt{2}\)

Therefore, \(\sqrt{50} = 5\sqrt{2}\).

This property is particularly useful for simplifying more complex radical expressions and for operations involving radicals, such as multiplication and division.

Practice Problems:

1. Simplify \(\sqrt{72}\)
2. Simplify \(\sqrt{18}\)
3. Simplify \(\sqrt{8 \cdot 2}\)

The quotient property of radicals is a useful tool in simplifying radical expressions that involve division. This property states that the radical of a quotient is equal to the quotient of the radicals. Mathematically, it can be expressed as:

\[ \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \quad \text{for} \quad b \neq 0 \]

Here are the steps to simplify a radical expression using the quotient property:

1. Separate the numerator and the denominator under individual radicals.
2. Simplify the radicals in the numerator and the denominator separately, if possible.
3. If there is a radical in the denominator, rationalize the denominator by multiplying both the numerator and the denominator by the conjugate of the denominator.

Let's look at an example to understand this better:

Example: Simplify \(\sqrt{\frac{45}{5}}\)

1. First, separate the radicals: \[ \sqrt{\frac{45}{5}} = \frac{\sqrt{45}}{\sqrt{5}} \]
2. Next, simplify the radicals individually: \[ \sqrt{45} = \sqrt{9 \times 5} = 3\sqrt{5} \] \[ \sqrt{5} = \sqrt{5} \]
3. Now, substitute back the simplified forms: \[ \frac{3\sqrt{5}}{\sqrt{5}} \]
4. Finally, simplify the expression by canceling out the common factor: \[ \frac{3\sqrt{5}}{\sqrt{5}} = 3 \]

In some cases, the denominator may still contain a radical even after simplification. In such cases, you can rationalize the denominator by multiplying the numerator and the denominator by the conjugate of the denominator.

Example: Simplify \(\frac{\sqrt{2}}{\sqrt{3}}\)

1. Multiply the numerator and the denominator by \(\sqrt{3}\) to rationalize the denominator: \[ \frac{\sqrt{2}}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{6}}{3} \]

Using these steps, you can simplify any radical expression involving division by applying the quotient property of radicals effectively.

Simplifying square roots involves breaking down the number inside the radical into its prime factors and then applying the property of square roots that allows us to simplify the expression. Here is a step-by-step guide:

1. Identify Perfect Square Factors:

   Find the largest perfect square factor of the number under the square root. For example, for \(\sqrt{72}\), identify that \(72 = 36 \times 2\), where 36 is a perfect square.

2. Rewrite the Radical:

   Express the radical as a product of two square roots. Using the example above:

   \[\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2}\]

3. Simplify the Square Root of the Perfect Square:

   Simplify the square root of the perfect square factor. Continuing with our example:

   \[\sqrt{36} = 6\]

4. Multiply the Results:

   Combine the simplified square root with the remaining radical. Therefore:

   \[\sqrt{72} = 6 \times \sqrt{2} = 6\sqrt{2}\]

This process ensures the square root is expressed in its simplest form. Let's look at another example:

• Example: Simplify \(\sqrt{50}\)

  1. Identify perfect square factors: \(50 = 25 \times 2\)
  2. Rewrite the radical: \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2}\)
  3. Simplify the square root of the perfect square: \(\sqrt{25} = 5\)
  4. Combine the results: \(\sqrt{50} = 5\sqrt{2}\)

By following these steps, you can simplify any square root expression effectively.

Higher-order roots, such as cube roots and fourth roots, can be simplified using similar techniques to those used for square roots. The key is to identify factors under the radical that are perfect powers matching the order of the root.

Here are the steps to simplify higher-order roots:

1. Factor the radicand into its prime factors:

   Break down the number or expression under the radical into its prime factors.

   Example: \(\sqrt[3]{40m^5}\)

   Prime factorization: \(40 = 2 \cdot 2 \cdot 2 \cdot 5\) and \(m^5 = m^3 \cdot m^2\)

   So, \(\sqrt[3]{40m^5} = \sqrt[3]{2^3 \cdot 5 \cdot m^3 \cdot m^2}\)

2. Group the factors into sets that match the root order:

   For a cube root, group the factors into sets of three.

   Example: \(\sqrt[3]{2^3 \cdot 5 \cdot m^3 \cdot m^2} = \sqrt[3]{2^3} \cdot \sqrt[3]{5} \cdot \sqrt[3]{m^3} \cdot \sqrt[3]{m^2}\)

3. Simplify each group:

   Take the cube root of each group. The cube root of a perfect cube (like \(2^3\) or \(m^3\)) is the base itself.

   Example: \(\sqrt[3]{2^3} = 2\), \(\sqrt[3]{m^3} = m\)

   So, \(\sqrt[3]{40m^5} = 2m \cdot \sqrt[3]{5m^2}\)

4. Multiply the simplified terms:

   Combine the simplified terms outside the radical with the remaining radical expression.

   Example: \(2m \cdot \sqrt[3]{5m^2}\)

By following these steps, you can simplify higher-order roots efficiently. This process involves breaking down the radicand, grouping the factors, and simplifying the expression step by step.

To simplify radical expressions using the prime factorization method, follow these steps:

1. Identify the radicand, which is the number under the radical sign.
2. Find the prime factors of the radicand.
3. Pair the prime factors in groups of two.
4. Take out each pair of factors that are the same and are outside the square root sign.
5. Multiply the numbers that are outside the square root sign.
6. Multiply the numbers that are inside the square root sign and put the answer inside the square root sign.
7. Make sure the product inside the square root is as simple as possible.

When simplifying radical expressions by pairing factors, follow these steps:

1. Identify the radicand, which is the number under the radical sign.
2. Factor the radicand into its prime factors.
3. Look for pairs of identical factors inside the square root sign.
4. For each pair, write one factor outside the square root sign.
5. If there are any remaining factors that cannot be paired, leave them inside the square root sign.
6. Simplify the expression further if possible by evaluating the square roots and multiplying any remaining factors.

To rationalize the denominator of a radical expression, follow these steps:

1. Identify the denominator of the fraction containing the radical.
2. Multiply both the numerator and the denominator by a suitable expression that will eliminate the radical from the denominator.
3. For a square root in the denominator, multiply by the conjugate of the denominator.
4. Simplify the resulting expression by distributing and combining like terms.
5. Verify that the denominator no longer contains any radicals.

To rationalize the denominator using conjugates, follow these steps:

1. Identify the radical in the denominator of the fraction.
2. Find the conjugate of the denominator, which is obtained by changing the sign of the radical term.
3. Multiply both the numerator and the denominator by this conjugate.
4. Distribute and simplify the resulting expression.
5. Verify that the denominator no longer contains any radicals.

When simplifying radicals containing variables, follow these steps:

1. Identify the radicand, which includes the variable under the radical sign.
2. Factor the expression under the radical into its prime factors, including the variable.
3. For each pair of like factors inside the square root, write one factor outside the square root.
4. If any factors cannot be paired inside the square root, leave them as they are.
5. Simplify any square roots of perfect squares and combine any remaining factors.
6. Ensure the expression is in its simplest form.

When combining like radicals, follow these steps:

1. Identify radicals that have the same index (such as square roots) and the same radicand.
2. Add or subtract the coefficients (numbers outside the radicals) of the like radicals.
3. Keep the radical part (the square root or other root) unchanged.
4. If there are no like radicals, leave the expression as it is.

When performing operations (addition, subtraction, multiplication, division) with radical expressions, follow these guidelines:

1. Adding and Subtracting: Combine like radicals by adding or subtracting their coefficients while keeping the radical part unchanged.
2. Multiplying: Multiply the entire expressions, including the coefficients and the radicals. Use the product property of radicals to simplify.
3. Dividing: Divide the entire expressions, including the coefficients and the radicals. Rationalize the denominator if necessary using conjugates.

Adding and subtracting radical expressions involves combining like terms, much like you would with polynomial expressions. Here's a step-by-step guide to help you understand the process:

Step-by-Step Guide

1. Simplify each radical expression: Before combining, ensure each radical expression is simplified.

   • Example:
     \(3 \sqrt{50} - 2 \sqrt{8} - 5 \sqrt{32}\)
     Simplify each term:
     \(\sqrt{50} = \sqrt{25 \cdot 2} = 5 \sqrt{2}\)
\(\sqrt{8} = \sqrt{4 \cdot 2} = 2 \sqrt{2}\)
\(\sqrt{32} = \sqrt{16 \cdot 2} = 4 \sqrt{2}\)

2. Combine like terms: Only radicals with the same radicand can be combined.

   • Example:
     \(3 \sqrt{50} - 2 \sqrt{8} - 5 \sqrt{32}\) simplifies to:
     3 \cdot 5 \sqrt{2} - 2 \cdot 2 \sqrt{2} - 5 \cdot 4 \sqrt{2} = 15 \sqrt{2} - 4 \sqrt{2} - 20 \sqrt{2} = -9 \sqrt{2}\)

Examples

Here are some additional examples to illustrate the process:

• Example 1: Simplify \(2 \sqrt{12} + \sqrt{27}\)

  Simplify each term:
  
  \(\sqrt{12} = 2 \sqrt{3}\)

\(\sqrt{27} = 3 \sqrt{3}\)
  
  Combine like terms:
  
  2 \cdot 2 \sqrt{3} + 3 \sqrt{3} = 4 \sqrt{3} + 3 \sqrt{3} = 7 \sqrt{3}

• Example 2: Simplify \(4 \sqrt{2} - 3 \sqrt{3}\)

  Since the radicands (2 and 3) are different, this expression cannot be simplified further.

• Example 3: Simplify \(4 \sqrt{ \frac{20}{9} } + 5 \sqrt{ \frac{45}{16} }\)

  Simplify each term:
  
  \(\sqrt{ \frac{20}{9} } = \frac{\sqrt{20}}{\sqrt{9}} = \frac{2 \sqrt{5}}{3}\)

\(\sqrt{ \frac{45}{16} } = \frac{\sqrt{45}}{\sqrt{16}} = \frac{3 \sqrt{5}}{4}\)
  
  Combine like terms:
  
  4 \cdot \frac{2}{3} \sqrt{5} + 5 \cdot \frac{3}{4} \sqrt{5} = \frac{8}{3} \sqrt{5} + \frac{15}{4} \sqrt{5} = \left( \frac{8}{3} + \frac{15}{4} \right) \sqrt{5} = \frac{77}{12} \sqrt{5}

Practice Exercises

Try these exercises to practice adding and subtracting radical expressions:

• Simplify: \( \sqrt{50} - \sqrt{32} \)
• Simplify: \( 2\sqrt{12} - 3 \sqrt{27} \)

Remember, always simplify the radicals first, and then combine like terms. Happy calculating!

Multiplying radical expressions involves a few straightforward steps. Here’s how you can do it:

1. Multiply the Coefficients:

   Start by multiplying the coefficients (the numbers outside the radical signs). For example, if you have \(3\sqrt{2}\) and \(4\sqrt{3}\), multiply the coefficients 3 and 4:

   \(3 \times 4 = 12\)

2. Multiply the Radicands:

   Next, multiply the numbers inside the radical signs (the radicands). Continuing with our example:

   \(\sqrt{2} \times \sqrt{3} = \sqrt{6}\)

3. Combine the Results:

   Now, combine the results from the previous steps. The product of \(3\sqrt{2}\) and \(4\sqrt{3}\) is:

   \(12\sqrt{6}\)

4. Simplify if Possible:

   If the radicand can be simplified, do so. For instance, if you have \(\sqrt{8}\), you can simplify it to \(2\sqrt{2}\) because 8 is \(4 \times 2\) and \(\sqrt{4} = 2\).

Let’s go through some examples to solidify these steps.

Example 1

Multiply \(5\sqrt{10}\) and \(3\sqrt{2}\).

1. Multiply the coefficients: \(5 \times 3 = 15\)
2. Multiply the radicands: \(\sqrt{10} \times \sqrt{2} = \sqrt{20}\)
3. Combine the results: \(15\sqrt{20}\)
4. Simplify the radicand: \(\sqrt{20} = \sqrt{4 \times 5} = 2\sqrt{5}\)
5. Final result: \(15 \times 2\sqrt{5} = 30\sqrt{5}\)

Example 2

Multiply \(2\sqrt{3}\) and \(4\sqrt{12}\).

1. Multiply the coefficients: \(2 \times 4 = 8\)
2. Multiply the radicands: \(\sqrt{3} \times \sqrt{12} = \sqrt{36}\)
3. Combine the results: \(8\sqrt{36}\)
4. Simplify the radicand: \(\sqrt{36} = 6\)
5. Final result: \(8 \times 6 = 48\)

Example 3

Multiply \(\sqrt{5}\) and \(\sqrt{20}\).

1. Since there are no coefficients, move directly to the radicands: \(\sqrt{5} \times \sqrt{20} = \sqrt{100}\)
2. Simplify the radicand: \(\sqrt{100} = 10\)
3. Final result: \(10\)

By following these steps, you can effectively multiply any radical expressions. Remember, practice is key to mastering this technique!

Dividing radical expressions involves simplifying the quotient of two radicals. Here are the steps to follow:

1. Combine the Radicals: Use the Quotient Property of Radicals to combine the division into a single radical expression. The property states:

   \[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]

2. Simplify the Fraction: Simplify the fraction inside the radical as much as possible by reducing common factors.

3. Simplify the Radical: Simplify the resulting radical expression by factoring out perfect squares (or cubes, etc.) if applicable.

4. Rationalize the Denominator (if necessary): If the denominator is still a radical, rationalize it by multiplying both the numerator and the denominator by a suitable radical to eliminate the radical in the denominator.

Let's look at some examples:

Example 1

Simplify:

\[\frac{\sqrt{72x^3}}{\sqrt{162x}}\]

1. Combine the radicals using the Quotient Property:

\[\sqrt{\frac{72x^3}{162x}}\]

2. Simplify the fraction inside the radical:

\[\sqrt{\frac{72 \cdot x^2 \cdot x}{162 \cdot x}} = \sqrt{\frac{4x^2}{9}}\]

3. Simplify the resulting radical:

\[\frac{2x}{3}\]

Example 2

Simplify:

\[\frac{\sqrt[3]{32x^2}}{\sqrt[3]{4x^5}}\]

1. Combine the radicals using the Quotient Property:

\[\sqrt[3]{\frac{32x^2}{4x^5}}\]

2. Simplify the fraction inside the radical:

\[\sqrt[3]{\frac{8}{x^3}}\]

3. Simplify the resulting radical:

\[\frac{2}{x}\]

Rationalizing the Denominator

If the denominator is a radical, we often need to rationalize it. This involves multiplying both the numerator and the denominator by a suitable radical that will eliminate the radical in the denominator. Here’s an example:

Example 3

Simplify and rationalize:

\[\frac{4}{\sqrt{5} + \sqrt{3}}\]

1. Multiply the numerator and the denominator by the conjugate of the denominator:

\[\frac{4}{\sqrt{5} + \sqrt{3}} \cdot \frac{\sqrt{5} - \sqrt{3}}{\sqrt{5} - \sqrt{3}} = \frac{4(\sqrt{5} - \sqrt{3})}{(\sqrt{5} + \sqrt{3})(\sqrt{5} - \sqrt{3})}\]

2. Apply the difference of squares formula in the denominator:

\[\frac{4(\sqrt{5} - \sqrt{3})}{5 - 3} = \frac{4(\sqrt{5} - \sqrt{3})}{2} = 2(\sqrt{5} - \sqrt{3})\]

These steps will help you simplify and divide radical expressions accurately.

Simplifying complex radical expressions involves breaking down the expression into its simplest form. Here are the steps and methods to simplify complex radicals:

Steps to Simplify Complex Radical Expressions

1. Factorize the Radicand:

   Identify and factorize the radicand (the number or expression inside the radical) into its prime factors or its simplest components.

   Example: \(\sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2}\)

2. Apply the Product and Quotient Properties:

   Use the properties of radicals to separate the factors inside the radical into separate radicals, if possible.

   Product Property: \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\)

   Quotient Property: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)

   Example: \(\sqrt{\frac{12}{5}} = \frac{\sqrt{12}}{\sqrt{5}} = \frac{2\sqrt{3}}{\sqrt{5}}\)

3. Simplify the Radicals:

   Simplify the individual radicals if they contain perfect square factors.

   Example: \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\)

4. Combine Like Terms:

   If the expression contains like radicals, combine them by adding or subtracting the coefficients.

   Example: \(3\sqrt{2} + 4\sqrt{2} = 7\sqrt{2}\)

5. Rationalize the Denominator:

   If there is a radical in the denominator, multiply the numerator and the denominator by the conjugate to eliminate the radical.

   Example: \(\frac{\sqrt{2} + \sqrt{3}}{\sqrt{5} - \sqrt{2}} \cdot \frac{\sqrt{5} + \sqrt{2}}{\sqrt{5} + \sqrt{2}} = \frac{(\sqrt{2} + \sqrt{3})(\sqrt{5} + \sqrt{2})}{3}\)

Example: Simplifying a Complex Radical Expression

Simplify the following expression: \(\sqrt{48x^6y^8}\)

1. Factorize the radicand: \(\sqrt{48 \times x^6 \times y^8} = \sqrt{16 \times 3 \times (x^3)^2 \times (y^4)^2}\)

2. Apply the product property: \(\sqrt{16 \times 3 \times (x^3)^2 \times (y^4)^2} = \sqrt{16} \times \sqrt{3} \times \sqrt{(x^3)^2} \times \sqrt{(y^4)^2}\)

3. Simplify the radicals: \(4 \times \sqrt{3} \times x^3 \times y^4\)

Final answer: \(4x^3y^4\sqrt{3}\)

By following these steps and using these properties, you can simplify even the most complex radical expressions efficiently.