Topic formula for perimeter of a sector of a circle: Discover the essential formula for calculating the perimeter of a sector of a circle. This comprehensive guide will walk you through the components, step-by-step calculations, and practical applications of this formula. Whether you're a student, teacher, or math enthusiast, this article will provide clear explanations and examples to enhance your understanding.
Table of Content
- Formula for Perimeter of a Sector of a Circle
- Introduction
- Definition of a Sector
- Formula for Perimeter of a Sector
- Components of the Perimeter Formula
- Example Calculations
- Arc Length Calculation
- Special Cases and Considerations
- Applications in Real Life
- Frequently Asked Questions (FAQs)
- Practice Problems
- Conclusion
- YOUTUBE:
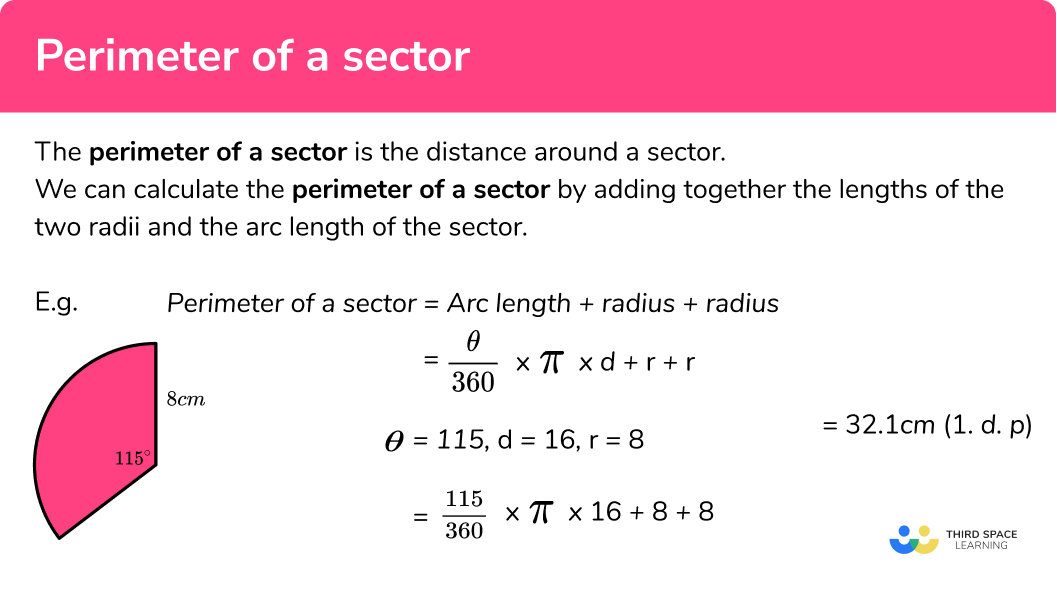
Formula for Perimeter of a Sector of a Circle
The perimeter of a sector of a circle is calculated by adding the lengths of the two radii and the arc length that forms the sector. The formula to determine the perimeter (P) of a sector of a circle with radius r and angle θ in degrees is given by:
\[ P = 2r + \frac{\theta}{360} \times 2\pi r \]
Step-by-Step Calculation
- Determine the Radius (r): Measure the distance from the center of the circle to any point on its boundary.
- Measure the Central Angle (θ): Identify the angle subtended by the sector at the center of the circle. Ensure it is in degrees.
- Calculate the Arc Length: Use the formula for arc length, \( L = \frac{\theta}{360} \times 2\pi r \), to find the length of the curved boundary of the sector.
- Sum the Components: Add the lengths of the two radii and the arc length to get the perimeter.
Example Calculation
For a sector with a radius of 5 units and a central angle of 60 degrees:
- Radius (\( r \)) = 5 units
- Central Angle (\( \theta \)) = 60 degrees
Using the formula for arc length:
\[ L = \frac{60}{360} \times 2\pi \times 5 = \frac{1}{6} \times 2\pi \times 5 = \frac{10\pi}{6} = \frac{5\pi}{3} \]
Calculate the perimeter:
\[ P = 2 \times 5 + \frac{5\pi}{3} = 10 + \frac{5\pi}{3} \]
Thus, the perimeter is:
\[ P = 10 + \frac{5\pi}{3} \approx 10 + 5.24 = 15.24 \text{ units} \]
Summary
The perimeter of a sector includes the lengths of the two radii plus the length of the arc. Using the formula \[ P = 2r + \frac{\theta}{360} \times 2\pi r \], you can easily calculate the perimeter by following the outlined steps.
READ MORE:
Introduction
The formula for the perimeter of a sector of a circle is a fundamental concept in geometry. Understanding this formula is crucial for solving various problems involving circular sectors. A sector of a circle is defined by two radii and an arc, forming a slice of the circle. The perimeter of the sector includes the lengths of the two radii and the arc. This article delves into the details of calculating the perimeter, offering a clear step-by-step approach to enhance your understanding and application of this concept.
- Definitions and Key Concepts
- What is a Sector?
- Components of a Sector: Radii and Arc
- Formula for Perimeter of a Sector
- Basic Formula: \( P = 2r + L \)
- Deriving the Arc Length: \( L = \theta r \) for radians
- Complete Formula: \( P = 2r + \theta r \)
- Steps to Calculate the Perimeter
- Step 1: Measure the Radius
- Step 2: Measure or Calculate the Central Angle
- Step 3: Calculate the Arc Length
- Step 4: Apply the Formula
- Example Calculations
- Example 1: Given Radius and Arc Length
- Example 2: Given Radius and Angle in Degrees
- Example 3: Given Radius and Angle in Radians
- Applications in Real Life
- Engineering and Architecture
- Design and Art
- Conclusion
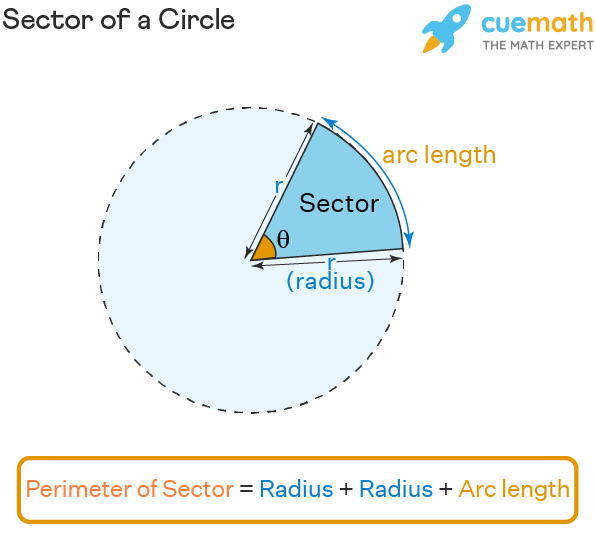
Definition of a Sector
A sector of a circle is a portion of the circle enclosed by two radii and the arc between them. It resembles a slice of pie or pizza. The central angle formed by the two radii determines the size of the sector.
Key characteristics of a sector include:
- Radius (r): The distance from the center of the circle to any point on the circle.
- Arc Length (l): The length of the curved line that forms the boundary of the sector.
- Central Angle (θ): The angle formed at the center of the circle by the two radii. This angle can be measured in degrees or radians.
Formulas Involving a Sector
The primary formulas related to a sector of a circle are for its area and perimeter:
- Area of a Sector: The area (A) of a sector with a central angle θ (in radians) and radius r is given by:
- In radians: \( A = \frac{1}{2} \theta r^2 \)
- In degrees: \( A = \frac{\theta}{360} \pi r^2 \)
- Perimeter of a Sector: The perimeter (P) of a sector is the sum of the lengths of the two radii and the arc length. The formula is:
- Perimeter: \( P = 2r + l \)
- Where arc length \( l \) is calculated as: \( l = \frac{\theta}{360} \times 2\pi r \) for θ in degrees or \( l = \theta r \) for θ in radians.
Formula for Perimeter of a Sector
The perimeter of a sector of a circle is the sum of the lengths of the two radii and the arc. The formula to calculate the perimeter of a sector is as follows:
Perimeter of a sector = \(2r + l\), where:
- \(r\) is the radius of the circle.
- \(l\) is the length of the arc.
The length of the arc can be calculated using the angle of the sector, \(\theta\), with the formula:
\(l = \left(\frac{\theta}{360}\right) \times 2\pi r\)
Thus, the complete formula for the perimeter of a sector is:
\(P = 2r + \left(\frac{\theta}{360}\right) \times 2\pi r\)
Steps to Calculate the Perimeter of a Sector:
- Determine the radius of the circle (\(r\)).
- Find the central angle of the sector (\(\theta\)) in degrees.
- Convert the central angle from degrees to radians if needed: \(\theta \text{(radians)} = \theta \text{(degrees)} \times \frac{\pi}{180}\).
- Calculate the arc length (\(l\)) using the formula \(l = \left(\frac{\theta}{360}\right) \times 2\pi r\).
- Compute the perimeter of the sector using \(P = 2r + l\).
Example Calculation:
For a sector with a radius of 8 units and a central angle of 30°:
- Radius (\(r\)) = 8 units
- Central angle (\(\theta\)) = 30°
- Convert 30° to radians: \(\theta = 30 \times \frac{\pi}{180} = \frac{\pi}{6}\)
- Calculate the arc length: \(l = \left(\frac{30}{360}\right) \times 2\pi \times 8 = \frac{1}{12} \times 2\pi \times 8 = \frac{16\pi}{12} = \frac{4\pi}{3}\)
- Compute the perimeter: \(P = 2 \times 8 + \frac{4\pi}{3} \approx 16 + 4.19 = 20.19\) units
Therefore, the perimeter of the sector is approximately 20.19 units.
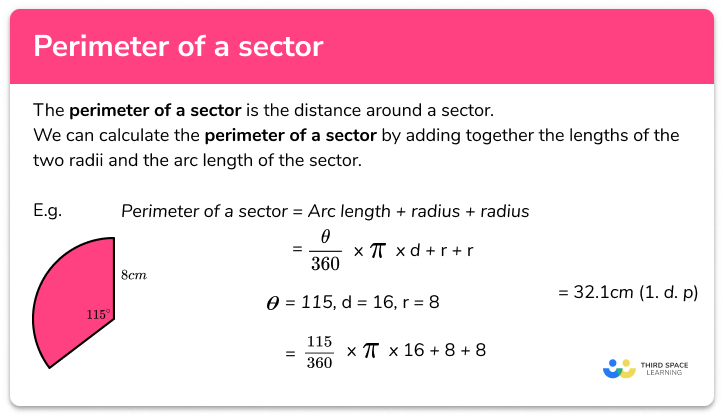
Components of the Perimeter Formula
The perimeter of a sector of a circle involves the measurement of its boundary, which includes both the arc length and the radii. Here, we break down the essential components of the formula used to calculate the perimeter.
- Radius (\( r \)): The radius is the distance from the center of the circle to any point on its circumference. For a sector, two radii are involved as it is the region bounded by two radii and the arc.
- Central Angle (\( \theta \)): The central angle of the sector is the angle formed by the two radii at the center of the circle. This angle can be in degrees or radians.
- Arc Length (\( L \)): The arc length is the distance along the curved line forming the boundary of the sector between the two radii.
Using these components, the perimeter \( P \) of a sector is given by the formula:
\[
P = 2r + L
\]
Here, \( L \) (the arc length) can be calculated if not directly provided, using the central angle \( \theta \) as follows:
\[
L = \theta \times r
\]
Thus, the perimeter formula can be expressed in terms of the radius and central angle as:
\[
P = 2r + \theta r = r (2 + \theta)
\]
Where:
- \( r \) - Radius of the circle
- \( \theta \) - Central angle in radians
- \( L \) - Arc length
This formula highlights that to find the perimeter of a sector, you need to know the radius of the circle and the central angle or the arc length. By understanding these components, you can accurately determine the perimeter of any sector.
Example Calculations
Understanding the formula for the perimeter of a sector of a circle is greatly enhanced through practical examples. Below are detailed step-by-step calculations for various scenarios.
Example 1: Given Radius and Central Angle
Let's calculate the perimeter of a sector with a radius of 8 cm and a central angle of 60 degrees.
- Step 1: Calculate the arc length.
- Arc length \( L = \frac{\theta}{360} \times 2 \pi r \)
- \( L = \frac{60}{360} \times 2 \pi \times 8 \)
- \( L = \frac{1}{6} \times 16 \pi \)
- \( L = \frac{16 \pi}{6} \)
- \( L \approx 8.38 \, \text{cm} \)
- Step 2: Calculate the total perimeter.
- Perimeter \( P = 2r + L \)
- \( P = 2 \times 8 + 8.38 \)
- \( P \approx 24.38 \, \text{cm} \)
Example 2: Given Diameter and Central Angle
Now, calculate the perimeter of a sector with a diameter of 24 cm and a central angle of 180 degrees.
- Step 1: Calculate the radius.
- Radius \( r = \frac{d}{2} \)
- \( r = \frac{24}{2} \)
- \( r = 12 \, \text{cm} \)
- Step 2: Calculate the arc length.
- Arc length \( L = \frac{\theta}{360} \times 2 \pi r \)
- \( L = \frac{180}{360} \times 2 \pi \times 12 \)
- \( L = \pi \times 12 \)
- \( L = 12 \pi \, \text{cm} \)
- Step 3: Calculate the total perimeter.
- Perimeter \( P = 2r + L \)
- \( P = 2 \times 12 + 12 \pi \)
- \( P \approx 24 + 37.7 \)
- \( P \approx 61.7 \, \text{cm} \)
Example 3: Given Radius and Arc Length
For a sector with a radius of 5.5 cm and an arc length of 20 cm, calculate the central angle and perimeter.
- Step 1: Calculate the central angle.
- Arc length \( L = \frac{\theta}{360} \times 2 \pi r \)
- \( 20 = \frac{\theta}{360} \times 2 \pi \times 5.5 \)
- \( \theta = \frac{20 \times 360}{2 \pi \times 5.5} \)
- \( \theta \approx 208.6 \, \text{degrees} \)
- Step 2: Calculate the total perimeter.
- Perimeter \( P = 2r + L \)
- \( P = 2 \times 5.5 + 20 \)
- \( P = 11 + 20 \)
- \( P = 31 \, \text{cm} \)
Arc Length Calculation
The arc length of a sector of a circle is the distance along the curved edge of the sector. To calculate the arc length, you need to know the radius of the circle and the measure of the central angle of the sector. The formula for the arc length (L) is:
\( L = \theta \times r \)
where:
- \( L \) = Arc length
- \( \theta \) = Central angle in radians
- \( r \) = Radius of the circle
If the central angle is given in degrees, you can convert it to radians by using the formula:
\( \theta (\text{radians}) = \theta (\text{degrees}) \times \frac{\pi}{180} \)
Then, use the converted angle in the arc length formula.
Step-by-Step Calculation
- Determine the radius (\( r \)) of the circle.
- Measure the central angle (\( \theta \)). If the angle is in degrees, convert it to radians using the formula: \( \theta (\text{radians}) = \theta (\text{degrees}) \times \frac{\pi}{180} \).
- Substitute the values of \( r \) and \( \theta \) into the arc length formula \( L = \theta \times r \).
- Calculate the result to find the arc length.
Example Calculation
Let's calculate the arc length of a sector with a radius of 10 cm and a central angle of 60 degrees.
- Convert the central angle from degrees to radians:
- Substitute the radius and the angle in radians into the arc length formula:
- Calculate the arc length:
\( 60^\circ \times \frac{\pi}{180} = \frac{\pi}{3} \) radians
\( L = \frac{\pi}{3} \times 10 \)
\( L = \frac{10\pi}{3} \approx 10.47 \) cm
Thus, the arc length of the sector is approximately 10.47 cm.
Special Cases and Considerations
When dealing with the perimeter of a sector, there are several special cases and considerations to keep in mind:
1. Perimeter of a Semi-Circle Sector
A semi-circle sector is when the central angle is 180 degrees or π radians. The perimeter of such a sector includes the arc length and the diameters. The arc length for a semi-circle can be calculated as:
\[
\text{Arc Length} = \pi r
\]
where \( r \) is the radius.
Therefore, the perimeter \( P \) of the semi-circle sector is:
\[
P = 2r + \pi r
\]
Thus, the formula simplifies to:
\[
P = r (2 + \pi)
\]
2. Perimeter of a Quadrant Sector
A quadrant sector is when the central angle is 90 degrees or π/2 radians. The arc length for a quadrant can be calculated as:
\[
\text{Arc Length} = \frac{\pi r}{2}
\]
where \( r \) is the radius.
Therefore, the perimeter \( P \) of the quadrant sector is:
\[
P = 2r + \frac{\pi r}{2}
\]
Thus, the formula simplifies to:
\[
P = r \left(2 + \frac{\pi}{2}\right)
\]
3. Sector with a Very Small Angle
When the central angle \( \theta \) is very small (approaching 0), the arc length becomes negligible, and the perimeter of the sector is approximately equal to twice the radius:
\[
P \approx 2r
\]
4. Sector with a Full Circle
When the central angle \( \theta \) is 360 degrees or \( 2\pi \) radians, the sector becomes a full circle. In this case, the perimeter is simply the circumference of the circle:
\[
P = 2\pi r
\]
5. Adjusting for Units
When performing calculations, ensure that all measurements are in consistent units. For example, if the radius is given in centimeters, the arc length and perimeter should also be calculated in centimeters.
6. Angle Conversion
When the angle is given in degrees, it must be converted to radians for use in formulas. The conversion is:
\[
\theta \text{ (in radians)} = \theta \text{ (in degrees)} \times \frac{\pi}{180}
\]
7. Real-life Applications
Sectors of circles appear in various real-life scenarios such as slices of pizza, pieces of pie, and segments of a circular track. Understanding the perimeter calculations helps in determining the boundaries and lengths in these practical applications.
Applications in Real Life
The formula for the perimeter of a sector of a circle has various practical applications in real life. Below are some of the significant uses:
- Architecture and Engineering:
In architecture and engineering, sectors are often used in the design of arches and curved structures. The perimeter calculation helps in determining the amount of materials needed, such as bricks or tiles, to cover the curved surfaces.
- Landscaping and Garden Design:
When designing gardens, especially those with circular or semi-circular layouts, the perimeter of sectors is used to plan paths, borders, and fencing. For instance, a garden with a circular path will require accurate perimeter calculations to determine the length of the edging materials.
- Manufacturing and Construction:
In manufacturing, especially in creating components with circular segments like pipes, gears, or circular windows, the perimeter of a sector is crucial for precise cutting and fitting. Construction projects may also use these calculations for elements like curved walls and domes.
- Surveying and Land Division:
Surveyors use the perimeter of sectors to calculate the boundaries of irregularly shaped plots of land, ensuring accurate land division and fencing requirements. This is particularly useful in determining the borders of properties that include curved boundaries.
- Navigation and Maritime Applications:
In maritime navigation, the concept of a sector is used to define the area covered by a lighthouse beam or radar sweep. The perimeter helps in understanding the range and coverage of these signals.
- Clock Design:
In designing analog clocks, the perimeter of a sector can determine the length of the clock hands and the spacing of the hour marks, ensuring an accurate and aesthetically pleasing layout.
- Education:
In educational settings, understanding the perimeter of sectors is essential for teaching geometry and trigonometry concepts. It provides practical examples that help students grasp the real-world applications of mathematical principles.
Frequently Asked Questions (FAQs)
-
What is the Perimeter of a Sector?
The perimeter of a sector of a circle is the total length of its boundary, which includes two radii and the arc length that forms the sector. It can be calculated if the length of the arc and the radius are known.
-
What is the Formula for the Perimeter of a Sector?
The formula to find the perimeter of a sector is:
\[ \text{Perimeter of sector} = 2r + l \]
where \( r \) is the radius and \( l \) is the arc length. If the arc length is not known, it can be calculated using the formula \( l = r \theta \) (where \( \theta \) is the central angle in radians).
-
How to Find the Perimeter of a Sector of a Circle?
The perimeter of a sector can be calculated using the following steps:
- Convert the central angle from degrees to radians if necessary using \( \text{Radians} = \frac{\text{Degrees} \times \pi}{180} \).
- Calculate the arc length using \( l = r \theta \) (where \( r \) is the radius and \( \theta \) is the central angle in radians).
- Use the formula \( \text{Perimeter of sector} = 2r + l \) to find the perimeter.
-
What are Some Practical Applications of Sector Perimeter and Area Calculations?
The calculations of the perimeter and area of a sector have various practical applications, including:
- Engineering: Used to calculate areas and perimeters in construction projects, such as determining the floor area of a building or fencing a park.
- Physics: Helps in various calculations involving circular motion and sectors.
- Design: Useful in graphic design and architecture to create circular segments and arcs.
-
What is the Difference Between a Sector and a Segment of a Circle?
A sector is a portion of a circle enclosed by two radii and the arc between them. A segment, however, is the area enclosed by an arc and a chord (a straight line connecting two points on the circle).
-
How Can I Convert the Central Angle from Degrees to Radians for Sector Calculations?
To convert an angle from degrees to radians, multiply the number of degrees by \( \frac{\pi}{180} \). For example, to convert 60 degrees to radians:
\[ 60^\circ \times \frac{\pi}{180} = \frac{\pi}{3} \]
Practice Problems
Here are some practice problems to help you master the calculation of the perimeter of a sector of a circle:
-
Problem 1: Calculate the perimeter of a sector with a radius of 10 cm and a central angle of 60 degrees.
Solution:
- Calculate the arc length:
\[ l = \frac{\theta}{360} \times 2\pi r = \frac{60}{360} \times 2\pi \times 10 = \frac{1}{6} \times 20\pi = \frac{10\pi}{3} \, \text{cm} \]
- Calculate the perimeter:
\[ P = 2r + l = 2 \times 10 + \frac{10\pi}{3} = 20 + \frac{10\pi}{3} \, \text{cm} \]
- Calculate the arc length:
-
Problem 2: Find the perimeter of a sector with a radius of 5 cm and an arc length of 6 cm.
Solution:
- Calculate the perimeter:
\[ P = 2r + l = 2 \times 5 + 6 = 10 + 6 = 16 \, \text{cm} \]
- Calculate the perimeter:
-
Problem 3: A sector has a radius of 7 units and an angle of 45 degrees. Find its perimeter.
Solution:
- Calculate the arc length:
\[ l = \frac{\theta}{360} \times 2\pi r = \frac{45}{360} \times 2\pi \times 7 = \frac{1}{8} \times 14\pi = \frac{7\pi}{4} \, \text{units} \]
- Calculate the perimeter:
\[ P = 2r + l = 2 \times 7 + \frac{7\pi}{4} = 14 + \frac{7\pi}{4} \, \text{units} \]
- Calculate the arc length:
-
Problem 4: Calculate the perimeter of a sector with a radius of 8 cm and a central angle of 90 degrees.
Solution:
- Calculate the arc length:
\[ l = \frac{\theta}{360} \times 2\pi r = \frac{90}{360} \times 2\pi \times 8 = \frac{1}{4} \times 16\pi = 4\pi \, \text{cm} \]
- Calculate the perimeter:
\[ P = 2r + l = 2 \times 8 + 4\pi = 16 + 4\pi \, \text{cm} \]
- Calculate the arc length:
-
Problem 5: A sector has an arc length of 12 cm and a radius of 6 cm. Find the perimeter of the sector.
Solution:
- Calculate the perimeter:
\[ P = 2r + l = 2 \times 6 + 12 = 12 + 12 = 24 \, \text{cm} \]
- Calculate the perimeter:
These problems should provide a good practice for calculating the perimeter of sectors in various scenarios. Remember, the key steps are to determine the arc length and then add the lengths of the two radii.
Conclusion
Understanding the formula for the perimeter of a sector of a circle is crucial for solving various geometric problems. The perimeter of a sector is the sum of the lengths of its two radii and the arc, calculated using the formula:
\[ \text{Perimeter} = 2r + l \]
where \( r \) is the radius and \( l \) is the arc length. The arc length can be found using:
\[ l = \frac{\theta}{360} \times 2\pi r \]
These formulas are essential in various real-life applications, including engineering, architecture, and design. They help in accurately determining the dimensions of circular segments, ensuring precise measurements and constructions.
By mastering the concepts and formulas related to the perimeter of a sector, you enhance your mathematical toolkit, enabling you to tackle more complex problems with confidence. Practice applying these formulas through diverse examples and problems to reinforce your understanding and proficiency.
In conclusion, the formula for the perimeter of a sector is a fundamental aspect of circle geometry, with wide-ranging applications in both academic and professional contexts. Mastery of this formula not only aids in solving geometric problems but also provides a strong foundation for further studies in mathematics and related fields.
Cách tìm chu vi của một cung tròn sử dụng công thức độ dài cung
READ MORE:
Kỹ Năng Chính - Tìm chu vi của một cung tròn.