Topic square root 90: Discover the fascinating world of square roots with our comprehensive guide on the square root of 90. Learn how to calculate, simplify, and apply this mathematical concept in various contexts, making it easy to grasp even for beginners. Dive into practical methods and real-life applications to enhance your understanding and appreciation of mathematics.
Table of Content
- Square Root of 90
- Introduction to Square Roots
- Definition and Basics of Square Roots
- Mathematical Representation of Square Root of 90
- Methods to Calculate Square Root of 90
- Prime Factorization Method
- Long Division Method
- Approximation Method
- Properties of Square Roots
- Rational and Irrational Numbers
- Is the Square Root of 90 Rational or Irrational?
- Simplifying Square Roots
- How to Simplify the Square Root of 90
- Practical Applications of Square Roots
- Use of Square Roots in Real Life
- Square Roots in Geometry
- Common Misconceptions about Square Roots
- Conclusion
- YOUTUBE: Cách đơn giản hóa căn bậc hai của 90 | Root 90
Square Root of 90
The square root of 90 is a mathematical expression that represents a value which, when multiplied by itself, equals 90. The square root of 90 can be represented in different forms such as radical form, exponential form, and decimal form.
Radical Form
The square root of 90 in radical form is:
Exponential Form
The square root of 90 in exponential form is:
\(90^{\frac{1}{2}}\)
Decimal Form
The square root of 90 in decimal form is approximately:
\(\sqrt{90} \approx 9.486832980505138\)
Simplifying the Square Root of 90
To simplify \(\sqrt{90}\), we factor 90 into its prime factors:
\(90 = 9 \times 10 = 3^2 \times 10\)
Using the property of square roots, we get:
\(\sqrt{90} = \sqrt{3^2 \times 10} = \sqrt{3^2} \times \sqrt{10} = 3\sqrt{10}\)
Steps to Calculate the Square Root of 90
- Factor 90 into its prime factors: \(90 = 3^2 \times 10\).
- Rewrite the square root of the product: \(\sqrt{3^2 \times 10} = \sqrt{3^2} \times \sqrt{10}\).
- Simplify: \(\sqrt{3^2} = 3\), hence \(\sqrt{90} = 3\sqrt{10}\).
Example Problem
Let's solve an example problem involving the square root of 90:
Jolly wants to fence her square garden which has an area of 90 square yards. How long will the fence be?
Given the area of the garden, \(90 = \text{side}^2\). The side of the garden is \(\sqrt{90} \approx 9.48\) yards. Therefore, the length of the fence is \(4 \times 9.48 = 37.92\) yards.
Key Points
- The square root of 90 is an irrational number.
- It lies between the perfect squares of 81 and 100, so \(\sqrt{90}\) is between 9 and 10.
- The square root of 90 rounded to the nearest tenth is approximately 9.5.
Frequently Asked Questions
- Is the square root of 90 a rational number? No, the square root of 90 is irrational because it cannot be expressed as a simple fraction.
- Can the square root of 90 be simplified? Yes, \(\sqrt{90}\) can be simplified to \(3\sqrt{10}\).
- What is the approximate value of the square root of 90? The approximate value of \(\sqrt{90}\) is 9.4868.

READ MORE:
Introduction to Square Roots
The concept of square roots is fundamental in mathematics, providing a basis for understanding more complex mathematical operations and concepts. A square root of a number \( x \) is a value that, when multiplied by itself, gives the original number \( x \). In mathematical terms, if \( y \) is the square root of \( x \), then \( y^2 = x \).
For example, the square root of 90 is a number which, when squared, equals 90. The symbol for the square root is \( \sqrt{} \), so the square root of 90 is written as \( \sqrt{90} \).
Square roots can be classified into two main types:
- Perfect Square Roots: These are square roots of perfect squares, such as 1, 4, 9, 16, etc., which are whole numbers.
- Non-Perfect Square Roots: These include square roots of numbers that are not perfect squares, such as 2, 3, 5, 7, 90, etc., which result in irrational numbers.
To understand square roots better, let's explore the properties and methods to calculate them:
- Properties of Square Roots:
- Every positive number has two square roots: a positive and a negative one. For example, the square roots of 25 are 5 and -5.
- The square root of zero is zero.
- Square roots of negative numbers are not real numbers but are complex numbers.
- Methods to Calculate Square Roots:
- Prime Factorization: Breaking down the number into its prime factors to simplify the square root.
- Long Division Method: A step-by-step approach to finding the square root of a number manually.
- Approximation Method: Estimating the square root by finding two consecutive integers between which the square root lies.
In the case of 90, we can use these methods to find that the square root of 90 is approximately 9.4868, which is an irrational number.
Definition and Basics of Square Roots
A square root of a number is a value that, when multiplied by itself, produces the original number. Mathematically, if \( y \) is the square root of \( x \), then \( y^2 = x \). The square root of a number \( x \) is denoted as \( \sqrt{x} \).
For example, \( \sqrt{90} \) represents the square root of 90. The number 90 is not a perfect square, so its square root is an irrational number. To express this concept clearly:
Let's consider the basics:
- Positive and Negative Roots: Every positive number has two square roots: a positive root and a negative root. For example, the square roots of 25 are 5 and -5 because \( 5^2 = 25 \) and \( (-5)^2 = 25 \).
- Perfect Squares: A number is a perfect square if its square root is an integer. Examples include 1, 4, 9, 16, 25, etc.
- Non-Perfect Squares: Numbers that are not perfect squares have square roots that are irrational numbers. Examples include 2, 3, 5, 7, 10, and 90.
To calculate the square root of a non-perfect square like 90, we can use various methods:
- Prime Factorization Method:
- First, find the prime factors of 90: \( 90 = 2 \times 3^2 \times 5 \).
- Group the prime factors into pairs: \( 90 = (3 \times 3) \times (2 \times 5) \).
- Take one factor from each pair: \( \sqrt{90} = 3 \times \sqrt{2 \times 5} \).
- Simplify the remaining square root: \( 3 \times \sqrt{10} \).
- Long Division Method:
- Pair the digits of the number from right to left: 90.
- Find the largest number whose square is less than or equal to 90. Here, it is 9, because \( 9^2 = 81 \).
- Divide and find the remainder: \( 90 - 81 = 9 \).
- Repeat the process to get a more precise value.
- Approximation Method:
- Identify two consecutive perfect squares between which 90 lies. Here, 81 (\( 9^2 \)) and 100 (\( 10^2 \)).
- Estimate the square root of 90 to be between 9 and 10.
- Refine the approximation through iterative methods or a calculator to get \( \sqrt{90} \approx 9.4868 \).
Understanding the definition and basics of square roots is crucial for solving various mathematical problems and for practical applications in science, engineering, and everyday life.
Mathematical Representation of Square Root of 90
The square root of a number is a value that, when multiplied by itself, results in the original number. The square root of 90 is represented as \( \sqrt{90} \). Since 90 is not a perfect square, its square root is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation is non-terminating and non-repeating.
To represent \( \sqrt{90} \) mathematically, we can break it down into several steps and forms:
- Prime Factorization:
- First, find the prime factors of 90:
\( 90 = 2 \times 3^2 \times 5 \)
- Next, express the square root using these factors:
\( \sqrt{90} = \sqrt{2 \times 3^2 \times 5} \)
- Simplify by taking the square root of the perfect square (32):
\( \sqrt{90} = \sqrt{3^2 \times 2 \times 5} = 3 \sqrt{10} \)
- First, find the prime factors of 90:
- Decimal Representation:
- Using a calculator or approximation methods, we find that:
\( \sqrt{90} \approx 9.4868 \)
- This decimal form is useful for practical calculations and estimations.
- Using a calculator or approximation methods, we find that:
- Expression in Terms of Square Roots:
- The square root of 90 can also be represented as:
\( \sqrt{90} = \sqrt{9 \times 10} = \sqrt{9} \times \sqrt{10} = 3 \sqrt{10} \)
- The square root of 90 can also be represented as:
The mathematical representation of \( \sqrt{90} \) in its simplest form is \( 3 \sqrt{10} \), and its approximate decimal value is 9.4868. These representations are crucial for various mathematical operations and practical applications, providing a clear understanding of the square root of 90.
Methods to Calculate Square Root of 90
To calculate the square root of 90, there are several methods available:
- Prime Factorization Method:
First, find the prime factors of 90, which are 2, 3, 3, and 5. Then, pair the factors to get √(2 * 3 * 3 * 5). This simplifies to 3√10.
- Long Division Method:
Estimate the square root of 90 (approximately 9.49). Divide 90 by an estimated value closer to the actual root, square the result, adjust the estimate, and repeat until sufficiently accurate.
- Approximation Method:
Use iterative methods like Newton's method or the Babylonian method to approximate the square root of 90.

Prime Factorization Method
- Identify the prime factors of 90: 2, 3, and 5.
- Group the prime factors in pairs inside the square root: √(2 * 3 * 5).
- Simplify the expression: √(2 * 3 * 5) = √(30).
- Therefore, √90 = √(2 * 3 * 5) = √30.
Long Division Method
The long division method is a manual technique for finding the square root of a number, and it can be particularly useful for approximating the square root of numbers that are not perfect squares, such as 90. Here is a step-by-step guide to finding the square root of 90 using the long division method:
-
Start by grouping the digits of the number in pairs, starting from the decimal point and moving both to the left and to the right. For 90, we consider it as 90.00 for ease of calculation:
90.00
-
Find the largest number whose square is less than or equal to the first pair. In this case, the first pair is 90. The largest number whose square is less than 90 is 9, because \(9^2 = 81\).
Write 9 as the first digit of the square root. Subtract 81 from 90 to get the remainder 9.
9 9 | 90 -81 09 -
Bring down the next pair of digits (00) to the right of the remainder, making it 900. Double the number already found in the quotient (9), which gives 18. This 18 is used as the starting part of the new divisor.
Now, we need to find a digit X such that 18X multiplied by X is less than or equal to 900. The suitable digit here is 4, because \(184 \times 4 = 736\).
Write 4 as the next digit of the square root. Subtract 736 from 900 to get the remainder 164.
94 9 | 9000 -736 1640 -
Bring down the next pair of zeros (00) to the right of the remainder, making it 16400. Double the number already found in the quotient (94), which gives 188. This 188 is used as the starting part of the new divisor.
Now, we need to find a digit Y such that 188Y multiplied by Y is less than or equal to 16400. The suitable digit here is 8, because \(1888 \times 8 = 15104\).
Write 8 as the next digit of the square root. Subtract 15104 from 16400 to get the remainder 1296.
948 9 | 1640000 -151040 129600 -
Continue this process to find more decimal places of the square root, if necessary. For most practical purposes, a few decimal places are sufficient.
Therefore, the approximate square root of 90 using the long division method is 9.486.
Approximation Method
To approximate the square root of 90, we can use a few different methods. One of the most common methods is the iterative approximation method, such as the Babylonian method (also known as Heron's method). Here's a step-by-step guide:
- Choose an Initial Guess: Start with an initial guess that is close to the actual square root. For example, since the square root of 81 is 9 and the square root of 100 is 10, we can start with a guess of 9.5.
- Apply the Iteration Formula: Use the formula:
\[
x_{n+1} = \frac{x_n + \frac{90}{x_n}}{2}
\]
where \(x_n\) is the current guess. - First Iteration: Substitute the initial guess (9.5) into the formula:
\[
x_1 = \frac{9.5 + \frac{90}{9.5}}{2} = \frac{9.5 + 9.4737}{2} = \frac{18.9737}{2} = 9.48685
\] - Second Iteration: Use the result from the first iteration (9.48685):
\[
x_2 = \frac{9.48685 + \frac{90}{9.48685}}{2} \approx 9.486832981
\] - Continue Iterating: Repeat the process until the desired level of accuracy is achieved. For practical purposes, two or three iterations are often sufficient.
After several iterations, you will find that the value converges to approximately 9.486832980505138. Therefore, the square root of 90 is approximately 9.487 when rounded to three decimal places.
Another method to approximate the square root of 90 is to use a calculator or a numerical approximation tool, which can quickly give a precise result.
Properties of Square Roots
The square root of a number has several interesting properties. Understanding these properties can help in various mathematical calculations and problem-solving scenarios. Here are some key properties of square roots:
- Non-Negative Result: The square root of a non-negative number is also non-negative. For any real number \( x \geq 0 \), the square root \( \sqrt{x} \) is always non-negative.
- Product Property: The square root of a product is the product of the square roots. For any non-negative numbers \( a \) and \( b \), \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \).
- Quotient Property: The square root of a quotient is the quotient of the square roots. For any non-negative numbers \( a \) and \( b \) (with \( b \neq 0 \)), \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
- Square of a Square Root: The square of the square root of a number returns the original number. For any non-negative number \( x \), \( (\sqrt{x})^2 = x \).
- Rational and Irrational Results: If the original number is a perfect square, its square root is rational. If the original number is not a perfect square, its square root is irrational. For example, \( \sqrt{90} \) is irrational because 90 is not a perfect square.
In mathematical notation using MathJax, these properties can be expressed as:
- \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
- \((\sqrt{x})^2 = x\)
These properties are fundamental to understanding and working with square roots in various mathematical contexts. They simplify calculations and provide a basis for more advanced mathematical operations involving square roots.
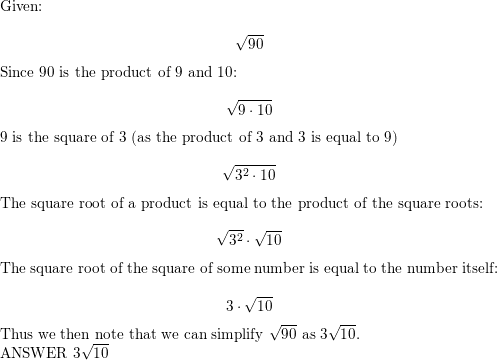
Rational and Irrational Numbers
Understanding the concepts of rational and irrational numbers is fundamental in mathematics, especially when dealing with square roots.
Rational Numbers
A rational number is any number that can be expressed as the quotient or fraction of two integers, where the numerator is an integer and the denominator is a non-zero integer. In other words, if a number can be written in the form \(\frac{a}{b}\) where \(a\) and \(b\) are integers and \(b \neq 0\), then it is a rational number.
- Examples of rational numbers include \(\frac{1}{2}\), \(\frac{-3}{4}\), and \(5\) (since \(5 = \frac{5}{1}\)).
- Rational numbers can have terminating or repeating decimal expansions.
Irrational Numbers
An irrational number, on the other hand, cannot be expressed as a simple fraction. Irrational numbers have non-terminating and non-repeating decimal expansions. They cannot be written as the ratio of two integers.
- Examples of irrational numbers include \(\pi\) (pi), \(e\) (Euler's number), and \(\sqrt{2}\).
- These numbers cannot be precisely written as fractions.
Is the Square Root of 90 Rational or Irrational?
The square root of 90, denoted as \(\sqrt{90}\), is an irrational number. This conclusion can be drawn by understanding that:
- The square root of a non-perfect square is always irrational. Since 90 is not a perfect square, \(\sqrt{90}\) cannot be a rational number.
- When calculated, \(\sqrt{90}\) is approximately 9.4868329805051. This number has a non-terminating and non-repeating decimal expansion, which is a hallmark of irrational numbers.
Therefore, \(\sqrt{90}\) is an irrational number because it cannot be written as a fraction of two integers, and its decimal form does not terminate or repeat.
Summary
To summarize:
- Rational numbers can be written as fractions and have terminating or repeating decimals.
- Irrational numbers cannot be written as fractions and have non-terminating, non-repeating decimals.
- The square root of 90 is irrational as it cannot be expressed as a fraction and has a non-terminating, non-repeating decimal expansion.
Is the Square Root of 90 Rational or Irrational?
The square root of a number is a value that, when multiplied by itself, gives the original number. In the case of the square root of 90, we need to determine whether this value is a rational or irrational number.
Understanding Rational and Irrational Numbers
- Rational Numbers: A rational number is any number that can be expressed as the quotient or fraction of two integers, where the denominator is not zero. Rational numbers have either terminating or repeating decimal expansions. For example, \(\frac{1}{2}\) and 0.75 are rational numbers.
- Irrational Numbers: An irrational number cannot be expressed as a simple fraction. These numbers have non-terminating and non-repeating decimal expansions. Examples include \(\pi\) (pi) and \(\sqrt{2}\).
Determining the Nature of \(\sqrt{90}\)
To determine whether \(\sqrt{90}\) is rational or irrational, we can follow these steps:
- Prime Factorization: First, let's find the prime factors of 90. We have:
90 = 2 × 3² × 5
- Check for Perfect Square: A number is a perfect square if all the exponents in its prime factorization are even. Here, the factorization of 90 shows that 90 is not a perfect square because the exponents are not all even.
- Decimal Expansion: Calculating \(\sqrt{90}\) gives us approximately 9.48683298050514, which is a non-terminating and non-repeating decimal.
Since 90 is not a perfect square, and \(\sqrt{90}\) has a non-terminating and non-repeating decimal expansion, it follows that \(\sqrt{90}\) cannot be expressed as a fraction of two integers.
Conclusion
Based on the above analysis, we can conclude that the square root of 90 is an irrational number. It cannot be expressed as a simple fraction, and its decimal representation does not terminate or repeat.
In summary:
- \(\sqrt{90}\) ≈ 9.48683298050514
- \(\sqrt{90}\) is not a perfect square.
- \(\sqrt{90}\) is an irrational number.
Simplifying Square Roots
Simplifying square roots involves expressing the square root in its simplest radical form. To simplify the square root of a number, follow these steps:
-
Identify the prime factors of the number under the square root.
- For example, the prime factors of 90 are 2, 3, 3, and 5.
-
Group the prime factors into pairs of equal factors.
- 90 can be written as \( 2 \times 3^2 \times 5 \).
-
Take one factor out of each pair and place it outside the radical.
- Since \( 3^2 \) is a pair, it comes out as 3.
-
Multiply the factors outside the radical and leave the remaining factors inside.
- This gives us \( 3\sqrt{10} \).
Therefore, the simplified form of \( \sqrt{90} \) is \( 3\sqrt{10} \).
Here are more examples of simplifying square roots:
Example 1: Simplify \( \sqrt{72} \)
- Prime factors of 72: \( 2^3 \times 3^2 \)
- Group into pairs: \( (2^2) \times (3^2) \times 2 \)
- Simplify: \( 2 \times 3 \sqrt{2} = 6\sqrt{2} \)
Example 2: Simplify \( \sqrt{50} \)
- Prime factors of 50: \( 2 \times 5^2 \)
- Group into pairs: \( (5^2) \times 2 \)
- Simplify: \( 5\sqrt{2} \)
Understanding how to simplify square roots is essential for solving many algebraic problems, making calculations more manageable and results more precise.
How to Simplify the Square Root of 90
Simplifying the square root of 90 involves breaking down the number into its prime factors and then applying the property of square roots that allows for simplification. Follow the detailed steps below:
- Find the Prime Factorization of 90
First, express 90 as a product of its prime factors:
- 90 ÷ 2 = 45
- 45 ÷ 3 = 15
- 15 ÷ 3 = 5
- 5 is a prime number
So, the prime factorization of 90 is \( 2 \times 3^2 \times 5 \).
- Group the Prime Factors
Group the prime factors in pairs where possible:
\( 90 = 2 \times 3^2 \times 5 \)
- Simplify Using the Square Root Property
Apply the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \):
\( \sqrt{90} = \sqrt{2 \times 3^2 \times 5} \)
Separate the perfect square (in this case, \( 3^2 \)):
\( \sqrt{90} = \sqrt{2} \times \sqrt{3^2} \times \sqrt{5} \)
Simplify the square root of the perfect square:
\( \sqrt{90} = \sqrt{2} \times 3 \times \sqrt{5} \)
Therefore:
\( \sqrt{90} = 3 \sqrt{10} \)
The simplified form of the square root of 90 is \( 3 \sqrt{10} \).

Practical Applications of Square Roots
Square roots have numerous practical applications in various fields, including mathematics, physics, engineering, and everyday life. Here are some key examples of how square roots are used:
-
Geometry and Area Calculation
Square roots are fundamental in geometry, especially when calculating the side length of a square given its area. For example, if the area of a square is \( A \) square units, the length of one side is \( \sqrt{A} \) units. This principle is applied in various construction and design projects.
-
Distance Measurement
In both 2D and 3D spaces, the distance between two points can be calculated using the Pythagorean theorem, which involves square roots. For example, the distance \( D \) between two points \((x_1, y_1)\) and \((x_2, y_2)\) in a 2-dimensional plane is given by:
\[
D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}
\]This formula is essential in fields such as navigation, physics, and computer graphics.
-
Quadratic Equations
Square roots are also crucial in solving quadratic equations, which appear in various scientific and engineering problems. The solutions to a quadratic equation \( ax^2 + bx + c = 0 \) are found using the quadratic formula:
\[
x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}
\] -
Physics: Free Fall and Gravity
Square roots are used to calculate the time it takes for an object to fall from a certain height under gravity. For instance, the time \( t \) in seconds for an object to fall from a height \( h \) feet is given by:
\[
t = \frac{\sqrt{h}}{4}
\]This formula helps in understanding motion and designing experiments related to free fall.
-
Accident Investigation
In forensic science, particularly in accident investigations, the length of skid marks can be used to estimate the speed of a vehicle before it stopped. The speed \( v \) in miles per hour can be calculated from the skid mark length \( d \) using the formula:
\[
v = \sqrt{24d}
\] -
Design and Architecture
Square roots are used to determine dimensions in design and architecture. For example, if a square garden has an area of 90 square yards, the length of each side is \( \sqrt{90} \approx 9.5 \) yards.
Understanding and applying square roots is crucial in solving real-world problems across different domains, making them an essential mathematical tool.
Use of Square Roots in Real Life
Square roots have a wide range of applications in real life, often appearing in various fields such as geometry, physics, engineering, and everyday problem-solving. Here are some detailed examples:
-
Geometry:
In geometry, square roots are used to determine the lengths of sides in right triangles. For example, using the Pythagorean Theorem, the length of the hypotenuse of a right triangle can be found if the lengths of the other two sides are known. The formula is:
\[ c = \sqrt{a^2 + b^2} \]
where \( c \) is the hypotenuse, and \( a \) and \( b \) are the other two sides.
-
Physics:
In physics, square roots are used to calculate quantities like the time it takes for an object to fall to the ground under the influence of gravity. For an object dropped from a height \( h \), the time \( t \) it takes to reach the ground is given by:
\[ t = \frac{\sqrt{h}}{4} \]
For example, if an object is dropped from a height of 64 feet, it takes:
\[ t = \frac{\sqrt{64}}{4} = \frac{8}{4} = 2 \text{ seconds} \]
-
Engineering:
Square roots are essential in engineering for analyzing forces, stresses, and strains in materials. For example, the stress \( \sigma \) in a beam under load can be related to the square root of its cross-sectional area \( A \):
\[ \sigma = \frac{F}{\sqrt{A}} \]
where \( F \) is the force applied.
-
Everyday Problem-Solving:
Square roots are also used in everyday situations, such as finding the side length of a square given its area. If the area \( A \) of a square is known, the side length \( s \) can be found using:
\[ s = \sqrt{A} \]
For instance, if a square has an area of 200 square feet, the length of each side is approximately:
\[ s = \sqrt{200} \approx 14.1 \text{ feet} \]
-
Accident Investigation:
Police use square roots to determine the speed of vehicles before an accident by analyzing the length of skid marks. If \( d \) is the length of the skid marks, the speed \( v \) can be estimated using:
\[ v = \sqrt{24d} \]
For example, if skid marks are 190 feet long, the speed of the vehicle was approximately:
\[ v = \sqrt{24 \times 190} \approx 67.5 \text{ mph} \]
These examples illustrate the versatility and importance of square roots in various practical applications, making them a fundamental concept in both academic and real-world contexts.
Square Roots in Geometry
The concept of square roots is fundamental in geometry, playing a crucial role in various geometric constructions and theorems. One of the most well-known applications of square roots in geometry is through the Pythagorean Theorem.
The Pythagorean Theorem
The Pythagorean Theorem states that in a right-angled triangle, the square of the length of the hypotenuse (\(c\)) is equal to the sum of the squares of the lengths of the other two sides (\(a\) and \(b\)). This can be written as:
\[ c^2 = a^2 + b^2 \]
To find the length of the hypotenuse when the lengths of the other two sides are known, we take the square root of the sum of the squares of these sides:
\[ c = \sqrt{a^2 + b^2} \]
Example with the Square Root of 90
Consider a right-angled triangle where one side is of length 9 and the other side is of length \(\sqrt{90}\). To find the hypotenuse, we use the Pythagorean Theorem:
\[ c^2 = 9^2 + (\sqrt{90})^2 \]
Since \((\sqrt{90})^2 = 90\), the equation becomes:
\[ c^2 = 81 + 90 \]
\[ c^2 = 171 \]
Therefore, the length of the hypotenuse \(c\) is:
\[ c = \sqrt{171} \]
Square Roots in Coordinate Geometry
Square roots are also essential in coordinate geometry, particularly when calculating distances between points in the Cartesian plane. The distance formula, which is derived from the Pythagorean Theorem, is given by:
\[ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
This formula calculates the distance \(d\) between two points \((x_1, y_1)\) and \((x_2, y_2)\). For example, if the points are \((0, 0)\) and \((9, \sqrt{90})\), the distance is:
\[ d = \sqrt{(9 - 0)^2 + (\sqrt{90} - 0)^2} \]
\[ d = \sqrt{81 + 90} \]
\[ d = \sqrt{171} \]
Geometric Constructions
Square roots also appear in geometric constructions. For instance, the construction of a square with a given area involves using the square root of that area to determine the length of each side. If we want to construct a square with an area of 90 square units, the length of each side will be:
\[ s = \sqrt{90} \approx 9.49 \text{ units} \]
Conclusion
Understanding square roots is essential for solving many geometric problems. Whether through the Pythagorean Theorem, distance calculations in coordinate geometry, or geometric constructions, the square root function helps simplify and solve various geometric tasks.
Common Misconceptions about Square Roots
Understanding square roots can sometimes lead to misconceptions and errors. Here are some common misunderstandings and clarifications to help improve comprehension:
- Misconception: All square roots are positive.
Clarification: By definition, the square root symbol (√) represents the principal (positive) square root. However, every positive number actually has two square roots: a positive and a negative one. For example, both 3 and -3 are square roots of 9.
- Misconception: The square root of a negative number is undefined.
Clarification: While the square root of a negative number is not a real number, it is defined in the realm of complex numbers. The square root of -1 is denoted as i, the imaginary unit, so the square root of any negative number can be expressed as a multiple of i.
- Misconception: \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\) always holds true.
Clarification: This property holds true for non-negative numbers \(a\) and \(b\), but fails for negative numbers due to the involvement of complex numbers. For example, \(\sqrt{-4 \cdot -9} \neq \sqrt{-4} \cdot \sqrt{-9}\).
- Misconception: \(\sqrt{a^2} = a\) for all \(a\).
Clarification: This is true only for non-negative \(a\). For negative \(a\), \(\sqrt{a^2} = |a|\), the absolute value of \(a\). For example, \(\sqrt{(-5)^2} = 5\), not -5.
- Misconception: Rational numbers have rational square roots.
Clarification: Not all rational numbers have rational square roots. For example, \(\sqrt{2}\) is an irrational number even though 2 is rational.
- Misconception: The principal square root of 0 is undefined.
Clarification: The principal square root of 0 is defined and is 0 itself since \(0^2 = 0\).
By understanding these common misconceptions, learners can avoid errors and gain a clearer understanding of square roots and their properties.

Conclusion
The exploration of the square root of 90 offers a comprehensive understanding of not only the value itself but also the methods and principles behind calculating square roots in general. The square root of 90, which is approximately 9.4868, demonstrates the intricate relationship between numbers and their roots, lying between the perfect squares of 81 and 100.
In this guide, we have covered various methods to calculate the square root of 90, including prime factorization, long division, and approximation techniques. Each method has its unique steps and benefits, showcasing the versatility of mathematical approaches.
Additionally, we have discussed the properties of square roots, distinguishing between rational and irrational numbers, and confirmed that the square root of 90 is irrational. Simplification of square roots was also addressed, providing clarity on how to express the square root of 90 in its simplest radical form as \(3\sqrt{10}\).
Real-life applications of square roots were highlighted, particularly in geometry, where the square root of a number often plays a crucial role in solving problems related to areas and distances. Understanding square roots is essential for various fields, including engineering, physics, and computer science, where precise calculations are imperative.
We have also addressed common misconceptions about square roots, ensuring a clear and accurate comprehension of this fundamental concept. By debunking myths and providing factual explanations, learners can confidently approach problems involving square roots.
In conclusion, the square root of 90 serves as a valuable example in the broader context of mathematical learning. Mastery of this concept enhances problem-solving skills and supports the application of mathematics in practical scenarios. Continued practice and exploration of square roots will undoubtedly enrich one's mathematical knowledge and proficiency.
Cách đơn giản hóa căn bậc hai của 90 | Root 90
Hướng dẫn đơn giản hóa căn bậc hai của 90 | Root 90 | Video
READ MORE:
Hướng dẫn căn bậc hai của 90 | Video
Hướng dẫn căn bậc hai của 90 | Video