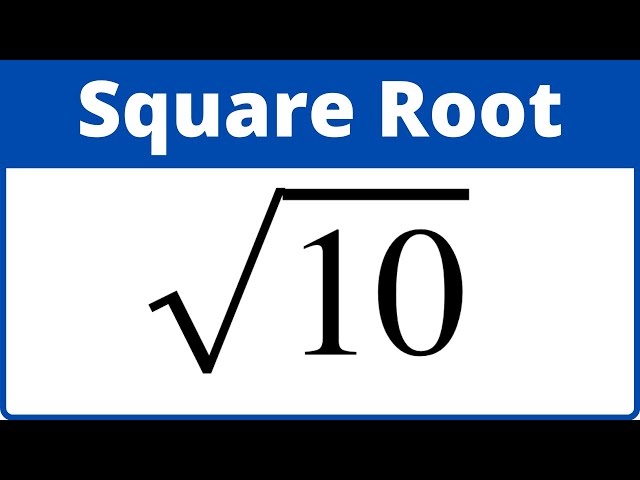
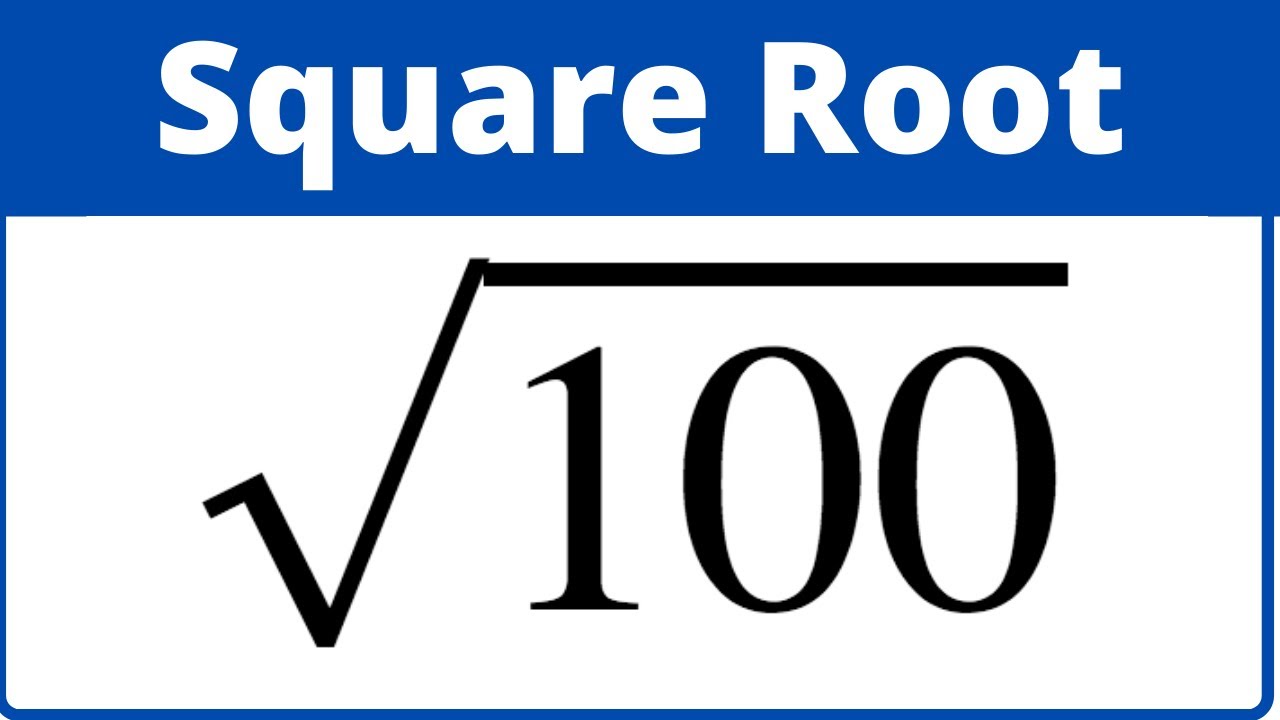
Topic square root of 100: Discover the fascinating world of square roots with a focus on the square root of 100. This guide delves into the mathematical principles, practical applications, and intriguing facts surrounding this perfect square. Whether you're a math enthusiast or simply curious, you'll find valuable insights and easy-to-understand explanations.
Table of Content
- Square Root of 100
- Introduction to Square Roots
- Understanding the Concept of Square Roots
- What is the Square Root of 100?
- Mathematical Definition and Explanation
- Methods to Calculate Square Roots
- Using Prime Factorization
- Long Division Method
- Using a Calculator
- Properties of Square Roots
- Perfect Squares and Their Roots
- Applications of Square Roots in Real Life
- Square Roots in Geometry
- Square Roots in Algebra
- Common Misconceptions About Square Roots
- Fun Facts About Square Roots
- Conclusion
- YOUTUBE:
Square Root of 100
The square root of 100 is represented as \( \sqrt{100} \).
Here are some facts:
- The square root of 100 equals 10.
- Mathematically, \( \sqrt{100} = 10 \).
- 100 is a perfect square because \( 10 \times 10 = 100 \).
In summary, \( \sqrt{100} = 10 \).
READ MORE:
Introduction to Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. In mathematical notation, if x is the square root of y, then \( x^2 = y \).
For example, the square root of 100 is a number which, when multiplied by itself, equals 100. This can be expressed as:
\[ \sqrt{100} = 10 \]
Here’s a step-by-step explanation of square roots:
- Identify the number: Start with the number for which you want to find the square root. In this case, it is 100.
- Determine if it's a perfect square: Check if the number is a perfect square. 100 is a perfect square because \( 10 \times 10 = 100 \).
- Find the root: Calculate the square root by finding the number that, when squared, gives the original number. For 100, the square root is 10.
Square roots are fundamental in various areas of mathematics, including algebra, geometry, and calculus. They are essential for solving quadratic equations, understanding geometric properties, and analyzing scientific data.
Below is a simple table illustrating some perfect squares and their square roots:
| Number | Square Root |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
| 36 | 6 |
| 49 | 7 |
| 64 | 8 |
| 81 | 9 |
| 100 | 10 |
Understanding square roots helps build a strong foundation for further mathematical learning and application.
Understanding the Concept of Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, yields the original number. The square root of a number n is denoted as \( \sqrt{n} \).
For instance, the square root of 100 can be expressed as:
\[ \sqrt{100} = 10 \]
This means that \( 10 \times 10 = 100 \).
Here’s a detailed step-by-step guide to understanding square roots:
- Identify the Number: Start with the number you want to find the square root of. For example, consider 100.
- Definition: The square root of a number n is a value m such that \( m^2 = n \). Therefore, \( 10^2 = 100 \).
- Perfect Squares: Some numbers are perfect squares, meaning their square roots are whole numbers. For example, 100, 81, 64, and 49 are perfect squares.
- Properties of Square Roots:
- Non-negative: Square roots are always non-negative because a negative number multiplied by itself gives a positive result. Thus, \( \sqrt{100} = 10 \) and not -10.
- Unique Positive Root: Each positive number has a unique positive square root. For 100, it is 10.
Square roots have various applications in different fields, including geometry, physics, and engineering. For instance, they are used to determine the dimensions of squares and to solve quadratic equations.
Here is a table summarizing some common numbers and their square roots:
| Number | Square Root |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
| 36 | 6 |
| 49 | 7 |
| 64 | 8 |
| 81 | 9 |
| 100 | 10 |
By grasping the concept of square roots, students and enthusiasts can better appreciate their significance and utility in solving mathematical problems.
What is the Square Root of 100?
The square root of 100 is a number which, when multiplied by itself, equals 100. In mathematical notation, this is expressed as:
\[ \sqrt{100} = 10 \]
This can be explained in several steps:
- Understanding Square Roots: The square root of a number n is a value m such that \( m^2 = n \). For 100, this means finding a number m where \( m \times m = 100 \).
- Perfect Squares: Recognize that 100 is a perfect square. A perfect square is an integer that is the square of another integer. Here, 100 is the square of 10, since \( 10 \times 10 = 100 \).
- Calculation: Calculate the square root by finding the number that, when squared, results in 100. The calculation is straightforward:
\[ 10 \times 10 = 100 \]
- Verification: Verify the result by squaring the obtained square root:
\[ 10^2 = 100 \]
This confirms that the square root of 100 is indeed 10.
The concept of square roots is fundamental in mathematics, with the square root of 100 serving as a classic example due to its simplicity and the fact that 100 is a perfect square.
Here is a table illustrating the square roots of some other perfect squares:
| Number | Square Root |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
| 36 | 6 |
| 49 | 7 |
| 64 | 8 |
| 81 | 9 |
| 100 | 10 |
Knowing the square root of 100 helps in various mathematical applications, including simplifying expressions, solving equations, and understanding geometric properties.
Mathematical Definition and Explanation
The square root of a number is a value that, when multiplied by itself, yields the original number. Mathematically, if n is the square root of x, then:
\[ n^2 = x \]
For the number 100, its square root is denoted as:
\[ \sqrt{100} = 10 \]
This implies that:
\[ 10 \times 10 = 100 \]
Here’s a detailed explanation of the mathematical definition and process:
- Definition of Square Roots: The square root of a number x is a value n such that \( n^2 = x \). This value n is called the principal square root and is always non-negative.
- Notation: The square root of a number is represented using the radical symbol \( \sqrt{} \). For example, \( \sqrt{100} \) represents the square root of 100.
- Perfect Squares: A perfect square is an integer that can be expressed as the square of another integer. In this case, 100 is a perfect square because \( 10^2 = 100 \).
- Finding Square Roots: To find the square root of a number, determine the value that, when multiplied by itself, equals the original number. For 100, this value is 10, since \( 10 \times 10 = 100 \).
- Properties of Square Roots:
- Non-negativity: The principal square root is always non-negative. For 100, the principal square root is 10, not -10.
- Uniqueness: Every non-negative number has a unique non-negative square root. Thus, \( \sqrt{100} = 10 \) is unique.
- Multiplicative Property: The square root of a product is equal to the product of the square roots of the factors. For example, \( \sqrt{100} = \sqrt{10 \times 10} = \sqrt{10} \times \sqrt{10} = 10 \).
Here is a table summarizing some properties and examples:
| Number | Square Root | Verification |
| 1 | 1 | \( 1 \times 1 = 1 \) |
| 4 | 2 | \( 2 \times 2 = 4 \) |
| 9 | 3 | \( 3 \times 3 = 9 \) |
| 16 | 4 | \( 4 \times 4 = 16 \) |
| 25 | 5 | \( 5 \times 5 = 25 \) |
| 36 | 6 | \( 6 \times 6 = 36 \) |
| 49 | 7 | \( 7 \times 7 = 49 \) |
| 64 | 8 | \( 8 \times 8 = 64 \) |
| 81 | 9 | \( 9 \times 9 = 81 \) |
| 100 | 10 | \( 10 \times 10 = 100 \) |
Understanding the mathematical definition and properties of square roots is essential for solving various mathematical problems and for applying these concepts in real-world scenarios.

Methods to Calculate Square Roots
Calculating square roots can be done using various methods, each suitable for different levels of accuracy and complexity. Here are some common methods to calculate the square root of a number, including the square root of 100:
1. Prime Factorization Method
- Identify the number: Start with the number you want to find the square root of. For example, 100.
- Factorize the number: Break down 100 into its prime factors:
\[ 100 = 2^2 \times 5^2 \]
- Apply the square root: Take the square root of each factor:
\[ \sqrt{100} = \sqrt{2^2 \times 5^2} = 2 \times 5 = 10 \]
2. Long Division Method
- Set up the division: Write 100 as a long division problem.
- Group the digits: Pair the digits from right to left (100 becomes 1 | 00).
- Find the largest square: Identify the largest number whose square is less than or equal to the first group (1). This is 1.
- Subtract and bring down: Subtract 1 squared (1) from 1 and bring down the next group of digits (00), making it 00.
- Double the quotient: Double the current quotient (1) to get 2. Determine how many times 2 fits into the new dividend without exceeding it. The answer is 5 (since 25 is the closest square to 100).
- Complete the division: Multiply 5 by 25 to get 100. Subtract to find the remainder is 0. Thus, the quotient is 10.
3. Using a Calculator
- Turn on the calculator: Ensure your calculator is in standard or scientific mode.
- Enter the number: Type 100 into the calculator.
- Press the square root button: This is usually denoted by \( \sqrt{} \). The calculator will display the result:
\[ \sqrt{100} = 10 \]
4. Estimation Method
- Find the nearest perfect squares: Identify perfect squares near 100. These are 81 (9) and 121 (11).
- Estimate the root: Since 100 is closer to 121 than to 81, the square root of 100 is closer to 10 than to 9. Thus, \( \sqrt{100} \approx 10 \).
Each method has its advantages, whether for mental math, detailed calculation, or quick results. Understanding these methods provides flexibility in calculating square roots depending on the context and tools available.
Using Prime Factorization
Prime factorization is a method used to express a number as the product of its prime factors. This method can also be used to find the square root of a number, such as 100. Here’s a detailed step-by-step guide to using prime factorization to calculate the square root of 100:
- Identify the number: Start with the number you want to find the square root of, which in this case is 100.
- Factorize the number: Break down 100 into its prime factors. This involves dividing the number by the smallest prime number and continuing the process with the quotient until all factors are prime numbers.
- 100 is divisible by 2 (the smallest prime number):
\[ 100 \div 2 = 50 \]
- 50 is divisible by 2:
\[ 50 \div 2 = 25 \]
- 25 is divisible by 5 (the next smallest prime number):
\[ 25 \div 5 = 5 \]
- 5 is a prime number and is divisible by itself:
\[ 5 \div 5 = 1 \]
Thus, the prime factorization of 100 is:
\[ 100 = 2^2 \times 5^2 \]
- 100 is divisible by 2 (the smallest prime number):
- Group the factors: Pair the prime factors in groups of two. Each pair represents a square of a prime factor:
\[ 100 = (2 \times 2) \times (5 \times 5) \]
- Take the square root: To find the square root, take one factor from each pair:
\[ \sqrt{100} = \sqrt{2^2 \times 5^2} = 2 \times 5 \]
- Multiply the factors: Multiply the results to get the final answer:
\[ 2 \times 5 = 10 \]
Therefore, the square root of 100 using prime factorization is:
\[ \sqrt{100} = 10 \]
This method is particularly useful for finding the square roots of large numbers or numbers that are not immediately recognizable as perfect squares. It reinforces the understanding of prime numbers and their role in the factorization process.
Long Division Method
The Long Division Method is a traditional technique used to find the square root of a number. This method involves a step-by-step approach to gradually approximate the square root. Here is how you can use the Long Division Method to find the square root of 100:
- Write the number 100 in pairs of digits from right to left. If the number of digits is odd, the leftmost digit will be a single digit. For 100, we write it as 1 | 00.
- Find the largest number whose square is less than or equal to the first pair (or single digit). In this case, the largest number whose square is less than or equal to 1 is 1 (since 12 = 1). Place this number as the first digit of the quotient. Subtract its square from the first pair and bring down the next pair. This gives us:
- 12 = 1
- 1 - 1 = 0
- Bring down 00, so we now have 00.
- Double the current quotient (which is 1) and write it below. This gives us 2.
- Now find a digit (X) such that 2X * X is less than or equal to the number we have (which is 00). The digit that satisfies this condition is 0 (since 20 * 0 = 0).
- Place this digit (0) next to the current quotient (making it 10) and also next to 2 (making it 20). Subtract 20 * 0 from the number we have:
- 20 * 0 = 0
- 00 - 0 = 0
- The quotient we have now is 10, and since there are no more digits to bring down, we conclude that the square root of 100 is 10.
Thus, using the Long Division Method, we find that the square root of 100 is 10.
Using a Calculator
Calculating the square root of a number using a calculator is straightforward. Here's a detailed step-by-step guide on how to do it:
- Turn on your calculator: Ensure that your calculator is turned on and functioning properly.
- Enter the number: Type in the number for which you want to find the square root. In this case, enter
100. - Locate the square root function: Find the square root button on your calculator. This button is usually labeled as
√orsqrt. - Execute the calculation: Press the square root button. The calculator will then display the square root of the number you entered. For
100, you should see10.
Here's an example using the steps outlined above:
- Turn on the calculator.
- Enter
100. - Press the
√button. - The display should show
10.
For calculators without a dedicated square root button, you can use the exponentiation method:
- Enter
100. - Use the exponentiation function, usually labeled as
^oryx. - Enter
0.5, which represents the square root (since1000.5is the same as√100). - Press the equals button (
=) to get the result.
Additionally, you can use online calculators for convenience. Websites like CalculatorSoup and Symbolab provide easy-to-use square root calculators where you simply input the number, and the website calculates the square root for you.
For example, to find the square root of 100 using an online calculator:
- Visit a square root calculator website.
- Enter
100in the input field. - Click the calculate button to get the result, which should be
10.

Properties of Square Roots
The square root has several fundamental properties that make it a crucial concept in mathematics. Here are the key properties:
-
Product Rule: The square root of a product is the product of the square roots of each factor.
Mathematically, this is expressed as:
\[\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\]
Example: \(\sqrt{36} = \sqrt{4 \cdot 9} = \sqrt{4} \cdot \sqrt{9} = 2 \cdot 3 = 6\)
-
Quotient Rule: The square root of a quotient is the quotient of the square roots of the numerator and the denominator.
Mathematically, this is expressed as:
\[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]
Example: \(\sqrt{\frac{25}{4}} = \frac{\sqrt{25}}{\sqrt{4}} = \frac{5}{2} = 2.5\)
-
Square of a Square Root: The square of a square root returns the original number.
Mathematically, this is expressed as:
\[(\sqrt{a})^2 = a\]
Example: \((\sqrt{7})^2 = 7\)
-
Square Root of a Negative Number: In the realm of real numbers, the square root of a negative number is not defined. However, in the set of complex numbers, it is defined using the imaginary unit \(i\), where \(i^2 = -1\).
Example: \(\sqrt{-9} = 3i\)
These properties are widely used in various mathematical problems and proofs, making the concept of square roots indispensable in algebra and beyond.
Perfect Squares and Their Roots
A perfect square is an integer that can be expressed as the square of another integer. Understanding perfect squares and their roots is fundamental in mathematics, especially when dealing with square roots.
Here are some key perfect squares and their respective square roots:
| Number | Square Root |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
| 36 | 6 |
| 49 | 7 |
| 64 | 8 |
| 81 | 9 |
| 100 | 10 |
These numbers are perfect squares because their square roots are integers. They are useful in various mathematical calculations and applications.
Applications of Square Roots in Real Life
Square roots have numerous practical applications across various fields:
- Engineering: Engineers use square roots in calculations involving power, voltage, and signal processing.
- Physics: In physics, square roots are essential for calculating forces, energy, and wave properties.
- Finance: Financial analysts use square roots in risk assessment, volatility measures, and option pricing.
- Medicine: Medical imaging technologies such as MRI rely on square roots for image reconstruction and analysis.
- Computer Graphics: Square roots are used in computer graphics for calculations involving distances, angles, and transformations.
- Statistics: Statisticians use square roots in standard deviation calculations and error analysis.
- Architecture: Architects use square roots in calculations for structural stability and load distribution.
These applications highlight the versatility and importance of square roots in everyday life and various professional fields.
Square Roots in Geometry
In geometry, the concept of square roots is essential, especially when dealing with the areas of squares. Consider a square with an area of 100 square units. To find the length of its side, which is the square root of the area, we calculate:
\[
\sqrt{100} = 10
\]
This means each side of the square measures 10 units. Square roots are also used in geometry to determine the dimensions of other shapes derived from squares, such as rectangles and right triangles, where one side might be a known square root value.

Square Roots in Algebra
In algebra, understanding square roots is crucial for solving equations involving squares. For instance, to solve the equation \( x^2 = 100 \), we take the square root of both sides:
\[
\sqrt{x^2} = \sqrt{100}
\]
This gives us two solutions: \( x = 10 \) and \( x = -10 \), because both \( 10^2 \) and \( (-10)^2 \) equal 100. Square roots also play a role in simplifying expressions and solving quadratic equations by factoring or using the quadratic formula, where determining whether the discriminant (b² - 4ac) is a perfect square affects the nature of the solutions.
Common Misconceptions About Square Roots
Understanding square roots is fundamental to grasping many mathematical concepts. However, there are several common misconceptions about square roots that can lead to confusion. Let's address and clarify these misconceptions:
-
Misconception 1: Only Positive Numbers Have Square Roots
Many believe that square roots apply only to positive numbers. However, every positive number has both a positive and a negative square root. For example, the square roots of 100 are \( \pm10 \).
In mathematical notation: \( \sqrt{100} = \pm10 \).
-
Misconception 2: The Square Root of a Number is Always Smaller
Some think that the square root of any number is always smaller than the original number. This is true for numbers greater than 1, but for numbers between 0 and 1, the square root is actually larger. For example, \( \sqrt{0.25} = 0.5 \), and \( \sqrt{0.04} = 0.2 \).
-
Misconception 3: Square Roots of Negative Numbers Do Not Exist
While it's true that there are no real square roots of negative numbers, they do exist in the complex number system. For instance, the square root of -1 is represented as \( i \) (the imaginary unit), so \( \sqrt{-1} = i \).
-
Misconception 4: Square Roots Can Be Simplified Indefinitely
Another common misconception is that square roots can always be simplified to a simpler form. While this is true for perfect squares, non-perfect squares cannot be simplified into exact integers. For example, \( \sqrt{2} \) is an irrational number and cannot be simplified further.
-
Misconception 5: The Square Root Function is the Inverse of Squaring
It is often believed that taking the square root of a squared number returns the original number. This is only partially correct because squaring a number and then taking the square root returns the absolute value of the original number. For example, \( \sqrt{(-10)^2} = 10 \), not -10.
Understanding these misconceptions can help build a stronger foundation in mathematics and prevent common errors when working with square roots.
Fun Facts About Square Roots
Square roots are fascinating and have many interesting properties and applications. Here are some fun facts about square roots:
- Historical Significance: The concept of square roots dates back to ancient civilizations, including the Babylonians who had methods to approximate square roots over 3,000 years ago.
- Perfect Squares: A perfect square is a number that is the square of an integer. For example, \(100\) is a perfect square because \(10^2 = 100\).
- Symbol Origin: The symbol for square root, \(\sqrt{\ }\), is believed to have originated from the Latin word "radix," meaning "root." This is why it is also called the radical symbol.
- Irrational Roots: Not all square roots are rational numbers. For instance, the square root of 2 (\(\sqrt{2}\)) is an irrational number, meaning it cannot be expressed as a simple fraction.
- Imaginary Numbers: The square roots of negative numbers are not real numbers. They are called imaginary numbers and involve the imaginary unit \(i\), where \(i = \sqrt{-1}\).
- Geometric Interpretation: Geometrically, the square root of a number can be represented as the length of the side of a square that has an area equal to that number.
- Golden Ratio Connection: The golden ratio, approximately \(1.618\), can be expressed using a nested radical: \(\phi = \sqrt{1 + \sqrt{1 + \sqrt{1 + \ldots}}}\).
These facts highlight the depth and beauty of mathematics through the lens of square roots, showcasing their relevance from ancient times to modern mathematical concepts.
Conclusion
The exploration of the square root of 100 has provided a comprehensive understanding of this fundamental mathematical concept. By examining various methods to calculate square roots, such as prime factorization, the long division method, and the use of calculators, we have demonstrated the different approaches to finding the square root of a number.
The square root of 100, which is 10, serves as an excellent example of a perfect square. This straightforward result highlights the simplicity of dealing with perfect squares, where the square root is always a whole number.
Additionally, we delved into the properties and applications of square roots in real life, illustrating their significance in fields such as geometry and algebra. From calculating distances in geometry to solving quadratic equations in algebra, the utility of square roots extends across various mathematical disciplines.
Understanding the square root of 100 also helps in dispelling common misconceptions, such as the belief that square roots are always complex or irrational numbers. As we have seen, the square root of a perfect square like 100 is a rational number, further reinforcing the clarity and consistency of mathematical principles.
In conclusion, mastering the concept of square roots, exemplified by the square root of 100, is essential for building a strong foundation in mathematics. It not only enhances problem-solving skills but also fosters a deeper appreciation for the logical structure of mathematical reasoning.

Căn bậc hai của 100: Hướng dẫn chi tiết
READ MORE:
Làm thế nào để đơn giản hóa căn bậc hai của 100: sqrt(100)