Topic square root upto 100: Discover the fascinating world of square roots up to 100 in this comprehensive article. Learn about their calculations, practical uses in mathematics, and historical context. Whether you're a student or a math enthusiast, delve into the significance and methods behind these fundamental mathematical entities.
Table of Content
- Square Roots up to 100
- Table of Contents
- Introduction
- Basic Concepts
- Calculation Methods
- List of Square Roots up to 100
- Applications in Mathematics
- Historical Significance
- Conclusion
- YOUTUBE: Học cách ghi nhớ các số bình phương từ 1 đến 25 một cách dễ dàng và nhanh chóng bằng phương pháp liên kết. Mẹo toán học giúp bạn tính nhẩm hiệu quả hơn.
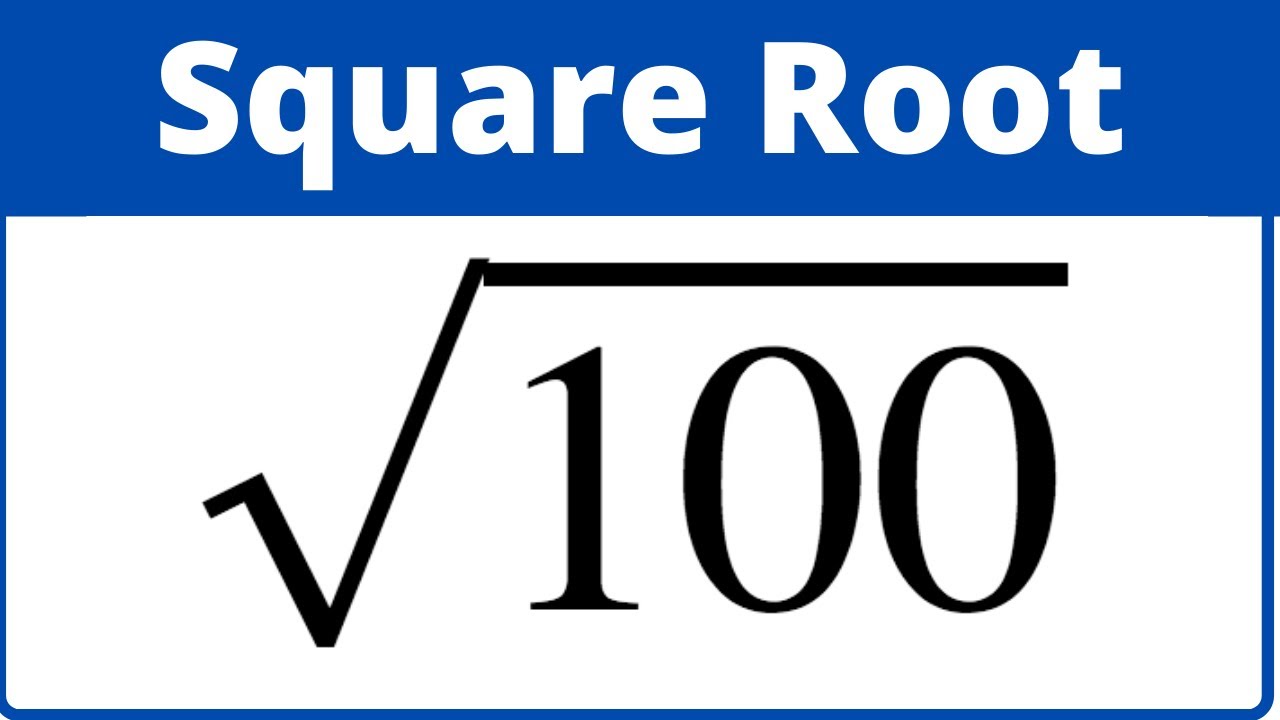
Square Roots up to 100
Here are the square roots of numbers from 1 to 100:
| Number | Square Root |
| 1 | 1.000 |
| 2 | 1.414 |
| 3 | 1.732 |
| 4 | 2.000 |
| 5 | 2.236 |
| 6 | 2.449 |
| 7 | 2.646 |
| 8 | 2.828 |
| 9 | 3.000 |
| 10 | 3.162 |
| ... | ... |
| 99 | 9.950 |
| 100 | 10.000 |

READ MORE:
Table of Contents
In this article, explore the comprehensive coverage of square roots up to 100:
- Introduction
- Overview of square roots and their importance in mathematics.
- Basic Concepts
- Definition and properties of square roots.
- Explanation of how square roots are calculated.
- Calculation Methods
- Methods for calculating square roots manually.
- Use of calculators and software for accurate calculations.
- List of Square Roots up to 100
- Complete list of square roots from 1 to 100.
- Numerical values and their mathematical significance.
- Applications in Mathematics
- Practical applications of square roots in various mathematical problems.
- Examples illustrating real-world uses of square roots.
- Historical Significance
- Historical development and evolution of the concept of square roots.
- Contributions of ancient and modern mathematicians.
- Conclusion
- Summary of key points covered in the article.
- Final thoughts on the importance of understanding square roots up to 100.
Introduction
Welcome to the exploration of square roots up to 100, a fundamental concept in mathematics that offers insights into numerical relationships and calculations. Understanding square roots is crucial for various applications, from geometry to engineering. This article dives into the basics of square roots, their significance, calculation methods, and practical uses. Join us on this journey through the realm of mathematical roots!
Basic Concepts
The concept of square roots is fundamental in mathematics, particularly in algebra and geometry. A square root of a number x is a number y such that y2 = x. In other words, a square root of x is a number y which, when multiplied by itself, gives x.
For example:
- The square root of 9 is 3, because 3 × 3 = 9.
- The square root of 16 is 4, because 4 × 4 = 16.
Square roots can be represented in various ways:
- The radical symbol (√): The square root of x is written as √x.
- Exponent notation: The square root of x can also be written as x1/2.
Key properties of square roots include:
- Non-negativity: The square root of a non-negative number is also non-negative.
- Product property: √(ab) = √a × √b.
- Quotient property: √(a/b) = √a / √b, for b ≠ 0.
Let's look at some examples:
| Number (x) | Square Root (√x) |
|---|---|
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
Understanding square roots is essential for solving quadratic equations, working with exponents, and various applications in physics and engineering. For instance, the Pythagorean theorem involves square roots in the calculation of the length of the hypotenuse in a right triangle.
With these basic concepts, you are now ready to explore more advanced methods of calculating square roots and their applications in different fields of study.
Calculation Methods
Calculating the square root of a number can be done using various methods. Here, we will discuss three primary methods: the Prime Factorization Method, the Long Division Method, and the approximation method using calculators.
Prime Factorization Method
This method works well for perfect squares. Here’s how you can find the square root using prime factorization:
- Factorize the number into its prime factors.
- Pair the prime factors.
- Take one number from each pair and multiply them together to get the square root.
For example, to find the square root of 81:
- Prime factorize 81: \(81 = 3 \times 3 \times 3 \times 3\).
- Pair the factors: \((3 \times 3) \times (3 \times 3)\).
- Take one number from each pair: \(3 \times 3 = 9\).
Long Division Method
This method is useful for both perfect and non-perfect squares. Here’s the step-by-step process:
- Write the number in decimal form (e.g., 5 as 5.000000).
- Group the digits in pairs, starting from the decimal point.
- Find the largest number whose square is less than or equal to the first group. This becomes the divisor and the first digit of the quotient.
- Subtract the product of the divisor and quotient from the first group and bring down the next pair of digits.
- Double the quotient for the new divisor, and determine the next digit by trial and error.
- Repeat the process until the desired accuracy is achieved.
For example, to find the square root of 5 using the long division method:
- Write 5 as 5.00000000.
- Determine the initial divisor: 2 (since \(2^2 = 4\) and \(4 < 5\)).
- Subtract and bring down the next pair: \(5 - 4 = 1.00\).
- Double the quotient (2) to get the new divisor (4).
- Continue with 42, 2.2, 2.23, etc., until the desired precision is reached.
Approximation Method Using Calculators
For quick calculations, especially for non-perfect squares, using a calculator is the most efficient method:
- Enter the number into the calculator.
- Use the square root function (usually marked as \(\sqrt{}\) or "√").
- The calculator provides an approximation of the square root.
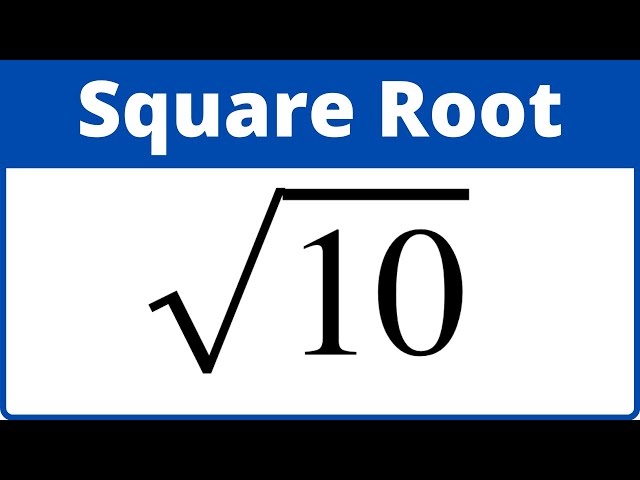
For example, using a calculator to find \(\sqrt{10}\) will yield approximately 3.162.
Examples
| Number | Square Root |
|---|---|
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
These methods cover a wide range of needs, from quick calculations to detailed, step-by-step processes for more precision.

List of Square Roots up to 100
The following table lists the square roots of integers from 1 to 100. For simplicity and clarity, the square roots are rounded to three decimal places.
| Number | Square Root |
|---|---|
| 1 | \(\sqrt{1} = 1.000\) |
| 2 | \(\sqrt{2} = 1.414\) |
| 3 | \(\sqrt{3} = 1.732\) |
| 4 | \(\sqrt{4} = 2.000\) |
| 5 | \(\sqrt{5} = 2.236\) |
| 6 | \(\sqrt{6} = 2.449\) |
| 7 | \(\sqrt{7} = 2.646\) |
| 8 | \(\sqrt{8} = 2.828\) |
| 9 | \(\sqrt{9} = 3.000\) |
| 10 | \(\sqrt{10} = 3.162\) |
| 11 | \(\sqrt{11} = 3.317\) |
| 12 | \(\sqrt{12} = 3.464\) |
| 13 | \(\sqrt{13} = 3.606\) |
| 14 | \(\sqrt{14} = 3.742\) |
| 15 | \(\sqrt{15} = 3.873\) |
| 16 | \(\sqrt{16} = 4.000\) |
| 17 | \(\sqrt{17} = 4.123\) |
| 18 | \(\sqrt{18} = 4.243\) |
| 19 | \(\sqrt{19} = 4.359\) |
| 20 | \(\sqrt{20} = 4.472\) |
| 21 | \(\sqrt{21} = 4.583\) |
| 22 | \(\sqrt{22} = 4.690\) |
| 23 | \(\sqrt{23} = 4.796\) |
| 24 | \(\sqrt{24} = 4.899\) |
| 25 | \(\sqrt{25} = 5.000\) |
| 26 | \(\sqrt{26} = 5.099\) |
| 27 | \(\sqrt{27} = 5.196\) |
| 28 | \(\sqrt{28} = 5.291\) |
| 29 | \(\sqrt{29} = 5.385\) |
| 30 | \(\sqrt{30} = 5.477\) |
| 31 | \(\sqrt{31} = 5.568\) |
| 32 | \(\sqrt{32} = 5.657\) |
| 33 | \(\sqrt{33} = 5.745\) |
| 34 | \(\sqrt{34} = 5.831\) |
| 35 | \(\sqrt{35} = 5.916\) |
| 36 | \(\sqrt{36} = 6.000\) |
| 37 | \(\sqrt{37} = 6.083\) |
| 38 | \(\sqrt{38} = 6.164\) |
| 39 | \(\sqrt{39} = 6.245\) |
| 40 | \(\sqrt{40} = 6.325\) |
| 41 | \(\sqrt{41} = 6.403\) |
| 42 | \(\sqrt{42} = 6.481\) |
| 43 | \(\sqrt{43} = 6.557\) |
| 44 | \(\sqrt{44} = 6.633\) |
| 45 | \(\sqrt{45} = 6.708\) |
| 46 | \(\sqrt{46} = 6.782\) |
| 47 | \(\sqrt{47} = 6.856\) |
| 48 | \(\sqrt{48} = 6.928\) |
| 49 | \(\sqrt{49} = 7.000\) |
| 50 | \(\sqrt{50} = 7.071\) |
| 51 | \(\sqrt{51} = 7.141\) |
| 52 | \(\sqrt{52} = 7.211\) |
| 53 | \(\sqrt{53} = 7.280\) |
| 54 | \(\sqrt{54} = 7.348\) |
| 55 | \(\sqrt{55} = 7.416\) |
| 56 | \(\sqrt{56} = 7.483\) |
| 57 | \(\sqrt{57} = 7.550\) |
| 58 | \(\sqrt{58} = 7.616\ ) |
| 59 | \(\sqrt{59} = 7.681\) |
| 60 | \(\sqrt{60} = 7.746\) |
| 61 | \(\sqrt{61} = 7.810\) |
| 62 | \(\sqrt{62} = 7.874\) |
| 63 | \(\sqrt{63} = 7.937\) |
| 64 | \(\sqrt{64} = 8.000\) |
| 65 | \(\sqrt{65} = 8.062\) |
| 66 | \(\sqrt{66} = 8.124\) |
| 67 | \(\sqrt{67} = 8.185\) |
| 68 | \(\sqrt{68} = 8.246\) |
| 69 | \(\sqrt{69} = 8.307\) |
| 70 | \(\sqrt{70} = 8.366\) |
| 71 | \(\sqrt{71} = 8.426\) |
| 72 | \(\sqrt{72} = 8.485\) |
| 73 | \(\sqrt{73} = 8.544\) |
| 74 | \(\sqrt{74} = 8.602\) |
| 75 | \(\sqrt{75} = 8.660\) |
| 76 | \(\sqrt{76} = 8.718\) |
| 77 | \(\sqrt{77} = 8.775\) |
| 78 | \(\sqrt{78} = 8.832\) |
| 79 | \(\sqrt{79} = 8.888\) |
| 80 | \(\sqrt{80} = 8.944\) |
| 81 | \(\sqrt{81} = 9.000\) |
| 82 | \(\sqrt{82} = 9.055\) |
| 83 | \(\sqrt{83} = 9.110\) |
| 84 | \(\sqrt{84} = 9.165\) |
| 85 | \(\sqrt{85} = 9.220\) |
| 86 | \(\sqrt{86} = 9.274\) |
| 87 | \(\sqrt{87} = 9.327\) |
| 88 | \(\sqrt{88} = 9.381\) |
| 89 | \(\sqrt{89} = 9.434\) |
| 90 | \(\sqrt{90} = 9.487\) |
| 91 | \(\sqrt{91} = 9.539\) |
| 92 | \(\sqrt{92} = 9.592\) |
| 93 | \(\sqrt{93} = 9.644\) |
| 94 | \(\sqrt{94} = 9.695\) |
| 95 | \(\sqrt{95} = 9.747\) |
| 96 | \(\sqrt{96} = 9.798\) |
| 97 | \(\sqrt{97} = 9.849\) |
| 98 | \(\sqrt{98} = 9.899\) |
| 99 | \(\sqrt{99} = 9.950\) |
| 100 | \(\sqrt{100} = 10.000\) |
Applications in Mathematics
The concept of square roots plays a crucial role in various mathematical applications. Here are some key areas where square roots are commonly used:
-
Pythagorean Theorem:
Square roots are essential in the Pythagorean Theorem, which relates the lengths of the sides of a right triangle. The theorem is expressed as \(a^2 + b^2 = c^2\), where \(c\) is the hypotenuse, and \(a\) and \(b\) are the other two sides. To find the length of the hypotenuse, we use the square root: \(c = \sqrt{a^2 + b^2}\).
-
Quadratic Equations:
Square roots are used to solve quadratic equations of the form \(ax^2 + bx + c = 0\). The solutions can be found using the quadratic formula:
\[
x = \frac{{-b \pm \sqrt{{b^2 - 4ac}}}}{2a}
\]
The term under the square root, \(b^2 - 4ac\), is called the discriminant and determines the nature of the roots. -
Distance Formula:
In coordinate geometry, the distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) in a plane is given by the formula:
\[
D = \sqrt{{(x_2 - x_1)^2 + (y_2 - y_1)^2}}
\]
This formula is derived from the Pythagorean Theorem and is used to calculate the length of a line segment between two points. -
Geometry:
Square roots are used in calculating the diagonal lengths of squares and rectangles. For a square with side length \(s\), the length of the diagonal is given by \(\sqrt{2} \cdot s\). For a rectangle with sides \(a\) and \(b\), the diagonal length is \(\sqrt{a^2 + b^2}\).
-
Complex Numbers:
Square roots extend into the complex number system. For a negative number, the square root involves imaginary numbers, denoted as \(i\), where \(i = \sqrt{-1}\). For example, \(\sqrt{-9} = 3i\).
-
Trigonometry:
In trigonometry, square roots appear in the identities and formulas involving sine, cosine, and tangent functions, particularly in the unit circle and right triangle relationships.
Historical Significance
The concept of the square root has a rich historical background, tracing its origins to ancient civilizations and evolving through significant mathematical discoveries.
Ancient Civilizations
Square roots were understood and used by ancient civilizations such as the Babylonians and Egyptians. Babylonian mathematicians approximated square roots using iterative methods, as evidenced by clay tablets dating back to 2000 BCE.
Greek Contributions
The Greeks made substantial contributions to the understanding of square roots, particularly through the works of Pythagoras and Euclid. Pythagoras’ theorem fundamentally relies on the concept of square roots, and Euclid’s "Elements" provided systematic methods for finding square roots.
Theodorus and the Spiral
Theodorus of Cyrene, a Greek mathematician, constructed the Spiral of Theodorus, which visually represents the square roots of successive natural numbers. This spiral is significant for its geometric representation of irrational numbers.
Islamic Golden Age
During the Islamic Golden Age, mathematicians like Al-Khwarizmi expanded upon Greek mathematics and introduced algorithms for calculating square roots. His works were later translated into Latin, influencing European mathematics.
Renaissance and Beyond
The Renaissance period saw further advancements in algebra and the systematic use of square roots. Mathematicians like Leonardo of Pisa (Fibonacci) and later, the invention of logarithms, facilitated more precise calculations of square roots.
Modern Era
In modern mathematics, square roots play a critical role in various fields such as engineering, physics, and computer science. Algorithms for computing square roots have been refined for speed and accuracy, critical for numerical methods and computer algorithms.
Conclusion
The study of square roots up to 100 offers valuable insights into fundamental mathematical concepts and practical applications. Understanding how to find and use square roots enhances problem-solving skills and mathematical literacy. This knowledge is crucial not only in academic settings but also in various real-world contexts, such as engineering, physics, and computer science.
Square roots are essential for simplifying complex equations, analyzing geometric properties, and optimizing algorithms. From ancient civilizations to modern times, the calculation and application of square roots have played a significant role in the advancement of science and technology.
Learning the different methods to calculate square roots, such as prime factorization, long division, and approximation techniques, provides a strong foundation for further mathematical exploration. Whether for academic purposes, professional use, or personal interest, mastering square roots up to 100 is a step towards a deeper appreciation of the beauty and utility of mathematics.
In conclusion, the knowledge of square roots is not only an academic exercise but also a practical tool that opens doors to numerous opportunities in various fields. Embrace this mathematical journey and continue to explore the fascinating world of numbers and their properties.

Học cách ghi nhớ các số bình phương từ 1 đến 25 một cách dễ dàng và nhanh chóng bằng phương pháp liên kết. Mẹo toán học giúp bạn tính nhẩm hiệu quả hơn.
Ghi Nhớ Các Số Bình Phương Bằng Cách Liên Kết - 25 Số Đầu Tiên - Mẹo Toán Nhanh
READ MORE:
Khám phá cách tính bình phương từ 1 đến 50 và học cách tính căn bậc hai từ 1 đến 50. Phương pháp đơn giản và dễ hiểu giúp bạn nắm vững kiến thức nhanh chóng.
Bình Phương Từ 1 Đến 50 || Học Căn Bậc Hai Từ 1 Đến 50