Topic simplify square root worksheet: Discover the most effective ways to simplify square roots with our comprehensive worksheets. This guide will help you master the basics, understand key concepts, and tackle a variety of problems with ease. Perfect for students and educators alike, these resources are designed to enhance learning and boost confidence in simplifying square roots.
Table of Content
- Simplifying Square Roots Worksheets
- Introduction to Simplifying Square Roots
- Methods for Simplifying Square Roots
- Perfect Squares and Their Properties
- Prime Factorization Method
- Examples of Simplifying Square Roots
- Simplifying Square Roots of Fractions
- Practice Problems and Solutions
- Worksheets and Printable Resources
- Common Mistakes and How to Avoid Them
- Advanced Topics in Simplifying Square Roots
- Applications of Simplifying Square Roots
- YOUTUBE: Hướng dẫn chi tiết về cách đơn giản hóa căn bậc hai, phù hợp cho học sinh và người mới bắt đầu. Xem video để hiểu rõ hơn về phương pháp này.
Simplifying Square Roots Worksheets
Simplifying square roots is an essential skill in mathematics, particularly useful for students in middle and high school. Below are various types of worksheets and exercises designed to help students master this skill. These resources include step-by-step instructions, practice problems, and solutions.
Finding Square Roots of Perfect Squares
- Practice finding the square roots of perfect squares (e.g., √25, √144).
- Worksheets provide exercises for both integers and decimals.
Reducing Non-Perfect Squares
- Learn to simplify non-perfect squares to their simplest radical form.
- Worksheets include problems like √50, √72, and more.
Square Root of Fractions
- Simplify the square root of fractions by separately simplifying the numerator and the denominator.
- Examples include √(4/9) and √(16/25).
Negative Numbers and Imaginary Roots
- Worksheets that teach how to handle square roots of negative numbers, resulting in imaginary numbers.
- Examples include √-25 and √-144.
Worksheet Examples
Here are some specific worksheet examples that you can download and practice:
Benefits of Practicing with Worksheets
- Helps clear concepts and enhances problem-solving skills.
- Prepares students for school exams and competitive exams like Olympiads.
- Improves mathematical calculations and accuracy.
Additional Resources
- Interactive online worksheets with instant feedback.
- Step-by-step solutions and explanations for each problem.
- Videos and tutorials for visual learners.
These worksheets are an excellent way to build confidence and proficiency in simplifying square roots. Regular practice will help students master the concepts and improve their mathematical skills.

READ MORE:
Introduction to Simplifying Square Roots
Simplifying square roots involves breaking down the number inside the square root into its simplest form. This process is essential for solving mathematical problems efficiently and accurately. In this section, we will explore the step-by-step methods to simplify square roots, including identifying perfect squares and using prime factorization.
- Identify Perfect Squares: Break down the number under the square root (radicand) into factors, identifying any perfect square factors. For example, 48 can be factored into 16 and 3, where 16 is a perfect square.
- Example: √48 = √(16 × 3) = √16 × √3 = 4√3
- Prime Factorization: If the radicand has no perfect square factors, break it down into its prime factors. Group pairs of identical factors.
- Example: √18 = √(9 × 2) = √9 × √2 = 3√2
- Example: √45 = √(9 × 5) = √9 × √5 = 3√5
- Combining Square Roots: For more complex expressions, combine and simplify using the rule √a × √b = √(a × b).
- Example: √6 × √15 = √(6 × 15) = √(90) = √(9 × 10) = 3√10
- Fractions: Simplify the numerator and denominator separately, then the whole fraction if necessary.
- Example: √(30/10) = √3
By mastering these techniques, you can simplify any square root expression effectively, making complex problems more manageable.
Methods for Simplifying Square Roots
There are several methods to simplify square roots, making it easier to work with them in mathematical problems. Here are some effective methods:
1. Prime Factorization Method
This method involves breaking down the radicand into its prime factors. Follow these steps:
- Factor the number inside the square root into its prime factors.
- Group the prime factors into pairs.
- For each pair, take one number out of the square root.
- Multiply the numbers outside the square root.
- The remaining factors inside the square root are left as they are.
Example:
\(\sqrt{72}\)
- Prime factorization: \(72 = 2 \times 2 \times 2 \times 3 \times 3\)
- Group into pairs: \((2 \times 2) \times 2 \times (3 \times 3)\)
- Simplify: \(2 \times 3 \times \sqrt{2} = 6\sqrt{2}\)
2. Perfect Square Method
In this method, you identify the largest perfect square factor of the radicand. Here are the steps:
- Find the largest perfect square factor of the number inside the square root.
- Rewrite the radicand as a product of this perfect square and another factor.
- Simplify the square root of the perfect square factor.
- Multiply the simplified result by the square root of the remaining factor.
Example:
\(\sqrt{50}\)
- Largest perfect square factor: \(25\)
- Rewrite as: \(\sqrt{25 \times 2}\)
- Simplify: \(5\sqrt{2}\)
3. Simplifying Square Roots of Fractions
To simplify the square root of a fraction, follow these steps:
- Simplify the numerator and denominator separately if possible.
- Take the square root of both the numerator and the denominator.
- Simplify further if needed.
Example:
\(\sqrt{\frac{9}{16}}\)
- Simplify numerator and denominator: \(\sqrt{9} = 3\), \(\sqrt{16} = 4\)
- Result: \(\frac{3}{4}\)
4. Using a Calculator
For numbers that do not simplify easily, you can use a calculator:
- Enter the radicand into the calculator.
- Use the square root function to find the approximate value.
- Round the result to the desired decimal places.
This method is useful for non-perfect squares and provides a quick approximation.
By mastering these methods, students can simplify square roots efficiently, enhancing their problem-solving skills in algebra and other mathematical fields.
Perfect Squares and Their Properties
Perfect squares are numbers that are the product of an integer multiplied by itself. Understanding perfect squares is crucial in simplifying square roots and solving quadratic equations. Here, we will explore the properties of perfect squares and how to identify them.
- A perfect square is always non-negative. For example, 0, 1, 4, 9, and 16 are perfect squares.
- The square root of a perfect square is always an integer. For instance, √4 = 2, √9 = 3.
- Perfect squares end in specific digits: 0, 1, 4, 5, 6, or 9. A number ending in other digits cannot be a perfect square.
- When a perfect square is expressed in its prime factorized form, all prime factors have even exponents. For example, 36 = 2^2 * 3^2.
Memorizing the first few perfect squares can be beneficial:
- 12 = 1
- 22 = 4
- 32 = 9
- 42 = 16
- 52 = 25
- 62 = 36
- 72 = 49
- 82 = 64
- 92 = 81
- 102 = 100
Recognizing these properties and memorizing common perfect squares can greatly aid in the simplification of square roots and other mathematical operations involving squares.
Prime Factorization Method
The prime factorization method is an efficient way to simplify square roots by breaking down the number into its prime factors. Here are the steps to follow:
- First, find the prime factorization of the number under the square root. For example, to simplify √72, determine its prime factors: 72 = 2 × 2 × 2 × 3 × 3.
- Next, pair the prime factors in groups of two. Each pair will come out of the square root as a single number. For √72, the pairs are (2,2) and (3,3).
- Write each pair as a single number outside the square root and leave any unpaired factors inside. So, √72 = √(2 × 2 × 2 × 3 × 3) = 2 × 3 × √2 = 6√2.
- Multiply the numbers outside the square root to get the simplified form. Thus, √72 simplifies to 6√2.
Using the prime factorization method helps in breaking down complex square roots into simpler, more manageable forms, enhancing understanding and problem-solving skills in mathematics.

Examples of Simplifying Square Roots
Simplifying square roots involves breaking down the number inside the root into its prime factors and then simplifying by extracting pairs of factors. Below are detailed steps and examples to help you master this technique.
-
Example: Simplify √50
- Start with the number 50.
- Prime factorize 50: 50 = 2 × 5 × 5.
- Group the factors: √(2 × 5 × 5).
- Extract pairs of factors: 5√2.
- So, √50 simplifies to 5√2.
-
Example: Simplify √72
- Start with the number 72.
- Prime factorize 72: 72 = 2 × 2 × 2 × 3 × 3.
- Group the factors: √(2 × 2 × 2 × 3 × 3).
- Extract pairs of factors: 2 × 3√2.
- So, √72 simplifies to 6√2.
-
Example: Simplify √128
- Start with the number 128.
- Prime factorize 128: 128 = 2 × 2 × 2 × 2 × 2 × 2 × 2.
- Group the factors: √(2 × 2 × 2 × 2 × 2 × 2 × 2).
- Extract pairs of factors: 2 × 2 × 2√2.
- So, √128 simplifies to 8√2.
Simplifying Square Roots of Fractions
When simplifying the square roots of fractions, it's important to understand that the process involves both the numerator and the denominator. The general rule is:
$$\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}$$
Let's go through the steps to simplify the square root of a fraction:
- Separate the square root of the fraction into the square roots of the numerator and the denominator.
- Simplify the square root of the numerator.
- Simplify the square root of the denominator.
- Combine the simplified square roots into a single fraction.
- If possible, further simplify the fraction.
Here's an example to illustrate these steps:
$$\sqrt{\frac{49}{81}}$$
- Separate the square root of the fraction:
- Simplify the square root of the numerator:
- Simplify the square root of the denominator:
- Combine the simplified square roots into a single fraction:
- Since the fraction is already in its simplest form, no further simplification is needed.
$$\sqrt{\frac{49}{81}} = \frac{\sqrt{49}}{\sqrt{81}}$$
$$\sqrt{49} = 7$$
$$\sqrt{81} = 9$$
$$\frac{\sqrt{49}}{\sqrt{81}} = \frac{7}{9}$$
Another example:
$$\sqrt{\frac{50}{72}}$$
- Separate the square root of the fraction:
- Simplify the square root of the numerator using prime factorization:
- Simplify the square root of the denominator using prime factorization:
- Combine the simplified square roots into a single fraction:
- Since the \( \sqrt{2} \) cancels out, the fraction simplifies to:
$$\sqrt{\frac{50}{72}} = \frac{\sqrt{50}}{\sqrt{72}}$$
$$\sqrt{50} = \sqrt{25 \cdot 2} = 5\sqrt{2}$$
$$\sqrt{72} = \sqrt{36 \cdot 2} = 6\sqrt{2}$$
$$\frac{\sqrt{50}}{\sqrt{72}} = \frac{5\sqrt{2}}{6\sqrt{2}}$$
$$\frac{5}{6}$$
Practicing with these steps will help you become proficient in simplifying the square roots of fractions. It's a skill that will be useful in various areas of mathematics.
Practice Problems and Solutions
Below are some practice problems designed to help you master the art of simplifying square roots. Each problem is followed by a detailed solution to guide you through the process.
Problem Set 1: Simplifying Square Roots
- Problem 1: Simplify \( \sqrt{50} \)
- Problem 2: Simplify \( \sqrt{72} \)
- Problem 3: Simplify \( \sqrt{98} \)
Solutions
-
Problem 1: Simplify \( \sqrt{50} \)
Solution:
- Factor 50 into prime factors: \( 50 = 2 \times 5^2 \)
- Apply the square root: \( \sqrt{50} = \sqrt{2 \times 5^2} = 5\sqrt{2} \)
-
Problem 2: Simplify \( \sqrt{72} \)
Solution:
- Factor 72 into prime factors: \( 72 = 2^3 \times 3^2 \)
- Apply the square root: \( \sqrt{72} = \sqrt{2^3 \times 3^2} = 6\sqrt{2} \)
-
Problem 3: Simplify \( \sqrt{98} \)
Solution:
- Factor 98 into prime factors: \( 98 = 2 \times 7^2 \)
- Apply the square root: \( \sqrt{98} = \sqrt{2 \times 7^2} = 7\sqrt{2} \)
Problem Set 2: Simplifying Square Roots of Fractions
- Problem 4: Simplify \( \sqrt{\frac{49}{16}} \)
- Problem 5: Simplify \( \sqrt{\frac{81}{25}} \)
- Problem 6: Simplify \( \sqrt{\frac{45}{20}} \)
Solutions
-
Problem 4: Simplify \( \sqrt{\frac{49}{16}} \)
Solution:
- Apply the square root to the numerator and the denominator separately: \( \sqrt{\frac{49}{16}} = \frac{\sqrt{49}}{\sqrt{16}} \)
- Simplify the square roots: \( \frac{\sqrt{49}}{\sqrt{16}} = \frac{7}{4} \)
-
Problem 5: Simplify \( \sqrt{\frac{81}{25}} \)
Solution:
- Apply the square root to the numerator and the denominator separately: \( \sqrt{\frac{81}{25}} = \frac{\sqrt{81}}{\sqrt{25}} \)
- Simplify the square roots: \( \frac{\sqrt{81}}{\sqrt{25}} = \frac{9}{5} \)
-
Problem 6: Simplify \( \sqrt{\frac{45}{20}} \)
Solution:
- Reduce the fraction inside the square root: \( \frac{45}{20} = \frac{9}{4} \)
- Apply the square root to the numerator and the denominator separately: \( \sqrt{\frac{9}{4}} = \frac{\sqrt{9}}{\sqrt{4}} \)
- Simplify the square roots: \( \frac{\sqrt{9}}{\sqrt{4}} = \frac{3}{2} \)
Problem Set 3: Mixed Practice
- Problem 7: Simplify \( \sqrt{200} \)
- Problem 8: Simplify \( \sqrt{\frac{121}{49}} \)
- Problem 9: Simplify \( \sqrt{2 \times 8} \)
Solutions
-
Problem 7: Simplify \( \sqrt{200} \)
Solution:
- Factor 200 into prime factors: \( 200 = 2^3 \times 5^2 \)
- Apply the square root: \( \sqrt{200} = \sqrt{2^3 \times 5^2} = 10\sqrt{2} \)
-
Problem 8: Simplify \( \sqrt{\frac{121}{49}} \)
Solution:
- Apply the square root to the numerator and the denominator separately: \( \sqrt{\frac{121}{49}} = \frac{\sqrt{121}}{\sqrt{49}} \)
- Simplify the square roots: \( \frac{\sqrt{121}}{\sqrt{49}} = \frac{11}{7} \)
-
Problem 9: Simplify \( \sqrt{2 \times 8} \)
Solution:
- Multiply the factors: \( 2 \times 8 = 16 \)
- Apply the square root: \( \sqrt{16} = 4 \)
Worksheets and Printable Resources
Simplifying square roots is a crucial skill in mathematics, and practice is essential to master it. Below are some excellent resources and worksheets to help students practice and reinforce their understanding of simplifying square roots.
Printable Worksheets
- Basic Simplifying Square Roots: These worksheets help students practice simplifying square roots of perfect squares and non-perfect squares. They are designed for 6th to 8th grade students and come with answer keys.
- Simplifying Square Roots of Fractions: These worksheets focus on simplifying square roots of fractions, helping students break down both the numerator and the denominator separately before combining them.
Online Resources
- : This website offers interactive worksheets that can be completed online or downloaded as PDFs. They also provide step-by-step solutions to help students understand the process of simplifying square roots.
- : A comprehensive collection of printable worksheets covering various aspects of square roots, including finding square roots of perfect squares, decimals, and fractions.
Additional Practice
For additional practice, students can explore various math games and puzzles related to square roots, which are available on several educational websites. Regular practice with these worksheets and resources will help solidify their understanding and improve their ability to simplify square roots efficiently.
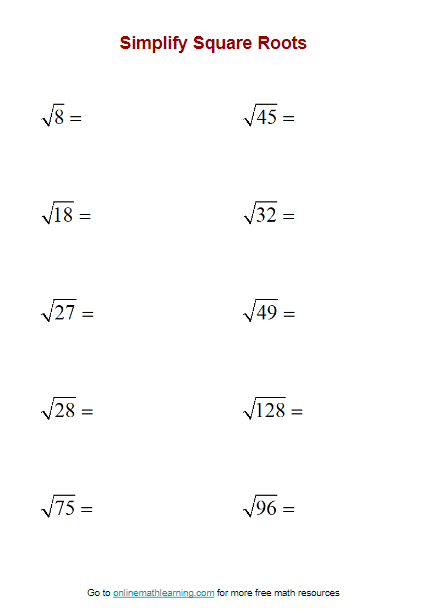
Common Mistakes and How to Avoid Them
When simplifying square roots, students often make several common mistakes. Identifying and understanding these errors is essential for mastering the concept. Here are some common mistakes and how to avoid them:
1. Incorrectly Simplifying the Radicand
Mistake: Failing to correctly factorize the number inside the square root.
Example: Simplifying \( \sqrt{50} \) incorrectly as \( 5\sqrt{2} \) instead of \( 5\sqrt{2} \).
Solution: Ensure you factorize the radicand into its prime factors and pair up the perfect squares.
\[
\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}
\]2. Incorrectly Handling Square Roots of Fractions
Mistake: Misapplying the rule for square roots of fractions.
Example: Simplifying \( \sqrt{\frac{9}{16}} \) as \( \frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4} \).
Solution: Apply the square root to both the numerator and the denominator separately.
\[
\sqrt{\frac{9}{16}} = \frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4}
\]3. Forgetting to Simplify Completely
Mistake: Stopping too early and not fully simplifying the expression.
Example: Leaving \( \sqrt{72} \) as \( \sqrt{36 \times 2} \) instead of simplifying further.
Solution: Always check if the number inside the square root can be factored further into perfect squares.
\[
\sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2}
\]4. Misunderstanding the Properties of Square Roots
Mistake: Incorrectly applying properties such as the product and quotient rules for square roots.
Example: Simplifying \( \sqrt{a \cdot b} \) as \( \sqrt{a} + \sqrt{b} \) instead of \( \sqrt{a} \cdot \sqrt{b} \).
Solution: Remember that \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \) and \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
\[
\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \quad \text{and} \quad \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\]5. Ignoring Negative Signs Under the Square Root
Mistake: Trying to simplify the square root of a negative number without recognizing it requires imaginary numbers.
Example: Attempting to simplify \( \sqrt{-4} \) without acknowledging it as \( 2i \).
Solution: Recognize that \( \sqrt{-1} = i \) (the imaginary unit).
\[
\sqrt{-4} = \sqrt{-1 \times 4} = 2i
\]By being aware of these common mistakes and understanding how to avoid them, students can improve their skills in simplifying square roots and gain confidence in their mathematical abilities.
Advanced Topics in Simplifying Square Roots
Exploring advanced topics in simplifying square roots allows students to deepen their understanding and tackle more complex problems. This section will cover various sophisticated techniques and examples.
1. Simplifying Square Roots with Higher Powers
When simplifying square roots involving higher powers, recognize that square roots and exponents are related operations. For example:
- \(\sqrt{x^4} = x^2\)
- \(\sqrt{x^6} = x^3\)
Generally, for any even power, \(\sqrt{x^{2n}} = x^n\).
2. Simplifying Expressions with Multiple Square Roots
Consider expressions that combine multiple square roots:
Example: Simplify \(\sqrt{20} \cdot \sqrt{5} \cdot \sqrt{2}\)
- Combine under one square root: \(\sqrt{20 \cdot 5 \cdot 2} = \sqrt{200}\)
- Factorize the number inside the square root: \(\sqrt{200} = \sqrt{4 \cdot 50} = \sqrt{4 \cdot 2 \cdot 25} = \sqrt{4 \cdot 2 \cdot 5^2}\)
- Simplify: \(\sqrt{4} \cdot \sqrt{2} \cdot \sqrt{25} = 2 \cdot \sqrt{2} \cdot 5 = 10\sqrt{2}\)
3. Working with Complex Numbers
Square roots of negative numbers involve complex numbers. For instance:
Example: Simplify \(\sqrt{-16}\)
- Express the negative number using \(i\): \(\sqrt{-16} = \sqrt{16 \cdot -1}\)
- Use the property \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\): \(\sqrt{16} \cdot \sqrt{-1} = 4i\)
4. Simplifying Radical Expressions with Variables
Radical expressions with variables can be simplified similarly:
Example: Simplify \(\sqrt{50x^4}\)
- Factorize inside the square root: \(\sqrt{50x^4} = \sqrt{25 \cdot 2 \cdot x^4}\)
- Simplify: \(\sqrt{25} \cdot \sqrt{2} \cdot \sqrt{x^4} = 5 \cdot \sqrt{2} \cdot x^2 = 5x^2\sqrt{2}\)
5. Rationalizing the Denominator
When a square root appears in the denominator, it can be rationalized:
Example: Rationalize \(\frac{5}{\sqrt{3}}\)
- Multiply the numerator and the denominator by \(\sqrt{3}\): \(\frac{5}{\sqrt{3}} \cdot \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
By mastering these advanced techniques, students can confidently approach a wide range of mathematical problems involving square roots.
Applications of Simplifying Square Roots
Simplifying square roots is an essential skill with numerous practical applications. This process is not only fundamental in mathematics but also in various real-world scenarios, ranging from engineering to everyday problem-solving.
-
Mathematical Problem Solving:
In mathematics, simplifying square roots is crucial for solving equations and inequalities. For instance, it is used to simplify quadratic equations, making them easier to solve. Additionally, it helps in operations involving surds, such as addition, subtraction, and multiplication of roots.
-
Geometry and Trigonometry:
Geometry frequently involves square roots when dealing with the Pythagorean theorem, calculating the lengths of sides in right triangles. Trigonometry uses simplified roots in various identities and equations.
-
Physics and Engineering:
Square roots appear in formulas for calculating distances, velocities, and accelerations. In engineering, simplified roots are used in structural analysis, electrical engineering, and fluid dynamics to make calculations more manageable.
-
Computer Science:
In computer algorithms, particularly those involving graphics and simulations, simplifying square roots is essential for optimizing performance and accuracy. It is used in algorithms for rendering graphics, computing distances, and normalizing vectors.
-
Everyday Life:
From determining the length of a diagonal in a rectangular room to calculating the area of circular objects, simplifying square roots finds applications in various daily tasks. It helps in making quick and accurate estimations and measurements.
Below are some examples demonstrating the practical applications of simplifying square roots:
-
Example 1: Geometry
To find the length of the diagonal of a rectangle with sides of 3 units and 4 units:
\[
\text{Diagonal} = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} = 5
\] -
Example 2: Physics
To calculate the velocity of an object using the formula \(v = \sqrt{2gh}\), where \(g\) is the acceleration due to gravity and \(h\) is the height:
If \(g = 9.8 \, \text{m/s}^2\) and \(h = 20 \, \text{m}\), then:
\[
v = \sqrt{2 \cdot 9.8 \cdot 20} = \sqrt{392} = 2\sqrt{98} = 2 \cdot 7\sqrt{2} = 14\sqrt{2} \, \text{m/s}
\]
Hướng dẫn chi tiết về cách đơn giản hóa căn bậc hai, phù hợp cho học sinh và người mới bắt đầu. Xem video để hiểu rõ hơn về phương pháp này.
Cách Đơn Giản Hóa Căn Bậc Hai
READ MORE:
Video hướng dẫn chi tiết cách đơn giản hóa căn bậc hai từ Math Antic. Phù hợp cho học sinh và những ai muốn học cách làm toán hiệu quả hơn.
Math Antic - Đơn Giản Hóa Căn Bậc Hai















