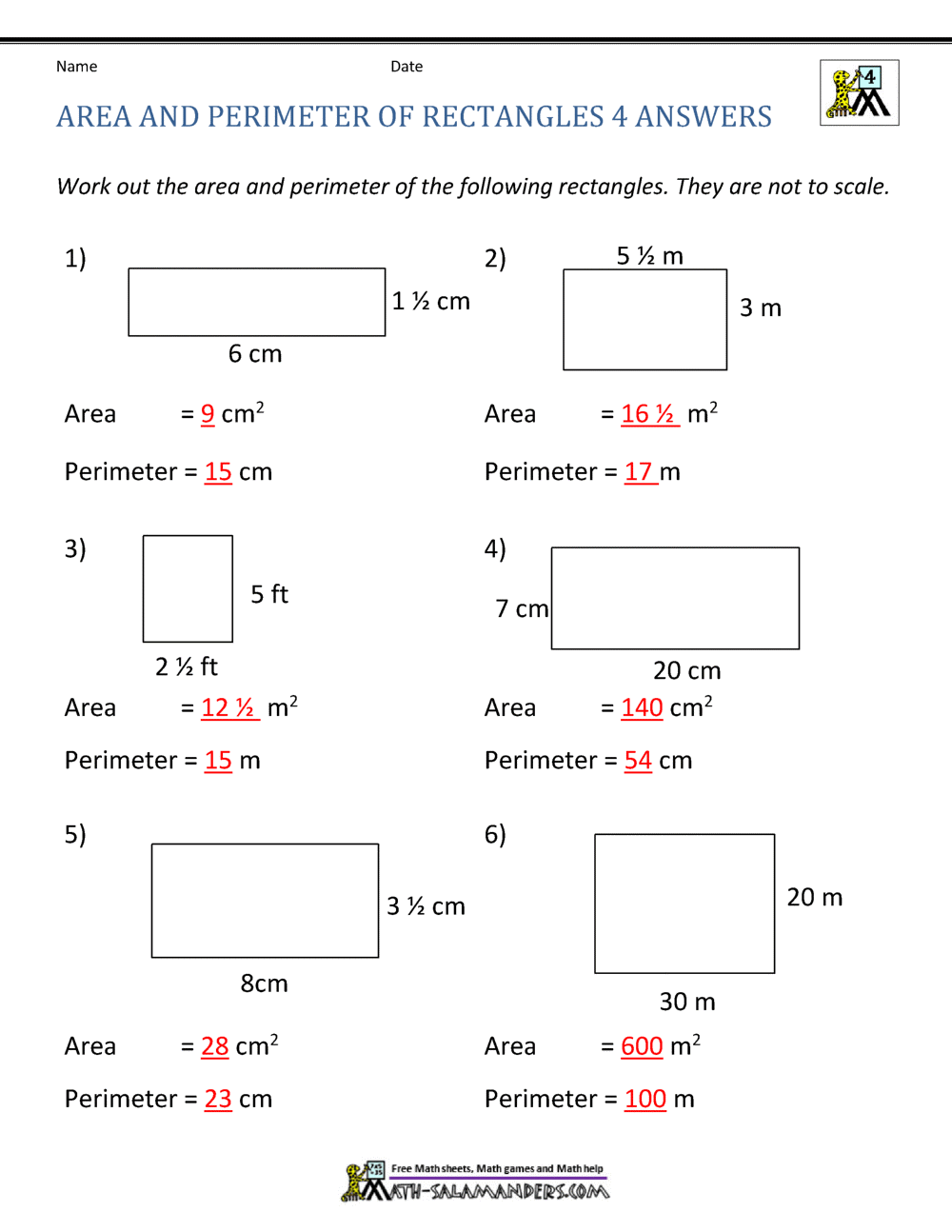
Topic triangle area and perimeter examples: Explore the fascinating world of triangles with our comprehensive guide on triangle area and perimeter examples. Learn the essential formulas, discover step-by-step examples, and apply these concepts to solve real-world problems. Perfect for students, teachers, and geometry enthusiasts looking to enhance their mathematical skills and understanding.
Table of Content
- Triangle Area and Perimeter Examples
- Introduction to Triangles
- Basic Properties of Triangles
- Types of Triangles
- Area of Triangles
- Formula for Area of a Triangle
- Examples of Area Calculation
- Perimeter of Triangles
- Formula for Perimeter of a Triangle
- Examples of Perimeter Calculation
- Special Triangle Formulas
- Using Heron's Formula for Area
- Real-life Applications of Triangle Calculations
- Practice Problems
- Conclusion
- YOUTUBE:
Triangle Area and Perimeter Examples
Understanding the area and perimeter of triangles is fundamental in geometry. This guide provides examples to illustrate how to calculate these values for various types of triangles.
1. Area of a Triangle
The area of a triangle can be calculated using the following formula:
\[
\text{Area} = \frac{1}{2} \times \text{base} \times \text{height}
\]
Example 1: Right Triangle
- Base (b): 6 cm
- Height (h): 4 cm
\[
\text{Area} = \frac{1}{2} \times 6 \, \text{cm} \times 4 \, \text{cm} = 12 \, \text{cm}^2
\]
Example 2: Equilateral Triangle
For an equilateral triangle with side length \(a\), the height \(h\) can be calculated as:
\[
h = \frac{\sqrt{3}}{2} \times a
\]
- Side (a): 8 cm
\[
h = \frac{\sqrt{3}}{2} \times 8 \, \text{cm} = 4\sqrt{3} \, \text{cm}
\]
Thus, the area is:
\[
\text{Area} = \frac{1}{2} \times 8 \, \text{cm} \times 4\sqrt{3} \, \text{cm} = 16\sqrt{3} \, \text{cm}^2
\]
2. Perimeter of a Triangle
The perimeter of a triangle is the sum of the lengths of its sides. For a triangle with sides \(a\), \(b\), and \(c\), the formula is:
\[
\text{Perimeter} = a + b + c
\]
Example 3: Scalene Triangle
- Side (a): 5 cm
- Side (b): 7 cm
- Side (c): 9 cm
\[
\text{Perimeter} = 5 \, \text{cm} + 7 \, \text{cm} + 9 \, \text{cm} = 21 \, \text{cm}
\]
Example 4: Isosceles Triangle
- Equal sides (a): 6 cm
- Base (b): 8 cm
\[
\text{Perimeter} = 6 \, \text{cm} + 6 \, \text{cm} + 8 \, \text{cm} = 20 \, \text{cm}
\]
3. Special Formulas
For triangles where the height is not known, Heron's formula can be used to calculate the area:
\[
\text{Area} = \sqrt{s(s-a)(s-b)(s-c)}
\]
where \(s\) is the semi-perimeter:
\[
s = \frac{a + b + c}{2}
\]
Example 5: Using Heron's Formula
- Side (a): 7 cm
- Side (b): 8 cm
First, calculate the semi-perimeter \(s\):
\[
s = \frac{7 \, \text{cm} + 8 \, \text{cm} + 9 \, \text{cm}}{2} = 12 \, \text{cm}
\]
Then, use Heron's formula to find the area:
\[
\text{Area} = \sqrt{12 \, \text{cm} \times (12 \, \text{cm} - 7 \, \text{cm}) \times (12 \, \text{cm} - 8 \, \text{cm}) \times (12 \, \text{cm} - 9 \, \text{cm})}
\]
\[
\text{Area} = \sqrt{12 \times 5 \times 4 \times 3} = \sqrt{720} = 12\sqrt{5} \, \text{cm}^2
\]

READ MORE:
Introduction to Triangles
Triangles are one of the most fundamental shapes in geometry, defined as a three-sided polygon with three vertices and three angles. Each triangle's properties are determined by its sides and angles, making them versatile in various applications from simple calculations to complex problem-solving.
Here are some key characteristics of triangles:
- Triangles have three sides, three angles, and three vertices.
- The sum of the interior angles of a triangle is always 180 degrees.
- Triangles can be classified based on their side lengths and angles.
There are different types of triangles:
- Equilateral Triangle: All three sides are of equal length, and all three angles are equal (each being 60 degrees).
- Isosceles Triangle: Has two sides of equal length and two equal angles.
- Scalene Triangle: All three sides and all three angles are of different lengths and measures.
- Right Triangle: One of the angles is a right angle (90 degrees).
- Acute Triangle: All three angles are less than 90 degrees.
- Obtuse Triangle: One of the angles is greater than 90 degrees.
Understanding the basics of triangles is essential before delving into calculating their area and perimeter. These calculations are crucial in various fields such as engineering, architecture, and various real-world applications.
The general formulas for the area and perimeter of a triangle are as follows:
- Area: \[ \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} \]
- Perimeter: \[ \text{Perimeter} = a + b + c \] where \( a \), \( b \), and \( c \) are the lengths of the sides of the triangle.
With these fundamentals in mind, you can now explore specific examples and applications of triangle area and perimeter calculations in the following sections.
Basic Properties of Triangles
Triangles possess several basic properties that are fundamental to understanding their geometry. These properties are essential for calculating the area, perimeter, and other characteristics of triangles.
Here are the key properties of triangles:
- Sum of Interior Angles: The sum of the interior angles of a triangle is always 180 degrees. \[ \angle A + \angle B + \angle C = 180^\circ \]
- Triangle Inequality Theorem: The sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
- \( a + b > c \)
- \( a + c > b \)
- \( b + c > a \)
- Types of Angles: Triangles can be classified based on their angles:
- Acute Triangle: All three angles are less than 90 degrees.
- Right Triangle: One angle is exactly 90 degrees.
- Obtuse Triangle: One angle is greater than 90 degrees.
- Types of Sides: Triangles can also be classified based on the lengths of their sides:
- Equilateral Triangle: All three sides are equal in length.
- Isosceles Triangle: Two sides are equal in length.
- Scalene Triangle: All three sides have different lengths.
Additionally, triangles have several important points associated with their geometry:
- Centroid: The point where the three medians intersect. It is the center of mass of the triangle.
- Circumcenter: The point where the perpendicular bisectors of the sides intersect. It is the center of the circumcircle, which passes through all the vertices of the triangle.
- Incenter: The point where the angle bisectors intersect. It is the center of the incircle, which is tangent to all three sides of the triangle.
- Orthocenter: The point where the altitudes intersect.
Understanding these basic properties is crucial for solving problems involving triangles and for applying more advanced concepts in geometry. With these properties in mind, you can explore more specific examples and applications of triangle calculations in the following sections.
Types of Triangles
Triangles can be classified based on their sides and angles. Understanding the different types of triangles is fundamental to studying their properties and applications.
Classification by Sides
- Equilateral Triangle: All three sides are of equal length, and all three angles are equal, each measuring \(60^\circ\).
- Isosceles Triangle: Two sides are of equal length, and the angles opposite these sides are equal.
- Scalene Triangle: All three sides and all three angles are of different lengths and measures.
Classification by Angles
- Acute Triangle: All three angles are less than \(90^\circ\).
- Right Triangle: One angle is exactly \(90^\circ\). The side opposite this angle is the hypotenuse, and the other two sides are called the legs.
- Obtuse Triangle: One angle is greater than \(90^\circ\).
Visual Representation
| Type | Diagram |
|---|---|
| Equilateral Triangle |  |
| Isosceles Triangle |  |
| Scalene Triangle |  |
| Acute Triangle |  |
| Right Triangle |  |
| Obtuse Triangle |  |
Area of Triangles
The area of a triangle is the amount of space enclosed by its three sides. There are several methods to calculate the area of a triangle, depending on the information available about the triangle. Here are the most common formulas:
- Using Base and Height:
- Using Heron's Formula:
- Using Two Sides and the Included Angle:
- Equilateral Triangle:
- Isosceles Triangle:
If the base \( b \) and height \( h \) of the triangle are known, the area \( A \) can be calculated using the formula:
\[
A = \frac{1}{2} \times b \times h
\]
For example, if the base of a triangle is 10 inches and the height is 5 inches, the area would be:
\[
A = \frac{1}{2} \times 10 \times 5 = 25 \text{ square inches}
\]
If the lengths of all three sides of the triangle are known, the area can be calculated using Heron's formula. First, calculate the semi-perimeter \( s \):
\[
s = \frac{a + b + c}{2}
\]
Then, the area \( A \) is given by:
\[
A = \sqrt{s(s - a)(s - b)(s - c)}
\]
For example, if a triangle has sides of lengths 7, 8, and 9 units:
\[
s = \frac{7 + 8 + 9}{2} = 12
\]
\[
A = \sqrt{12(12 - 7)(12 - 8)(12 - 9)} = \sqrt{12 \times 5 \times 4 \times 3} = \sqrt{720} \approx 26.83 \text{ square units}
\]
If two sides and the included angle are known, the area can be calculated using the formula:
\[
A = \frac{1}{2} \times a \times b \times \sin(C)
\]
For example, if a triangle has sides of lengths 5 and 6 units with an included angle of 60 degrees:
\[
A = \frac{1}{2} \times 5 \times 6 \times \sin(60^\circ) = \frac{1}{2} \times 5 \times 6 \times \frac{\sqrt{3}}{2} = 7.5\sqrt{3} \approx 12.99 \text{ square units}
\]
For an equilateral triangle (all sides equal), if the side length is \( a \), the area is given by:
\[
A = \frac{\sqrt{3}}{4} \times a^2
\]
For example, if each side of an equilateral triangle is 4 units:
\[
A = \frac{\sqrt{3}}{4} \times 4^2 = \sqrt{3} \times 4 = 4\sqrt{3} \approx 6.93 \text{ square units}
\]
For an isosceles triangle (two sides equal), if the equal sides have length \( a \) and the base has length \( b \), the area is given by:
\[
A = \frac{1}{4} \times b \times \sqrt{4a^2 - b^2}
\]
For example, if the equal sides are 5 units and the base is 6 units:
\[
A = \frac{1}{4} \times 6 \times \sqrt{4 \times 5^2 - 6^2} = \frac{1}{4} \times 6 \times \sqrt{100 - 36} = \frac{1}{4} \times 6 \times \sqrt{64} = \frac{1}{4} \times 6 \times 8 = 12 \text{ square units}
\]
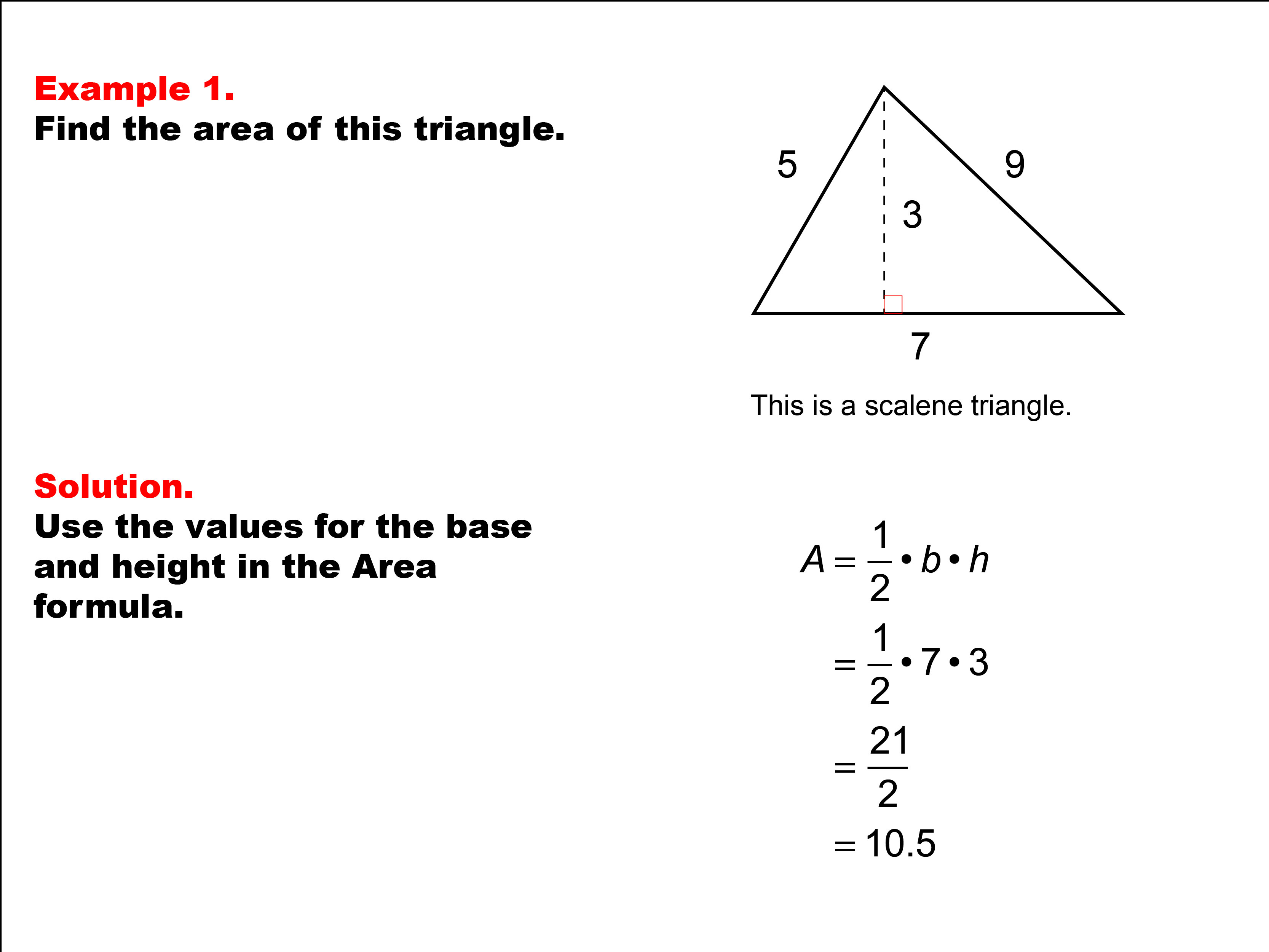
Formula for Area of a Triangle
The area of a triangle can be calculated using several formulas depending on the given dimensions. Here are the most common methods:
- Base and Height: The most basic formula uses the base and height of the triangle. If the base \( b \) and height \( h \) are known, the area \( A \) is given by: \[ A = \frac{1}{2} \times b \times h \]
- Heron's Formula: For a triangle with sides \( a \), \( b \), and \( c \), the area can be found using Heron's formula. First, calculate the semi-perimeter \( s \): \[ s = \frac{a + b + c}{2} \] Then, the area is: \[ A = \sqrt{s(s - a)(s - b)(s - c)} \]
- Two Sides and Included Angle: If two sides and the included angle \( \theta \) are known, the area is given by: \[ A = \frac{1}{2} \times a \times b \times \sin(\theta) \]
- Equilateral Triangle: For an equilateral triangle with side length \( a \), the area is: \[ A = \frac{\sqrt{3}}{4} \times a^2 \]
- Isosceles Triangle: For an isosceles triangle with equal sides \( a \) and base \( b \), the area can be found using: \[ A = \frac{1}{4} \times b \times \sqrt{4a^2 - b^2} \]
Examples of Area Calculation
- Example 1: Find the area of a triangle with a base of 10 inches and a height of 5 inches.
Solution:
\[
A = \frac{1}{2} \times 10 \times 5 = 25 \text{ in}^2
\] - Example 2: Find the area of an equilateral triangle with a side of 6 cm.
Solution:
\[
A = \frac{\sqrt{3}}{4} \times 6^2 \approx 15.59 \text{ cm}^2
\] - Example 3: Calculate the area of a triangle with sides 7 cm, 8 cm, and 9 cm using Heron's formula.
Solution:
\[
s = \frac{7 + 8 + 9}{2} = 12 \\
A = \sqrt{12(12 - 7)(12 - 8)(12 - 9)} = \sqrt{12 \times 5 \times 4 \times 3} = \sqrt{720} \approx 26.83 \text{ cm}^2
\]
Examples of Area Calculation
Calculating the area of a triangle can be approached in various ways depending on the information available. Below are several examples demonstrating different methods to find the area of triangles.
Example 1: Using Base and Height
Given a triangle with a base \( b = 10 \, \text{cm} \) and height \( h = 5 \, \text{cm} \):
Formula: \( A = \frac{1}{2} \times b \times h \)
Calculation:
\[
A = \frac{1}{2} \times 10 \, \text{cm} \times 5 \, \text{cm} = \frac{1}{2} \times 50 \, \text{cm}^2 = 25 \, \text{cm}^2
\]
Example 2: Using Heron's Formula
For a triangle with sides \( a = 7 \, \text{cm} \), \( b = 8 \, \text{cm} \), and \( c = 9 \, \text{cm} \):
Steps:
- Calculate the semi-perimeter \( s \): \[ s = \frac{a + b + c}{2} = \frac{7 + 8 + 9}{2} = 12 \, \text{cm} \]
- Apply Heron's formula: \[ A = \sqrt{s(s - a)(s - b)(s - c)} = \sqrt{12(12 - 7)(12 - 8)(12 - 9)} = \sqrt{12 \times 5 \times 4 \times 3} = \sqrt{720} \approx 26.83 \, \text{cm}^2 \]
Example 3: Using Trigonometry
For a triangle with sides \( a = 6 \, \text{cm} \), \( b = 8 \, \text{cm} \) and included angle \( \theta = 45^\circ \):
Formula: \( A = \frac{1}{2} \times a \times b \times \sin(\theta) \)
Calculation:
\[
A = \frac{1}{2} \times 6 \, \text{cm} \times 8 \, \text{cm} \times \sin(45^\circ) = \frac{1}{2} \times 6 \times 8 \times 0.707 \approx 16.97 \, \text{cm}^2
\]
Example 4: Equilateral Triangle
Given an equilateral triangle with side \( a = 4 \, \text{cm} \):
Formula: \( A = \frac{\sqrt{3}}{4} \times a^2 \)
Calculation:
\[
A = \frac{\sqrt{3}}{4} \times 4^2 = \frac{\sqrt{3}}{4} \times 16 \approx 6.93 \, \text{cm}^2
\]
Example 5: Isosceles Triangle
For an isosceles triangle with equal sides \( a = 5 \, \text{cm} \) and base \( b = 6 \, \text{cm} \):
Formula:
\[
A = \frac{1}{4} b \sqrt{4a^2 - b^2}
\]
Calculation:
\[
A = \frac{1}{4} \times 6 \times \sqrt{4 \times 5^2 - 6^2} = \frac{1}{4} \times 6 \times \sqrt{100 - 36} = \frac{1}{4} \times 6 \times \sqrt{64} = \frac{1}{4} \times 6 \times 8 = 12 \, \text{cm}^2
\]
Perimeter of Triangles
The perimeter of a triangle is the total length of its boundary, which is the sum of the lengths of its three sides. The formula to calculate the perimeter of a triangle is simple:
\[ \text{Perimeter} = a + b + c \]
where \( a \), \( b \), and \( c \) are the lengths of the sides of the triangle.
Steps to Calculate the Perimeter
- Identify and note down the lengths of all three sides of the triangle.
- Ensure that all the side lengths are in the same unit of measurement.
- Add the lengths of the three sides to get the perimeter.
Examples
- Example 1: Equilateral Triangle
- Example 2: Isosceles Triangle
- Example 3: Scalene Triangle
An equilateral triangle has all three sides of equal length. If each side is \( a \) units, the perimeter \( P \) is given by:
\[ P = 3a \]
For example, if \( a = 10 \) units, then:
\[ P = 3 \times 10 = 30 \text{ units} \]
An isosceles triangle has two sides of equal length. If the equal sides are \( a \) units each and the base is \( b \) units, the perimeter \( P \) is given by:
\[ P = 2a + b \]
For example, if \( a = 15 \) units and \( b = 20 \) units, then:
\[ P = 2 \times 15 + 20 = 30 + 20 = 50 \text{ units} \]
A scalene triangle has all three sides of different lengths. If the sides are \( a \), \( b \), and \( c \) units, the perimeter \( P \) is given by:
\[ P = a + b + c \]
For example, if \( a = 9 \) units, \( b = 12 \) units, and \( c = 15 \) units, then:
\[ P = 9 + 12 + 15 = 36 \text{ units} \]
Perimeter of Specific Triangles
- Right Triangle
A right triangle has one angle of \( 90^\circ \). If the lengths of the legs are \( a \) and \( b \), and the hypotenuse is \( c \), then:
\[ c = \sqrt{a^2 + b^2} \]
The perimeter \( P \) is given by:
\[ P = a + b + c \]
For example, if \( a = 3 \) units and \( b = 4 \) units, then:
\[ c = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} = 5 \text{ units} \]
Thus, the perimeter is:
\[ P = 3 + 4 + 5 = 12 \text{ units} \]
Formula for Perimeter of a Triangle
The perimeter of a triangle is the total length of its boundary, which is the sum of the lengths of its three sides. The formula for the perimeter \(P\) of a triangle is:
\[ P = a + b + c \]
where \(a\), \(b\), and \(c\) are the lengths of the sides of the triangle.
Types of Triangles and Their Perimeter Formulas
Equilateral Triangle
An equilateral triangle has all three sides of equal length. If each side is of length \(a\), then the perimeter \(P\) is:
\[ P = 3a \]
Isosceles Triangle
An isosceles triangle has two sides of equal length. If the equal sides are of length \(a\) and the base is of length \(b\), then the perimeter \(P\) is:
\[ P = 2a + b \]
Scalene Triangle
A scalene triangle has all sides of different lengths. If the sides are of lengths \(a\), \(b\), and \(c\), then the perimeter \(P\) is:
\[ P = a + b + c \]
Right Triangle
A right triangle has one angle of \(90^\circ\). The sides include the base \(b\), the height \(h\), and the hypotenuse \(c\). The perimeter \(P\) is:
\[ P = b + h + c \]
Using the Pythagorean theorem, the hypotenuse \(c\) can be found as:
\[ c = \sqrt{b^2 + h^2} \]
Thus, the perimeter can also be expressed as:
\[ P = b + h + \sqrt{b^2 + h^2} \]
Examples
Example 1: Equilateral Triangle
Find the perimeter of an equilateral triangle with each side of length 7 cm.
\[ P = 3a = 3 \times 7 = 21 \text{ cm} \]
Example 2: Isosceles Triangle
Find the perimeter of an isosceles triangle with equal sides of length 5 m and a base of length 8 m.
\[ P = 2a + b = 2 \times 5 + 8 = 10 + 8 = 18 \text{ m} \]
Example 3: Scalene Triangle
Find the perimeter of a scalene triangle with sides of length 6 in, 8 in, and 10 in.
\[ P = a + b + c = 6 + 8 + 10 = 24 \text{ in} \]
Example 4: Right Triangle
Find the perimeter of a right triangle with base 3 ft, height 4 ft, and hypotenuse 5 ft.
\[ P = b + h + c = 3 + 4 + 5 = 12 \text{ ft} \]
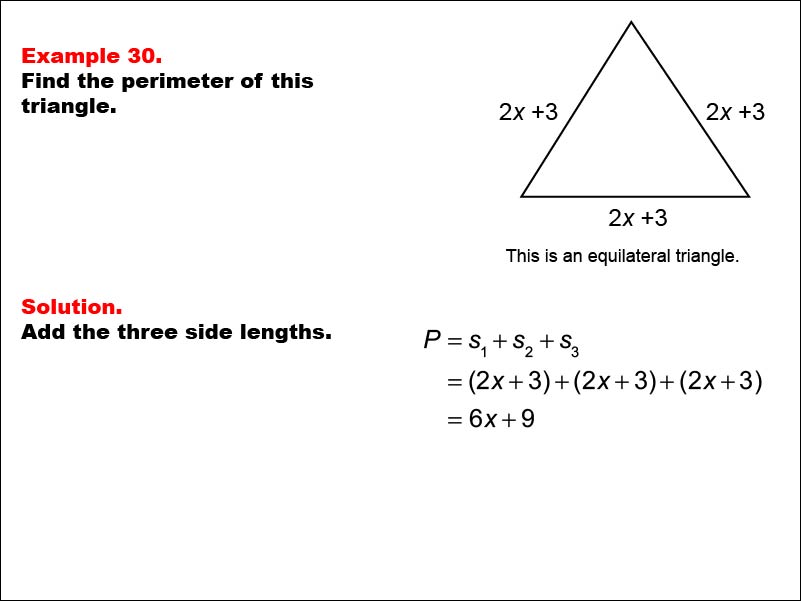
Examples of Perimeter Calculation
Calculating the perimeter of a triangle involves adding the lengths of its three sides. Here are some examples to illustrate this process:
Example 1: Equilateral Triangle
An equilateral triangle has all three sides of equal length.
- Side length: \( a = 10 \, \text{cm} \)
The formula for the perimeter \( P \) is:
\[ P = 3a \]
Calculation:
\[ P = 3 \times 10 = 30 \, \text{cm} \]
Example 2: Isosceles Triangle
An isosceles triangle has two sides of equal length.
- Equal side lengths: \( a = 7 \, \text{m} \)
- Base: \( b = 5 \, \text{m} \)
The formula for the perimeter \( P \) is:
\[ P = 2a + b \]
Calculation:
\[ P = 2 \times 7 + 5 = 14 + 5 = 19 \, \text{m} \]
Example 3: Scalene Triangle
A scalene triangle has all sides of different lengths.
- Side \( a = 8 \, \text{in} \)
- Side \( b = 6 \, \text{in} \)
- Side \( c = 5 \, \text{in} \)
The formula for the perimeter \( P \) is:
\[ P = a + b + c \]
Calculation:
\[ P = 8 + 6 + 5 = 19 \, \text{in} \]
Example 4: Right Triangle
A right triangle has one angle of \( 90^\circ \).
- Base: \( b = 9 \, \text{cm} \)
- Height: \( h = 12 \, \text{cm} \)
- Hypotenuse: \( c \) (calculated using the Pythagorean theorem)
First, find the hypotenuse \( c \):
\[ c = \sqrt{b^2 + h^2} = \sqrt{9^2 + 12^2} = \sqrt{81 + 144} = \sqrt{225} = 15 \, \text{cm} \]
The formula for the perimeter \( P \) is:
\[ P = b + h + c \]
Calculation:
\[ P = 9 + 12 + 15 = 36 \, \text{cm} \]
Example 5: Word Problem
A triangular garden has side lengths of \( 20 \, \text{m} \), \( 15 \, \text{m} \), and \( 25 \, \text{m} \). What is the perimeter?
The formula for the perimeter \( P \) is:
\[ P = a + b + c \]
Calculation:
\[ P = 20 + 15 + 25 = 60 \, \text{m} \]
These examples demonstrate how to calculate the perimeter of different types of triangles by adding the lengths of their sides.
Special Triangle Formulas
Triangles have various properties and formulas that can be used to calculate their area and perimeter. Here, we focus on special triangle formulas that apply to different types of triangles:
Area Formulas for Special Triangles
- Scalene Triangle:
For a scalene triangle with sides \(a\), \(b\), and \(c\), and semi-perimeter \(s = \frac{a + b + c}{2}\), the area is given by Heron's formula:
\[
\text{Area} = \sqrt{s(s - a)(s - b)(s - c)}
\] - Equilateral Triangle:
For an equilateral triangle with side length \(a\), the area is given by:
\[
\text{Area} = \frac{\sqrt{3}}{4} a^2 - Isosceles Triangle:
For an isosceles triangle with equal sides \(a\) and base \(b\), the area can be calculated using:
\[
\text{Area} = \frac{1}{4} b \sqrt{4a^2 - b^2}
Perimeter Formulas for Special Triangles
- Scalene Triangle:
The perimeter of a scalene triangle is simply the sum of its sides:
\[
\text{Perimeter} = a + b + c - Equilateral Triangle:
For an equilateral triangle, the perimeter is:
\[
\text{Perimeter} = 3a - Isosceles Triangle:
The perimeter of an isosceles triangle is:
\[
\text{Perimeter} = 2a + b
Using Trigonometry to Find Area
In some cases, knowing two sides and the included angle of a triangle can help in finding the area. This is useful for any type of triangle:
- General Triangle:
If two sides \(a\) and \(b\) and the included angle \(C\) are known, the area is:
\[
\text{Area} = \frac{1}{2} ab \sin(C)
Example Calculations
- Example 1: Area of a Scalene Triangle
Given sides \(a = 5\), \(b = 6\), and \(c = 7\), calculate the area using Heron's formula:
First, find the semi-perimeter \(s\):
\[
s = \frac{a + b + c}{2} = \frac{5 + 6 + 7}{2} = 9Next, apply Heron's formula:
\[
\text{Area} = \sqrt{9(9 - 5)(9 - 6)(9 - 7)} = \sqrt{9 \times 4 \times 3 \times 2} = \sqrt{216} \approx 14.7
\] - Example 2: Area of an Equilateral Triangle
For a side length of \(a = 4\), the area is:
\[
\text{Area} = \frac{\sqrt{3}}{4} \times 4^2 = \frac{\sqrt{3}}{4} \times 16 = 4\sqrt{3} \approx 6.93
\] - Example 3: Area using Trigonometry
For sides \(a = 7\), \(b = 8\), and included angle \(C = 30^\circ\):
\[
\text{Area} = \frac{1}{2} \times 7 \times 8 \times \sin(30^\circ) = \frac{1}{2} \times 7 \times 8 \times 0.5 = 14
\]
Conclusion
Understanding these special formulas for calculating the area and perimeter of different types of triangles is crucial for solving various geometric problems. Each type of triangle has unique properties that can simplify calculations when the appropriate formulas are applied.
Using Heron's Formula for Area
Heron's formula provides a way to calculate the area of a triangle when the lengths of all three sides are known. The formula is named after Hero of Alexandria, a Greek engineer and mathematician. The steps to apply Heron's formula are as follows:
- Calculate the semi-perimeter:
The semi-perimeter (s) is half of the triangle's perimeter. If the sides of the triangle are \(a\), \(b\), and \(c\), then:
\[
s = \frac{a + b + c}{2}
\] - Apply Heron's formula:
Using the semi-perimeter \(s\) and the lengths of the sides \(a\), \(b\), and \(c\), the area (A) of the triangle can be calculated using the formula:
\[
A = \sqrt{s(s - a)(s - b)(s - c)}
\]
Example Calculation
Consider a triangle with side lengths \(a = 7\), \(b = 8\), and \(c = 9\).
- First, calculate the semi-perimeter:
\[
s = \frac{7 + 8 + 9}{2} = 12
\] - Next, apply Heron's formula:
\[
A = \sqrt{12(12 - 7)(12 - 8)(12 - 9)}
\]\[
A = \sqrt{12 \times 5 \times 4 \times 3}
\]\[
A = \sqrt{720} \approx 26.83 \text{ square units}
\]
Special Cases
Heron's formula can be simplified for specific types of triangles:
- Equilateral Triangle:
For an equilateral triangle where all sides are equal (\(a = b = c\)), the formula simplifies to:
\[
A = \frac{\sqrt{3}}{4}a^2
\] - Isosceles Triangle:
For an isosceles triangle where two sides are equal (\(a = b\)), the area can be calculated using the original Heron's formula, but recognizing the repeated sides can simplify the process.
Practice Problems
- Problem 1:
Calculate the area of a triangle with sides 6, 8, and 10.
- Problem 2:
Find the area of an equilateral triangle with each side measuring 5 units.
- Problem 3:
Determine the area of an isosceles triangle with sides 7, 7, and 10.
Use Heron's formula to solve these problems and verify your answers.
Real-life Applications of Triangle Calculations
Triangles are fundamental shapes in both mathematics and the real world. Their properties and calculations are used in various fields. Here are some common real-life applications of triangle calculations:
1. Architecture and Construction
Triangles are used extensively in architecture and construction. Their ability to provide structural stability makes them ideal for supporting frameworks in buildings and bridges. Understanding the area and perimeter of triangular components helps engineers and architects ensure stability and optimize material usage.
- Roof Trusses: Triangular trusses distribute weight evenly, making them essential in the design of roofs.
- Bridge Support: Triangular sections are used to construct bridges, as they can bear significant loads and provide strength.
2. Land Surveying
Surveyors use triangles to measure and map land. By dividing areas into a series of triangles, they can calculate distances and areas accurately.
- Triangulation: This method involves dividing the survey area into triangles to determine distances and plot positions.
- Plotting Boundaries: Calculating the area of triangular sections helps in accurately defining property boundaries.
3. Navigation
Triangles are crucial in navigation, particularly in methods like triangulation and GPS technology.
- Triangulation: Navigators use triangles formed by points on the ground or sea to determine their position.
- GPS Systems: GPS technology relies on the principles of triangulation to provide precise location data.
4. Art and Design
In art and design, triangles are used to create aesthetically pleasing and stable compositions. Artists and designers calculate areas and perimeters to plan their work.
- Geometric Art: Triangular shapes are often used to form complex patterns and designs.
- Interior Design: Triangles help in planning the layout and structure of spaces.
5. Aerospace and Mechanical Engineering
Triangles are used in the design of various components in aerospace and mechanical engineering due to their strength and stability.
- Aircraft Design: The triangular structures in wings and fuselages provide the necessary strength while minimizing weight.
- Mechanical Linkages: Triangular configurations are used in designing mechanical linkages for their rigidity and stability.
6. Robotics
Robotics frequently employs triangular configurations to ensure movement precision and structural integrity.
- Robot Arms: The joints and linkages of robotic arms often form triangles to maintain stability and range of motion.
- Structural Frames: Triangular frames support various parts of robots, ensuring they can handle different loads and stresses.
7. Physics and Engineering Calculations
Triangle calculations are essential in physics and engineering to solve problems related to forces, motion, and energy.
- Force Analysis: Triangles help in breaking down and analyzing forces acting on structures.
- Statics and Dynamics: Triangular components are used to study the equilibrium and motion of bodies.
8. Agriculture and Forestry
In agriculture and forestry, triangles help in planning and managing land use effectively.
- Plot Division: Triangles are used to divide agricultural land for efficient planting and harvesting.
- Forest Mapping: Triangular calculations assist in mapping forest areas and planning conservation efforts.

Practice Problems
Applying the formulas for the area and perimeter of triangles to solve various problems can help reinforce your understanding. Here are several practice problems that range from basic to more advanced levels, including step-by-step solutions.
1. Basic Area and Perimeter Calculation
-
Problem 1: Find the area and perimeter of a triangle with sides \( a = 5 \, \text{cm} \), \( b = 6 \, \text{cm} \), and \( c = 7 \, \text{cm} \).
- Calculate the semi-perimeter \( s \): \[ s = \frac{a + b + c}{2} = \frac{5 + 6 + 7}{2} = 9 \, \text{cm} \]
- Use Heron's formula to find the area \( A \): \[ A = \sqrt{s(s-a)(s-b)(s-c)} = \sqrt{9(9-5)(9-6)(9-7)} = \sqrt{9 \times 4 \times 3 \times 2} = 6 \sqrt{6} \, \text{cm}^2 \]
- Calculate the perimeter \( P \): \[ P = a + b + c = 5 + 6 + 7 = 18 \, \text{cm}
-
Problem 2: Determine the area of a triangle with a base \( b = 8 \, \text{cm} \) and height \( h = 5 \, \text{cm} \).
- Use the area formula for a triangle: \[ A = \frac{1}{2} \times b \times h = \frac{1}{2} \times 8 \times 5 = 20 \, \text{cm}^2 \]
2. Finding Missing Side Lengths
-
Problem 3: A triangle has sides \( a = 10 \, \text{cm} \) and \( b = 24 \, \text{cm} \). The perimeter of the triangle is \( 60 \, \text{cm} \). Find the length of the third side \( c \).
- Use the perimeter formula: \[ P = a + b + c \]
- Substitute the known values and solve for \( c \): \[ 60 = 10 + 24 + c \implies c = 60 - 34 = 26 \, \text{cm} \]
3. Using the Pythagorean Theorem
-
Problem 4: A right-angled triangle has legs \( a = 9 \, \text{cm} \) and \( b = 12 \, \text{cm} \). Find the length of the hypotenuse \( c \).
- Apply the Pythagorean theorem: \[ c = \sqrt{a^2 + b^2} = \sqrt{9^2 + 12^2} = \sqrt{81 + 144} = \sqrt{225} = 15 \, \text{cm} \]
4. Advanced Applications
-
Problem 5: Given an equilateral triangle with a side length of \( 6 \, \text{cm} \), find its area.
- Use the formula for the area of an equilateral triangle: \[ A = \frac{\sqrt{3}}{4} a^2 = \frac{\sqrt{3}}{4} \times 6^2 = \frac{\sqrt{3}}{4} \times 36 = 9 \sqrt{3} \, \text{cm}^2 \]
5. Solving Real-world Problems
-
Problem 6: A triangular plot of land has sides \( a = 50 \, \text{m} \), \( b = 60 \, \text{m} \), and \( c = 70 \, \text{m} \). Calculate the area of the plot.
- First, find the semi-perimeter \( s \): \[ s = \frac{a + b + c}{2} = \frac{50 + 60 + 70}{2} = 90 \, \text{m} \]
- Then, use Heron's formula to find the area \( A \):
\[
A = \sqrt{s(s-a)(s-b)(s-c)} = \sqrt{90(90-50)(90-60)(90-70)} = \sqrt{90 \times 40 \times 30 \times 20}
\]
Simplifying inside the square root gives:
\[
A = \sqrt{90 \times 40 \times 30 \times 20} = \sqrt{2160000} = 147.32 \, \text{m}^2
\]
6. Mixed Problem Set
For additional practice, try solving these problems:
- Calculate the perimeter of a triangle with sides \( 8 \, \text{cm} \), \( 15 \, \text{cm} \), and \( 17 \, \text{cm} \).
- Find the area of a triangle with a base of \( 10 \, \text{cm} \) and a height of \( 7 \, \text{cm} \).
- Given a triangle with sides \( a = 9 \, \text{cm} \), \( b = 12 \, \text{cm} \), and \( c = 15 \, \text{cm} \), verify if it's a right triangle.
- Determine the height of an isosceles triangle with a base of \( 10 \, \text{cm} \) and equal sides of \( 13 \, \text{cm} \).
These problems are designed to enhance your understanding of triangle calculations. Keep practicing to master these concepts!
Conclusion
Triangles are not just basic geometric shapes; they are fundamental to various fields and applications in real life. From architectural marvels to the precision of land surveying, the calculations involving the area and perimeter of triangles provide essential insights and solutions.
In this comprehensive guide, we've explored:
- The basic properties and types of triangles, establishing a foundational understanding.
- The formulas for calculating the area and perimeter of different types of triangles.
- Practical examples and problems that illustrate how these formulas are applied in real-world contexts.
- Special triangle formulas, including Heron's formula and its usage in diverse scenarios.
- The significant role triangles play in fields such as architecture, navigation, and engineering.
Understanding the area and perimeter of triangles allows us to solve numerous practical problems. Whether it's designing a bridge, determining the boundaries of a plot of land, or navigating using GPS, these calculations are indispensable.
As you continue to apply these concepts, remember:
- Always verify the type of triangle you're dealing with to choose the appropriate formula.
- In complex problems, break them down into simpler triangular components to simplify your calculations.
- Use tools and resources like Mathjax to visualize and solve problems effectively.
By mastering these fundamental concepts, you'll be well-equipped to tackle a variety of mathematical challenges and appreciate the beauty and utility of triangles in both simple and complex scenarios.
Keep practicing and exploring the fascinating world of triangles. Each problem you solve will deepen your understanding and enhance your ability to apply these principles in practical and theoretical contexts.
Thank you for joining us on this journey through the area and perimeter of triangles. We hope this guide has provided valuable insights and a solid foundation for further exploration and learning.
Cách Tìm Diện Tích và Chu Vi của Tam Giác | Toán Học với Thầy J
How to Find the Area and Perimeter of a Triangle
Cách Tìm Chu Vi của Tam Giác