Topic 5 square root of 10: Discover the fascinating world of mathematics with our in-depth exploration of 5 square root of 10. Learn how to simplify, calculate, and apply this mathematical expression in various contexts. Dive into practical examples and enhance your understanding of square roots and their significance in everyday life.
Table of Content
- Search Results for "5 square root of 10"
- Introduction to Square Roots
- Understanding the Square Root of 10
- Methods to Calculate Square Roots
- Applications of Square Root of 10
- Simplifying Expressions Involving Square Root of 10
- FAQs on Square Root of 10
- YOUTUBE: Hướng dẫn cách nhân căn bậc 2 của 5 với căn bậc 2 của 10 một cách dễ hiểu và chính xác.
Search Results for "5 square root of 10"
The square root of 10 is approximately 3.162. When multiplied by 5, it equals:
\[ 5 \sqrt{10} \approx 5 \times 3.162 = 15.81 \]

READ MORE:
Introduction to Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 9 is 3, because \(3 \times 3 = 9\). The square root symbol is represented by \(\sqrt{}\).
Square roots have several important properties and applications in various fields of mathematics and science. Understanding how to work with square roots is fundamental for solving quadratic equations, understanding geometric properties, and more.
Here are some key properties of square roots:
- For any positive number \(a\), the square root of \(a\) is a number \(b\) such that \(b^2 = a\).
- The square root of a negative number is not a real number; instead, it is an imaginary number.
- The square root of zero is zero: \(\sqrt{0} = 0\).
- Square roots of non-perfect squares are irrational numbers, meaning they cannot be expressed as a simple fraction.
Square roots can be represented in different forms, including the radical form \(\sqrt{a}\) and the exponent form \(a^{1/2}\). These representations are used interchangeably depending on the context.
To better understand square roots, consider the following table of common square roots:
| Number | Square Root |
|---|---|
| 1 | \(\sqrt{1} = 1\) |
| 4 | \(\sqrt{4} = 2\) |
| 9 | \(\sqrt{9} = 3\) |
| 16 | \(\sqrt{16} = 4\) |
| 25 | \(\sqrt{25} = 5\) |
With this basic understanding of square roots, we can explore more specific examples and applications, such as the square root of 10.
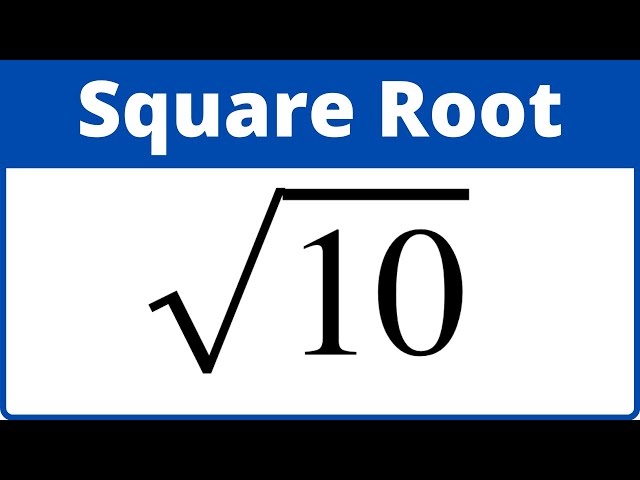
Understanding the Square Root of 10
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root of 10, denoted as \( \sqrt{10} \), is an important irrational number in mathematics.
Definition and Properties
The square root of 10 is an irrational number, which means it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating. In radical form, it is represented as \( \sqrt{10} \).
The approximate value of \( \sqrt{10} \) is 3.162. This value is derived using various mathematical methods such as approximation and the long division method.
Decimal Representation
When expressed in decimal form, the square root of 10 is approximately 3.162277660168379. For practical purposes, this is often rounded to 3.162.
Radical Form
In radical form, the square root of 10 is represented simply as \( \sqrt{10} \). This can also be broken down into the product of the square roots of its prime factors:
- \( 10 = 2 \times 5 \)
- \( \sqrt{10} = \sqrt{2 \times 5} = \sqrt{2} \times \sqrt{5} \)
Using approximate values for the square roots of 2 and 5:
\( \sqrt{10} \approx 1.414 \times 2.236 = 3.162 \)
Calculation Methods
There are several methods to calculate the square root of 10:
- Long Division Method: This is a manual method to find square roots which provides an approximate value of 3.162 for \( \sqrt{10} \).
- Approximation Method: By recognizing that \( \sqrt{10} \) lies between 3 and 4, we can narrow down its value through iterative approximations.
- Prime Factorization: By expressing 10 as the product of prime numbers and then taking the square root of each factor, we can determine \( \sqrt{10} = \sqrt{2 \times 5} = \sqrt{2} \times \sqrt{5} \).
While these methods provide different approaches, they converge on the same approximate value for the square root of 10.
Methods to Calculate Square Roots
Calculating square roots can be approached through various methods. Here, we outline some common techniques that can be used to find the square root of a number, including the square root of 10.
Long Division Method
The long division method is a systematic approach to finding square roots, especially for numbers that are not perfect squares. Here are the steps to calculate the square root of 10 using the long division method:
- Pair the digits of the number starting from the decimal point. For 10, we have 10.00.
- Find the largest number whose square is less than or equal to the first pair. For 10, this is 3 (since 32 = 9).
- Subtract the square of this number from the first pair, bring down the next pair of digits (00), and double the divisor.
- Estimate the next digit of the quotient, which when added to the divisor and multiplied by the new digit, gives a product less than or equal to the current dividend. Repeat the process until the desired precision is achieved.
Approximation Method
The approximation method involves using known square roots to estimate the value. For example, to approximate the square root of 10:
- Note that \( \sqrt{9} = 3 \) and \( \sqrt{16} = 4 \), so \( \sqrt{10} \) is between 3 and 4.
- Refine the estimate using averages. For instance, \( \sqrt{10} \approx 3.16 \) by iterative approximation.
Prime Factorization
Prime factorization involves expressing the number as a product of prime numbers and simplifying the square root of those products. Here’s how you can do it for 10:
- Prime factorize 10: \( 10 = 2 \times 5 \).
- Express the square root in terms of these factors: \( \sqrt{10} = \sqrt{2 \times 5} \).
- Since \( \sqrt{2} \approx 1.414 \) and \( \sqrt{5} \approx 2.236 \), \( \sqrt{10} \approx 1.414 \times 2.236 = 3.162 \).
Using these methods, you can find the square root of 10 to various degrees of precision depending on the technique employed.
Applications of Square Root of 10
The square root of 10 (\(\sqrt{10}\)) finds applications in various fields due to its mathematical properties and the prevalence of square roots in practical calculations. Here are some significant applications:
Mathematical Problems
- Geometry: In geometry, the square root of 10 can be used to calculate the lengths of diagonals in rectangular shapes and to solve problems involving right triangles using the Pythagorean theorem. For instance, the length of the diagonal \(d\) of a rectangle with side lengths \(a\) and \(b\) is given by \(d = \sqrt{a^2 + b^2}\). When the sum of the squares of the sides equals 10, \(d = \sqrt{10}\).
- Algebra: Square roots are essential in solving quadratic equations. The quadratic formula \(x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\) often involves calculating square roots, where the discriminant (\(b^2 - 4ac\)) might be equal to 10.
Real-Life Examples
- Engineering and Construction: Square roots are used to calculate diagonal lengths in construction and engineering designs to ensure structural integrity. For example, a builder might need to calculate the diagonal brace length in a structure where the sides form a right triangle with the area involved leading to \(\sqrt{10}\) as part of the calculation.
- Physics: In physics, square roots are used to determine quantities like the standard deviation in statistical mechanics or the root-mean-square speed of gas molecules, where \(\sqrt{10}\) might appear in derived formulas.
- Finance: The concept of volatility in finance, which measures the rate of change of asset prices, often involves taking the square root of time-variance data, and \(\sqrt{10}\) can come up in these calculations when dealing with specific data sets.
- Navigation: The distance formula in navigation uses square roots to calculate the shortest path between two points. For example, the distance \(D\) between two points \((x_1, y_1)\) and \((x_2, y_2)\) in a plane is given by \(D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\). If the coordinates lead to a sum of squares that equals 10, then the distance is \(\sqrt{10}\).
- Statistics: In statistics, the calculation of standard deviation, which is the square root of the variance, is crucial for understanding data dispersion. For data sets where the variance sums up to 10, the standard deviation would be \(\sqrt{10}\).

Simplifying Expressions Involving Square Root of 10
When working with expressions involving the square root of 10, there are several methods to simplify them effectively. These methods include rationalizing the denominator, dealing with products and quotients of radicals, and combining square roots. Let's explore these methods in detail.
Rationalizing the Denominator
To simplify an expression with a square root in the denominator, you can rationalize the denominator. This involves multiplying both the numerator and the denominator by a radical that will eliminate the square root in the denominator.
- Example: Simplify \(\frac{5}{\sqrt{10}}\).
- Multiply the numerator and the denominator by \(\sqrt{10}\): \[ \frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5 \sqrt{10}}{10} = \frac{\sqrt{10}}{2}. \]
Product and Quotient of Radicals
The product and quotient rules for radicals can help simplify expressions involving the square root of 10.
- Product of Radicals: \(\sqrt{a} \times \sqrt{b} = \sqrt{a \times b}\)
- Example: \(\sqrt{2} \times \sqrt{10} = \sqrt{20} = 2 \sqrt{5}\)
- Quotient of Radicals: \(\frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}}\)
- Example: \(\frac{\sqrt{10}}{\sqrt{2}} = \sqrt{5}\)
Combining Square Roots
When adding or subtracting square roots, combine like terms if possible.
- Example: Combine \(2\sqrt{10} + 3\sqrt{10}\): \[ 2\sqrt{10} + 3\sqrt{10} = 5\sqrt{10}. \]
- If the radicals are not like terms, they cannot be combined directly: \[ \sqrt{10} + \sqrt{5} \neq \sqrt{15}. \]
Using these techniques, you can simplify expressions involving the square root of 10 more effectively, making them easier to work with in mathematical problems and real-life applications.
FAQs on Square Root of 10
-
Why is Square Root of 10 Irrational?
The square root of 10 is irrational because it cannot be expressed as a fraction of two integers. The decimal representation of \(\sqrt{10}\) is non-terminating and non-repeating, approximately equal to 3.162277660168379.
-
How to Simplify \(\frac{5}{\sqrt{10}}\)?
To simplify \(\frac{5}{\sqrt{10}}\), we rationalize the denominator:
- Multiply the numerator and the denominator by \(\sqrt{10}\): \(\frac{5}{\sqrt{10}} \times \frac{\sqrt{10}}{\sqrt{10}} = \frac{5\sqrt{10}}{10}\).
- Simplify the fraction: \(\frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2}\).
What is \(12\sqrt{10}\)?
To simplify \(12\sqrt{10}\), recognize that it is the product of 12 and the square root of 10:
- Express the result: \(12 \times \sqrt{10}\).
- Since \(\sqrt{10} \approx 3.162\), calculate the product: \(12 \times 3.162 \approx 37.944\).
Hướng dẫn cách nhân căn bậc 2 của 5 với căn bậc 2 của 10 một cách dễ hiểu và chính xác.
Nhân căn bậc 2 của 5 với căn bậc 2 của 10 || Nhân các căn bậc
READ MORE:
Hướng dẫn cách xấp xỉ căn bậc hai của một số để giúp bạn tính toán nhanh chóng và chính xác.
Cách Xấp Xỉ Căn Bậc Hai của Một Số