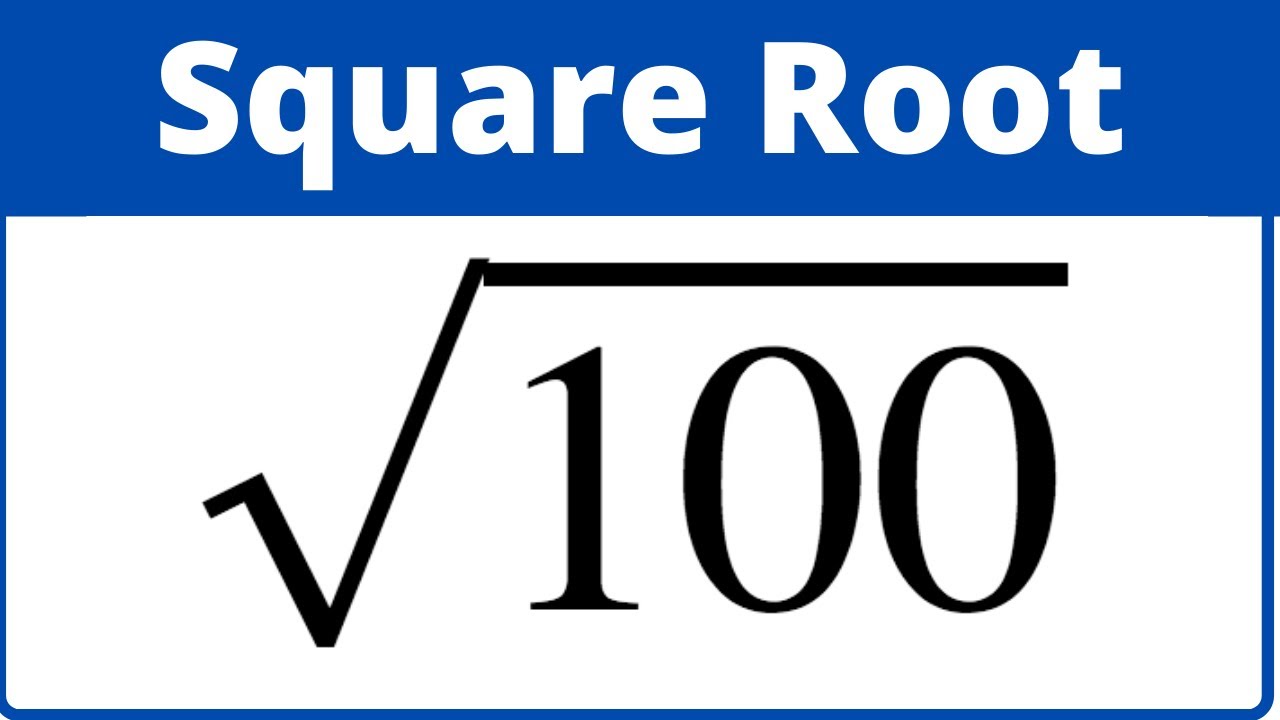
Topic the square root of 100: The square root of 100 is a fascinating mathematical concept that simplifies into the whole number 10. Understanding this perfect square opens doors to various applications in geometry, algebra, and real-life scenarios. Dive into the world of square roots and discover the elegance and simplicity behind the number 100.
Table of Content
- The Square Root of 100
- Introduction to Square Roots
- Understanding the Square Root of 100
- Definition and Basic Concepts
- Mathematical Representation
- Calculation Method
- Properties of the Square Root of 100
- Practical Examples and Applications
- Comparing Square Roots of Different Numbers
- Common Misconceptions
- Square Roots in Geometry
- Square Roots in Real Life
- Advanced Topics
- Historical Context
- Conclusion and Summary
- YOUTUBE:
The Square Root of 100
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root of 100 is one such value.
Definition
The square root of 100 is written as \( \sqrt{100} \). In mathematical terms, this is the number \( x \) such that \( x \times x = 100 \).
Calculation
To find the square root of 100, we can use the following equation:
\[
\sqrt{100} = x \quad \text{where} \quad x^2 = 100
\]
Solving for \( x \), we find:
\[
x = 10 \quad \text{since} \quad 10 \times 10 = 100
\]
Therefore, \( \sqrt{100} = 10 \).
Properties
- The square root of 100 is a rational number.
- It is an integer.
- The square root of 100 is a perfect square.
Examples
Here are some practical examples involving the square root of 100:
- If you have a square with an area of 100 square units, each side of the square will be \( \sqrt{100} = 10 \) units long.
- In geometry, the distance formula between two points (0, 0) and (10, 0) on a coordinate plane is \( \sqrt{(10-0)^2} = 10 \).
- If you split a group of 100 items into 10 equal parts, each part will have \( \sqrt{100} = 10 \) items.
Square Roots of Other Numbers
For comparison, here are the square roots of some other numbers:
| Number | Square Root |
| 64 | 8 |
| 81 | 9 |
| 121 | 11 |
| 144 | 12 |
Conclusion
The square root of 100 is an important and commonly encountered value in mathematics. It is an integer (10) and a perfect square, making it a simple yet significant example of square roots.
READ MORE:
Introduction to Square Roots
Square roots are fundamental mathematical operations that find the original value which, when multiplied by itself, produces the given number. They are denoted using the radical symbol \( \sqrt{} \). For example, \( \sqrt{100} \) equals 10 because \( 10 \times 10 = 100 \).
The concept of square roots is essential in various branches of mathematics, including algebra, geometry, and calculus. Understanding square roots helps in solving quadratic equations, analyzing geometric shapes, and simplifying complex mathematical expressions.
Here are some key points about square roots:
- Square roots can be positive or negative because both \( 10 \times 10 \) and \( -10 \times -10 \) equal 100.
- Not all numbers have integer square roots. For example, \( \sqrt{2} \) is an irrational number.
- Perfect squares are numbers that have integer square roots, such as 1, 4, 9, 16, 25, 36, 49, 64, 81, and 100.
To further illustrate, let's look at some examples of perfect squares and their square roots:
| Number | Square Root |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
| 36 | 6 |
| 49 | 7 |
| 64 | 8 |
| 81 | 9 |
| 100 | 10 |
Square roots also play a crucial role in real-world applications, such as calculating areas, understanding proportions, and solving physical problems. Mastery of square roots is not only important for academic success but also for practical problem-solving in everyday life.
Understanding the Square Root of 100
The square root of 100 is a fundamental concept in mathematics. It is the number which, when multiplied by itself, results in 100. Mathematically, this can be expressed as:
\[
\sqrt{100} = x \quad \text{where} \quad x^2 = 100
\]
To find the square root of 100, we need to determine the value of \( x \) that satisfies the equation \( x^2 = 100 \). Solving for \( x \), we find:
\[
x = 10 \quad \text{because} \quad 10 \times 10 = 100
\]
Therefore, the square root of 100 is 10. This value is significant for several reasons:
- It is a perfect square: 100 is a perfect square, meaning its square root is an integer.
- Positive and negative roots: Both \( 10 \) and \( -10 \) are square roots of 100, as \( (-10) \times (-10) = 100 \).
- Simplicity: The number 10 is an easy-to-understand, whole number, making it straightforward to use in calculations.
Here is a step-by-step breakdown of calculating the square root of 100:
- Start with the equation: \( x^2 = 100 \).
- Identify the number that, when squared, equals 100.
- Recognize that \( 10 \times 10 = 100 \).
- Thus, \( \sqrt{100} = 10 \).
To better understand the concept, consider these practical examples:
- Geometry: If you have a square with an area of 100 square units, each side of the square will be \( \sqrt{100} = 10 \) units long.
- Measurement: In real life, if a room has an area of 100 square feet, the length of each side would be 10 feet if it were a square.
Additionally, understanding the square root of 100 can help with solving quadratic equations. For example, the quadratic equation \( x^2 - 100 = 0 \) has solutions \( x = 10 \) and \( x = -10 \), showing both positive and negative roots.
In summary, the square root of 100 is a key mathematical concept that is not only easy to compute but also widely applicable in various fields of study and everyday situations.
Definition and Basic Concepts
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root operation is denoted by the radical symbol \( \sqrt{} \). For example, the square root of 100 is represented as \( \sqrt{100} \).
Mathematically, the square root of 100 is defined as the number \( x \) such that:
\[
x^2 = 100
\]
Solving for \( x \), we find that:
\[
x = 10 \quad \text{because} \quad 10 \times 10 = 100
\]
Thus, \( \sqrt{100} = 10 \). This illustrates that the square root of 100 is 10. However, it is important to note that both positive and negative values can be square roots. Hence, the complete solution includes both \( 10 \) and \( -10 \):
\[
\sqrt{100} = \pm 10
\]
Key concepts related to square roots include:
- Perfect Squares: Numbers like 100 that have integer square roots are called perfect squares. Other examples include 1, 4, 9, 16, 25, and so on.
- Principal Square Root: The non-negative square root of a number is referred to as the principal square root. For 100, the principal square root is 10.
- Radical Symbol: The symbol \( \sqrt{} \) is used to denote the square root operation. For instance, \( \sqrt{100} = 10 \).
- Rational and Irrational Numbers: The square root of a perfect square is a rational number. However, the square root of a non-perfect square, such as 2, is an irrational number (cannot be expressed as a simple fraction).
To further illustrate, consider the following examples of perfect squares and their square roots:
| Number | Square Root |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
| 36 | 6 |
| 49 | 7 |
| 64 | 8 |
| 81 | 9 |
| 100 | 10 |
Understanding the definition and basic concepts of square roots is essential for solving a variety of mathematical problems. Square roots are widely used in equations, geometric calculations, and real-life applications, making them a fundamental aspect of mathematical knowledge.
Mathematical Representation
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root of 100 can be represented mathematically as follows:
- The square root symbol is √.
- The square root of 100 is written as √100.
Using Mathjax, the mathematical representation of the square root of 100 can be written as:
\[\sqrt{100}\]
The number 100 is a perfect square, which means it can be expressed as the product of an integer with itself. For 100, this integer is 10:
\[100 = 10 \times 10\]
Therefore, the square root of 100 is:
\[\sqrt{100} = 10\]
We can also represent this in the context of an equation:
\[x^2 = 100\]
Solving for \(x\), we get:
\[x = \sqrt{100} = 10\]
Here are the steps to find the square root of 100:
- Identify the number for which you want to find the square root (in this case, 100).
- Determine if the number is a perfect square. Since 100 is \(10 \times 10\), it is a perfect square.
- Write the number as the product of its square root: \(100 = 10^2\).
- Extract the square root from the equation: \(\sqrt{100} = 10\).
In conclusion, the square root of 100 is simply 10, and it can be mathematically represented as \(\sqrt{100} = 10\).

Calculation Method
To calculate the square root of 100, we can follow several methods. Below, we outline some common and easy-to-understand methods:
Method 1: Prime Factorization
Prime factorization involves breaking down the number into its prime factors and then simplifying.
- Find the prime factors of 100: \(100 = 2^2 \times 5^2\).
- Take the square root of each prime factor: \(\sqrt{100} = \sqrt{2^2 \times 5^2}\).
- Simplify the expression by taking the square root of each factor: \(\sqrt{2^2} \times \sqrt{5^2} = 2 \times 5 = 10\).
Thus, the square root of 100 is 10.
Method 2: Using the Long Division Method
The long division method is a manual method that involves the following steps:
- Group the digits of the number in pairs, starting from the decimal point. For 100, the pairs are (1, 00).
- Find the largest number whose square is less than or equal to the first pair. For 1, the largest number is 1 because \(1^2 = 1\).
- Subtract the square of this number from the first pair and bring down the next pair. Here, \(1 - 1 = 0\) and bring down 00 to get 00.
- Double the quotient obtained in step 2 (which is 1), and write it down with a space next to it (making it 2_). Find a number (x) such that \(2x \times x \leq 0\). Here, the number is 0 because \(20 \times 0 = 0\).
- Continue this process until all pairs are used up.
The quotient obtained through this method will be the square root. In this case, the square root of 100 is 10.
Method 3: Using a Calculator
The most straightforward method is to use a calculator:
- Simply enter the number 100 into the calculator.
- Press the square root (√) button.
- The calculator will display 10 as the result.
Mathematical Representation
The square root of 100 can be represented mathematically as:
\[
\sqrt{100} = 10
\]
Visual Representation
We can visualize the square root of 100 using a table:
| Number | Square Root |
|---|---|
| 100 | 10 |
| 10 | 3.162 (approx) |
| 4 | 2 |
| 1 | 1 |
From the table, we can see that the square root of 100 is a whole number, 10.
Using these methods, you can understand and calculate the square root of 100 effectively.
Properties of the Square Root of 100
The square root of 100 has several interesting properties that make it a notable number in mathematics. Here are some key properties:
1. Perfect Square
100 is a perfect square, meaning it is the product of an integer multiplied by itself:
\[
100 = 10 \times 10 \implies \sqrt{100} = 10
\]
2. Non-Negative Real Number
The square root of a non-negative number is also non-negative. Thus, the square root of 100 is a non-negative real number:
\[
\sqrt{100} = 10 \geq 0
\]
3. Rational Number
The square root of 100 is a rational number because it can be expressed as the ratio of two integers:
\[
\sqrt{100} = \frac{10}{1} = 10
\]
4. Multiplicative Property
The square root function has the property that the square root of a product is the product of the square roots:
\[
\sqrt{100} = \sqrt{10^2} = 10
\]
5. Square Root of a Perfect Square
Since 100 is a perfect square, its square root is an integer:
\[
\sqrt{100} = 10
\]
6. Even Integer
10 is an even integer, meaning it is divisible by 2:
\[
10 \div 2 = 5
\]
7. Relationship with Powers of 10
100 is \(10^2\), which shows the relationship between square roots and exponents:
\[
\sqrt{10^2} = 10
\]
8. Application in Area Calculation
The square root of 100 can be applied in geometry to find the side length of a square with an area of 100 square units:
\[
\text{Area} = 100 \implies \text{Side length} = \sqrt{100} = 10
\]
9. Properties in Coordinate Geometry
In coordinate geometry, the distance formula uses square roots. The distance between points \((0, 0)\) and \((10, 0)\) is 10 units, which is the square root of 100:
\[
\sqrt{(10 - 0)^2 + (0 - 0)^2} = \sqrt{100} = 10
\]
10. Practical Application in Measurement
In practical applications, the square root of 100 can be used in measurements and conversions. For example, if the area of a square is 100 square meters, each side will measure 10 meters:
\[
\text{Side length} = \sqrt{100} = 10 \text{ meters}
\]
These properties highlight the significance and versatility of the square root of 100 in various mathematical and practical contexts.
Practical Examples and Applications
The square root of 100, which is 10, has various practical applications in everyday life and different fields. Below are some examples and applications:
1. Geometry and Area Calculation
In geometry, the square root is used to determine the side length of a square when the area is known:
If the area of a square is 100 square units, the side length is:
\[
\text{Side length} = \sqrt{100} = 10 \text{ units}
\]
2. Construction and Design
Architects and engineers use square roots to calculate dimensions. For example, to find the length of a side of a square plot of land with an area of 100 square meters:
\[
\text{Side length} = \sqrt{100} = 10 \text{ meters}
\]
3. Measurement Conversions
In measurement conversions, the square root helps in scaling. For instance, a square tile with an area of 100 square centimeters has a side length of:
\[
\text{Side length} = \sqrt{100} = 10 \text{ centimeters}
\]
4. Physics and Engineering
In physics, the square root function is used to calculate quantities such as root mean square (RMS) values. For example, if the RMS voltage is given by:
\[
\text{RMS voltage} = \sqrt{\text{sum of squared voltages}/\text{number of voltages}} = \sqrt{100} = 10 \text{ volts}
\]
5. Financial Calculations
In finance, the square root is used to calculate the standard deviation, which measures the amount of variation or dispersion of a set of values. If the variance is 100, the standard deviation is:
\[
\text{Standard deviation} = \sqrt{\text{Variance}} = \sqrt{100} = 10
\]
6. Distance Measurement
In coordinate geometry, the square root is used in the distance formula to find the distance between two points. For example, the distance between the points \((0, 0)\) and \((10, 0)\) is:
\[
\text{Distance} = \sqrt{(10 - 0)^2 + (0 - 0)^2} = \sqrt{100} = 10 \text{ units}
\]
7. Data Analysis and Statistics
In statistics, the square root is used to calculate the standard deviation and variance of data sets. If a data set has a variance of 100, the standard deviation is:
\[
\text{Standard deviation} = \sqrt{100} = 10
\]
8. Resistor and Capacitor Values
In electronics, the square root is used to calculate impedance and resonance frequencies. For example, the resonant frequency in an LC circuit where \( L = 1 \) H and \( C = 0.01 \) F is:
\[
f_r = \frac{1}{2\pi\sqrt{LC}} = \frac{1}{2\pi\sqrt{1 \times 0.01}} = \frac{1}{2\pi \times 0.1} = \frac{1}{0.628} \approx 1.59 \text{ Hz}
\]
These examples highlight the practical applications of the square root of 100 in various fields, demonstrating its importance in solving real-world problems.
Comparing Square Roots of Different Numbers
Comparing square roots of different numbers can help us understand their relative sizes and properties. Here, we will compare the square root of 100 with the square roots of other significant numbers.
Square Roots of Perfect Squares
Perfect squares are numbers that have whole numbers as their square roots. Let's compare the square roots of some perfect squares:
| Number | Square Root | Calculation |
|---|---|---|
| 1 | 1 | \(\sqrt{1} = 1\) |
| 4 | 2 | \(\sqrt{4} = 2\) |
| 9 | 3 | \(\sqrt{9} = 3\) |
| 16 | 4 | \(\sqrt{16} = 4\) |
| 25 | 5 | \(\sqrt{25} = 5\) |
| 36 | 6 | \(\sqrt{36} = 6\) |
| 49 | 7 | \(\sqrt{49} = 7\) |
| 64 | 8 | \(\sqrt{64} = 8\) |
| 81 | 9 | \(\sqrt{81} = 9\) |
| 100 | 10 | \(\sqrt{100} = 10\) |
From the table, we see that the square root of 100 (which is 10) is larger than the square roots of smaller perfect squares like 1, 4, 9, etc., and smaller than the square roots of larger perfect squares like 121 (11), 144 (12), and so on.
Square Roots of Non-Perfect Squares
Non-perfect squares are numbers that do not have whole numbers as their square roots. Here are some examples:
| Number | Square Root | Approximate Value |
|---|---|---|
| 2 | \(\sqrt{2}\) | 1.414 |
| 3 | \(\sqrt{3}\) | 1.732 |
| 5 | \(\sqrt{5}\) | 2.236 |
| 10 | \(\sqrt{10}\) | 3.162 |
| 20 | \(\sqrt{20}\) | 4.472 |
| 50 | \(\sqrt{50}\) | 7.071 |
Comparing these values with \(\sqrt{100} = 10\), we observe that the square root of 100 is significantly larger than those of non-perfect squares like 2, 3, 5, and even 50.
Visual Comparison
A visual comparison can help us better understand the relative sizes of these square roots. Below is a bar chart representation:
| Number | Square Root | Visual Representation |
|---|---|---|
| 1 | 1 | ■ |
| 4 | 2 | ■■ |
| 9 | 3 | ■■■ |
| 16 | 4 | ■■■■ |
| 25 | 5 | ■■■■■ |
| 36 | 6 | ■■■■■■ |
| 49 | 7 | ■■■■■■■ |
| 64 | 8 | ■■■■■■■■ |
| 81 | 9 | ■■■■■■■■■ |
| 100 | 10 | ■■■■■■■■■■ |
From this chart, we can clearly see how the square root of 100 compares to other square roots in a visual format.
By comparing the square roots of different numbers, we gain a better understanding of their relative sizes and how they are used in various mathematical and practical contexts.

Common Misconceptions
There are several misconceptions surrounding the square root of 100:
- It's only a single number: Some people think the square root of 100 is just 10, but it's actually ±10 because both -10 and 10 squared give 100.
- It's the only square root of 100: While 10 is the principal square root of 100, -10 is also a valid square root since (-10)^2 = 100.
- It's an irrational number: Contrary to popular belief, the square root of 100 is rational because it equals a whole number.
- It has no practical use: Square roots, including √100, are used extensively in various fields such as mathematics, engineering, and physics for calculations and problem-solving.
- It's the only number with two square roots: Many numbers have two square roots, including 100. The positive and negative roots are equally valid.
- It's just about multiplication: Understanding square roots involves more than just multiplication; it's about finding a number that, when squared, equals the original number.
These misconceptions often arise from oversimplifications or incomplete understanding of mathematical principles related to square roots.
Square Roots in Geometry
In geometry, the square root of 100 plays a significant role, particularly in the context of squares and areas:
- Area of a Square: Given a square with side length 10 units, the area can be calculated as \( 10 \times 10 = 100 \) square units. The side length, 10 units, is the square root of 100.
- Diagonal of a Square: The diagonal of a square whose side length is 10 units can be found using the formula \( \sqrt{2} \times \text{side length} = \sqrt{2} \times 10 = 10\sqrt{2} \) units.
- Pythagorean Theorem: In right triangles where the legs are each 10 units long, the hypotenuse (diagonal of the square) measures \( 10\sqrt{2} \) units, confirming the relationship between squares, triangles, and square roots.
- Geometric Constructions: Understanding square roots aids in geometric constructions involving squares and their properties, such as inscribing and circumscribing circles.
These applications illustrate how the square root of 100 is not only a numerical value but also a fundamental concept in geometrical calculations and constructions.
Square Roots in Real Life
The square root of 100 has practical applications in everyday life beyond mathematical exercises:
- Measurement: When measuring a square plot of land with an area of 100 square units, each side would measure 10 units, which is the square root of 100.
- Engineering: In construction and engineering, understanding square roots is essential for calculating dimensions, such as the length of sides or diagonals in square structures.
- Finance: Square roots are used in financial calculations, such as calculating compound interest rates or assessing risk in investments.
- Technology: Square roots are employed in various technological applications, from programming algorithms to signal processing and cryptography.
- Physics: Concepts involving energy, motion, and forces often use square roots in equations and measurements.
- Medicine: Medical imaging techniques, such as ultrasound, utilize square roots in processing signals and interpreting data.
Understanding the square root of 100 and its applications in real-life scenarios highlights its relevance and utility beyond theoretical mathematics.
Advanced Topics
When exploring advanced topics related to the square root of 100, we delve into more complex mathematical concepts and applications. Here, we cover a range of topics from imaginary numbers to applications in various fields.
Imaginary and Complex Numbers
While the square root of 100 is straightforward, yielding ±10, the concept extends into the realm of imaginary numbers when dealing with negative values. The square root of a negative number introduces the imaginary unit \(i\), where \(i = \sqrt{-1}\). Therefore, the square root of -100 is expressed as:
\[
\sqrt{-100} = \sqrt{100 \cdot -1} = 10i
\]
This concept is foundational in complex number theory, where numbers are expressed in the form \(a + bi\), with \(a\) and \(b\) being real numbers.
Quadratic Equations
Square roots play a crucial role in solving quadratic equations. For example, consider the quadratic equation:
\[
x^2 - 100 = 0
\]
By solving for \(x\), we get:
\[
x = \pm \sqrt{100} = \pm 10
\]
This shows the roots of the equation are 10 and -10. Quadratic equations are fundamental in various mathematical fields, including algebra and calculus.
Higher-Dimensional Geometry
In geometry, square roots are used to determine distances and dimensions. For instance, the length of the diagonal of a square with side length \(a\) is given by:
\[
\text{Diagonal} = a\sqrt{2}
\]
For a square with a side length of 10 (where \(a = 10\)), the diagonal is:
\[
10\sqrt{2} \approx 14.14
\]
Applications in Real Life
Square roots have practical applications in various fields such as physics, engineering, and computer science. For instance, in physics, they are used to calculate root mean square values in alternating current (AC) circuits.
Advanced Mathematical Techniques
- Iterative Methods: Methods such as the Newton-Raphson method are used to approximate square roots for non-perfect squares.
- Prime Factorization: The square root of 100 can be derived by prime factorization: \(100 = 2^2 \times 5^2\), and hence \(\sqrt{100} = 10\).
- Infinite Series: Series expansion techniques can also be used to approximate square roots.
Historical Context
The concept of square roots dates back to ancient civilizations, with significant contributions from Greek mathematicians like Euclid. The symbol \(\sqrt{\ }\) (radical) was introduced in the 16th century by mathematician Christoph Rudolff.
Conclusion
Understanding the square root of 100 opens up a range of advanced mathematical topics. From imaginary numbers to applications in quadratic equations and geometry, the concept of square roots is a cornerstone of both pure and applied mathematics.

Historical Context
The concept of square roots has a rich and diverse history, spanning several ancient civilizations and mathematical traditions. The square root of 100, specifically, has been understood and utilized for thousands of years.
Ancient Civilizations
- Babylonia: The Babylonians, around 1800 BC, had advanced knowledge of mathematics, as evidenced by the YBC 7289 clay tablet, which demonstrates an approximation of the square root of 2.
- Egypt: The Rhind Mathematical Papyrus (circa 1650 BC) shows that the Egyptians used an inverse proportion method to calculate square roots, demonstrating their practical approach to solving mathematical problems.
- India: Indian mathematicians around 800-500 BC, as seen in the Sulba Sutras, developed techniques for approximating square roots. Notably, the Baudhayana Sulba Sutra provides methods for calculating the square roots of numbers like 2 and 3 with remarkable accuracy.
Greek Mathematics
The ancient Greeks made significant contributions to the understanding of square roots. Around 380 BC, Theaetetus proved that the square roots of non-square integers are irrational, a fundamental theorem in number theory. This work laid the groundwork for later developments in algebra and number theory.
Medieval and Renaissance Europe
During the medieval period, Islamic mathematicians translated and expanded upon Greek works, preserving and enhancing knowledge about square roots. In the Renaissance, European mathematicians like Leonardo of Pisa (Fibonacci) reintroduced these concepts to Europe, further developing algebraic methods to calculate square roots.
Modern Era
In contemporary mathematics, the understanding of square roots is essential in various fields, from algebra to calculus and beyond. The exact square root of 100, known to be 10, is a simple yet foundational concept used extensively in both theoretical and applied mathematics.
Conclusion and Summary
The square root of 100, which is exactly 10, offers a straightforward yet profound insight into various mathematical principles and applications. Throughout this article, we have explored multiple facets of this seemingly simple number, revealing its significance in both theoretical and practical contexts.
- Definition and Basic Concepts: The square root of 100, denoted as \( \sqrt{100} \), is 10. This is because 10 multiplied by itself (10 × 10) equals 100.
- Mathematical Representation: Mathematically, \( \sqrt{100} = 10 \). This integer value simplifies many calculations, especially those involving perfect squares.
- Calculation Method: The square root of 100 can be derived through various methods, including prime factorization, where 100 is expressed as \( 2^2 \times 5^2 \), and taking the square root of these factors gives \( 2 \times 5 = 10 \).
- Properties: As a perfect square, 100’s square root is a whole number, making it a rational number. This property distinguishes it from non-perfect squares, which yield irrational square roots.
- Practical Applications: Understanding the square root of 100 is essential in real-world scenarios such as determining the side length of a square with an area of 100 square units, or in finance for calculating compound interest where square roots are frequently used.
- Comparisons and Misconceptions: Unlike non-perfect squares, the square root of 100 does not require approximation. Common misconceptions include the confusion between squaring a number and taking its square root, which this article clarifies.
- Geometric and Real-Life Contexts: In geometry, knowing the square root of 100 helps in understanding the properties of shapes and forms. In everyday life, it simplifies calculations related to measurements and quantities.
In conclusion, the square root of 100 is a fundamental mathematical concept that extends beyond simple arithmetic. It underpins a variety of mathematical theories and real-world applications, demonstrating the elegance and utility of mathematics in everyday life.
Căn bậc hai của 100
READ MORE:
Làm thế nào để đơn giản hóa căn bậc hai của 100: sqrt(100)